bb8e92a8748069115c151af4f17f81f1.ppt
- Количество слайдов: 60







Example 1 A: Applying the Perpendicular Bisector Theorem and Its Converse
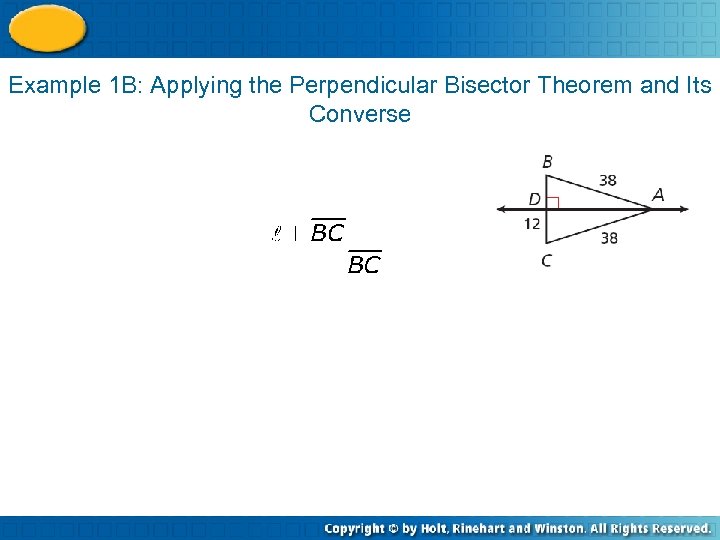
Example 1 B: Applying the Perpendicular Bisector Theorem and Its Converse
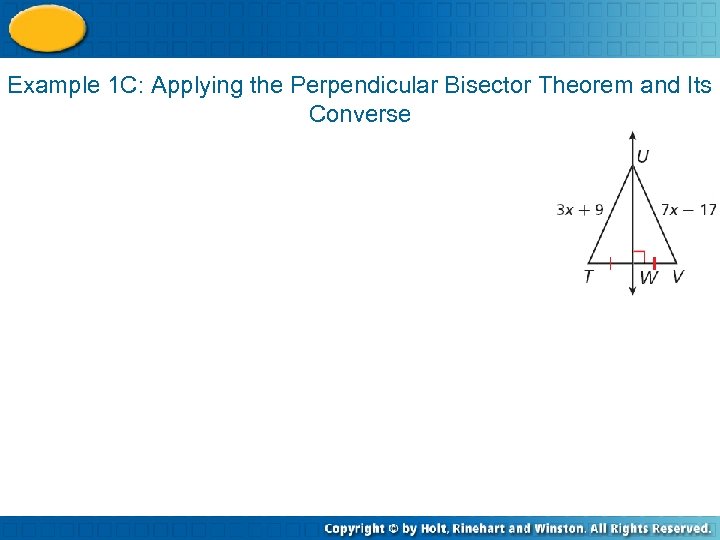
Example 1 C: Applying the Perpendicular Bisector Theorem and Its Converse
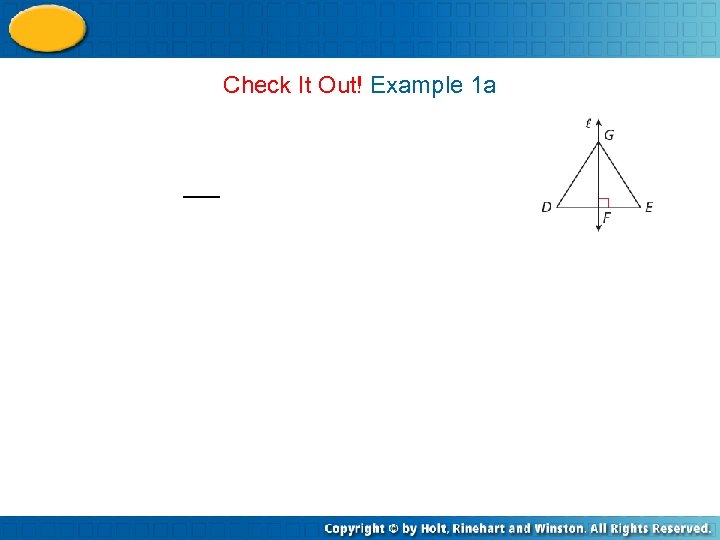
Check It Out! Example 1 a
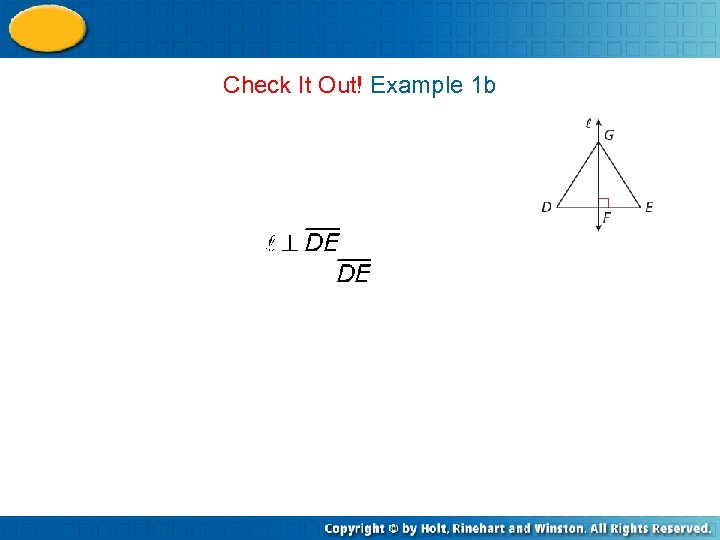
Check It Out! Example 1 b

Remember that the distance between a point and a line is the length of the perpendicular segment from the point to the line.
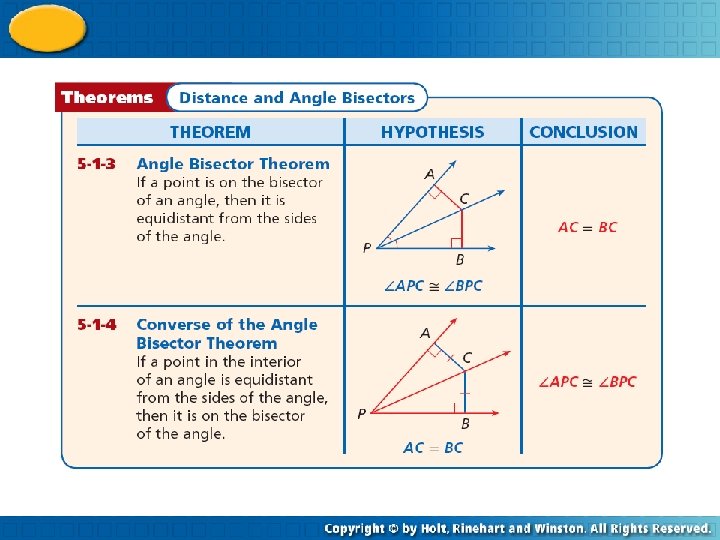

Based on these theorems, an angle bisector can be defined as the locus of all points in the interior of the angle that are equidistant from the sides of the angle.
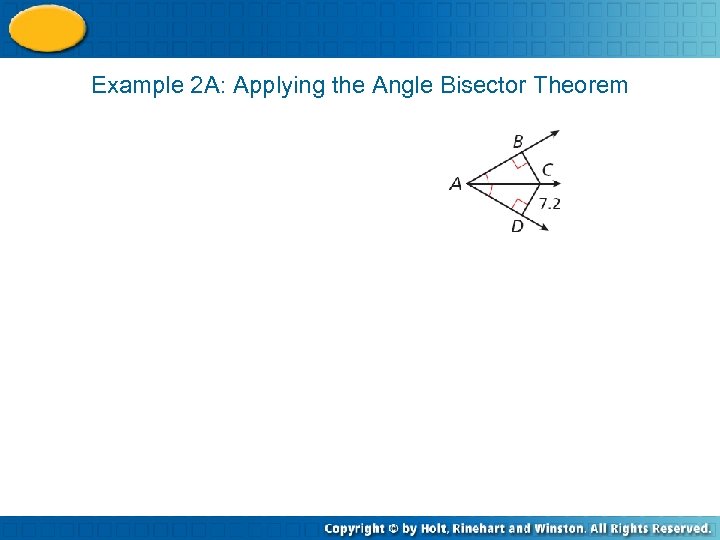
Example 2 A: Applying the Angle Bisector Theorem

Example 2 B: Applying the Angle Bisector Theorem and , bisects of the Angle Bisector Theorem.
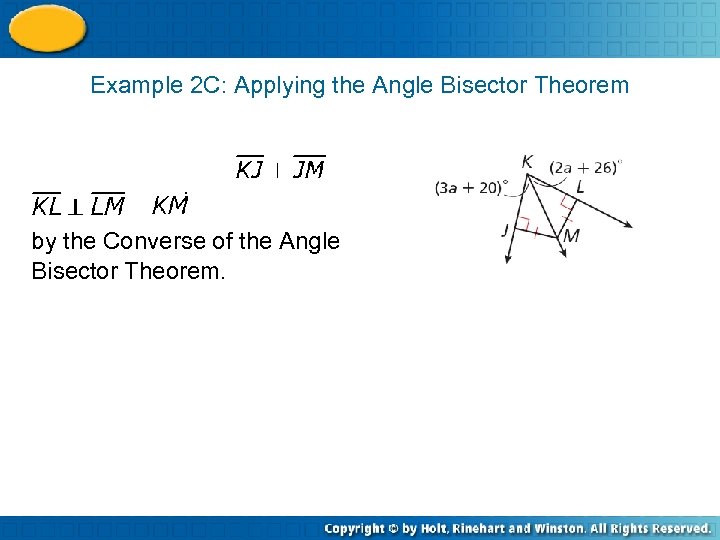
Example 2 C: Applying the Angle Bisector Theorem by the Converse of the Angle Bisector Theorem.
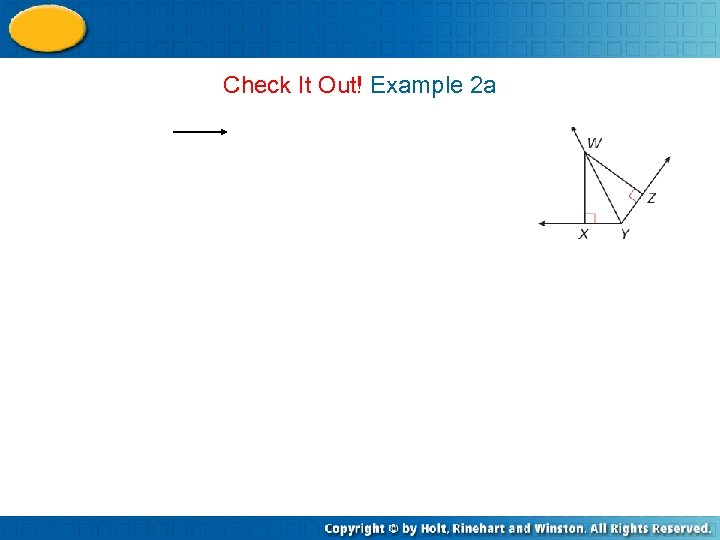
Check It Out! Example 2 a

Check It Out! Example 2 b
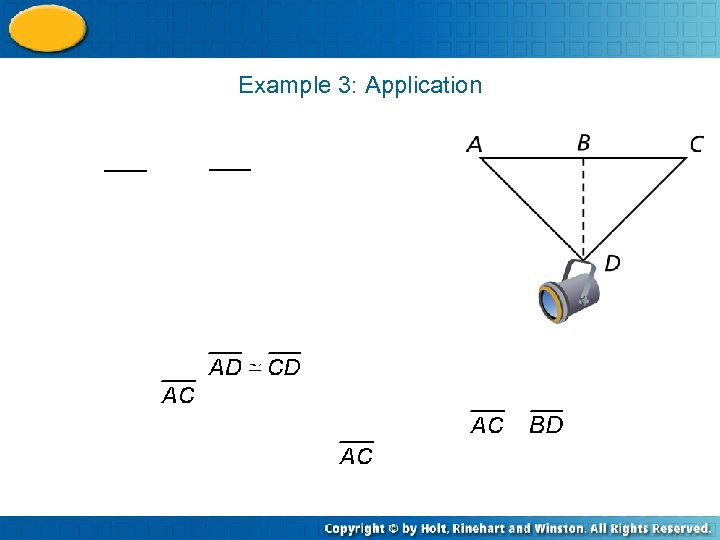
Example 3: Application
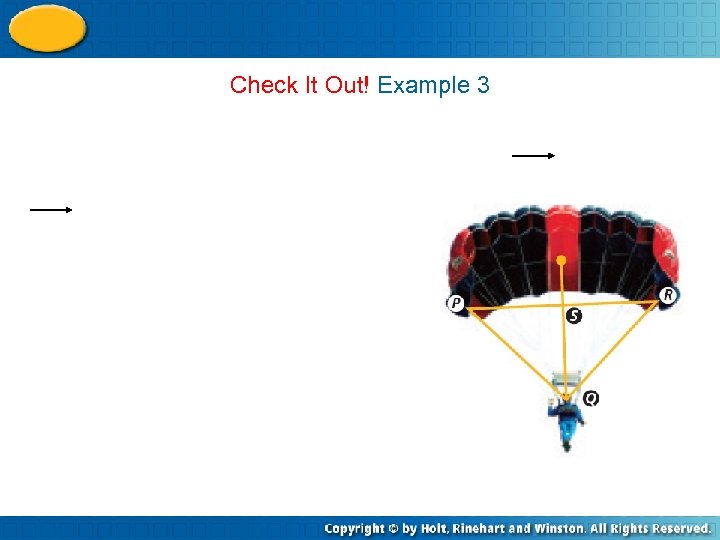
Check It Out! Example 3

Example 4: Writing Equations of Bisectors in the Coordinate Plane The perpendicular bisector of is perpendicular to at its midpoint.
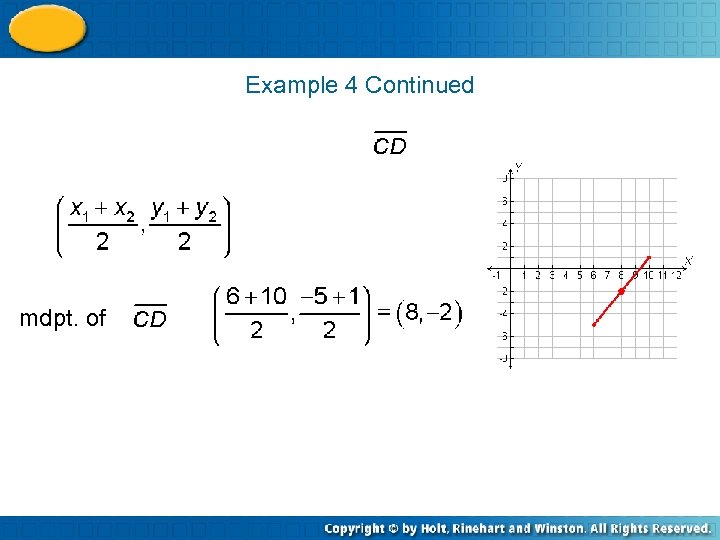
Example 4 Continued mdpt. of =
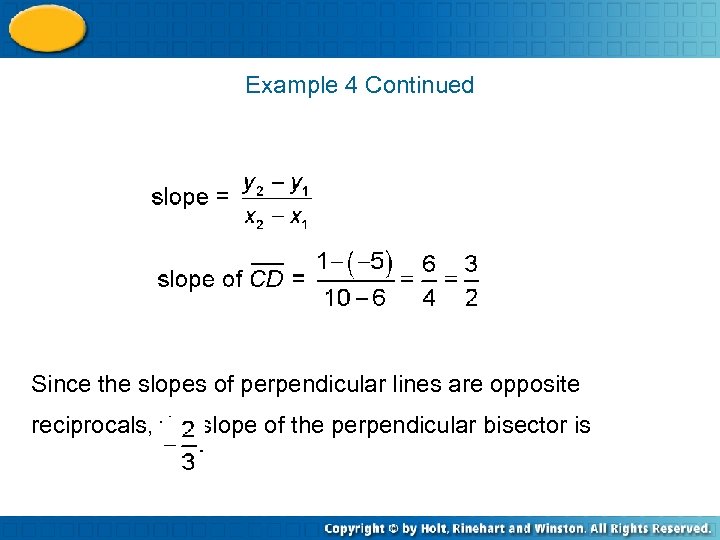
Example 4 Continued Since the slopes of perpendicular lines are opposite reciprocals, the slope of the perpendicular bisector is
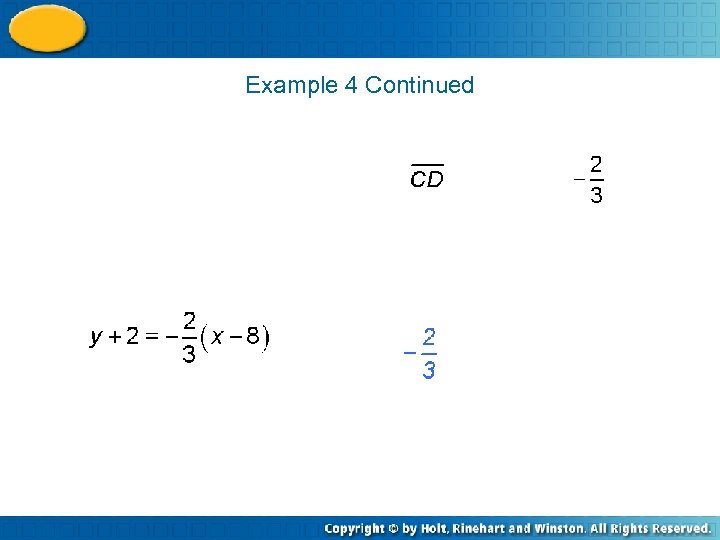
Example 4 Continued
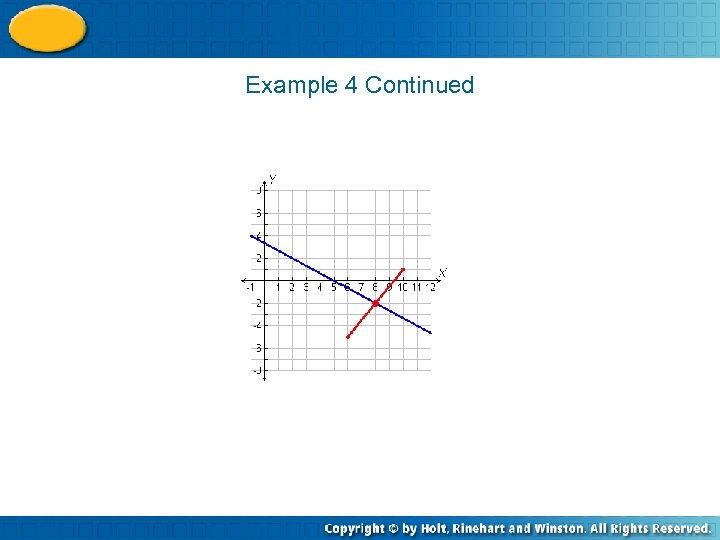
Example 4 Continued
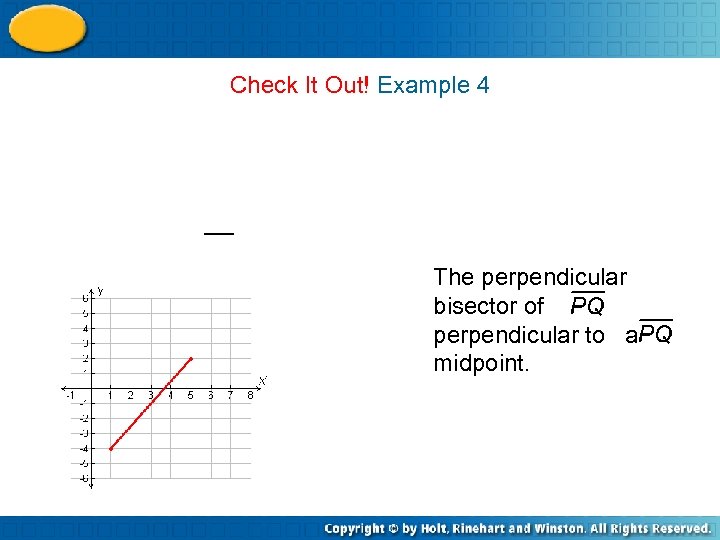
Check It Out! Example 4 The perpendicular bisector of is perpendicular to at its midpoint.
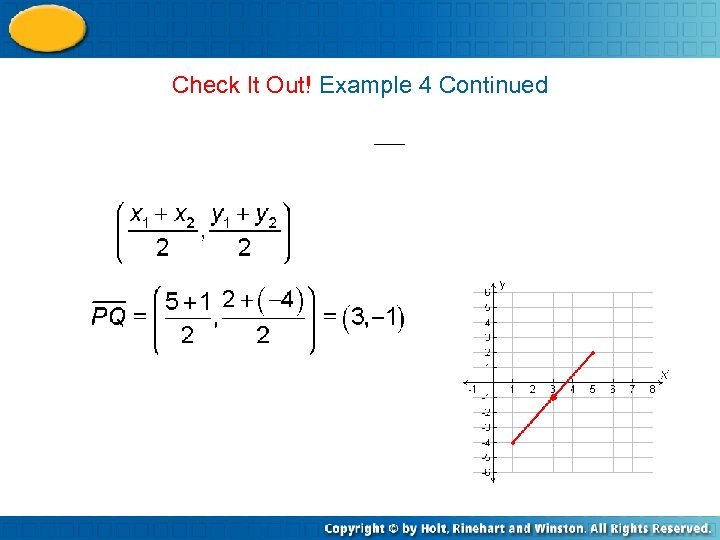
Check It Out! Example 4 Continued

Check It Out! Example 4 Continued Since the slopes of perpendicular lines are opposite reciprocals, the slope of the perpendicular bisector is .
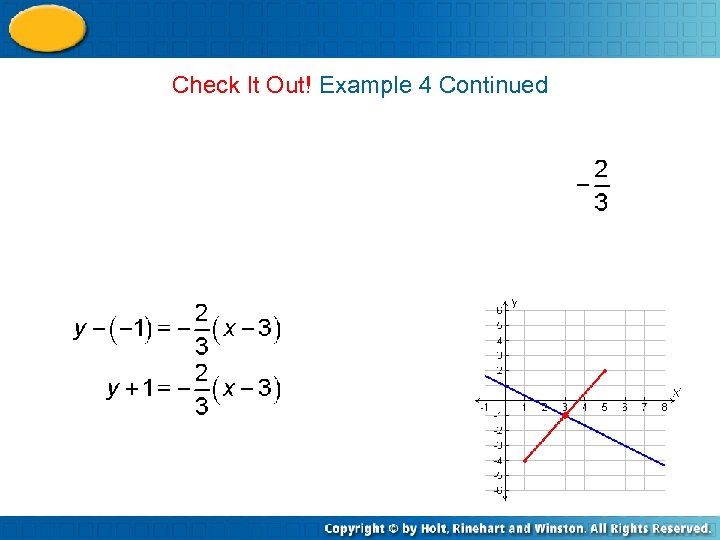
Check It Out! Example 4 Continued



Since a triangle has three sides, it has three perpendicular bisectors. When you construct the perpendicular bisectors, you find that they have an interesting property.
The perpendicular bisector of a side of a triangle does not always pass through the opposite vertex.


The circumcenter can be inside the triangle, outside the triangle, or on the triangle.

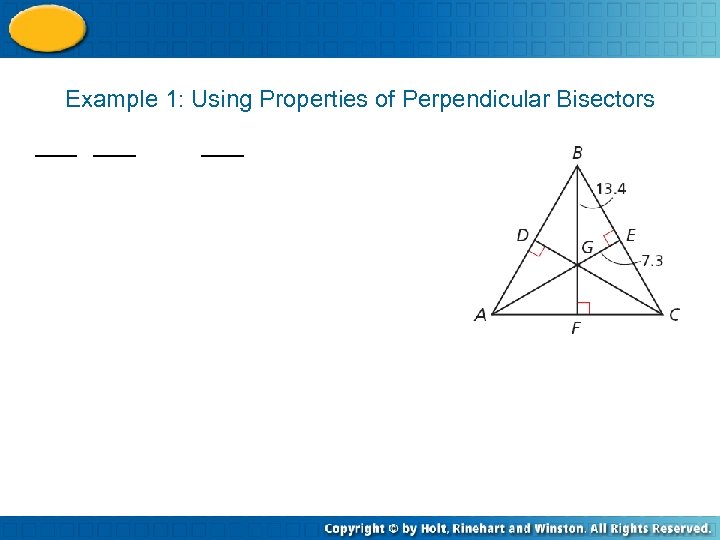
Example 1: Using Properties of Perpendicular Bisectors
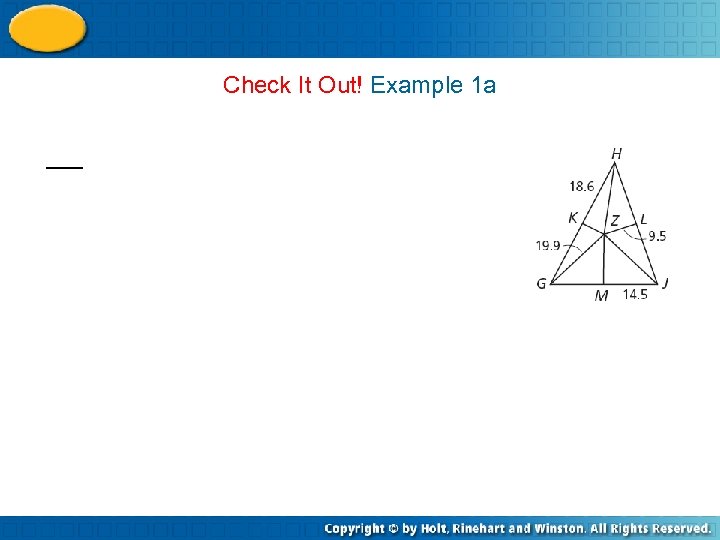
Check It Out! Example 1 a
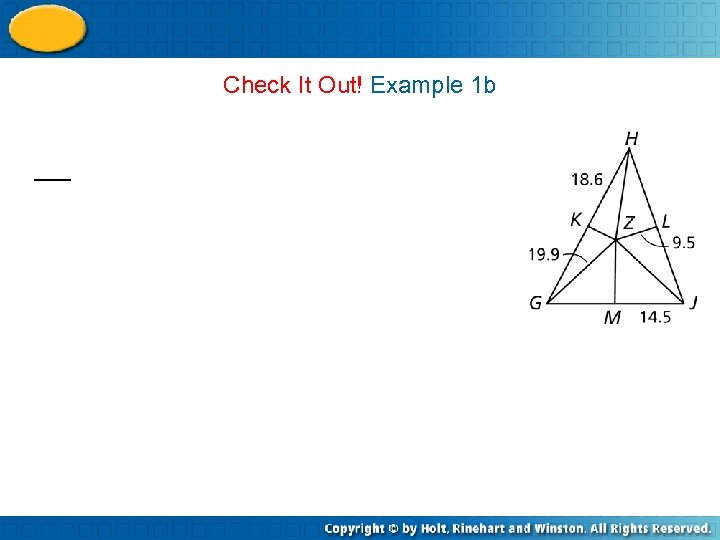
Check It Out! Example 1 b
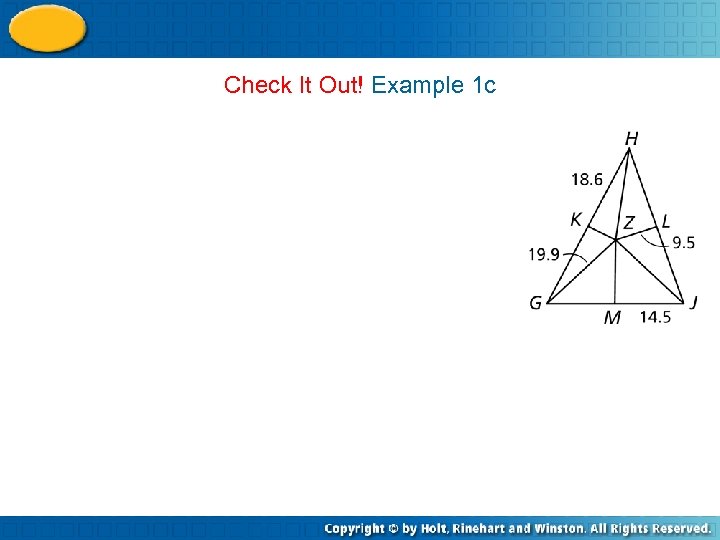
Check It Out! Example 1 c
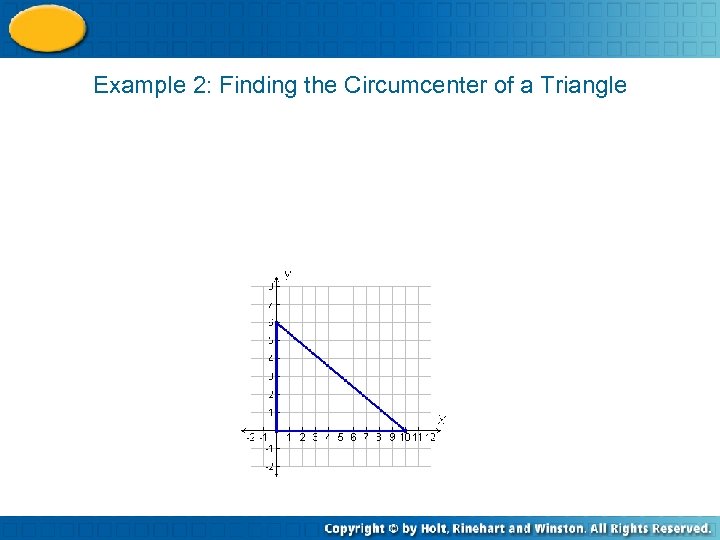
Example 2: Finding the Circumcenter of a Triangle
Example 2 Continued

Example 2 Continued
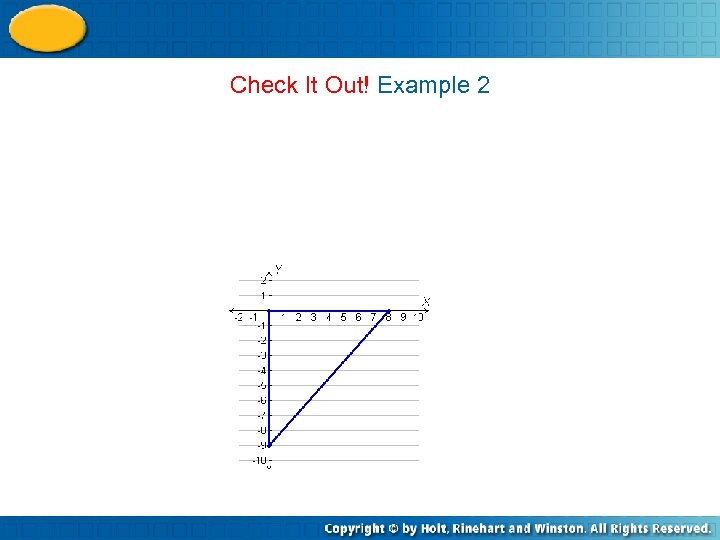
Check It Out! Example 2
Check It Out! Example 2 Continued
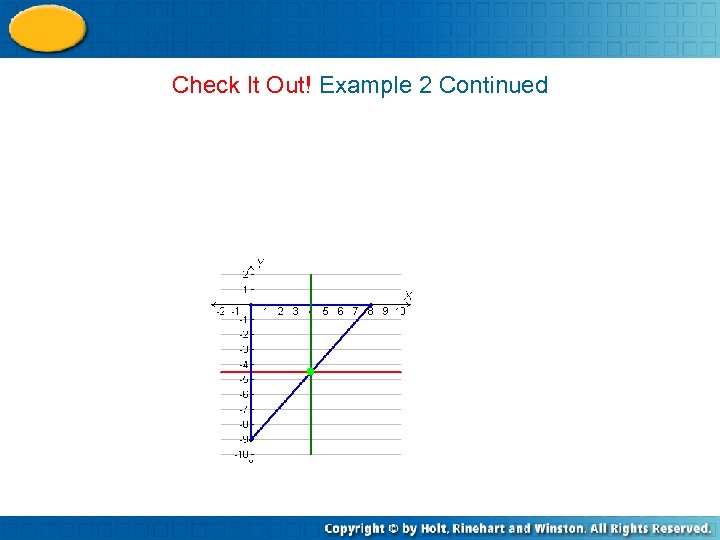
Check It Out! Example 2 Continued
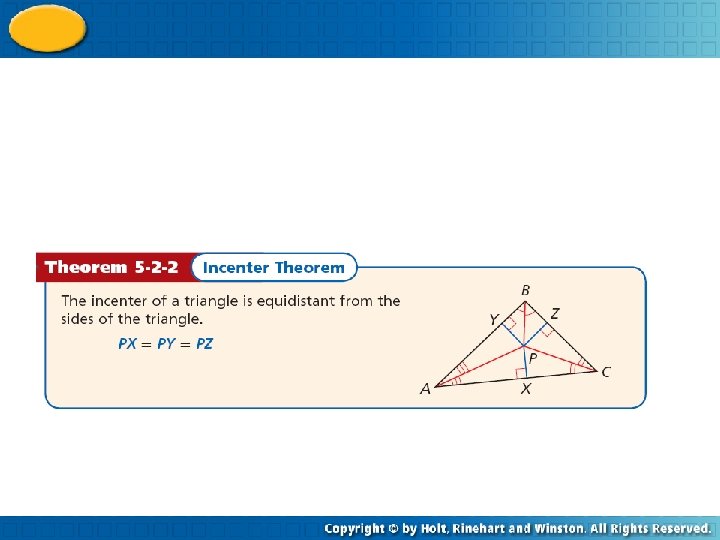

The distance between a point and a line is the length of the perpendicular segment from the point to the line.
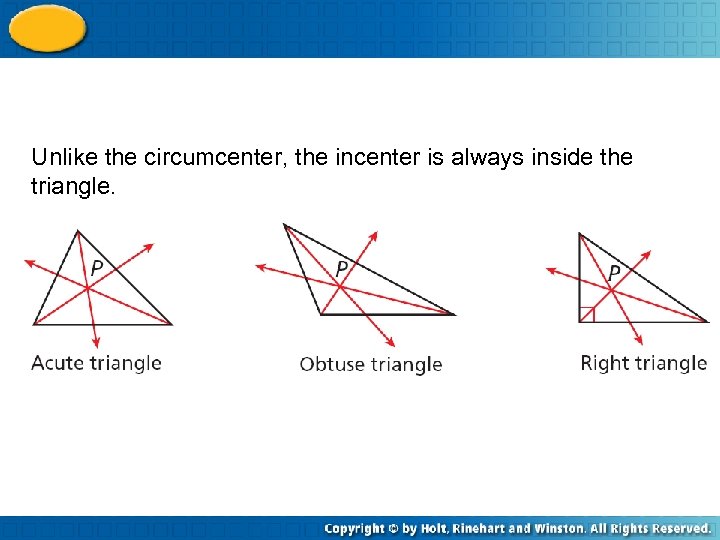
Unlike the circumcenter, the incenter is always inside the triangle.


Example 3 A: Using Properties of Angle Bisectors
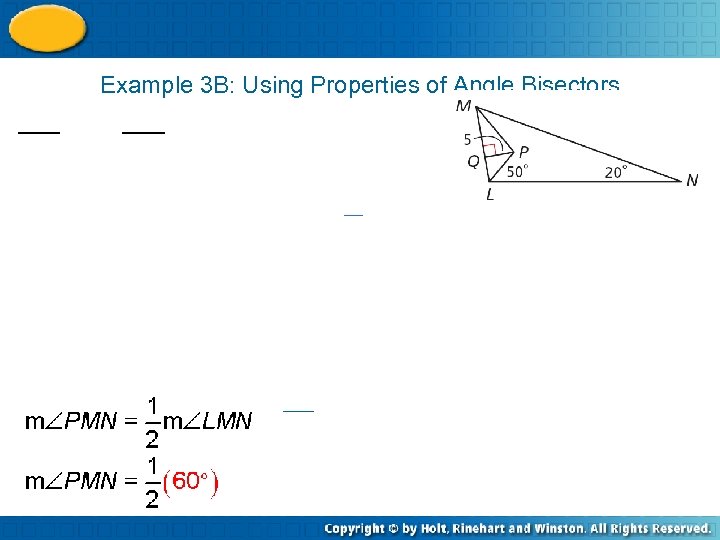
Example 3 B: Using Properties of Angle Bisectors
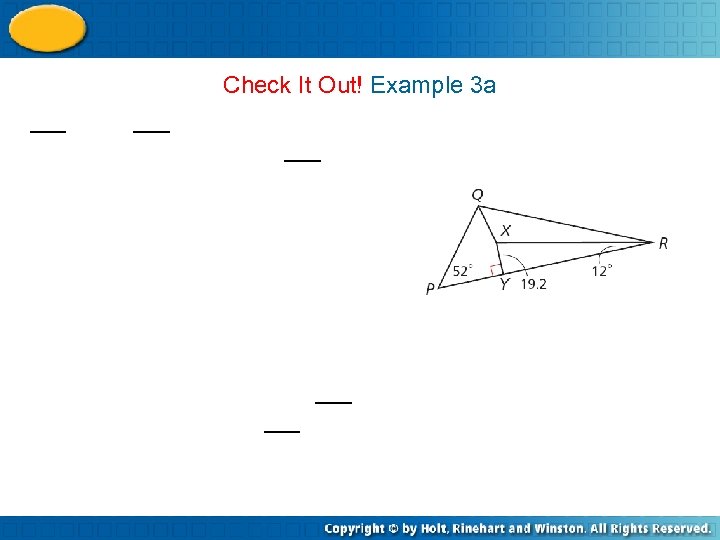
Check It Out! Example 3 a
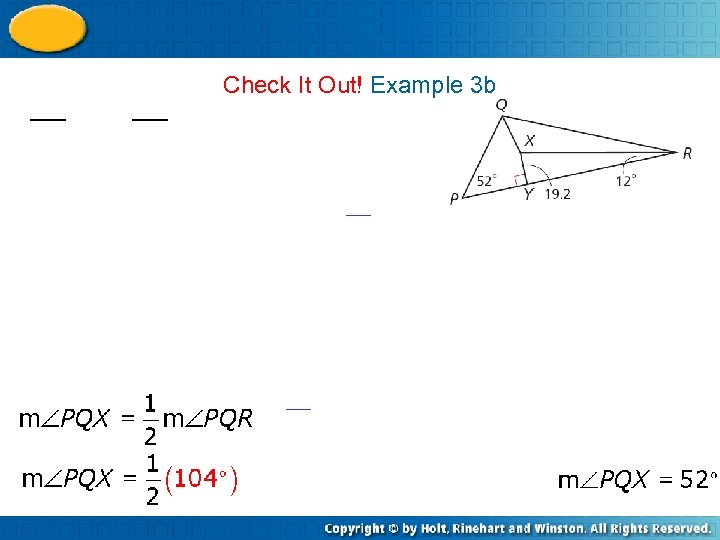
Check It Out! Example 3 b
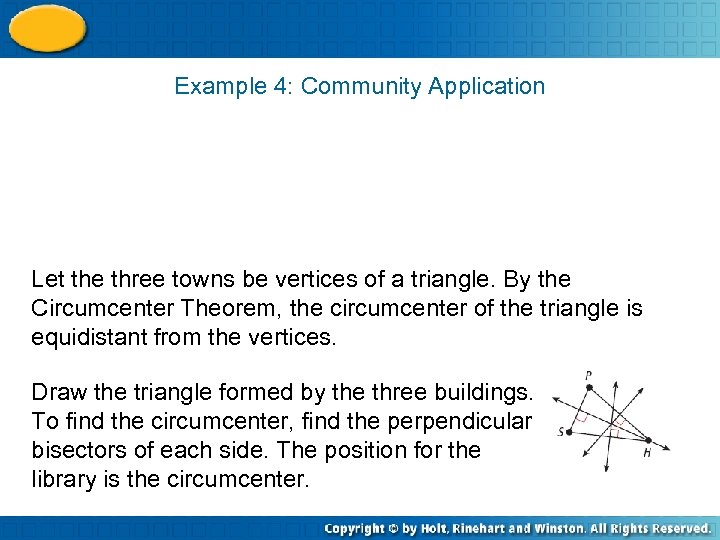
Example 4: Community Application Let the three towns be vertices of a triangle. By the Circumcenter Theorem, the circumcenter of the triangle is equidistant from the vertices. Draw the triangle formed by the three buildings. To find the circumcenter, find the perpendicular bisectors of each side. The position for the library is the circumcenter.
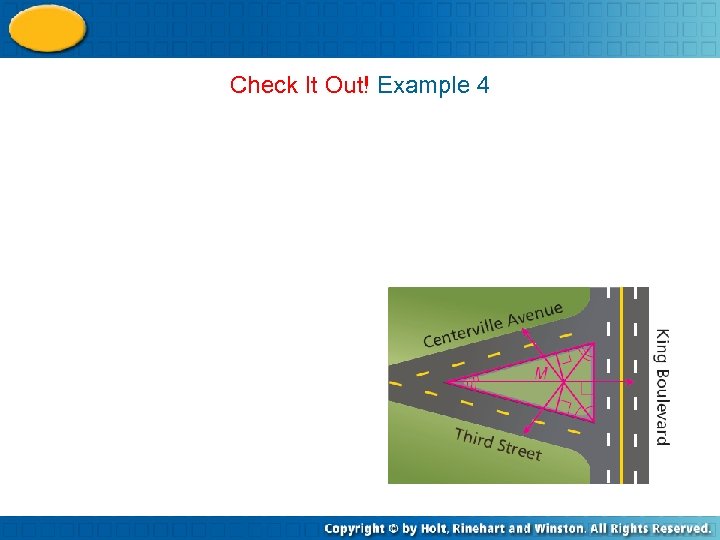
Check It Out! Example 4

Lesson Quiz: Part I 17 3
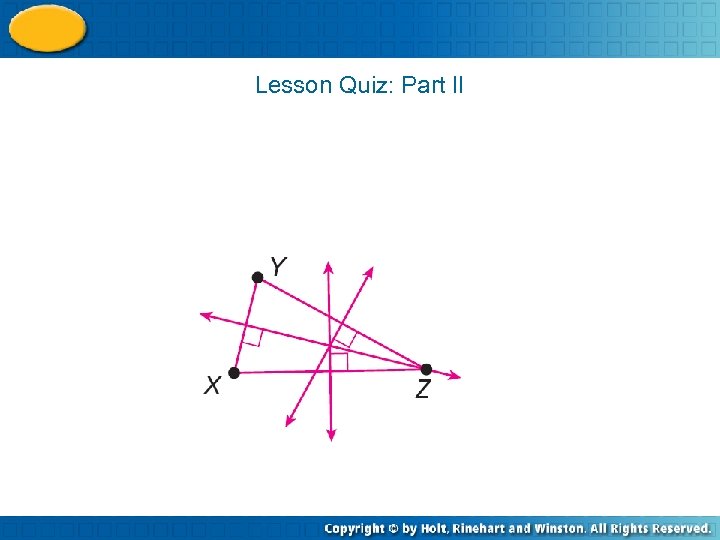
Lesson Quiz: Part II
bb8e92a8748069115c151af4f17f81f1.ppt