WIUU BF-2, Fall 2013, A. Zaporozhetz 1 Class

WIUU BF-2, Fall 2013, A. Zaporozhetz 1 Class 1 -2 R&R. CAPM. Portfolio MGT Diversification. 2 -asset portfolio. Sharpe’s and Treynor’s measures. Portfolio MGT strategies and techniques. Study materials: RWJ: Ch. 9,

WIUU BF-2, Fall 2013, A. Zaporozhetz 2 CORE CONCEPTS P 1 – P 0 ( + ∑ Dividends) HPR, % = P 0)1/(])([ 1 _ 2 nrri i CV = sigma / mean return Return, % = P 1 / P 0 —

WIUU BF-2, Fall 2013, A. Zaporozhetz 3 Risk and return relationship,

WIUU BF-2, Fall 2013, A. Zaporozhetz

WIUU BF-2, Fall 2013, A. Zaporozhetz 5 FINANCE: CORE CONCEPTS 2. Risk and return relationship

WIUU BF-2, Fall 2013, A. Zaporozhetz 6 Risk and return relationship

WIUU BF-2, Fall 2013, A. Zaporozhetz 7 Stock Behavior: Beta and Alpha

WIUU BF-2, Fall 2013, A. Zaporozhetz 8 How can we describe a stock behavior? Find CAT: Beta? Alpha? Mean return? CV? Correl? Sigma?

WIUU BF-2, Fall 2013, A. Zaporozhetz 9 Security Market Line (SML) Return, %. r f. Risk-free rate Market Portfolio. Market return r m Beta 1. 0 SML equation: r f + ( r m — r f )

WIUU BF-2, Fall 2013, A. Zaporozhetz 10 Portfolio is a combination of financial assets (stocks, bonds, etc). In theory of investments, we study a 2 -asset portfolio behavior. If you invested $50, 000 this way: $20, 000 into CAT stocks and $30, 000 into WMT stocks, then your portfolio in fully-invested (no spare cash), with their components’ weights of 40% and 60% respectively. All stock indices could be considered as fully-invested portfolios.

WIUU BF-2, Fall 2013, A. Zaporozhetz 112 -asset portfolio: effect of diversification

WIUU BF-2, Fall 2013, A. Zaporozhetz 12 DIVERSIFICATION Asset allocation among varied securities (fin instruments). Combining in 1 portfolio securities with low positive or (ideally) negative correlations. KO MSFT Sigma, %Sample Portfolio: 33% in MSFT and 67% in KOReturn, %

WIUU BF-2, Fall 2013, A. Zaporozhetz 13 Portfolio with 2 Assets

WIUU BF-2, Fall 2013, A. Zaporozhetz 14 Portfolio Standard Deviations

WIUU BF-2, Fall 2013, A. Zaporozhetz 15 P o rtfo lio R is k : S T D E VNumber of Stocks in Portfolio. Systematic Risk Unsystematic risk. Total risk (Diversifiable risk, Company-specific risk) (Non-diversifiable, Market risk)Components of total risk

WIUU BF-2, Fall 2013, A. Zaporozhetz 16 Unsystematic risk Несистематический риск ( диверсифицируемый риск , собственный риск ; non-systematic risk, diversifiable risk, company-specific risk, unique risk, σ e ). Часть общего риска финансового актива. Связан с внутренними факторами как эмитента, так и поведения участников рынка (продавцов и покупателей). Его значение будет различаться в зависимости от того, против какого рынка (индекса) мы измеряем систематический риск инструмента. Этот риск устраняется частично или полностью при помощи диверсификации активов в инвестиционном портфеле.

WIUU BF-2, Fall 2013, A. Zaporozhetz 17 Portfolio MGT Definition Actual return, CAPM-return Sigma CV Portfolio characteristic line (PCL) Beta Alpha Portfolio MGT measures (ratios): Sharpe’s Treynor’s Unsystematic risk (diversifiable risk)

WIUU BF-2, Fall 2013, A. Zaporozhetz 18 Portfolio: return The rate of return on a portfolio is a weighted average of the rates of return of each asset comprising the portfolio, with the portfolio proportions ($) as weights. r p = w 1 r 1 + w 2 r 2 w 1 = Proportion of funds in Security 1, % w 2 = Proportion of funds in Security 2, % r 1 = Expected return on Security 1, % r 2 = Expected return on Security 2, %

WIUU BF-2, Fall 2013, A. Zaporozhetz 19 Portfolio: Total risk (sigma) When two risky assets with variances 1 2 and 2 2 , respectively, are combined into a portfolio with portfolio weights w 1 and w 2 , respectively, the portfolio variance is given by: p 2 = w 1 2 + w 2 2 + 2 w 1 w 2 Cov(r 1 r 2 ) = = w 1 2 + w 2 2 + 2 w 1 w 2 ρ 1 2 Hence, the portfolio’s standard deviation is the square root of the above.

WIUU BF-2, Fall 2013, A. Zaporozhetz 20 Portfolio: Sigma Rule: Sigma of a 2 -stock portfolio is the weighted average of the 2 sigmas, ONLY when those 2 stocks are perfectly positively correlated. Rule: When a risky asset is combined with a risk-free asset, the portfolio standard deviation equals the risky asset’s standard deviation multiplied by the portfolio proportion invested in the risky asset. riskyassetpw

WIUU BF-2, Fall 2013, A. Zaporozhetz 21 Portfolio systematic risk: Beta The Beta of a portfolio is the weighted average of the rates of return of each asset comprising the portfolio, with the portfolio proportions ($) as weights. ß p = w 1 ß 1 + w 2 ß 2 w 1 = Proportion of funds in Security 1, % w 2 = Proportion of funds in Security 2, % ß 1 = Beta of Security 1 ß 2 = Beta of Security

WIUU BF-2, Fall 2013, A. Zaporozhetz 22 Sharpe’s measure: do we care about those slopes? CAL line Port 1 Port 2 Market AXP WBD AAPL 0, 00% 0, 50% 1, 00% 1, 50% 2, 00% 2, 50% 3, 00% 3, 50% 4, 00% 4, 50% 5, 00% 0, 00%2, 00%4, 00%6, 00%8, 00%10, 00%12, 00% Sigma R eturn ‘

WIUU BF-2, Fall 2013, A. Zaporozhetz 23 SML line Port 1 Port 2 AAPL WBD AXP Market 0, 00%0, 50%1, 00%1, 50%2, 00%2, 50%3, 00%3, 50%4, 00%4, 50%5, 00% 0, 00 0, 50 1, 00 1, 50 2, 00 2, 50 Beta. R eturn ‘ Treynor’s measure

WIUU BF-2, Fall 2013, A. Zaporozhetz 24 Minimum Variance & Sigma Portfolio For a two-stock portfolio, the minimum sigma portfolio, weight on Asset AXP is: BABABAB Aw , 22 2 Example: Correl = 0. 57, r(AXP) = 0. 20% and = 4. 71% r(INTC) = 0. 10% and = 3. 11% %9 )0311. 0(0471. 057. 02)0311. 0(0471. 0 0311. 00471. 057. 00311. 0 22 2 * Ax Therefore, for minimum variance invest in 9% of AXP and 91% of INTC.

WIUU BF-2, Fall 2013, A. Zaporozhetz 25 Portfolio MGT: Techniques 1. Reduce your portfolio’s sigma though diversification. 2. Choose stocks with positive alphas. BUT mind the effect of momentum. 3. When the market is growing, choose aggressive stocks. 4. “ Buy&hold” vs. Active trading. Costs vs. benefits? 5. Technical analysis vs. Fundamental analysis 6. Long positions vs. Short positions 7. If volatility / uncertainty / ambiguity is going higher, stay in your money. 8. Monitor your portfolio performance regularly. 9. Combine various measurements (ratios) to get a fuller picture. 10. Past is a good indicator of the Future ? NOT always. 11. “ Re-shake” your portfolio if needs be. 12. Watch your profits/losses. 13. Watch your cash flows.

WIUU BF-2, Fall 2013, A. Zaporozhetz 26 World Indices

WIUU BF-2, Fall 2013, A. Zaporozhetz 27 Futures: Gold, Oil, RTSI

WIUU BF-2, Fall 2013, A. Zaporozhetz 28 Technical analysis: MICEX today

WIUU BF-2, Fall 2013, A. Zaporozhetz 29 Gazprom: Oil, Futures, Indices

WIUU BF-2, Fall 2013, A. Zaporozhetz 30 Food for thought: Correlation of PZLT ( «Полюс золото» ) in 2010 with: Market (RTSI): 0, 43 Competitor ( “ Polimetall ” ): 0, 49 Gold (LME): 0,

WIUU BF-2, Fall 2013, A. Zaporozhetz 31 More Sources:

WIUU BF-2, Fall 2013, A. Zaporozhetz 32 Class 2 Portfolio MGT Additional materials

WIUU BF-2, Fall 2013, A. Zaporozhetz 33 SML in 2009:

WIUU BF-2, Fall 2013, A. Zaporozhetz 34 Beta vs. D/A in 2009, DJIA companies:

WIUU BF-2, Fall 2013, A. Zaporozhetz 35“ CV curse” © A. Zaporozhetz According to the CV rule, we need to chose the lowest CV securities. The risk-free US T-bills/notes have the lowest CV, too, as their sigma is MIN. 1. BUT do we like those returns? Therefore, the CV rule may be misleading. 2. The securities on the CML have NOT the same CVs. We need to design some other portfolio MGT ratios to adjust for the risk-free factor, to overcome the “CV curse”, e. g. , Sharpe’s, Treynor’s…

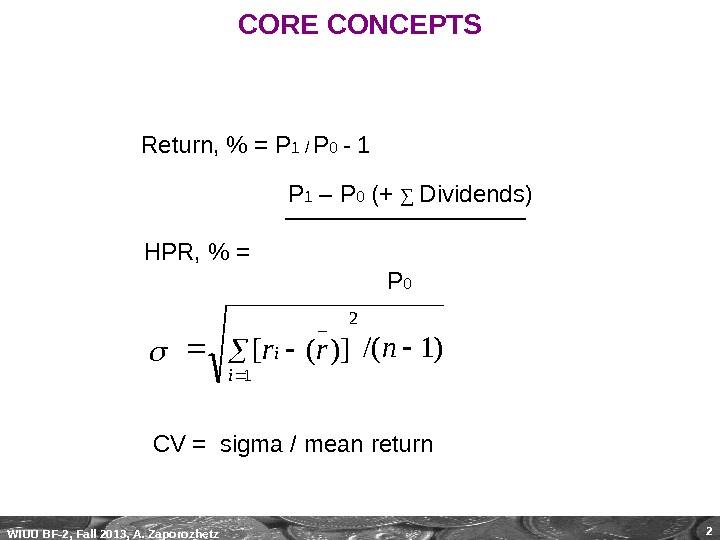
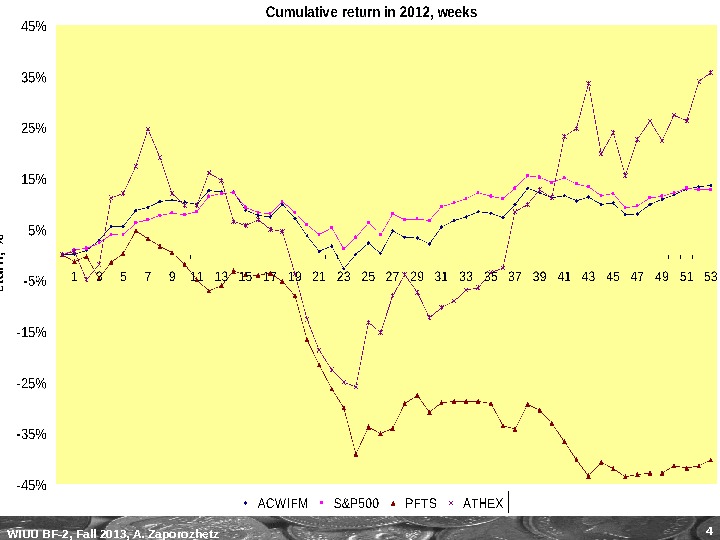
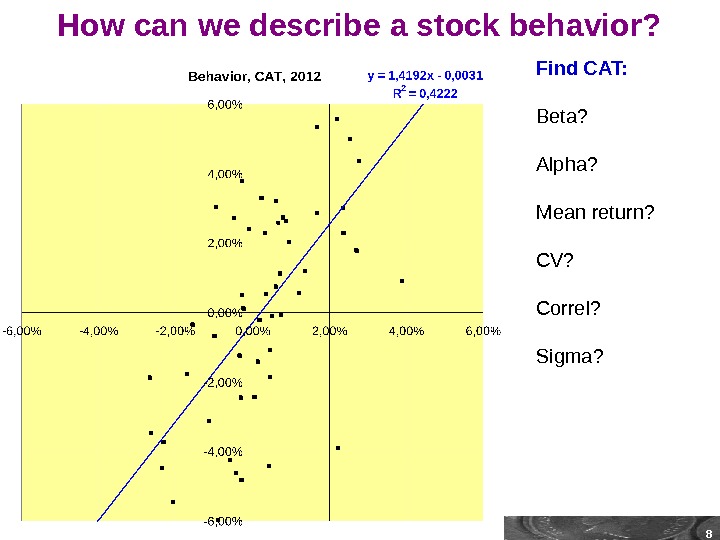
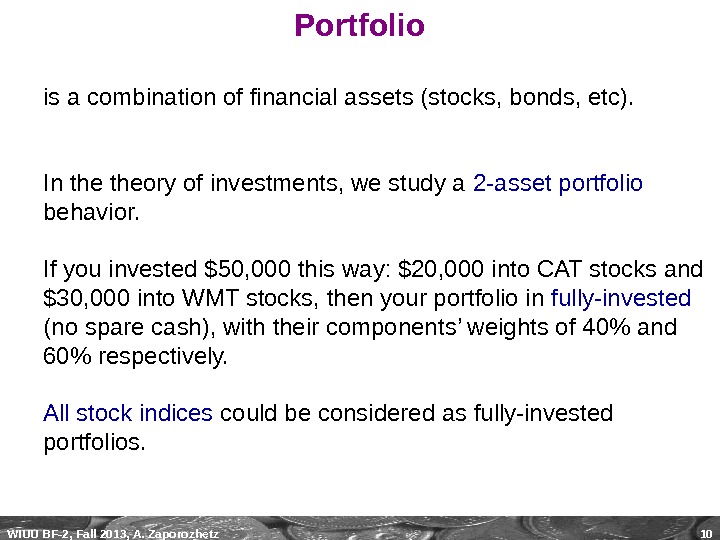
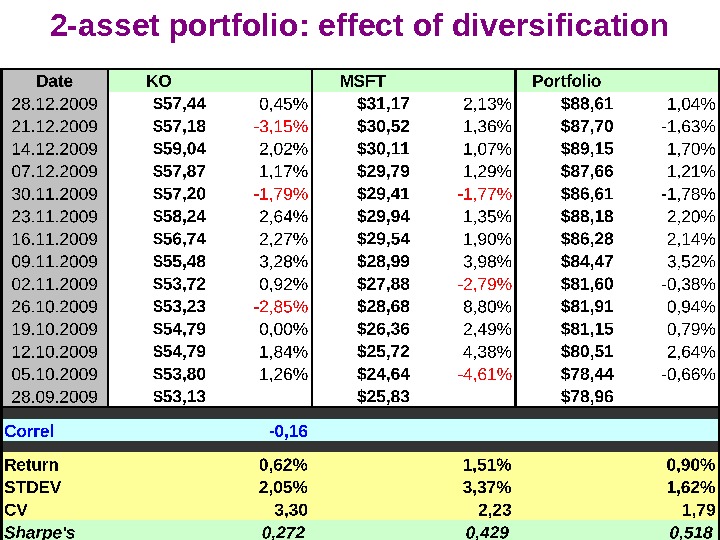
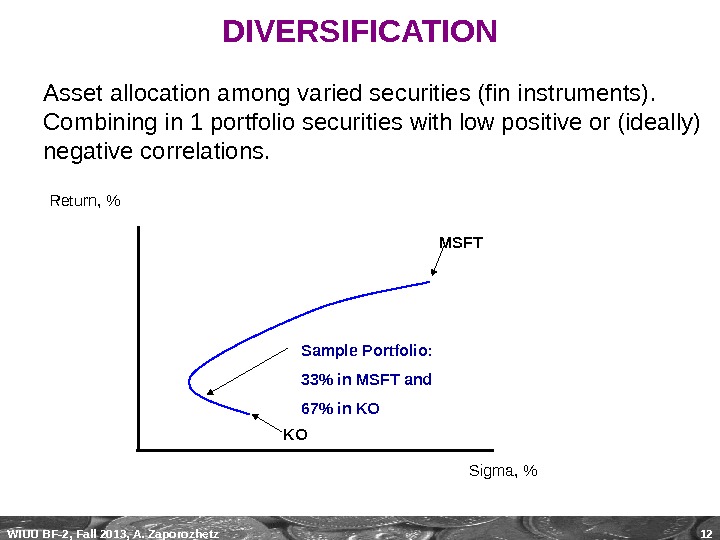
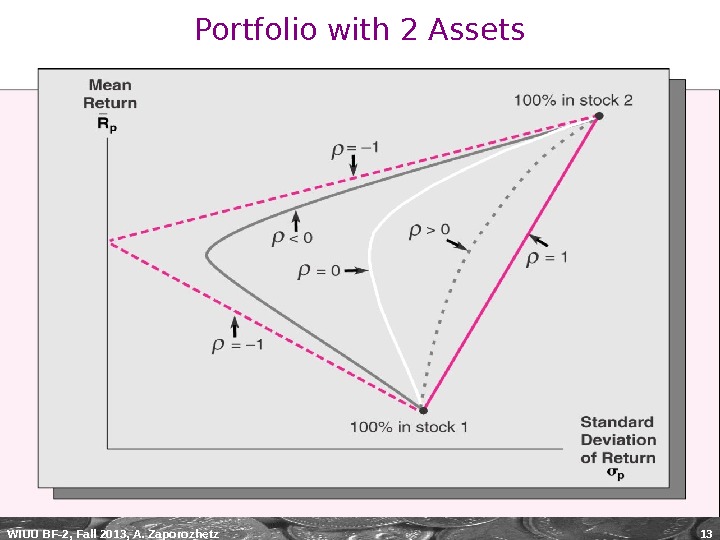
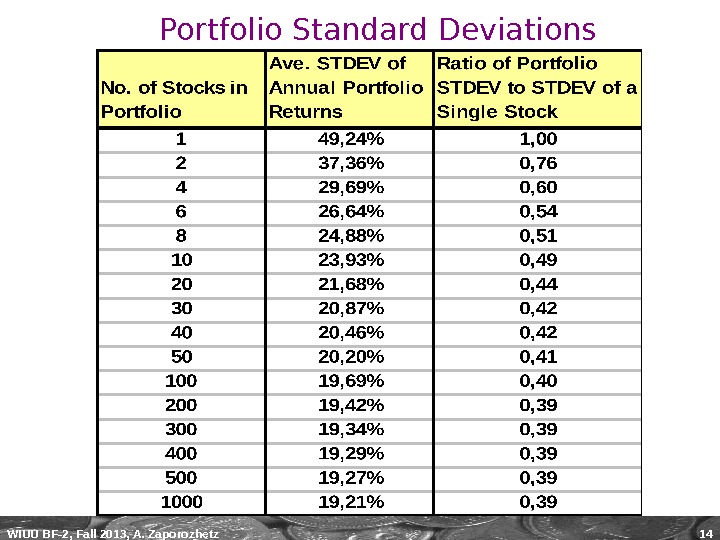
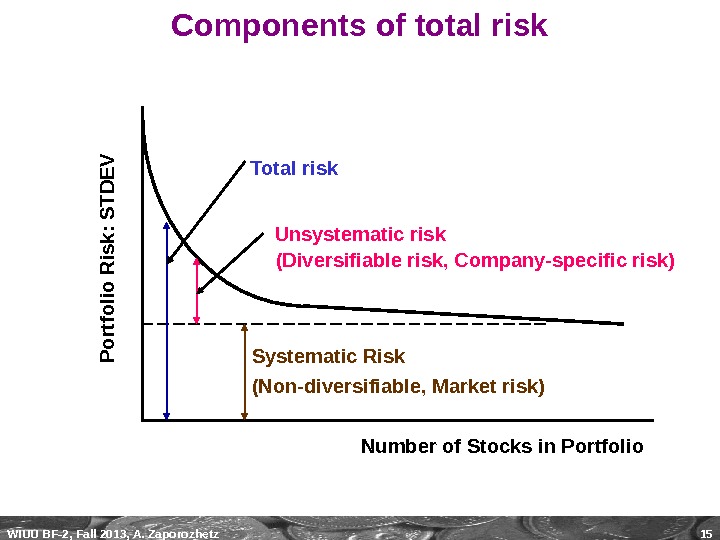
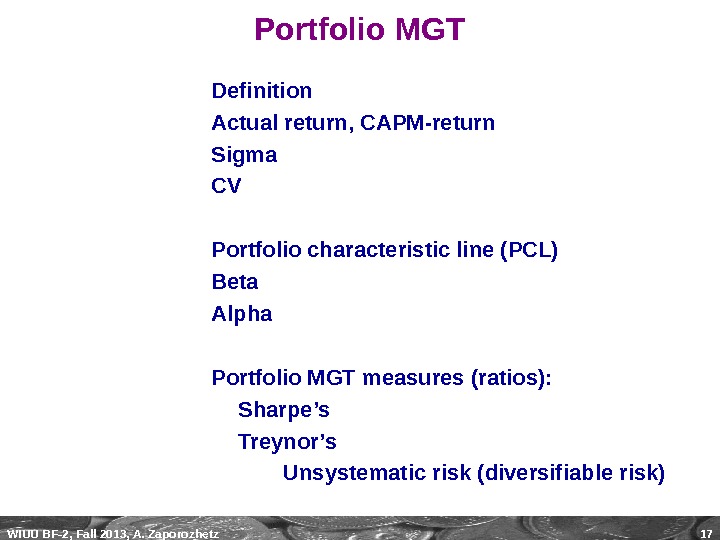
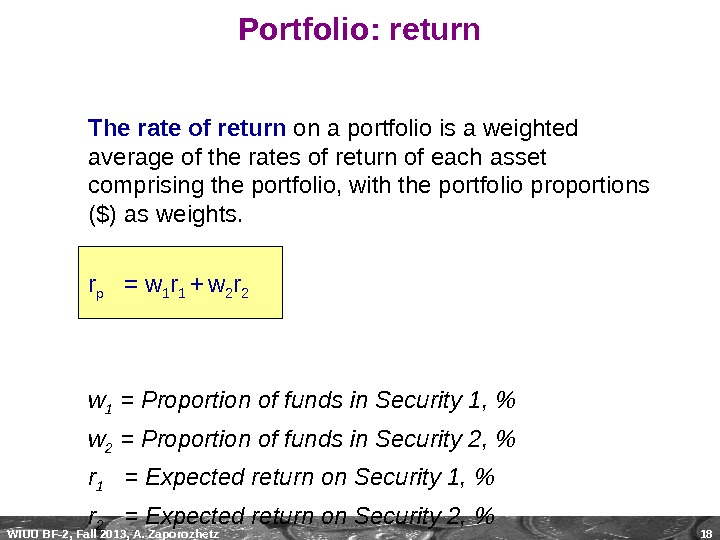
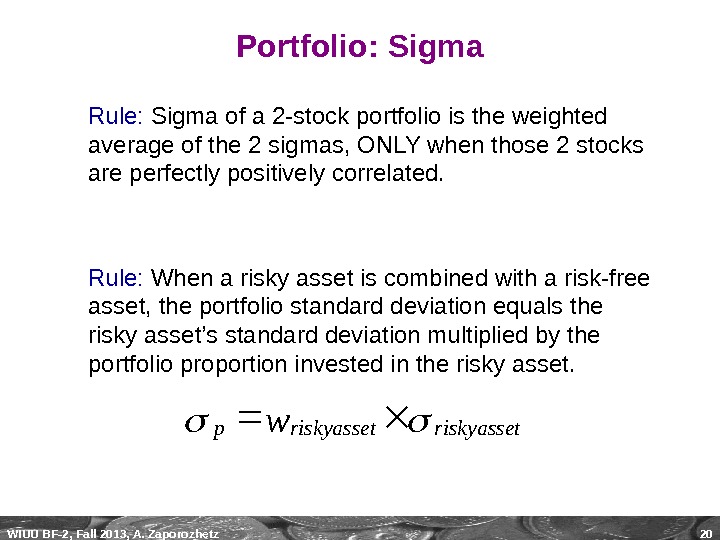
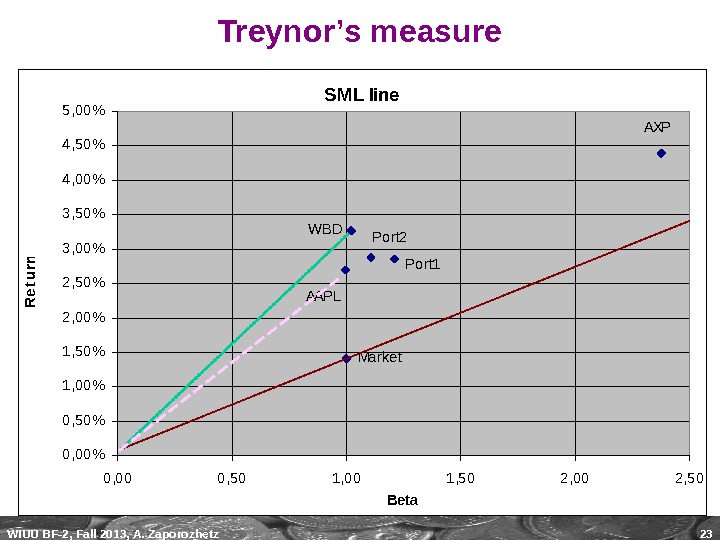
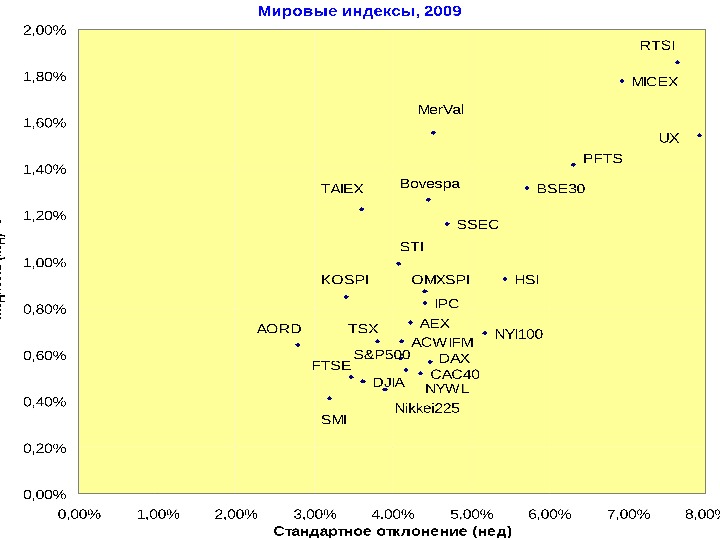
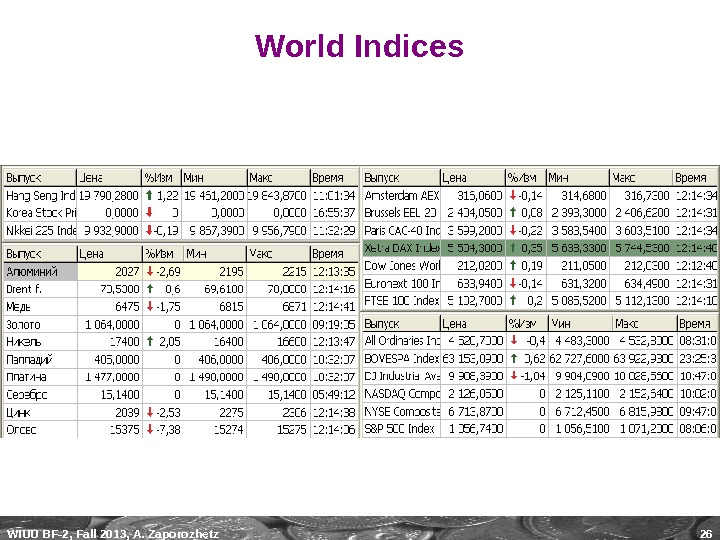
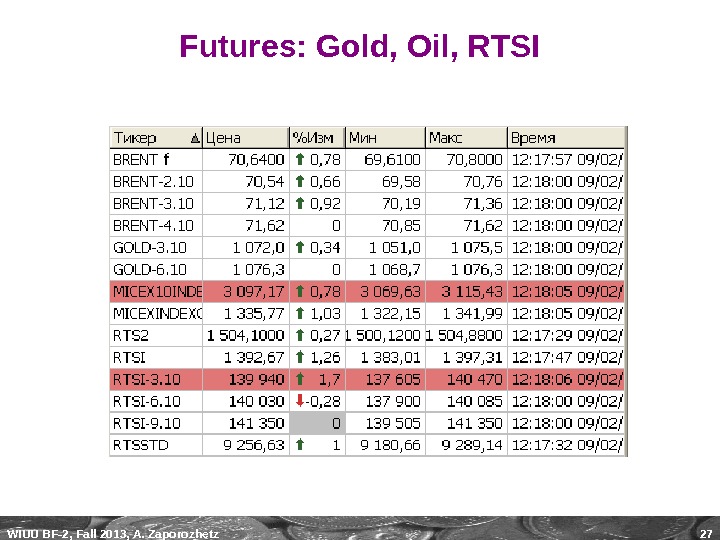
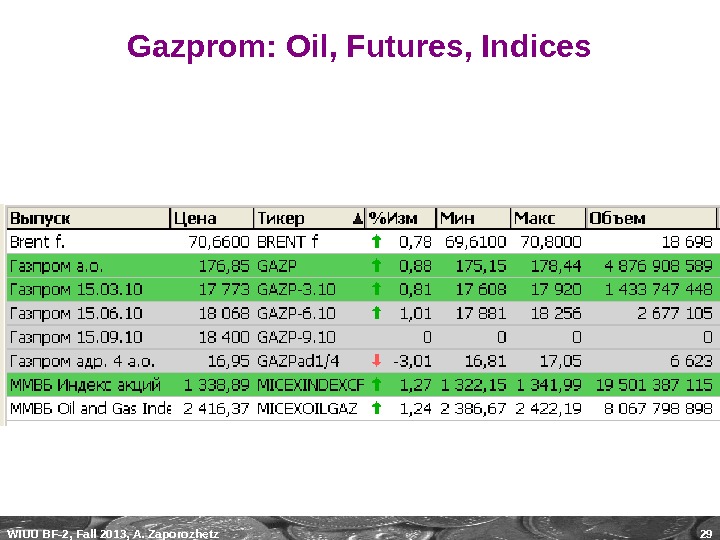
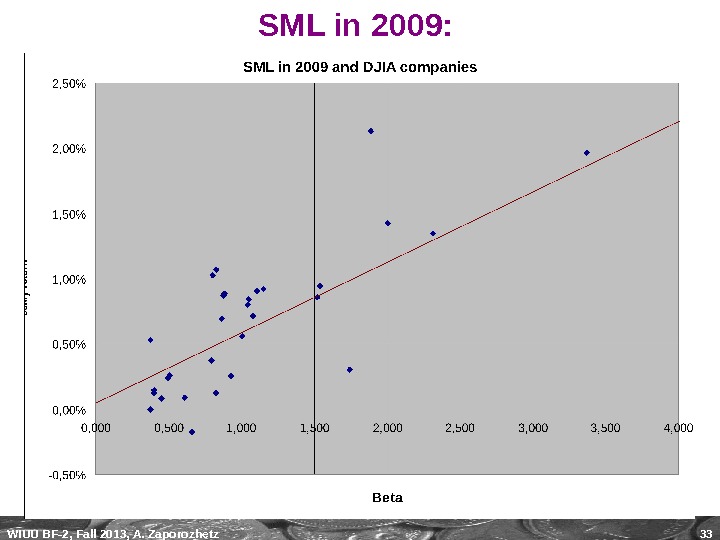
WIUU BF-2, Fall 2013, A. Zaporozhetz 36 Correlation is a measure of the linear association between the 2 variables. In the world of finance, a statistical measure of how two securities move in relation to each other. ρ 1 , 2 = Cov 1, 2 / σ 1 σ 2 Correlation coefficient is ALWAS [-1; 1]. Perfect positive correlation (a correlation coefficient of +1) implies that as one security moves, either up or down, the other security will move in lockstep, in the same direction. Alternatively, perfect negative correlation means that if one security moves in either direction the security that is perfectly negatively correlated will move by an equal amount in the opposite direction. If the correlation is 0, the movements of the securities are said to have no correlation; they are completely random.