Where How When What Why 2017 -18 Who

Where? How? When? What? Why? 2017 -18 Who? Managerial Economics Stefan Markowski Input sourcing and investment The economics of competitive advantage

Detailed course schedule Day no Topic Textbook ch. 1 (24 Nov; 3 hrs) 1. Introduction. Decision making process and its elements. The scope of economic decision making. Application of marginal analysis Chs. 1 -2 2 3 3 3 2. Demand analysis and demand elasticities Ch. 3 3. Buyer product valuation and choices. Consumer surplus. Buyer pricing decisions Ch. 4 4 (27 Nov; 2 hrs) 4. Production/transformation process. Production technologies and input-output structure Ch. 5 5 (28 Nov; 2 hrs) 5. Cost structure and cost drivers of producer pricing strategies. Production scale and scope. Chs. 5 and 7 6 (1 Dec; 3 hrs) 6. Structure-conduct-performance. Market structures: competition and contestability. Pricing strategies of buyers and sellers Ch. 8 7 (2 Dec; 3 hrs) 7. Market structures: monopoly/monopsony, monopolistic competition and oligopoly. Pricing strategies and strategic behaviour Chs. 9 -10 8 (3 Dec; 3 hrs) 8. Input sourcing and investment Chs. 6 and 11 9 (4 Dec; 2 hrs) 9. Decision making under conditions of uncertainty. Informational asymmetries and risk management. Pricing and market power Ch. 12 10 (5 Dec; 2 hrs) 10. Market research and market analysis. Auction and rings. Strategic behaviour Ch. 13 11 (8 Dec; 2 hrs ) 12 (9 Dec; 2 hrs) 11. Public sector perspective Ch. 14 12. Revision 13. Examination 13 (11 Dec; 2 hrs) Examination (25 Nov; hrs) (26 Nov; hrs)

Input sourcing and investment Contents 8. 1 Managerial perspective 8. 2 Input sourcing 8. 3 Investment value calculations 8. 4 The cost of capital 8. 5 Life Cycle Costing (LCC) 8. 6 Portfolio investment 8. 7 Real options considerations 8. 8 Further reading

8. 1 Managerial perspective • Input sourcing activities use resources and, thus, incur costs. There also alternative ways of achieving costs particular outcomes: investment options • Each of these options has an opportunity cost in that it can be valued in terms of the best alternative option forgone as well as the actual (historic) cost incurred • Capital budgeting refers to the evaluation of investment options associated with – replacement of old equipment – purchase of new capacity – adoption of new production methods – capacity expansion – new product development – compliance with government regulations • This Topic is concerned with the viability of different investments and the selection of the best option

8. 2 Input sourcing • When sourcing inputs firms face make or buy decisions • They can buy materials in spot markets, hire labour, rent equipment, borrow and raise capital through the market • They can also integrate vertically upstream and produce inputs in-house (e. g. , components, power) • Many inputs are highly specific to their subsequent deployment, they have no substitute uses and, thus, high deployment sunk cost (e. g. , asset specificity in manufacturing) • Input suppliers and buyers also have market power and once they enter into a contract they may engage in opportunistic behaviour • They may get away with it when the transaction cost of switching to an alternative source of supply or demand is high (hold-up problem) problem • Contracts are also incomplete which leads to frustrated expectations

8. 3 Investment value calculations • $1 today is worth more than $1 received in future because the opportunity cost of the latter is the foregone interest that would have been earned were $1 received today • This opportunity cost reflects the time value of money • To understand you must first understand the concept of present value (PV) of a future value (FV) to be received n years in the future PV = FV/(1+i)n, where i the interest rate • Example: FV=$100 to be received in 10 years with i = 0. 07 PV = $100/(1+0. 07)10 = $100/1. 9672 = $50. 83

8. 3 Investment value calculations • General PV formula • PV = FV 1/(1+i)1 + FV 2/(1+i)2 + … + FVn/(1+i)n • PV = S FVt/(1+i)t , where t = 1, 2, … n • Net present value is the present value of the income stream produced by an investment option (opportunity) less the current (present) cost of taking up that opportunity • To choose between alternative investment options we must first value their net worth (for the investor), i. e. , their expected (future) benefits net of their expected costs, that is, the net present value

8. 3 Investment value calculations • The net present value (NPV) of an investment option is the present value of all future revenues, Rt, (where benefits are revenues from sales) less costs, Ct, discounted at the investor’s cost of capital minus the initial (upfront) capital outlay, C 0, over the time period t = 1, … n NPV = S (Rt–Ct) /(1+r)t – C 0 • This is net cash flow or anticipated profit on equity provided by the investor, providing she/he captures all of it. If the NPV is positive the investor increases his/her wealth by taking up (exercising) the investment option • t = 0 is the base date) and the end point, n (option end date) is the terminal date; (Rt–Ct) - estimated net cash flow in time period t and C 0 - the initial capital outlay; and r - rate of discount and 1/(1+r)t is the discount factor applied to (Rt–Ct)

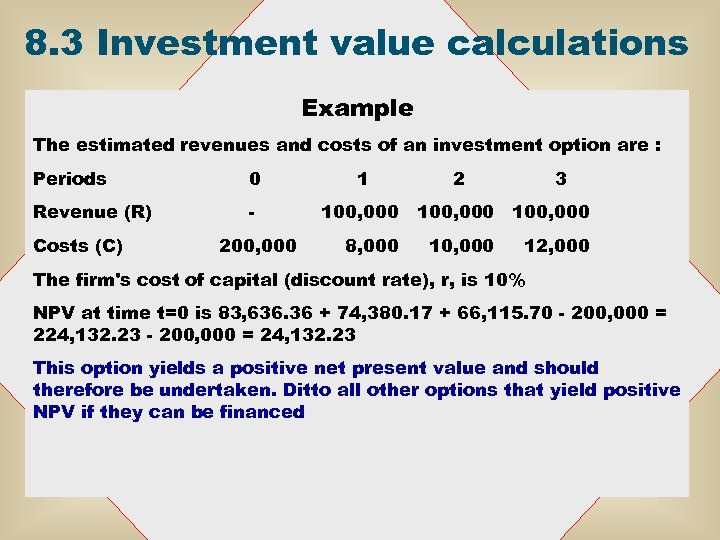8. 3 Investment value calculations Example The estimated revenues and costs of an investment option are : Periods 0 Revenue (R) - Costs (C) 200, 000 1 2 3 100, 000 8, 000 10, 000 12, 000 The firm's cost of capital (discount rate), r, is 10% NPV at time t=0 is 83, 636. 36 + 74, 380. 17 + 66, 115. 70 - 200, 000 = 224, 132. 23 - 200, 000 = 24, 132. 23 This option yields a positive net present value and should therefore be undertaken. Ditto all other options that yield positive NPV if they can be financed

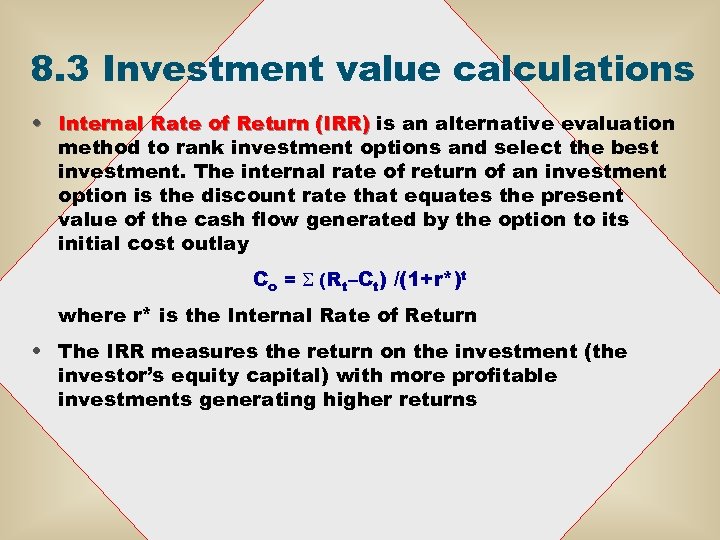8. 3 Investment value calculations • Internal Rate of Return (IRR) is an alternative evaluation method to rank investment options and select the best investment. The internal rate of return of an investment option is the discount rate that equates the present value of the cash flow generated by the option to its initial cost outlay Co = S (Rt–Ct) /(1+r*)t where r* is the Internal Rate of Return • The IRR measures the return on the investment (the investor’s equity capital) with more profitable investments generating higher returns

8. 3 Investment value calculations • Investment options can, thus, be ranked in term of their IRR and the investor should undertake those projects or take up those options where the IRR is greater than or equal to the discount rate he/she uses to evaluate the cost of their capital (the marginal cost of capital) • This reflects the opportunity cost of employing equity capital in the activity under consideration as opposed to the best opportunity for its employment elsewhere (its opportunity cost). If you could invest your own money safely at 10% elsewhere you would expect to earn at least 10% if you invest it in your own business

8. 3 Investment value calculations Example • The estimated revenues and costs of an investment option/project are as follows: Period 0 Revenue (R) Costs (C) 1 2 100, 000 200, 000 8, 000 10, 000 3 100, 000 12, 000 The investor's cost of capital is 10% • Thus r*is approximately equal to 17%. This is greater than 10% (the cost of capital) and if this is the only investment option available (other than the opportunity investment) it should proceed. But, if there are other options they should be IRR-ranked first

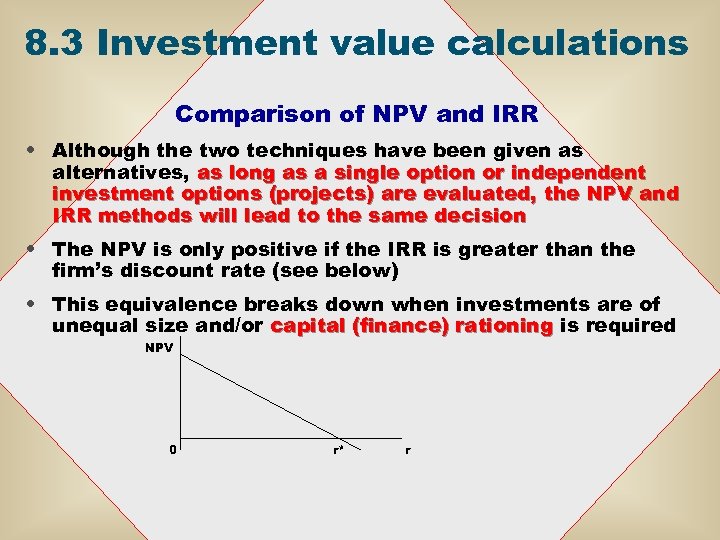8. 3 Investment value calculations Comparison of NPV and IRR • Although the two techniques have been given as alternatives, as long as a single option or independent investment options (projects) are evaluated, the NPV and IRR methods will lead to the same decision • The NPV is only positive if the IRR is greater than the firm’s discount rate (see below) • This equivalence breaks down when investments are of unequal size and/or capital (finance) rationing is required NPV 0 r* r

8. 3 Investment value calculations • The rule that the investor should invest if the NPV is positive is equivalent to the rule that he/she should invest if the IRR is greater than the discount rate • Another way of expressing the rule is to state that the investor should invest up to the point where the marginal return on investment equals the marginal cost of investment • Note that two small projects may each have smaller NPVs than a larger project, but together may increase the net worth of the firm more than the large project. If there is no shortage of capital all such project should be funded but see capital rationing below

8. 3 Investment value calculations Capital Rationing • Capital rationing may be needed when the investor wishes to avoid the strains of rapid business expansion or he/she does not want to become overexposed under unfavourable market conditions • When investors cannot undertake all projects with positive NPVs, they may rank projects according to their profitability index (PI), which measures net cash flow per dollar cost of investment outlay, and choose the projects with the highest PI index values

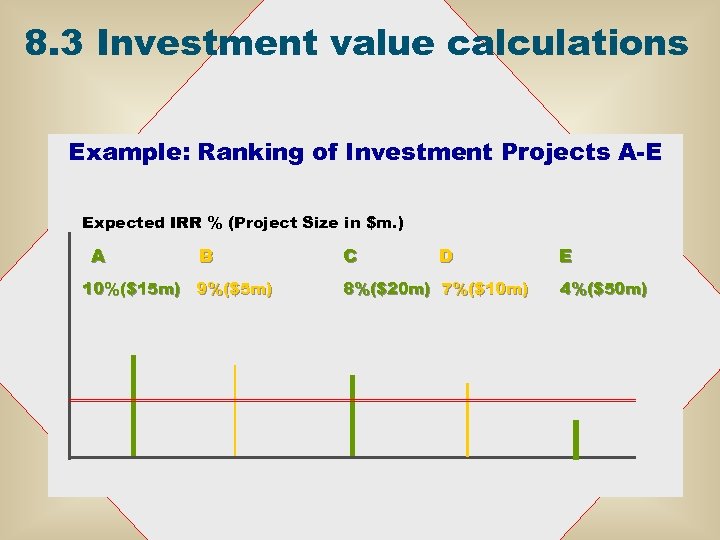8. 3 Investment value calculations Example: Ranking of Investment Projects A-E Expected IRR % (Project Size in $m. ) A B 10%($15 m) 9%($5 m) C D 8%($20 m) 7%($10 m) E 4%($50 m)

8. 3 Investment value calculations Payback Period • Another popularly used capital budgeting procedure is the payback period criterion • Under this rule, if the investment generates a net flow of returns that covers the initial cost before some arbitrarily set time period, the option should be taken up • For example, if an investment option cost $300, 000 and returns $50, 000 in each succeeding year, it will be undertaken if the investor sets a payback period of 6 years but not if a payback period of 4 years is adopted • This procedure ignores the time value of money (cash flow is not discounted) but it is often used as a rule of thumb in property development

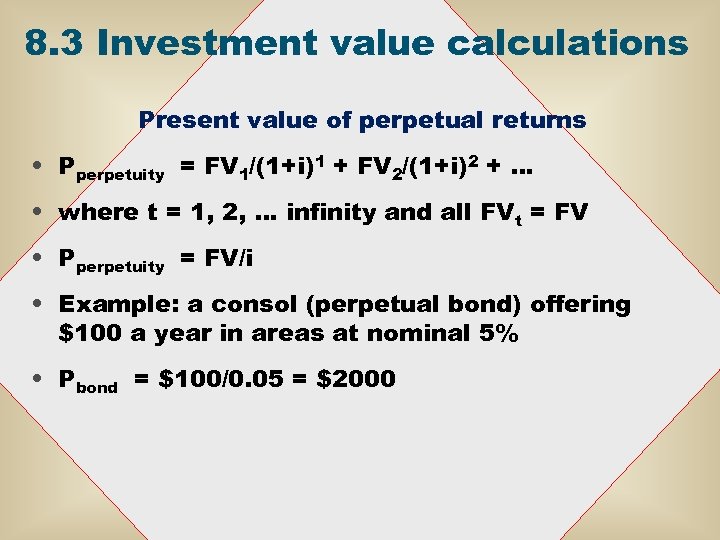8. 3 Investment value calculations Present value of perpetual returns • Pperpetuity = FV 1/(1+i)1 + FV 2/(1+i)2 + … • where t = 1, 2, … infinity and all FVt = FV • Pperpetuity = FV/i • Example: a consol (perpetual bond) offering $100 a year in areas at nominal 5% • Pbond = $100/0. 05 = $2000

8. 4 The Cost of capital • The cost of raising capital to invest is an essential element of the capital budgeting process, using either the NPV or IRR method of evaluation • Private investors raise capital from many sources – externally from the issuing of debt and sale of stock (common and preferred) The cost of using external funding is the lowest rate of return that will ensure that lenders or stakeholders keep their funds in the business. This is debt funding – internally from the use of retained earnings (own money). The cost of using retained earnings is the opportunity cost (the foregone return) on investing these funds outside the business. This is equity funding

8. 4 The Cost of capital The Cost of Debt • The incremental cost of debt (as we consider new investments we are concerned with the marginal cost of debt, not the average cost) is the interest paid to lenders debt of new debt, net of tax. Since the interest payments on borrowed funds are allowed as a tax deduction from the investor’s income, the after-tax rate of interest is the true cost of debt • For example, if the investor borrows at 15% but faces a 40% marginal tax rate on their taxable income, the aftertax cost of the funds is not 15% but [15% – (40% of 15%)] = 15% – 6% = 9% • More generally, the after-tax cost of debt = interest rate (1 – tax rate)

8. 4 The Cost of capital The Cost of Equity • The cost of equity capital is the rate of return the investor must offer stockholders to induce them to invest in the venture. There is no tax adjustment to the cost of equity as dividends paid are not allowed as a tax deduction. (However, a business firm may pre-pay income tax due on the dividend and distribute dividend payments to shareholders with the income tax prepaid) • Purchasing stock in a business entails accepting some risk of not getting a return • Dividends vary with the company’s profits, so an investor assumes the risk associated with its performance

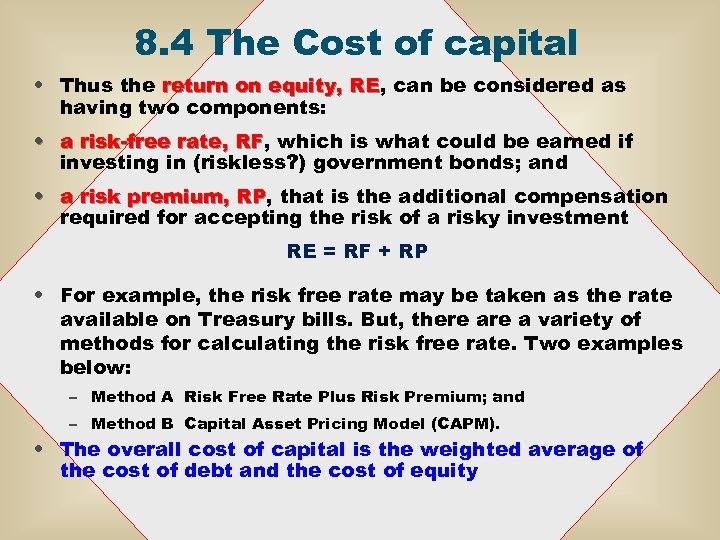8. 4 The Cost of capital • Thus the return on equity, RE, can be considered as RE having two components: • a risk-free rate, RF, which is what could be earned if RF investing in (riskless? ) government bonds; and • a risk premium, RP, that is the additional compensation RP required for accepting the risk of a risky investment RE = RF + RP • For example, the risk free rate may be taken as the rate available on Treasury bills. But, there a variety of methods for calculating the risk free rate. Two examples below: – Method A Risk Free Rate Plus Risk Premium; and – Method B Capital Asset Pricing Model (CAPM). • The overall cost of capital is the weighted average of the cost of debt and the cost of equity

8. 4 The Cost of capital Method A Risk Free Rate plus Risk Premium • The risk premium can be considered as having two components. First, there is the risk involved in investing in an option under consideration rather than buying government bonds. This is usually measured as the difference between the return on government bonds and that on the investor’s borrowing (e. g. , bonds). Second, when investors borrow from lenders they incur an obligation to pay interest to them; dividend payments are made only after interest payments are met. Therefore there is a risk involved in purchasing the stock of the company rather than lending to the company. This additional risk in purchasing the company’s stock rather than bonds is measured by the historic difference between yields on stocks and bonds issued by private companies, say, at about 4%. For example, if the return on government bonds is 10% and the return on the investor’s bonds is 13%, the total risk premium is RP = (13% – 10%) + 4% = 7% and the cost of equity is RE =RF + RP = 10% + 7% = 17%

8. 4 The Cost of capital Method B Capital Asset Pricing Model (CAPM) • This commonly used model takes into account the risk differential between a particular investor’s (say, company) stock and that of the stock of private investors/companies in general, as well as the differential between the investor’s stock and government securities. • The former differential is measured by an investor’s beta coefficient, which is the ratio of the variability (e. g. , as measured by the coefficient of variation) of return on their stock to the variability in the average return on the stock of all investors/companies. A beta coefficient of 1. 0 means that the particular investor’s stock is as variable in its return as the average; a coefficient of less than 1. 0 indicates less variability (less risk); greater than 1. 0 indicates more variability (greater risk). • Using this model, the cost of equity capital to the investor is measured by RE = RF + beta (RM – RF), where RM is the average return on the stock of all investors/companies.

8. 4 The Cost of capital • The second term is the beta coefficient times the risk premium on average stock, i. e. , the risk premium on holding stock of a particular investor/company. Therefore the cost of equity is equal to the risk free rate plus the particular investor’s risk premium. • For example, if the return on government bonds is 10%, the average return on stocks is 17% and the beta coefficient for a particular investor is 0. 75, then the cost of equity to the investor is RE = 10% + 0. 75 (17% – 10%) = 10% + 5. 25% =15. 25%

8. 4 The Cost of capital The Aggregate Cost of Capital • The overall cost of capital is the weighted average of the cost of debt and the cost of equity, where the weights are the proportions of the investor’s overall capital that comes from the two financing methods • For example, if the cost of debt is 8% and the cost of equity is 15%, and 30% of the company’s capital comes from debt and 70% from equity, the cost of capital is R = 8% x 0. 30 + 15% x 0. 70 = 12. 9% • Since debt financing is cheaper than equity finance, investors could lower their cost of capital by borrowing. However, they should not opt for debt financing (high gearing) exclusively. As gearing the proportion of their debt increases, lenders see the lending as being riskier and demand higher risk premium from heavilyindebted companies: the marginal cost of capital increases with gearing

8. 5 Life Cycle Costing (LCC) • LCC is normally defined as the sum of all funds expended in the acquisition, ownership and disposal of an asset or activity over its lifetime • LCC aims to optimise the life-long cost of an asset/activity by identifying all significant cost drivers over its lifetime • LCC is often confined to the cost side of the costbenefit evaluation; however, benefits are negative costs • In public investments, it is often assumed that benefits of alternative investment options are the same but cost streams are different. But, it is net worth that matters

8. 6 Portfolio investment • Diversification of risky activities and assets by acquiring portfolios of activities/assets to reduce the average risk • This could be used to tailor risk-return combinations to the requirements of particular investors, e. g. , pension funds, logistic managers or venture capitalists

8. 7 Real options considerations • Traditional approach to investment appraisal involves a projection of an investment opportunity into the future on the assumption that we can understand it and describe it in sufficient detail upfront. But, can we? • In this section, we look at areas where traditional methods of capital budgeting do not work well. These are: – strategic/radical and costly investments with a lot of uncertainty about how they might develop and operate; – investment projects that evolve and must adapt to changing environments; and – complex investment projects that involve many stakeholders such as prime contractors, original equipment manufacturers, maintainers, government agencies

8. 7 Real options considerations • Through these notes the term “investment option” has been used in contradistinction to “projects” or “investment proposals”. This is to emphasise the opportunity and associated right to engage in an investment activity before they become an obligation or a commitment • In contrast to traditional investment appraisal, real options approach involves decision making that is contingent on the revealed rather than assumed or projected reality. That is, a decision to proceed (to exercise an option to act) is only taken AFTER it act becomes apparent what the reality is like. The payoff is nonlinear – it changes depending on what the reality is: a different decision is made if the outcome is good than if it is bad (e. g. , the option may or may not be exercised)

8. 7 Real options considerations • Under real options approach monolithic and complex (hard to fathom) investment projects that must be approved or rejected upfront are fragmented into staged activities which can be assessed and accepted/rejected as they are revealed and understood, i. e. , firm decisions to proceed are taken when CONTINGENT EVENTS materialise and they are only taken as uncertainty is reduced, i. e. , new knowledge gained and the apparent risk better understood • There is a payoff in postponement of risky activity if it results in fewer regrets • In short, UNCERTAINTY CREATES OPPORTUNITIES

2. 6 Further reading Baye (2010): chs. 1 and 6 If you are interested in real options you may try to find and look up • Amram, M. And Kulatilaka, N. (1999) Real Options, Managing Strategic Investment in an Uncertain World, Harvard Business School Press, ch. 2 “Uncertainty creates opportunities”: 13 -28 • Dixit, A. K. and Pindyck, R. S. (1994) Investment under Uncertainty, Princeton University Press: ch. 2 “Developing the concepts through simple examples”: 26 -55