Unit 12 C Apportionment The House of Representatives

Unit 12 C Apportionment: The House of Representatives and Beyond Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 1

Apportionment n n For the United States House of Representatives, apportionment is a process used to divide the available seats among the states. More generally, apportionment is a process used to divide any set of people or objects among various individuals or groups. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 2

Example 1 The House has 435 representatives and is currently apportioned based on the 2010 census, which reported a U. S. population of 309 million. On average, using the 2010 population, how many people are represented by each representative? Suppose that the total number of representatives were the constitutional limit of one for every 30, 000 people. How many representatives would there be in that case? Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 3

The Standard Divisor and Quota n n The standard divisor is the average number of people per seat (in the House of Representatives) for the entire population of the United States: The standard quota for a state is the number of seats it would be entitled to if fractional seats were allowed: Note: These each also apply to apportionment problems besides that of the House of Representatives. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 4

Example 2 The 2010 census lists a population of 989, 000 for Montana and 564, 000 for Wyoming. Using these census data, find the standard quota for each state. The 2010 apportionment left each of these states with the constitutional minimum of one representative. Compare their standard quotas to their actual representations. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 5

Example 3 A small school district is reapportioning its 14 elementary teachers among its three elementary schools, which have the following enrollments Washington Elementary, 197 students; Lincoln Elementary, 106 students; and Roosevelt Elementary, 145 students. Find the standard quota of teachers for each school. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 6

Hamilton’s Method After finding the standard quota for each state: n n First, give each state the number of seats found by rounding its standard quota down. This number is the state’s minimum quota (or lower quota). If there any extra seats after each state has been given its minimum quota, look at each state’s fractional remainder—the fraction that remains in the standard quota after subtracting the minimum quota. Give one each of the extra seats to the states with the highest fractional remainders, until all the seats are gone. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 7

Fairness Issues with Hamilton’s Method n n n The Alabama paradox occurs when the total number of available seats increases, yet one state (or more) loses seats as a result. The population paradox occurs when a slowgrowing state gains a seat at the expense of a faster-growing state. The new states paradox occurs when the apportionment for existing states changes as a result of additional seats being added to accommodate a new state. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 8

Example 4 Apply Hamilton’s method to determine the apportionment of teachers among the schools in Example 3. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 9

Jefferson’s Method Begin by finding the standard divisor, standard quotas, and minimum quotas (as in Hamilton’s method). If there are no extra seats after each state has been given its minimum quota, then the apportionment is complete. If there are extra seats, start the computation over as follows. n Choose a modified divisor that is lower than the standard divisor. Use it to compute modified quotas by dividing the state’s population by the modified divisor. Round these modified quotas down to find a new set of minimum quotas. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 10

Jefferson’s Method n If there are just enough seats to fill all the minimum quotas, the apportionment is complete. Otherwise, do one of the following: 1. If there are still extra seats with the new minimum quotas, start again with a lower modified divisor. 2. If there are not enough total seats to fill at the minimum quotas, start again with a higher modified divisor (but still smaller than the standard divisor). Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 11

Application of Jefferson’s Method An application of Jefferson’s method to four states with a total population of 10, 000, with 100 available seats: Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 12

The Quota Criterion For a fair apportionment, the number of seats assigned to each state should be its standard quota rounded either up or down to the nearest integer. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 13

Example 6 Consider a four-state legislature with 100 seats, in which the states have the following populations: State A, 680; State B, 1626; State C, 1095; and State D, 6599. Use Jefferson’s method to apportion the 100 seats. Is the quota criterion satisfied? Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 14

Webster’s Method Webster’s method is similar to Jefferson’s method except that Webster’s method seeks modified quotas that give the correct total number of seats by using standard rounding rules. Note that the modified divisor in Webster’s method may be either greater or less than the standard divisor. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 15

Webster’s Method An application of Webster’s method to four states with a total population of 10, 000, with 100 available seats: Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 16

The Hill-Huntington Method The Hill-Huntington method is almost identical to Webster’s method, except it uses rounding based on the geometric mean of the integers on either side of the modified quota. The geometric mean of any two numbers x and y is Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 17

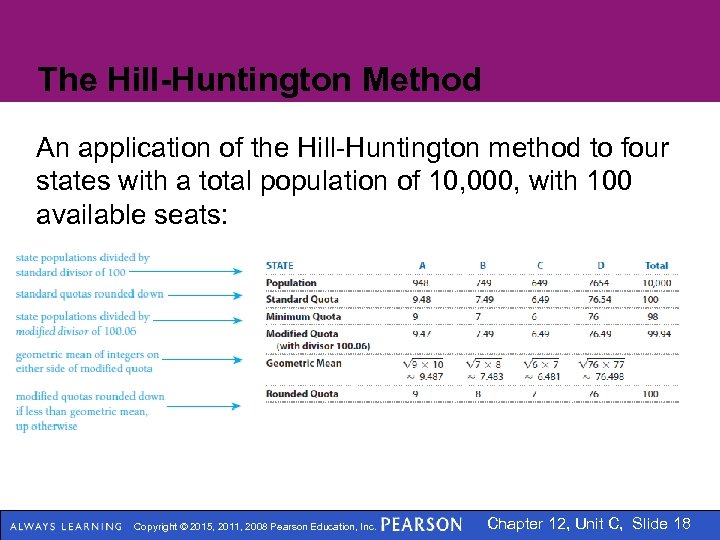The Hill-Huntington Method An application of the Hill-Huntington method to four states with a total population of 10, 000, with 100 available seats: Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 18

Best Method for Apportionment Mathematicians have studied the methods by running simulations of many possible apportionments. Results show that, like Jefferson’s method, Webster’s method and the Hill-Huntington method can also violate the quota criterion. However, the violations occur much less frequently with Webster’s or the Hill-Huntington method than with Jefferson’s method, making them arguably fairer. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 19

Best Method for Apportionment Is there any apportionment system that is unquestionably fair in all circumstances? Such an apportionment system would have to satisfy the quota criterion in all cases, while also being immune to the three paradoxes that affect Hamilton’s method. Unfortunately, a theorem proved by mathematicians M. L. Balinsky and H. P. Young states that such a system is impossible. In essence, the Balinsky and Young theorem for apportionment tells us that we cannot choose between apportionment procedures on the basis of fairness alone. Copyright © 2015, 2011, 2008 Pearson Education, Inc. Chapter 12, Unit C, Slide 20