TIPS JIM Meeting The JWST Point Spread Function Calculation

TIPS/JIM Meeting The JWST Point Spread Function: Calculation Methods and Expected Properties Russell B. Makidon Stefano Casertano, Colin Cox, & Roeland P. van der Marel Telescopes Group & JWST OTE / WFS&C Team Space Telescope Science Institute June 21, 2007 Russell B. Makidon

Importance of PSF Quality and Stability • The ability to obtain groundbreaking discoveries relies heavily on the quality and understanding of the telescope’s point spread function (PSF). – The Point Spread Function (PSF) describes the response of an imaging system to a point source or point object. • Critical elements: – that the PSF is of the highest possible quality – that the PSF is as stable as possible – that the PSF can be accurately modeled and understood during the data analysis stage. June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Changes in the HST PSF • HST has a stiff, monolithic, temperature-controlled primary mirror. • Changes in the HST PSF arise almost exclusively due to variations in the distance of the secondary mirror from the primary mirror. – Changes occur at the level of microns on orbital and secular timescales – Orbital “breathing” due to thermal variations associated with daynight transitions; Multi-year changes due to OTA desorption (150 microns since launch) – See M. Lallo et al. (2005), Instrument Science Report TEL 2005 -03 June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

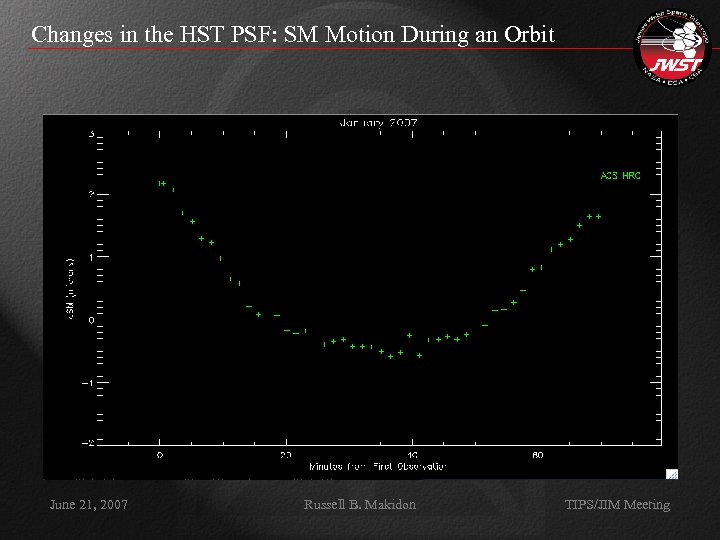Changes in the HST PSF: SM Motion During an Orbit June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

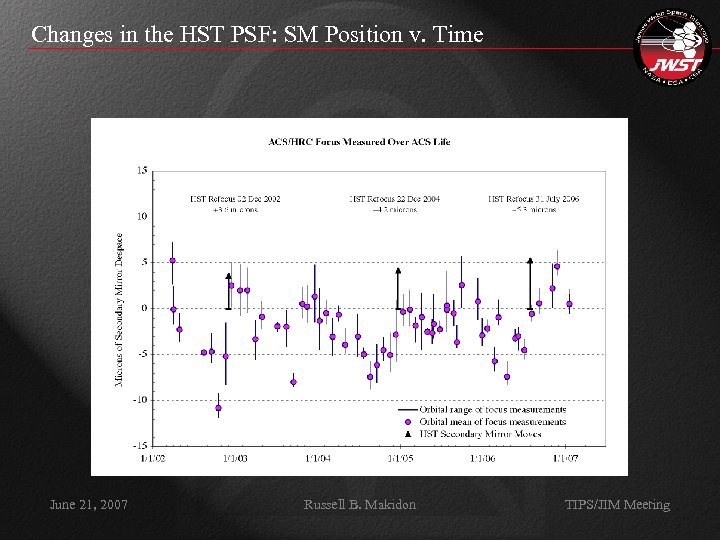Changes in the HST PSF: SM Position v. Time June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Challenges for JWST PSF Stability • The situation will be quite different for JWST. • The 6. 5 m primary mirror consists of 18 semi-rigid segments. – Each segment has 7 controllable degrees of freedom (tip, tilt, clocking, piston, two translations, and radius of curvature) – The secondary mirror has an additional 6 degrees of freedom (no radius of curvature correction). • JWST is passively cooled, but will never be fully in thermal equilibrium. • Thermal variations combined with of 132 degrees of freedom will yield a much higher-dimensional parameter space of JWST PSFs than for HST. June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Requirements on JWST WFE and WFS&C • A good, stable PSF is critically important for many types of science envisioned with JWST. • Requirements on image quality exist at a high level – Govern diffraction limit of JWST, change in encircled energy, and wavefront error (WFE) over the FOV (OBS-1607, OBS-88, OBS-90, OBS-1599) • Wavefront sensing and control (WFS&C) will enable correction of misalignments in the primary mirror segments and secondary mirror. – will ensure that the PSF never exceed the requirement of 131 nm RMS WFE over the Optical Telescope Element (OTE) field of view (FOV). • Many different PSFs are consistent with the JWST WFE budget. June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

PSFs Determined by Aperture Shape and WFE • To lowest order the PSF of an imaging system is determined by two things: the aperture shape and the wavefront errors. • Often, the shape of the aperture is well known and relatively simple – circular or annular apertures – more complex apertures increasingly common (JWST and Keck) • Errors in the wavefront arise from a variety of sources – imperfections in the system’s optics (static or semi-static) – atmospheric variations (as in the case for ground-based observations) – can be extremely difficult to determine. June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

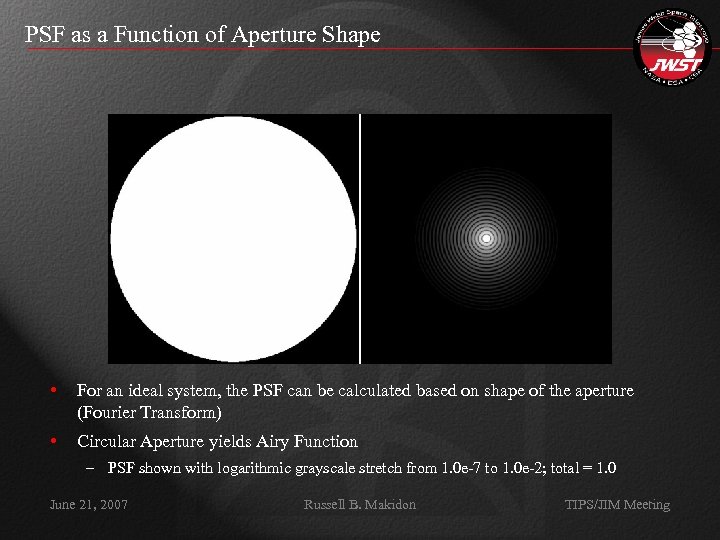PSF as a Function of Aperture Shape • For an ideal system, the PSF can be calculated based on shape of the aperture (Fourier Transform) • Circular Aperture yields Airy Function – PSF shown with logarithmic grayscale stretch from 1. 0 e-7 to 1. 0 e-2; total = 1. 0 June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

PSF as a Function of Aperture Shape • Hexagonal Aperture, 6. 5 m point-to-point – Six-fold symmetry in PSF – Flux in circular symmetric rings diffracted into “spikes” at 60 intervals June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

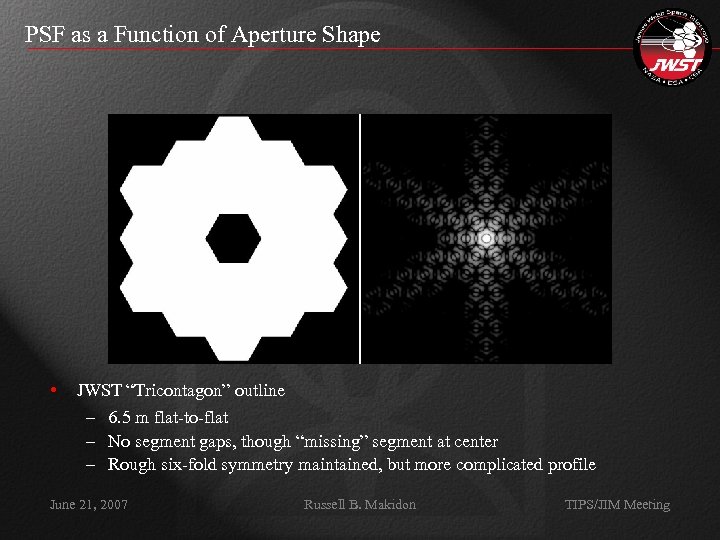PSF as a Function of Aperture Shape • JWST “Tricontagon” outline – 6. 5 m flat-to-flat – No segment gaps, though “missing” segment at center – Rough six-fold symmetry maintained, but more complicated profile June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

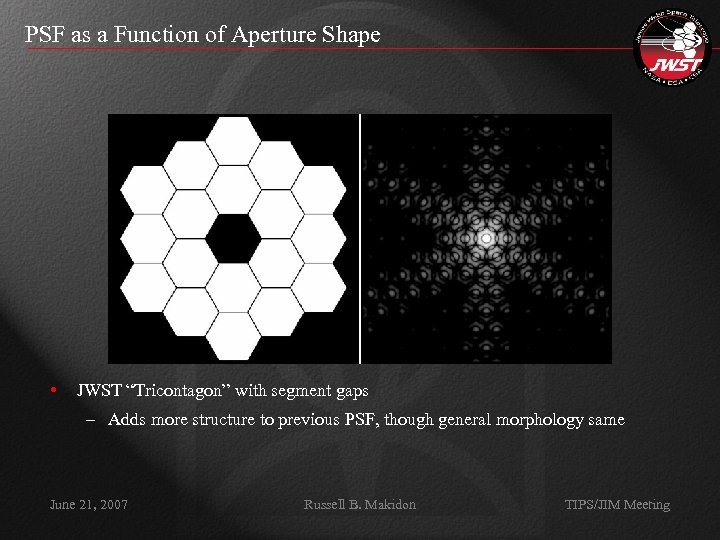PSF as a Function of Aperture Shape • JWST “Tricontagon” with segment gaps – Adds more structure to previous PSF, though general morphology same June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

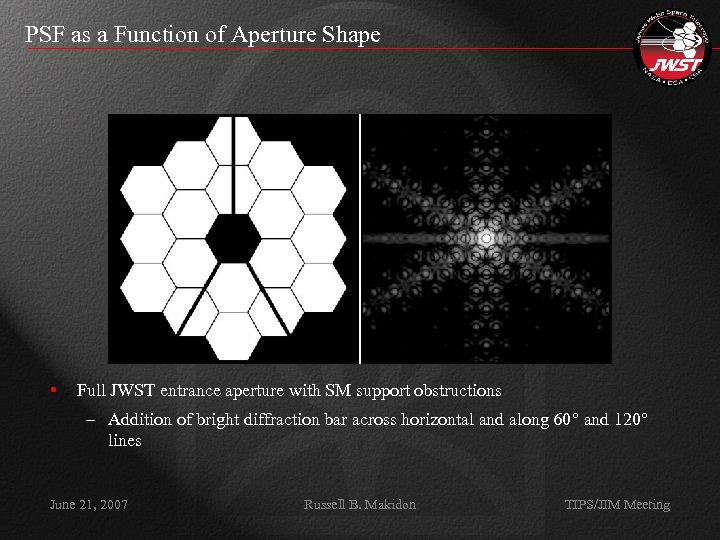PSF as a Function of Aperture Shape • Full JWST entrance aperture with SM support obstructions – Addition of bright diffraction bar across horizontal and along 60 and 120 lines June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

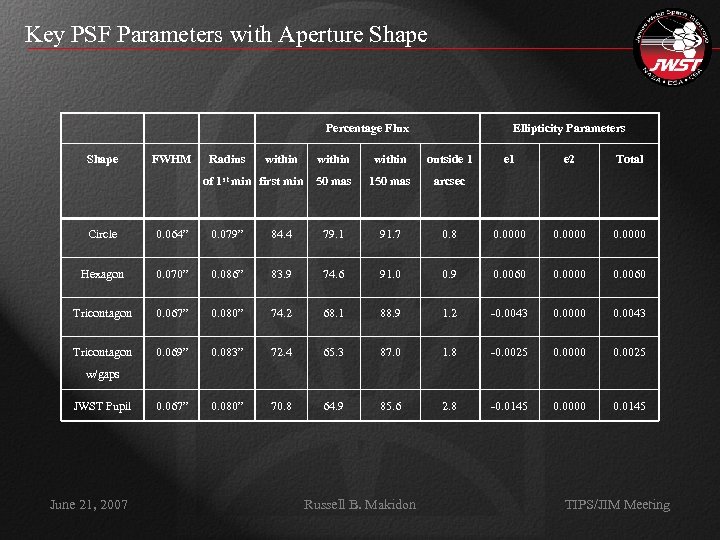Key PSF Parameters with Aperture Shape Percentage Flux Shape FWHM Radius within of 1 st min first min Ellipticity Parameters within outside 1 50 mas 150 mas e 1 e 2 Total arcsec Circle 0. 064” 0. 079” 84. 4 79. 1 91. 7 0. 8 0. 0000 Hexagon 0. 070” 0. 086” 83. 9 74. 6 91. 0 0. 9 0. 0060 0. 0000 0. 0060 Tricontagon 0. 067” 0. 080” 74. 2 68. 1 88. 9 1. 2 -0. 0043 0. 0000 0. 0043 Tricontagon 0. 069” 0. 083” 72. 4 65. 3 87. 0 1. 8 -0. 0025 0. 0000 0. 0025 0. 067” 0. 080” 70. 8 64. 9 85. 6 2. 8 -0. 0145 0. 0000 0. 0145 w/gaps JWST Pupil June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Optical Modeling Tools Used on JWST Project • Many different tools available to model JWST optical systems – Ray tracing codes: ZEMAX, CODE V, OSLO, MACOS, ASAP – Wavefront manipulation: PROPER, JWPSF, MACOS – Integrated modeling: ITM (Ball proprietary) • JWPSF (James Webb Point Spread Function) developed in-house (Cox and Hodge 2006) • Extensive experience with ZEMAX exists at STSc. I; familiarity with PROPER • STSc. I purchasingle CODE V license – All JWST optical models delivered to project as CODE V macros June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Calculation of PSFs using JWPSF • Calculated PSFs using Fourier Transform method, as implemented in JWPSF software – pervious PSFs: apertures without wavefront errors – subsequent PSFs: use JWST aperture and optical error budget realizations provided by Ball Aerospace (Optical Error Budget “Revision T”) • Optical Path Difference (OPD) describes the difference between a perfect wavefront and an aberrated wavefront – all points on the wavefront no longer in phase – result is a degraded PSF June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

PSF as a Function of OPD ma. CRIN + setvtrfe. Isea 0 mt. P= • MMRl +rn. FPO Sh. Ser t. R E 2 nw : : SMm s 0 i 1– I gi ta DT O ta Dm. O h Sµ P 1 0 • • • At left: perfect JWST aperture • At right: one realization of JWST Rev T optical error budget June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

PSF as a Function of OPD • Two realizations of JWST Rev T optical error budget • OPD at left: 110. 3 nm RMS • OPD at right: 109. 6 nm RMS • Measurable triangularity in PSF core at left; relatively circular core in PSF at right. • PSFs at = 2. 0 µm June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

NIRCam PSFs at 2. 0µm Sharpness Ellipticity mean min, max F 070 W 0. 155 0. 026 0. 107, 0. 194 F 200 W 0. 076 0. 002 0. 073, 0. 082 June 21, 2007 Flux inside 0. 15” e 1 e 2 0. 72 0. 01 0. 046 0. 154 -0. 031 0. 147 0. 80 0. 014 0. 024 -0. 010 0. 030 (total = 1. 0) Russell B. Makidon TIPS/JIM Meeting

PSF as a Function of OPD and Wavelength • Two realizations of JWST Rev T optical error budget • OPD at left: 110. 3 nm RMS • OPD at right: 109. 6 nm RMS • PSFs at top: F 070 W • PSFs at bottom: F 200 W • PSFs shown on same angular scale; same logarithmic grayscale June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

PSF Variation with Wavelength • Quantified radial PSF profile and encircled energy for broadband NIRCam filters • Cases shown for single input OPD • PSFs approach ideal for long wavelengths; still very good at short wavelengths June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

PSF Variation with Wavelength • Encircled energy within 0. 15 arcsec shows peak at = 2. 0 µm (diffraction limit) • PSFs core width continues to improve toward short wavelengths (despite absence of requirements) June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Now and the Near Future • Currently able to do relatively simple optical analyses using a combination of JWPSF and ZEMAX • PROPER available, though STSc. I experience is limited – has been used to support NIRCam coronagraph studies – segmented primary; generation of OPDs • Obtaining CODE V as a means to vet current models. – All JWST optical models delivered to the Project as CODE V macros June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

ZEMAX Model: Monolithic JWST and NIRCAM • Monolithic primary with full NIRCam optical train • Useful to adjust positions of optical elements, and determine OPDs due to defocus, misalignment, etc. – Add changes to Ball OPDs June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Using ZEMAX Predictions with JWPSF • Left: PSF in F 187 N for single Rev T OPD error realization. • Right: PSF in F 187 N for same Rev T OPD error realization – added 0. 2 waves of defocus to Ball-supplied OPD map using the predictions ZEMAX model with monolithic JWST primary mirror June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

PSF as a Function of OPD and Wavelength • Left: PSF in F 187 N for single Rev T OPD error realization. – Top from JWPSF – Bottom from PROPER • Right: PSF in F 187 N for same Rev T OPD error realization – Top: ZEMAX model with monolithic JWST primary mirror; 0. 2 waves defocus – Bottom: PROPER with 0. 15 waves of defocus • Zernike normalization issue? June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Conclusions • Presented and explored various methods to calculate the PSF http: //www. stsci. edu/jwst/externaldocs/technicalreports • Presented PSF properties of astronomical interest given current understanding of telescope design • Understanding JWST PSF will be a challenge – First step toward providing an understanding of the PSF useful for JWST observers • Start of development of tools of use to S&OC developed to address tradeoffs between PSF quality and operations scenarios • JWST compares favorably with HST at wavelengths as short as 0. 70 microns; far exceeds capabilities of NICMOS at NIR wavelengths. June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Comparison: HST/ACS with JWST NIRCam Images courtesy M. Stiavelli • ACS image of HUDF in V, I, and Z-bands (left) and simulated JWST NIRCam image in F 070 W, F 090 W, and F 115 W (right) June 21, 2007 Russell B. Makidon TIPS/JIM Meeting

Comparison: HST/ACS with JWST NIRCam Images courtesy M. Stiavelli • ACS image of HUDF in V, I, and Z-bands (left) and simulated JWST NIRCam image in F 070 W, F 090 W, and F 115 W (right) June 21, 2007 Russell B. Makidon TIPS/JIM Meeting