This is a Power Point presentation on market

This is a Power. Point presentation on market processes. A left mouse click or the enter key will add and element to a slide or move you to the next slide. The back space key will take you back an element or slide. If you wish to exit the presentation, the escape key will do it! 1 R. Larry Reynolds ã 1997

Markets as Allocative Process · Markets are a social process that allocates scarce resources and goods among alternative uses. · A market exchange is a transfer of property rights based on “quid pro quo” [“I will give you this for that!”] · Market exchange · barter · monetized 2

Barter · Barter is an market exchange that is a trade of one good for another · Barter can be costly · double coincidence of wants · transaction costs of information and trade · Exchange ratio is the relative amounts of one good given in exchange for another 3

Monetized Economy · Because the transaction costs of barter can be significant, societies often adopt the use of a numeraire good, “money, ” to reduce those costs. · Money as a medium of exchange makes exchange easier · commodity money · fiat money · Exchange ratios expressed in monetary terms 4

Consider a closed economy with 50 people, each given one case of cola and one case of beer. This distribution does not guarantee that the welfare or utility of the group is maximized. some people like cola but not beer, others like beer but not cola, others might like neither or both. How could these 50 individuals increase their utility? Voluntary exchange! Individuals who do not like beer could trade for cola, those who like cola could trade for beer. What they lack is information about the terms of trade and who will trade with them. To acquire this information they offer to trade a few bottles of what the don’t like for bottles of what they do like. 5

Through trading and observing others who trade, an “exchange ratio” tends to emerge. On average two colas [2 C] seems to trade for one beer [1 B]. 2 C = 1 B. This exchange ratio reflects the initial distribution of cola and beer, the preferences of the individuals and the abilities to acquire information. A different distribution or a group of individuals with different preferences would result in a different exchange ratio. Notice that this example does not explain the process of how to decide “HOW MUCH OF EACH SHOULD BE PRODUCED. ” Rather than look for individuals who are willing to trade and then haggling, a numeraire good [money] may be used. The numeraire good is universally acceptable so individuals will accept it in payment even though it is not the good they want. . 6

The exchange ratio that emerges from the trading determines a set of relative prices. Using the example 2 C = 1 B, a price of $1 for colas [Pc = 1] implies a price of $2 for beer [Pb= 2] or, [Pb= 1] would imply [Pc =. 50] or, [Pc = 2] implies [Pb = 4], These relative prices represent the preferences of the individuals, the initial distribution and abilities to acquire information or bargain [haggle]. It is the relative prices of beer and cola that reflect relevant information! Market exchange is a process that provides information [relative prices] and incentives that coordinates the choices of individuals. . 7

These relative prices, when established through voluntary exchange reflect the relative value of each good to the group. Relative prices can be altered by changes in: a. the preferences of individuals in the group, b. the distribution of the initial endowment {relative incomes of the group}, c. the relative amounts of the goods, d. the information and bargaining abilities of the individuals, e. or, . . . Each individual engaging in a voluntary exchange is “better off” or “no worse off; ” a move toward a Pareto Efficient solution. Utilitarianism is the Philosophy that rationalizes free market economies!. . 8

Utilitarianism · Utilitarianism is the underlying philosophy of the market system · Jeremy Bentham [1748 -1832] · Philosophy of market system is based on each individual attempting to optimize [maximize] their individual welfare or utility · Maximize the welfare or utility of the members of society · utility of individuals is “additive, ” [if each person maximizes their welfare it maximizes the welfare of society] 9

Jeremy Bentham [1748 -1832] Jeremy Bentham was trained in the law but became interested in social philosophy. His major contribution to economics is the philosophy of Utilitarianism. It became the basic principle underlying the philosophy of Western, industrial market economies. In one of his major books, An Introduction to the Principles of Morals and Legislation [1789] he attempts to show the welfare of society can be increased. 10

Coordination of Individual Actions · Individuals, organizations and society may have competing interests · Markets are one way in which the competing interests and subsequent actions can be coordinated · Market philosophy is based on individuals having information and acting on that information in “rational” ways 11

Adam Smith [1723 - 1790] · Popularized the idea that markets could be a dominant allocative mechanism in society · “But man has almost constant occasion for the help of his brethren, an it is vain for him to expect it from their benevolence only. He will be more likely to prevail if he can interest their self-love in his favor, and shew them that it is for their own advantage to do for him what he requires of them. Whoever offers to another a bargain of any kind proposes to do this. Give me that which I want , and you shall have this which you want, is the meaning of every such offer; . . . It is not from the benevolence of the butcher, the brewer, or the baker, that we expect our dinner, but from their regard to their self interest. ” Wealth of Nations, 1776 12

Need For Justice · In the book Theory of Moral Sentiments, Smith argues: · “Justice, on the contrary, is the main pillar that upholds the whole edifice. If it is removed, the great, the immense fabric of human society, that fabric which, to raise and support, seems, in this world, if I may so, to have been the peculiar and darling care of nature, must in a moment crumble into atoms. ” Theory of Moral Sentiments 1759 13

Markets, Justice and Jurisprudence · Adam Smith’s system included three segments; · A Theory of Moral Sentiments [1759] · Argued that Justice was crucial for a society · An Inquiry into the Nature and Causes of the Wealth of Nations [1776] · argued the importance of markets and self interest · A third book, on jurisprudence [Lectures on Jurisprudence], was burned about the time of his death and showed the importance of law 14

Totals, Averages, Marginals and Optimization · · total, average and marginal values are used to describe relationships in benefit, utility, revenue, product, cost, . . . There is a mathematical relationship between totals, averages and marginals These relationships can be used to evaluate alternative choices with respect to different alternatives These relationships will be demonstrated using revenue. The principles are the same with benefits or utility. 15

Totals · · · Total Revenue is defined as Price times Quantity TR = PQ. For 2 goods, X and Y, TR = Px. Qx +Py Qy. This can be expanded to include as many goods as required. Note that this is also the total expenditure. Total cost is defined as the total expenditure to produce a given output. TC = PL L + PK K+. . . + PR R, where L = Labour, K = kapital, R = land [as many inputs as you want can be included. Sometimes inputs are classified as “variable or fixed. ” The cost of the variable inputs is VC, the cost of the fixed inputs is FC. In this case; TC = VC + FC 16

A relationship between the price of a good [P] and the quantity purchased [Q] is estimated through observation and statistical analysis. Q = 10 -. 5 P This can also be expressed: P = 20 - 2 Q Since we usually identify the horizontal axis as Q [quantity], the equation for the graph that we typically construct is; P = 20 - 2 Q Total Revenue can be calculated: TR º PQ, TR = (20 -2 Q)Q, or TR = 20 Q- 2 Q 2 R= 20 A P AR = TR/Q, AR = 20 - 2 Q [ AR = P] DTR MR = or, DQ MR = 20 - 4 Q nd ma De TR MR 10 Q Demand, AR, TR and MR can also be shown using a table. . . 17

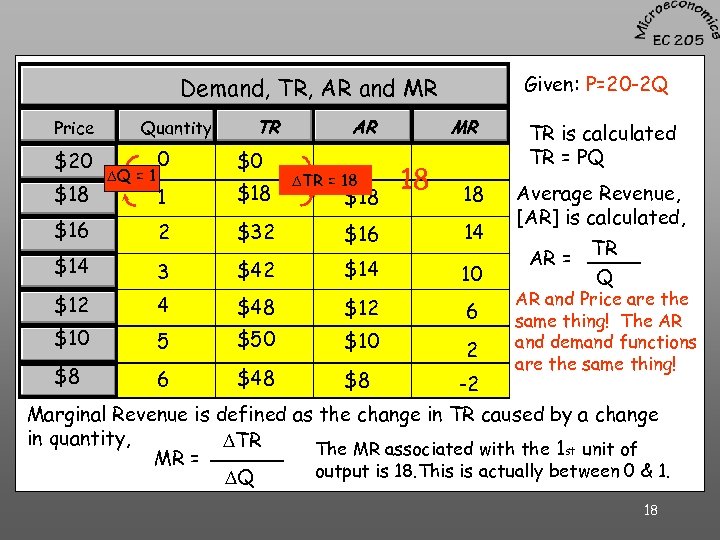Given: P=20 -2 Q Demand, TR, AR and MR Price Quantity TR AR MR 0 $0 1 $18 2 $32 $16 14 $14 3 $42 $14 10 $12 4 $48 $12 $10 6 5 $50 $10 $8 2 6 $48 $8 $20 $18 $16 DQ = 1 DTR = 18 $18 18 18 TR is calculated TR = PQ Average Revenue, [AR] is calculated, AR = TR Q AR and Price are the same thing! The AR and demand functions are the same thing! -2 Marginal Revenue is defined as the change in TR caused by a change in quantity, DTR The MR associated with the 1 st unit of MR = output is 18. This is actually between 0 & 1. DQ 18

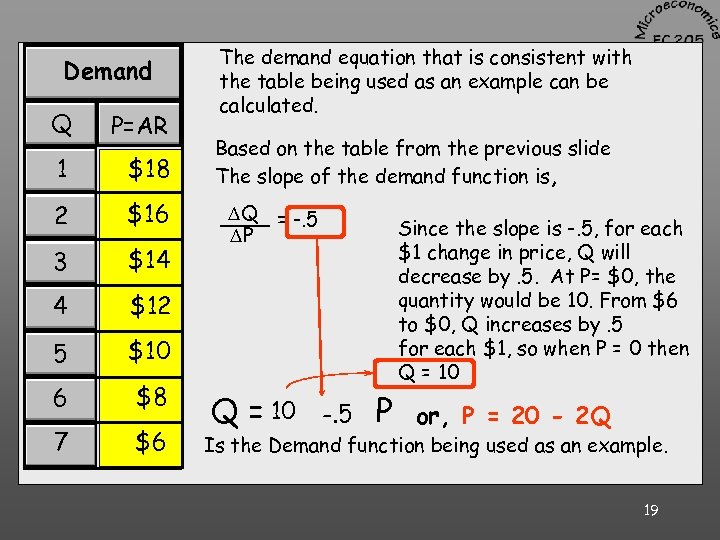Demand Q P=AR 1 $18 2 $16 3 $14 4 $12 5 $10 6 $8 7 $6 The demand equation that is consistent with the table being used as an example can be calculated. Based on the table from the previous slide The slope of the demand function is, DQ = -. 5 DP Q = 10 --. 5 P a m Since the slope is -. 5, for each $1 change in price, Q will decrease by. 5. At P= $0, the quantity would be 10. From $6 to $0, Q increases by. 5 for each $1, so when P = 0 then Q = 10 or, P = 20 - 2 Q Is the Demand function being used as an example. 19

Demand, TR, AR and MR Price $20 $18 $16 $14 Quantity 0 1 2 TR $0 $18 $32 AR MR $18 18 14 $42 $10 3 4 5 $16 $14 $48 $50 $12 $10 $8 6 $48 $8 10 6 2 -2 MR is the change in TR [DTR]“caused” by a change in Q [DQ]. In principles of econ we will let DQ = 1. MR is then the change in TR that results from a 1 unit change in Q. When the table has DQ in one unit increments, TR can be calculated by s subtraction. as Q increases from 0 to 1, TR increases by 18 [18 -0] as Q increases from 1 to 2, TR increases by 14 [32 -18] 20

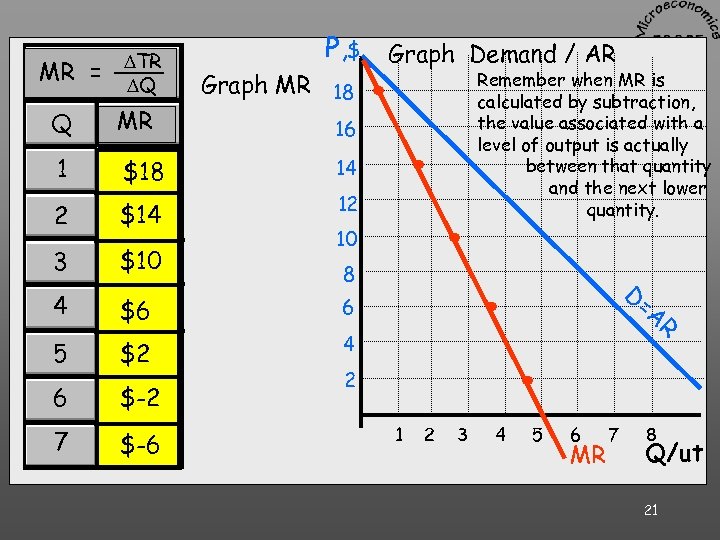Demand MR = DTR DQ . P, $ Graph Demand / AR Graph MR 18 Q MR P=AR 1 $18 2 $16 $14 12 3 $14 $10 4 $12 $6 6 5 $10 $2 4 6 $8 $-2 7 $6 $-6 . 14 Remember when MR is calculated by subtraction, the value associated with a level of output is actually between that quantity and the next lower quantity. 16 10 . 8 . 2 1 2 3 4 D= AR . 5 6 MR 7 8 Q/ut 21

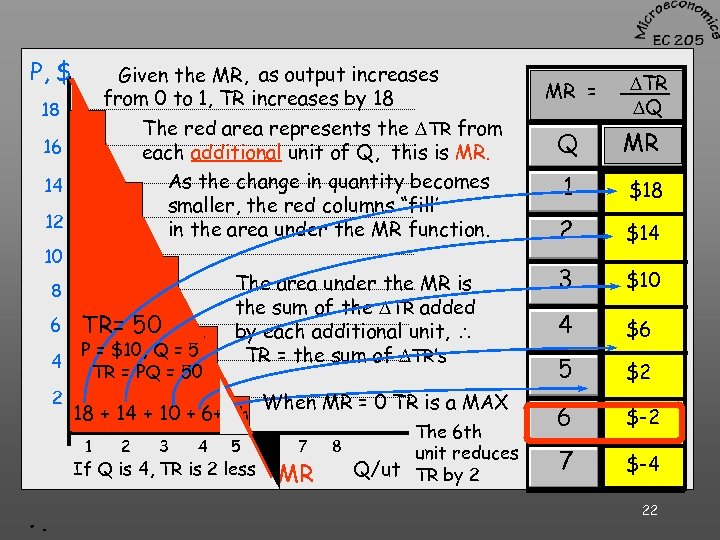12 10 8 6 4 2 TR= 50 DTR of 4 rth 14 DTR from firs unit 16 DTR from 3 unit 18 Given the MR, as output increases from 0 to 1, TR increases by 18 The red area represents the DTR from each additional unit of Q, this is MR. As the change in quantity becomes smaller, the red columns “fill’ in the area under the MR function. DTR from second unit P, $ P = $10, Q = 5 TR = PQ = 50 The area under the MR is the sum of the DTR added by each additional unit, TR = the sum of DTR’s 5 th 18 + 14 + 10 + 6+2 1 2 3 4 5 If Q is 4, TR is 2 less . . When MR = 0 TR is a MAX 6 7 MR The 6 th 8 unit reduces Q/ut TR by 2 MR = DTR DQ Q MR 1 $18 2 $14 3 $10 4 $6 5 $2 6 $-2 7 $-4 22

P, $ 18 As price increases. TR decreases. TR can also be related to AR or the demand function. ep|> 1; elastic At a price of $16, Q is 2: TR = PQ = 32 16 14 TR=PQ 12 at P = $12 TR = $10 x 5=$50 Q = 4; 16 x 2=maximum 8 The TR = 32=TR $48 10 6 4 2 . ep = -1 As price decreases, TR decreases. D= TR is at$8, Q=6 At P = the “midpoint” of TR = $48 a linear demand. At a price of $4, Q is 8: TR = PQ = 32 1 2 3 4 5 Remember that the ep = -1 at the point where TR is a Maximum. 6 AR 7 |ep| < 1; inelastic 8 Q/ut 23

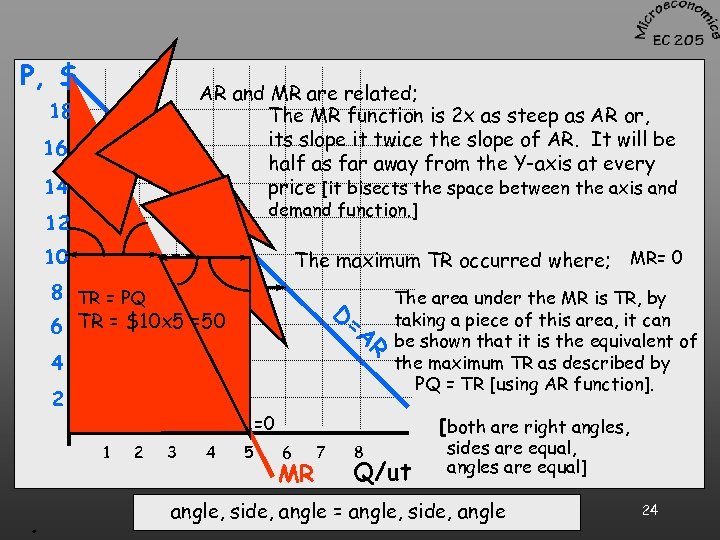P, $ AR and MR are related; The MR function is 2 x as steep as AR or, its slope it twice the slope of AR. It will be half as far away from the Y-axis at every price [it bisects the space between the axis and 18 16 14 demand function. ] 12 10 The maximum TR occurred where; MR= 0 3 3 8 TR = PQ 6 TR = $10 x 5 =50 D= AR 4 2 MR=0 1 . The area under the MR is TR, by taking a piece of this area, it can be shown that it is the equivalent of the maximum TR as described by PQ = TR [using AR function]. 2 3 4 5 [both are right angles, 6 MR 7 8 Q/ut sides are equal, angles are equal] angle, side, angle = angle, side, angle 24

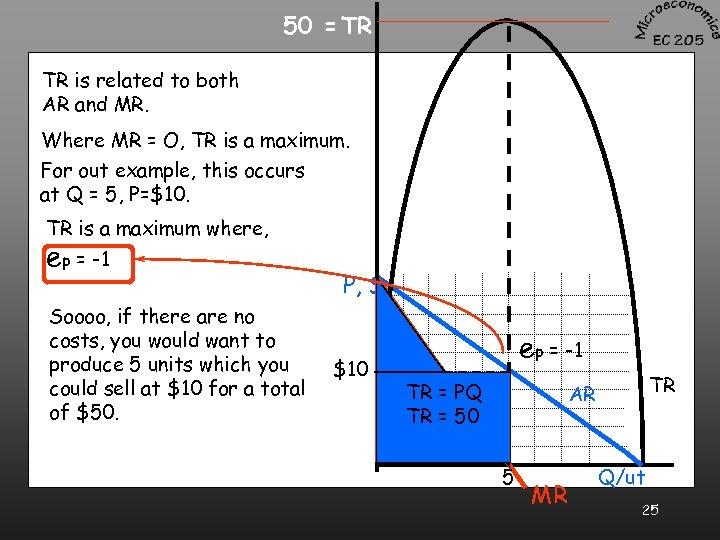50 = TR TR is related to both AR and MR. Where MR = O, TR is a maximum. For out example, this occurs at Q = 5, P=$10. TR is a maximum where, ep = -1 Soooo, if there are no costs, you would want to produce 5 units which you could sell at $10 for a total of $50. P, $ $10 max TR ep = -1 TR = PQ Sum of 50 TR = DTR’s TR AR 5 MR Q/ut 25

Consider the choices of picking blackberries. Instead of TR you want to maximize Total utility [TU] or Total Benefit [TB] As more and more blackberries are picked, the marginal benefit [MB] of each additional unit of blackberries decreases. A rational person would pick the easiest blackberries first, therefore as they pick additional units of fruit the marginal cost rises. Marginal Decision Rule: When the objective is to maximize net benefits from an action, MB > MC Do it! [or MR > MC] Net benefits are maximized where MB = MC MB < MC Don’t do it! [or MR < MC] 26

Picking Blackberries [BB] As more BB are picked, the effort required to pick an additional unit rises. Hence, the MC has a positive slope. MC, d A MB MC MC =MB C To maximize the net benefits, pick BB until MC = MB As the amount of BB available f MB for consumption in a period 0 of time increases, each 1 BB/ut additional unit adds a smaller amount of utility; the MB function declines. The marginal decision rule indicates you should pick the first unit of BB The MB of the first unit exceeds the MC of the first unit, MB > MC The benefit associated with the first unit of BB is the blue area 01 Ad. This is the MB associated with the first unit of BB. The cost associated with the first unit of BB is the yellow area 01 Cf. This is the MC associated with the first unit of BB The net benefits that result from the first unit of BB is the red area f. CAd. . 27

If one unit less is picked, [X-1], the net benefit declines. MC, MB MC t MC=MB e r Net benefits are reduced by area ret. 0 w To maximize the net benefits, pick s [X-1] X [X+1] If one more unit is picked [X+1], net benefits are reduced by area esw BB until MC = MB MB BB/ut Net benefits are maximized where MC = MB, profits will be maximized where MR = MC. . 28

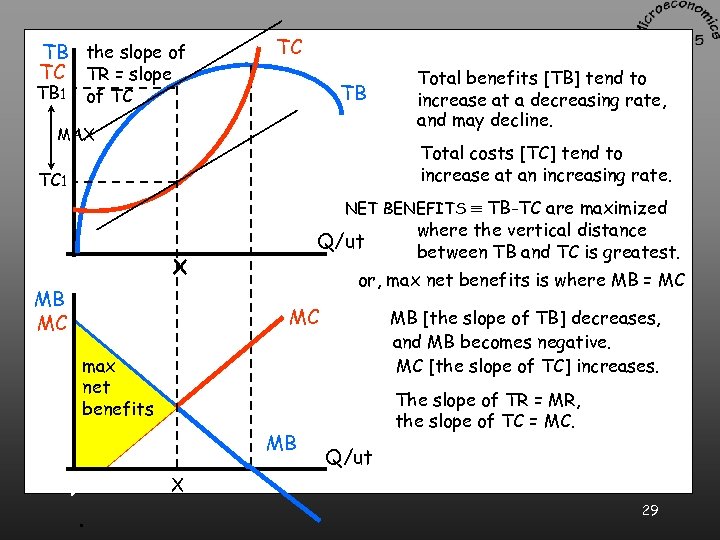TB the slope of TC TR = slope TB 1 TC TB of TC MAX Total benefits [TB] tend to increase at a decreasing rate, and may decline. Total costs [TC] tend to increase at an increasing rate. TC 1 NET BENEFITS Q/ut X MB MC º TB-TC are maximized where the vertical distance between TB and TC is greatest. or, max net benefits is where MB = MC MC MB [the slope of TB] decreases, and MB becomes negative. MC [the slope of TC] increases. max net benefits MB The slope of TR = MR, the slope of TC = MC. Q/ut X . 29

Highest Valued Use · An important function of markets is to insure that resources are used in their highest valued use. · markets can be viewed as an “information system” that uses relative prices as a signaling mechanism · individuals react to relative prices in an attempt to optimize the achievement of their objectives 30

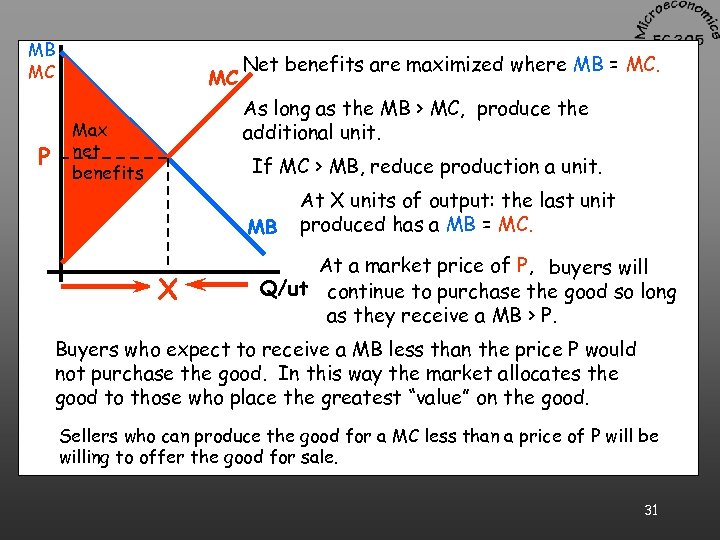MB MC P MC Net benefits are maximized where MB = MC. As long as the MB > MC, produce the additional unit. Max net benefits If MC > MB, reduce production a unit. MB X X At X units of output: the last unit produced has a MB = MC. At a market price of P, buyers will Q/ut continue to purchase the good so long as they receive a MB > P. Buyers who expect to receive a MB less than the price P would not purchase the good. In this way the market allocates the good to those who place the greatest “value” on the good. Sellers who can produce the good for a MC less than a price of P will be willing to offer the good for sale. 31

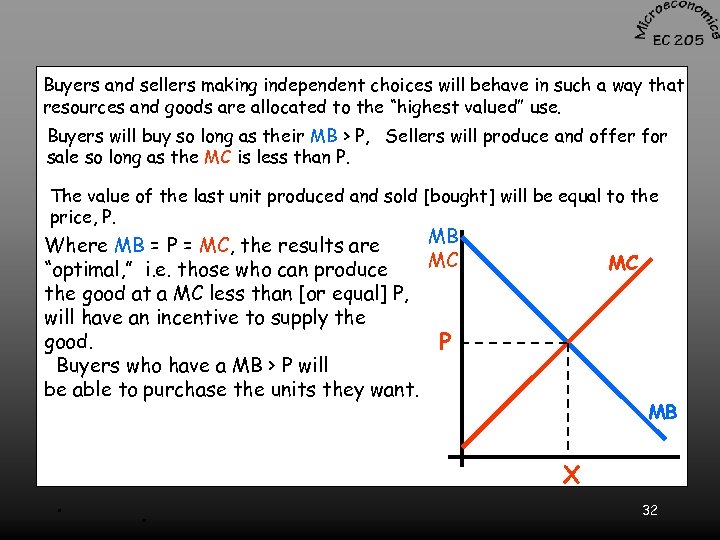Buyers and sellers making independent choices will behave in such a way that resources and goods are allocated to the “highest valued” use. Buyers will buy so long as their MB > P, Sellers will produce and offer for sale so long as the MC is less than P. The value of the last unit produced and sold [bought] will be equal to the price, P. MB Where MB = P = MC, the results are MC “optimal, ” i. e. those who can produce the good at a MC less than [or equal] P, will have an incentive to supply the good. P Buyers who have a MB > P will be able to purchase the units they want. . MC MB X. 32

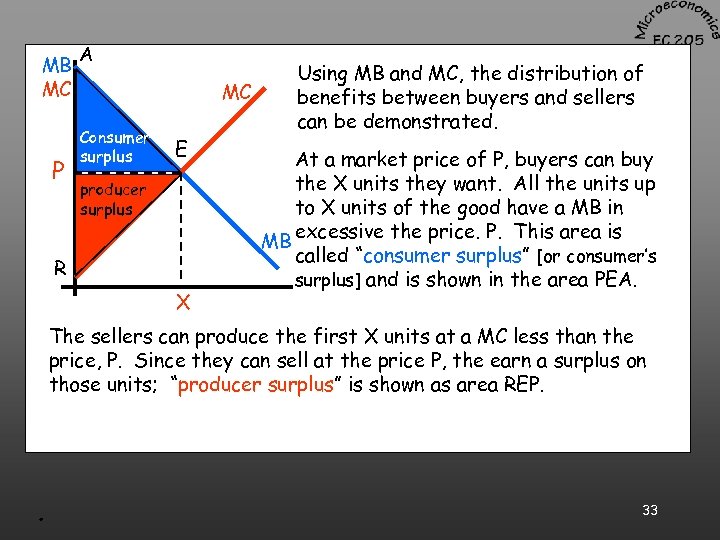MB MC P A MC Consumer surplus E producer surplus R X Using MB and MC, the distribution of benefits between buyers and sellers can be demonstrated. At a market price of P, buyers can buy the X units they want. All the units up to X units of the good have a MB in excessive the price. P. This area is MB called “consumer surplus” [or consumer’s surplus] and is shown in the area PEA. The sellers can produce the first X units at a MC less than the price, P. Since they can sell at the price P, the earn a surplus on those units; “producer surplus” is shown as area REP. . 33

MB = P = MC Under ideal circumstances the market will produce the ideal result: The market price [P] will represent the value of the last unit that any buyer is willing to purchase. This same price [P] also represents the cost of producing that last unit! The “ideal” is the benchmark that we use to gauge the performance of the processes that actually occur. When MB ¹ P ¹ MC, there is a problem. It is the job of the economic analysis to determine why the market forces have not established a price that reflects the conditions of buyers and sellers. . 34

Price Distortions · “Noncompetitive markets” i. e buyers or sellers have the power to influence the market price · monopoly, monopsony, oligopoly, oligopsony, imperfect competitive markets, . . . {structure} · collusion, price discrimination, . . . {process or behavior} · Property rights problems · externalities · collective or public goods · common property resources 35

Noncompetitive Markets · Monopoly -- a single seller of a good with no close substitutes. · Monopsony -- a single buyer of a good. · oligopoly -- a “few” sellers who recognize their interdependence. · oligopsony -- a “few” buyers who recognize their interdependence. · imperfect competition, monopolistic competition · pure competition -- the “ideal” market structure that results in P = MR = MC = AR = ATC 36

Competition · Pure competition as the ideal -- a structural concept of competition. · large number of buyers and sellers no one of which can influence the market · homogeneous product · relatively free entry and exit · Competition as rivalry -- a process concept of competition. 37

Property Rights · For markets to produce optimal results, nonattenuated property rights are required · exclusive · transferable · enforceable · “weakened” or “attenuated” property rights result in” · externalities · collective or public goods · common property resources 38

Externalities -- A market transaction imposes a cost [negative externality] or confers a benefit [positive externality] on another person(s) who is (are) not involved with the market transaction. When all costs and benefits are recognized by the parties in the transaction, MB = P = MC. A negative externality imposes a cost on a person(s) who is not included as a party to the exchange. these costs must be added to the MC that was recognized as part of the exchange. MC + external costs MB MC MC P’ P P is the market price but, the higher price, P’, correctly reflects MB & MC. MB X 0 X The parties to the exchange will agree to X amount as optimal, if the externality had been included, only X 0 would be optimal. 39

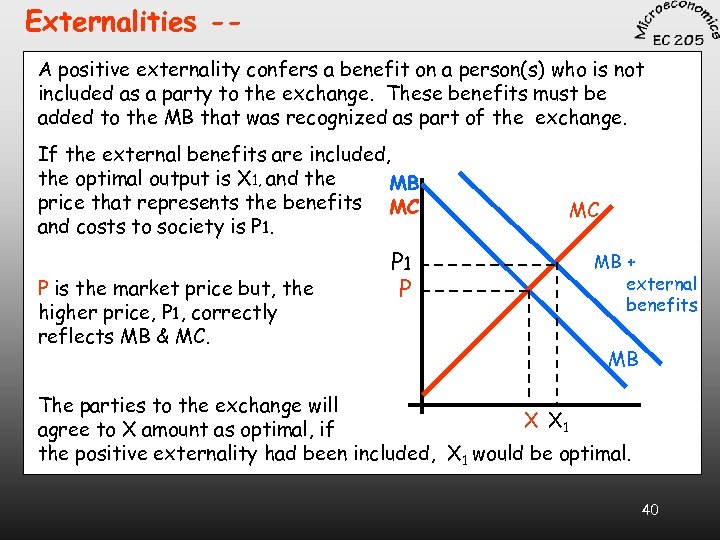Externalities -A positive externality confers a benefit on a person(s) who is not included as a party to the exchange. These benefits must be added to the MB that was recognized as part of the exchange. If the external benefits are included, the optimal output is X 1, and the MB price that represents the benefits MC and costs to society is P 1. P is the market price but, the higher price, P 1, correctly reflects MB & MC. P 1 P MC MB + external benefits MB The parties to the exchange will X X 1 agree to X amount as optimal, if the positive externality had been included, X 1 would be optimal. 40

Public or Collective Goods When it is impossible to exclude individuals from using a good and their use of that good imposes no opportunity cost, or MC on society, that good is called a collective or public good. National defense is the most often used example of a collective good. After a given level of national defense is provided, a new member of society cannot be excluded from that protection and the additional person protected does not increase the MC of national defense. Since the individual cannot be excluded, the have no incentive to pay for the good even though they benefit from it; they become “free riders. ” To pay for the collective good government may tax the individuals and provide the good. In which case individuals may become “forced riders. ” A quasi-public good is like a road or park, we could exclude an individual from using the good, but it may not be desirable to do so. Because of the problems associated with collective goods and quasi-public goods, markets do not result in optimal allocations. 41

Common Property Resources These are resources that are held “in common, ” or are “fugitive” resources; whoever captures the resources acquires the right to use it. Unlike collective or public goods, common property resources are subject to a marginal cost for their use. If I get it, you don’t. In the case of exhaustable or depletable resources, individuals tend to capture as much as they can as quickly as they can. Resources such as buffalo. whales, carrier pigeons, oceans, air, [biosphere], common lands, etc. tend to be “overused” and may be driven to extinction or depletion. . . 42

Summary · Markets are a social institution that will coordinate individuals’ behavior · Problems develop when · lack of competition · property rights are not; · exclusive · enforceable · transferable 43