Скачать презентацию Theorem of rational roots This method is mainly Скачать презентацию Theorem of rational roots This method is mainly

Theorem of rational roots.pptx

  • Количество слайдов: 8

Theorem of rational roots This method is mainly useful for solving high order polynomials Theorem of rational roots This method is mainly useful for solving high order polynomials i. e. When the power of x is greater than 4. Quadratics, we can solve in many ways (graphing, trial and improvement, factorising, formula, etc) For cubics and quartics we can use factor theorem to find a root (if the root is rational i. e. can be written as fraction p/q) and then use methods of division or Horner’s etc to find other roots.

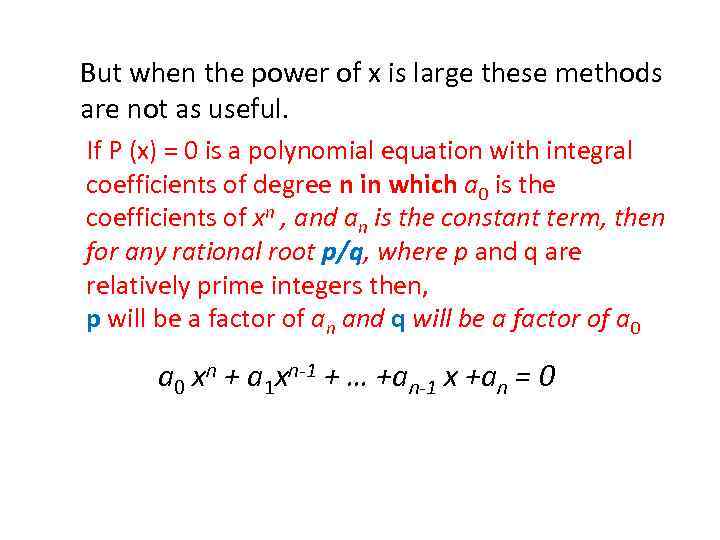But when the power of x is large these methods are not as useful. But when the power of x is large these methods are not as useful. If P (x) = 0 is a polynomial equation with integral coefficients of degree n in which a 0 is the coefficients of xn , and an is the constant term, then for any rational root p/q, where p and q are relatively prime integers then, p will be a factor of an and q will be a factor of a 0 xn + a 1 xn-1 + … +an-1 x +an = 0

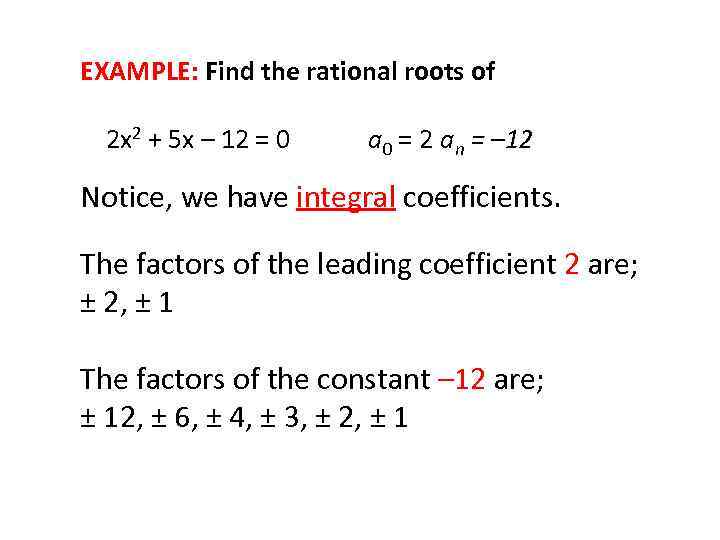EXAMPLE: Find the rational roots of 2 x 2 + 5 x – 12 EXAMPLE: Find the rational roots of 2 x 2 + 5 x – 12 = 0 a 0 = 2 an = – 12 Notice, we have integral coefficients. The factors of the leading coefficient 2 are; ± 2, ± 1 The factors of the constant – 12 are; ± 12, ± 6, ± 4, ± 3, ± 2, ± 1

Now, we take each of the factors of the constant and put them over Now, we take each of the factors of the constant and put them over each of the factors of the leading coefficient (p/q). All of those fractions will be possible solutions (roots) along with their negatives.

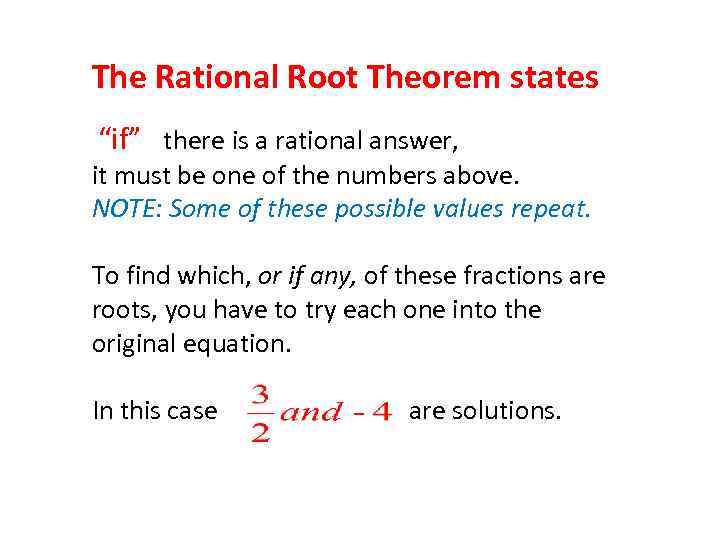The Rational Root Theorem states “if” there is a rational answer, it must be The Rational Root Theorem states “if” there is a rational answer, it must be one of the numbers above. NOTE: Some of these possible values repeat. To find which, or if any, of these fractions are roots, you have to try each one into the original equation. In this case are solutions.

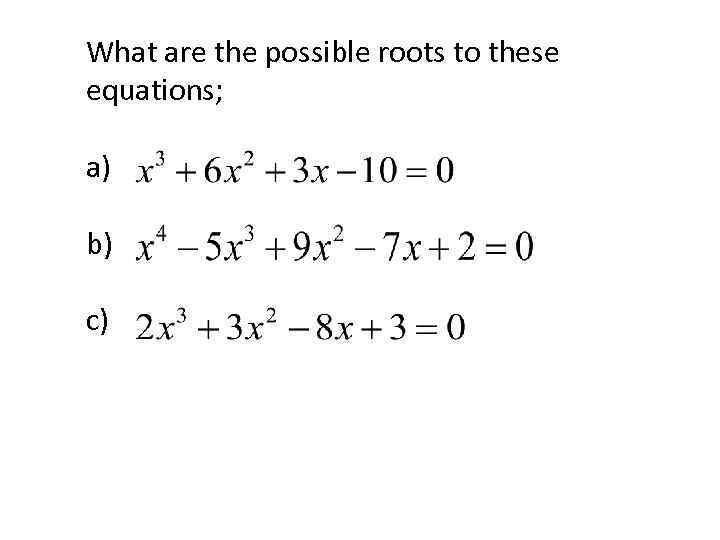What are the possible roots to these equations; a) b) c) What are the possible roots to these equations; a) b) c)

a) b) c) Now, find all roots a) b) c) Now, find all roots

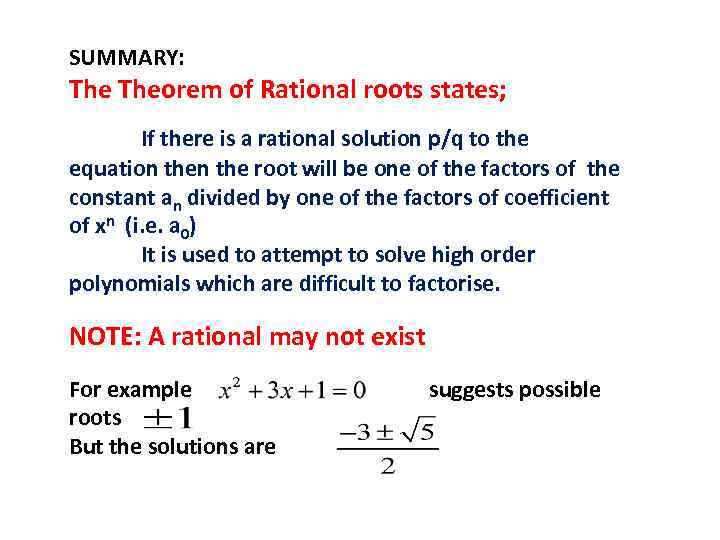SUMMARY: Theorem of Rational roots states; If there is a rational solution p/q to SUMMARY: Theorem of Rational roots states; If there is a rational solution p/q to the equation the root will be one of the factors of the constant an divided by one of the factors of coefficient of xn (i. e. a 0) It is used to attempt to solve high order polynomials which are difficult to factorise. NOTE: A rational may not exist For example roots But the solutions are suggests possible