The Time Value of Money A core concept

The Time Value of Money A core concept in financial management 1

Lesson Objectives n n To introduce the time value concept Calculate present and future values of any set of expected future cash flows. 2

Time Value ? ? ? n n n Rs. 1000 you received today or Rs. 1000 will be received tomorrow. What do you prefer? Simple reason is your time preference for money. Therefore, you may expect to get an extra cash amount as compensation for delaying. It is called the interest. This Interest for the Time Preference for Money is called the Time Value of Money 3

Why we need a premium for future cash flows? n n n Alternative uses of money. n (Investment opportunities) Individual’s preference for early consumption (time preference theory ) The risk associated with future cash flows The interest rate for the time value of money can be regarded as the opportunity cost 4

Comparison of CF at different Time intervals • A rupee received today is more valuable than a rupee received tomorrow. • Thus, cash flows in different time periods cannot be compared as they are. • There are two ways • Future value • Present value 5

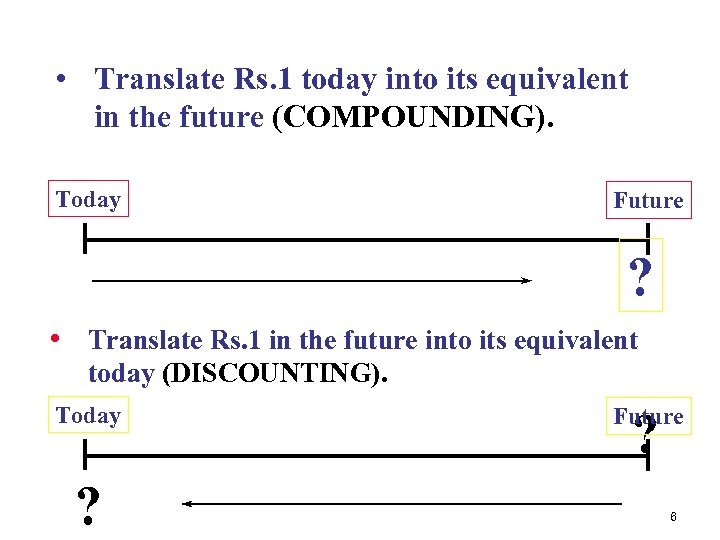• Translate Rs. 1 today into its equivalent in the future (COMPOUNDING). Today Future ? • Translate Rs. 1 in the future into its equivalent today (DISCOUNTING). Today ? Future ? 6

ASSUMPTIONS n n n A point in time is denoted by the letter “t”. Unless otherwise stated, t=0 represents today (the decision point). Unless otherwise stated, cash flows occur at the end of a time interval. Cash inflows are treated as positive amounts, while cash outflows are treated as negative amounts. Compounding frequency is the same as the cash flow frequency. 7

The Time Line Today t=0 End of the third year t=1 t=2 Beginning of the fourth year t=3 t=4 8

Cash Flows n Single cash flow n Annuity n Multiple/ uneven cash flows 9

Future Value and Compounding Process n Future value n Is the total of the principle amount and the interest accumulated on the principle for a given period. n Is the sum which an initial amount of principle (or present value (PV)) is expected to grow over a given (n) period at a given interest rate. 10

Example 1: Suppose you place Rs. 100 in a savings account that earns 6% interest compounded annually. How much can you get at the end of each period? 11

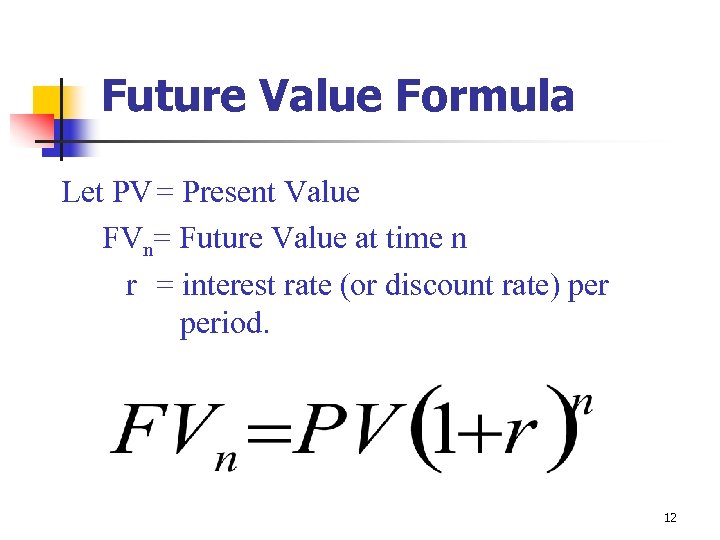Future Value Formula Let PV = Present Value FVn= Future Value at time n r = interest rate (or discount rate) period. 12

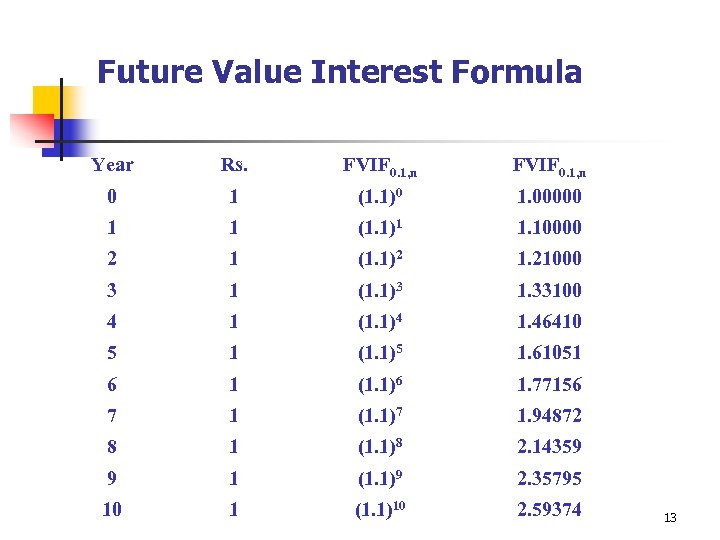Future Value Interest Formula Year Rs. FVIF 0. 1, n 0 1 (1. 1)0 1. 00000 1 1 (1. 1)1 1. 10000 2 1 (1. 1)2 1. 21000 3 1 (1. 1)3 1. 33100 4 1 (1. 1)4 1. 46410 5 1 (1. 1)5 1. 61051 6 1 (1. 1)6 1. 77156 7 1 (1. 1)7 1. 94872 8 1 (1. 1)8 2. 14359 9 1 (1. 1)9 2. 35795 10 1 (1. 1)10 2. 59374 13

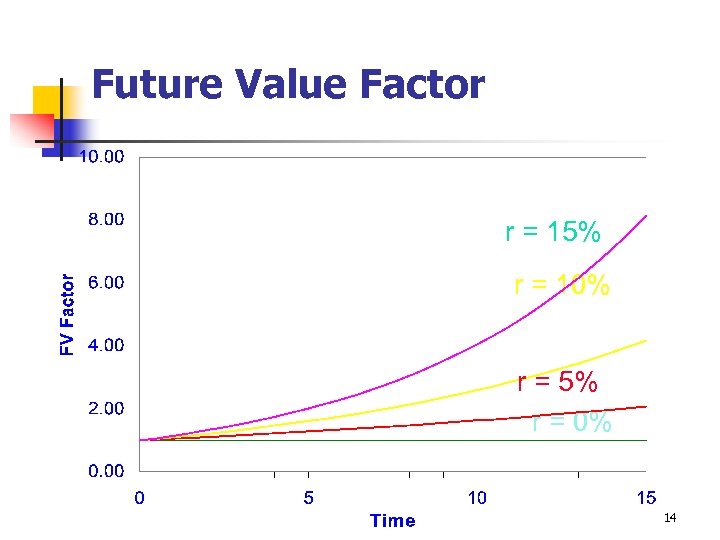Future Value Factor r = 15% r = 10% r = 5% r = 0% 14

More Frequent Compounding n n n Interest may be compounded more than once a year. The Nominal Rate (Annual Percentage Rate (APR)) is the periodic rate times the number of periods per year. The Effective rate (Annual Percentage Yield (APY)) is the “true” annually compounded interest rate. 15

Effect of Compounding Frequency on Future Value Find the future value at the end of one year if the present value is Rs. 20, 000 and the interest rate is 16%. Use the following compounding frequencies: • • • Annual Compounding Semiannual Compounding Quarterly Compounding Monthly Compounding Daily Compounding Continuous Compounding 16

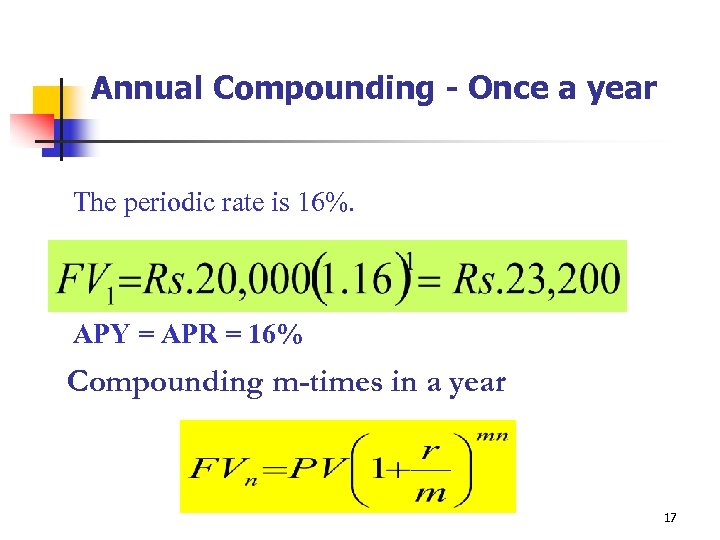Annual Compounding - Once a year The periodic rate is 16%. APY = APR = 16% Compounding m-times in a year 17

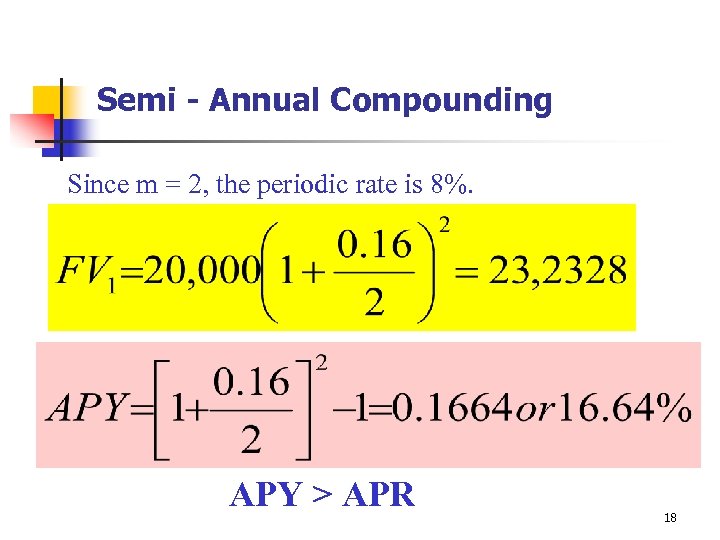Semi - Annual Compounding Since m = 2, the periodic rate is 8%. APY > APR 18

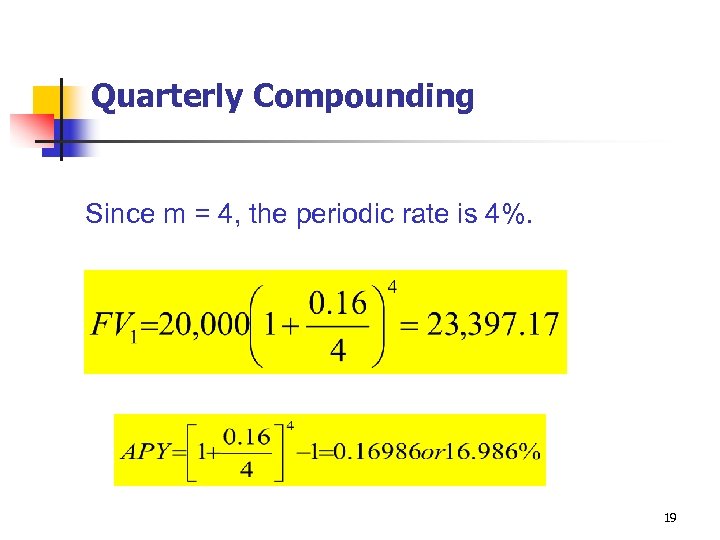Quarterly Compounding Since m = 4, the periodic rate is 4%. 19

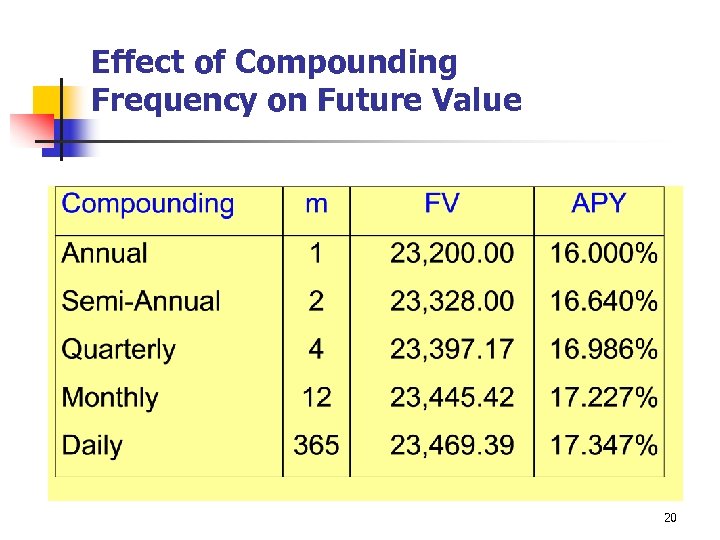Effect of Compounding Frequency on Future Value 20

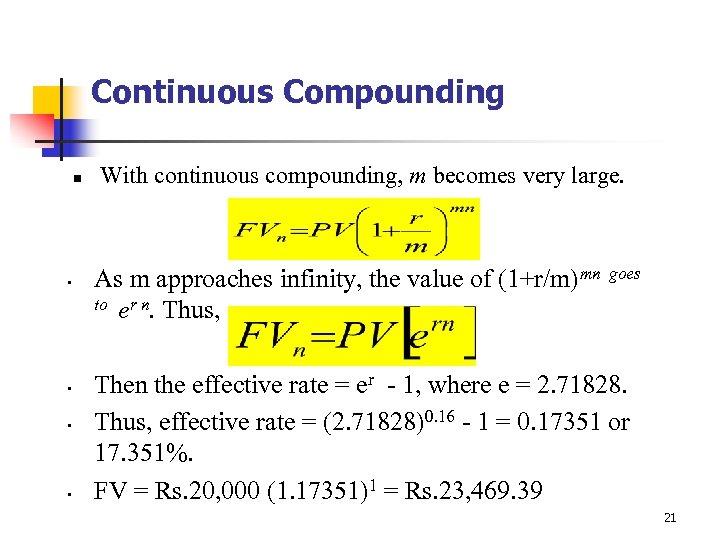Continuous Compounding n • • With continuous compounding, m becomes very large. As m approaches infinity, the value of (1+r/m)mn to er n. Thus, goes Then the effective rate = er - 1, where e = 2. 71828. Thus, effective rate = (2. 71828)0. 16 - 1 = 0. 17351 or 17. 351%. FV = Rs. 20, 000 (1. 17351)1 = Rs. 23, 469. 39 21

Present Value Future value Thus the Present Value When we get the present value, the interest rate is referred as the Discount Rate and this process is called as Discounting 22

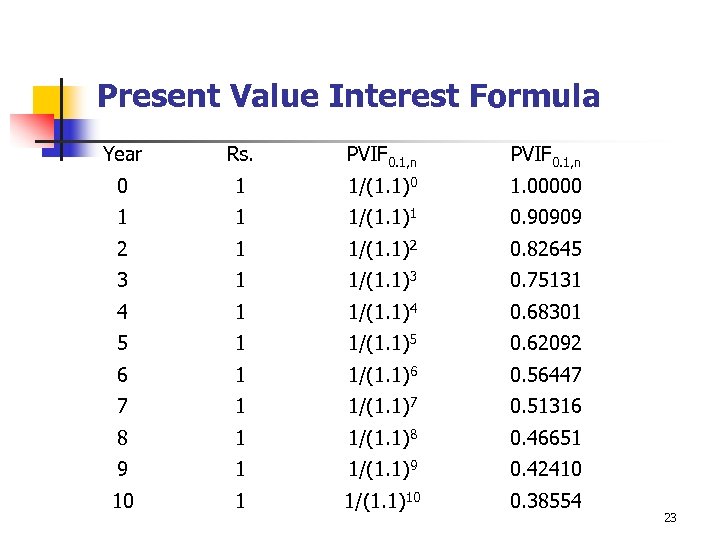Present Value Interest Formula Year Rs. PVIF 0. 1, n 0 1 1/(1. 1)0 1. 00000 1 1 1/(1. 1)1 0. 90909 2 1 1/(1. 1)2 0. 82645 3 1 1/(1. 1)3 0. 75131 4 1 1/(1. 1)4 0. 68301 5 1 1/(1. 1)5 0. 62092 6 1 1/(1. 1)6 0. 56447 7 1 1/(1. 1)7 0. 51316 8 1 1/(1. 1)8 0. 46651 9 1 1/(1. 1)9 0. 42410 10 1 1/(1. 1)10 0. 38554 23

Present Value - single sums 1. If you will receive Rs. 100 one year from now, what is the PV of that Rs. 100 if your opportunity cost is 6% 2. If you receive Rs. 100 5 years from now, what is the PV of that Rs. 100, if your opportunity cost is 6%? 3. What is the PV of Rs. 1, 000 to be received 15 years from now if your opportunity cost is 7%? 24

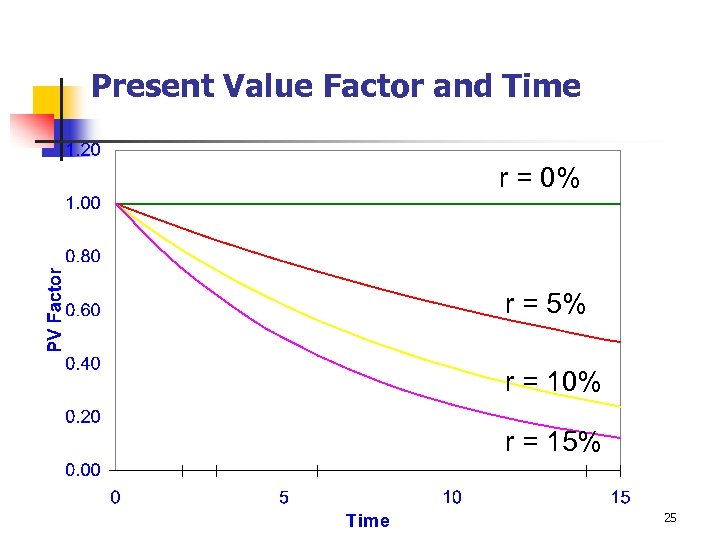Present Value Factor and Time r = 0% r = 5% r = 10% r = 15% 25

Present Value - single sums Ex. 1. If you sold land for Rs. 11, 933 that you bought 5 years ago for Rs. 5, 000, what is your annual rate of return? 2. Suppose you placed Rs. 100 in an account that pays 9. 6% interest, compounded monthly. How long will it take for your account to grow to Rs. 500? 26

Multiple Cash Flows n n PV of multiple cash flows = the sum of the present values of the individual cash flows. FV of multiple cash flows at a common point in time = the sum of the future values of the individual cash flows at that point in time. 27

Uneven Cash Flows FV -10, 000 2, 000 0 1 n 4, 000 2 6, 000 7, 000 3 4 How do we find the FV/PV of a cash flow stream when cash flows are different? (Use a 10% interest rate). 28

Annuities n n n An annuity is a series of identical cash flows that are expected to occur each period for a specified number of periods. Thus, CF 1 = CF 2 = CF 3 = Cf 4 =. . . = CFn Examples of annuities: n Installment loans (car loans, mortgages). n Coupon payment on corporate bonds. n Rent payment on your apartment. 29

Types of Annuities n n n Ordinary Annuity: n An annuity with End-of-Period cash flows, beginning one period from today. Annuity Due: n An annuity with Beginning-of-Period cash flows. Deferred Annuity: n An annuity that begins more than one period from today. 30

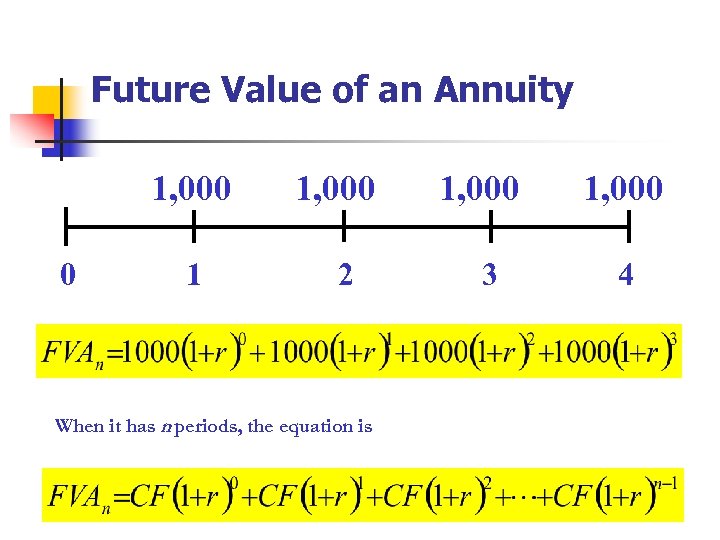Future Value of an Annuity 1, 000 0 1 1, 000 2 1, 000 3 1, 000 4 When it has n periods, the equation is 31

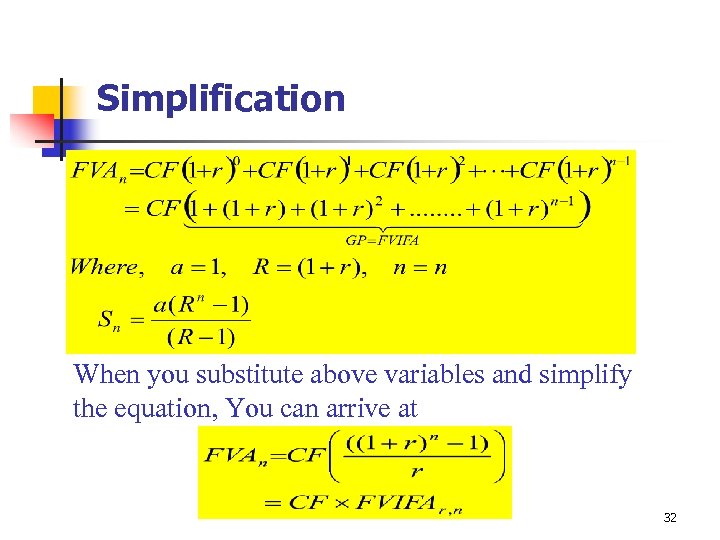Simplification When you substitute above variables and simplify the equation, You can arrive at 32

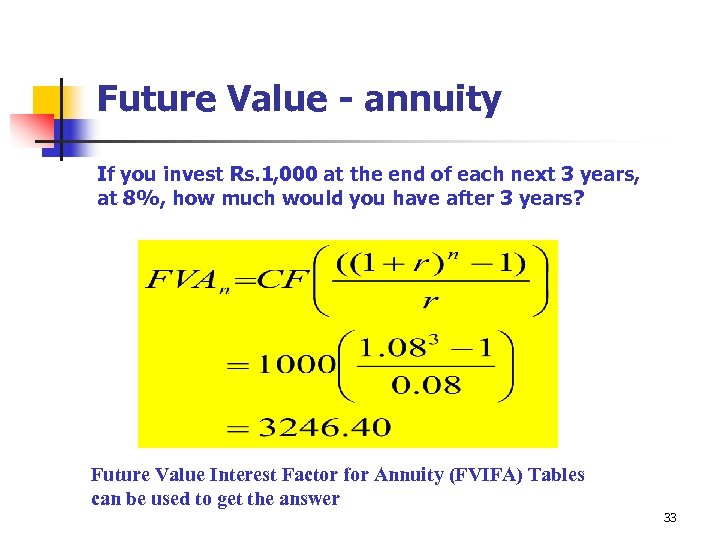Future Value - annuity If you invest Rs. 1, 000 at the end of each next 3 years, at 8%, how much would you have after 3 years? Future Value Interest Factor for Annuity (FVIFA) Tables can be used to get the answer 33

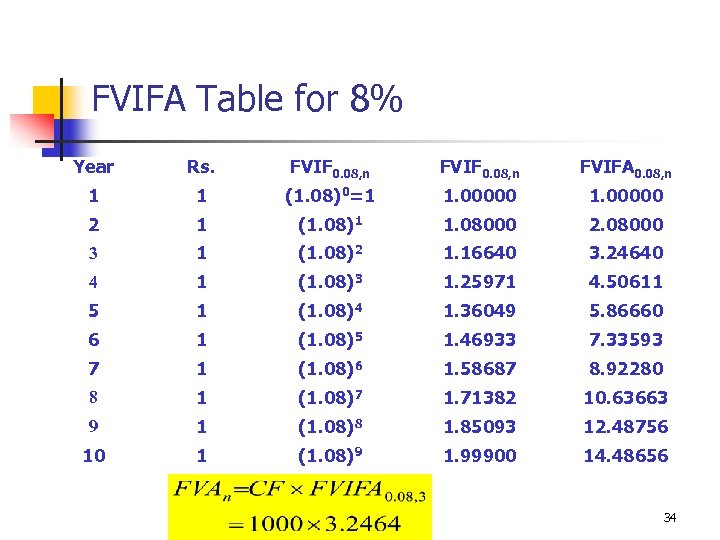FVIFA Table for 8% Year Rs. FVIF 0. 08, n FVIFA 0. 08, n 1 1 (1. 08)0=1 1. 00000 2 1 (1. 08)1 1. 08000 2. 08000 3 1 (1. 08)2 1. 16640 3. 24640 4 1 (1. 08)3 1. 25971 4. 50611 5 1 (1. 08)4 1. 36049 5. 86660 6 1 (1. 08)5 1. 46933 7. 33593 7 1 (1. 08)6 1. 58687 8. 92280 8 1 (1. 08)7 1. 71382 10. 63663 9 1 (1. 08)8 1. 85093 12. 48756 10 1 (1. 08)9 1. 99900 14. 48656 34

Present Value Of an Annuity 1, 000 0 1 1, 000 2 1, 000 3 1, 000 4 When it has n periods, the equation is 35

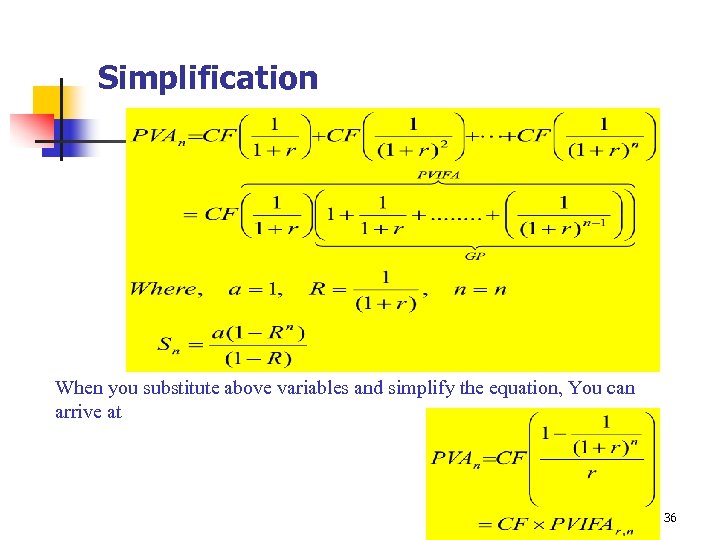Simplification When you substitute above variables and simplify the equation, You can arrive at 36

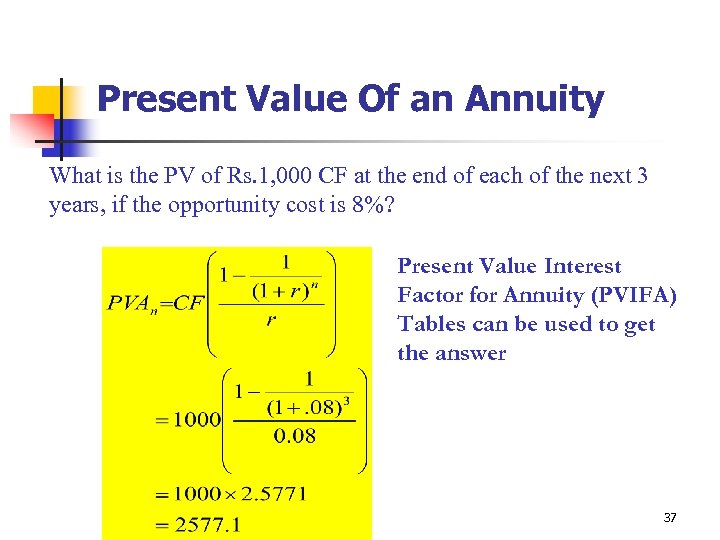Present Value Of an Annuity What is the PV of Rs. 1, 000 CF at the end of each of the next 3 years, if the opportunity cost is 8%? Present Value Interest Factor for Annuity (PVIFA) Tables can be used to get the answer 37

PVIFA Table for 8% Year Rs. PVIF 0. 08, n PVIFA 0. 08, n 1 1 1/(1. 08)1 0. 92593 2 1 1/(1. 08)2 0. 85734 1. 78326 3 1 1/(1. 08)3 0. 79383 2. 57710 4 1 1/(1. 08)4 0. 73503 3. 31213 5 1 1/(1. 08)5 0. 68058 3. 99271 6 1 1/(1. 08)6 0. 63017 4. 62288 7 1 1/(1. 08)7 0. 58349 5. 20637 8 1 1/(1. 08)8 0. 54027 5. 74664 9 1 1/(1. 08)9 0. 50025 6. 24689 10 1 1/(1. 08)10 0. 46319 6. 71008 38

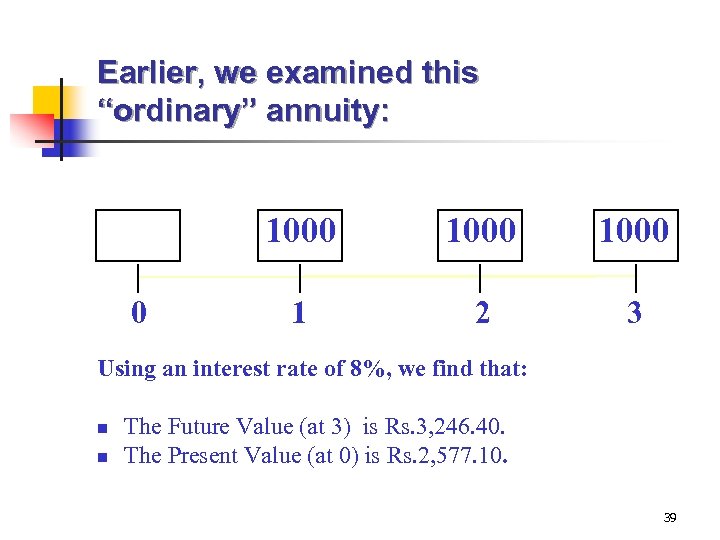Earlier, we examined this “ordinary” annuity: 1000 0 1000 1 2 3 Using an interest rate of 8%, we find that: n n The Future Value (at 3) is Rs. 3, 246. 40. The Present Value (at 0) is Rs. 2, 577. 10. 39

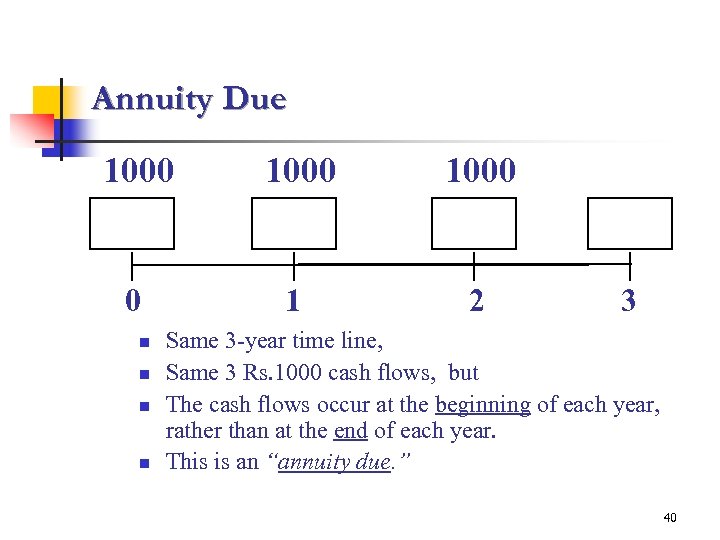Annuity Due 1000 0 1 2 n n 3 Same 3 -year time line, Same 3 Rs. 1000 cash flows, but The cash flows occur at the beginning of each year, rather than at the end of each year. This is an “annuity due. ” 40

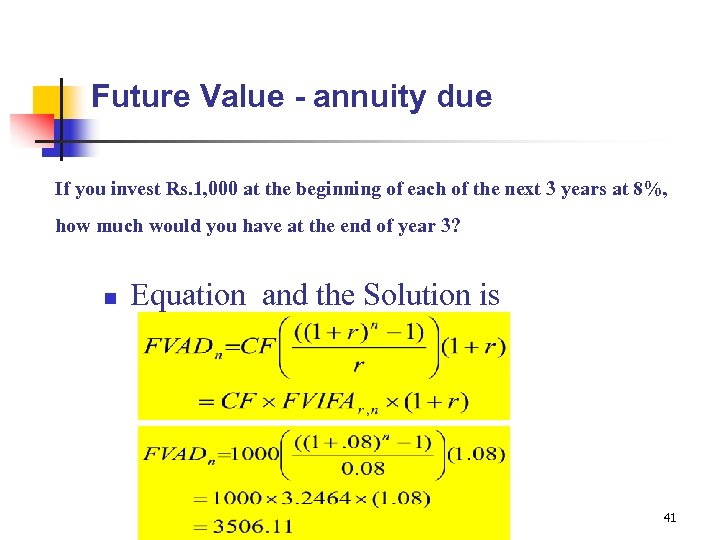Future Value - annuity due If you invest Rs. 1, 000 at the beginning of each of the next 3 years at 8%, how much would you have at the end of year 3? n Equation and the Solution is 41

Present Value - Annuity due What is the PV of Rs. 1, 000 at the beginning of each of the next 3 years, if your opportunity cost is 8%? 1000 0 n 1000 1 2 Equation and the solution is 3 42

Deferred Annuity n n The first cash flow in a deferred annuity is expected to occur later than t=1. The PV of the deferred annuity can be computed as the difference in the PVs of two annuities. 43

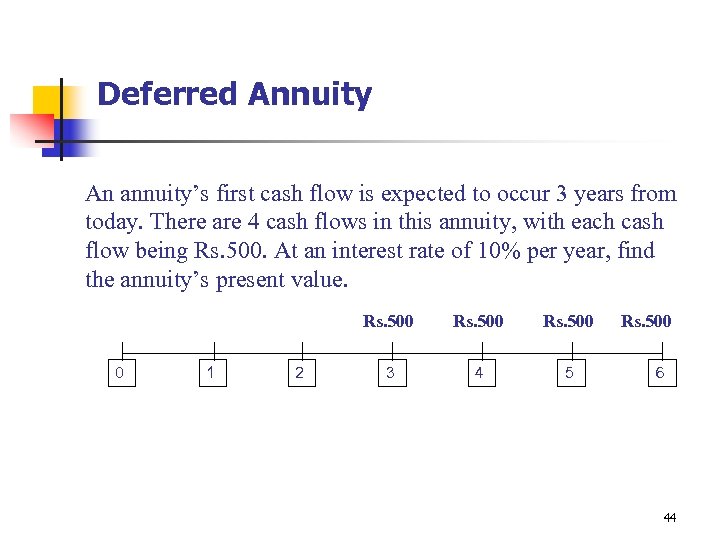Deferred Annuity An annuity’s first cash flow is expected to occur 3 years from today. There are 4 cash flows in this annuity, with each cash flow being Rs. 500. At an interest rate of 10% per year, find the annuity’s present value. Rs. 500 0 1 2 Rs. 500 3 4 5 Rs. 500 6 44

Example n n n Cash flows from an investment are expected to be Rs. 40, 000 per year at the end of years 4, 5, 6, 7, and 8. If you require a 20% rate of return, what is the PV of these cash flows? After graduation, you plan to invest Rs. 400 per month in the stock market. If you earn 12% per year on your stocks, how much will you have accumulated when you retire in 30 years? If you borrow Rs. 100, 000 at 7% fixed interest for 30 years in order to buy a house, what will be your monthly house payment? 45

Team Assignment Ø Ø Upon retirement, your goal is to spend 5 years travelling around the world. To travel in style, it will require Rs. 250, 000 per year at the beginning of each year. If you plan to retire in 30 years, what are the equal monthly (end of month) payments necessary to achieve this goal? Ø Ø The funds in your retirement account will compound at 10% per annum on monthly basis. 46

Present Value of Your Bank Loan Cynthia Smart agrees to repay her loan in 24 monthly installments of Rs. 250 each. If the interest rate on the loan is 0. 75% per month, what is the present value of her loan payments? n You wish to retire 25 years from today with Rs. 2, 000 in the bank. If the bank pays 10% interest per year, how much should you save each year to reach your goal? n Rob Steinberg borrows Rs. 10, 000 to be repaid in four equal annual installments, beginning one year from today. What is Rob’s annual payment on this loan if the bank charges him 14% interest per year? n 47

Loan Amortization Schedule n n n It shows how a loan is paid off over time. It breaks down each payment into the interest component and the principal component. We will illustrate this using Rob Steinberg’s 4 -year Rs. 10, 000 loan which calls for annual payments of Rs. 3, 432. 05. Recall that the interest rate on this loan is 14% per year. 48

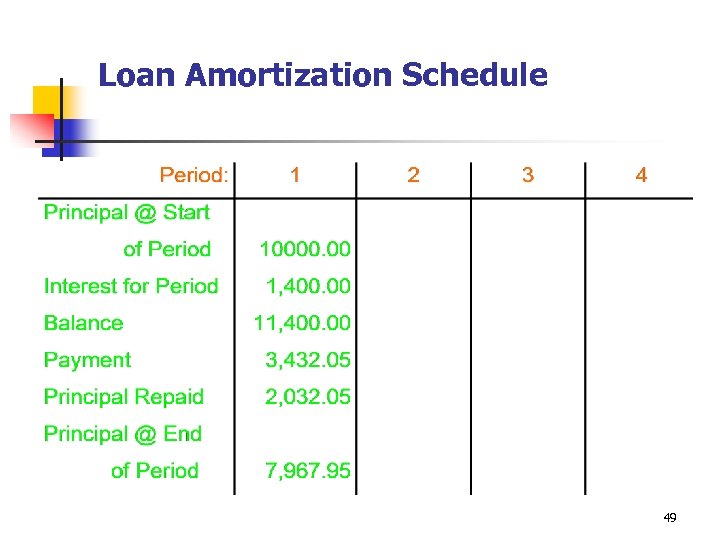Loan Amortization Schedule 49

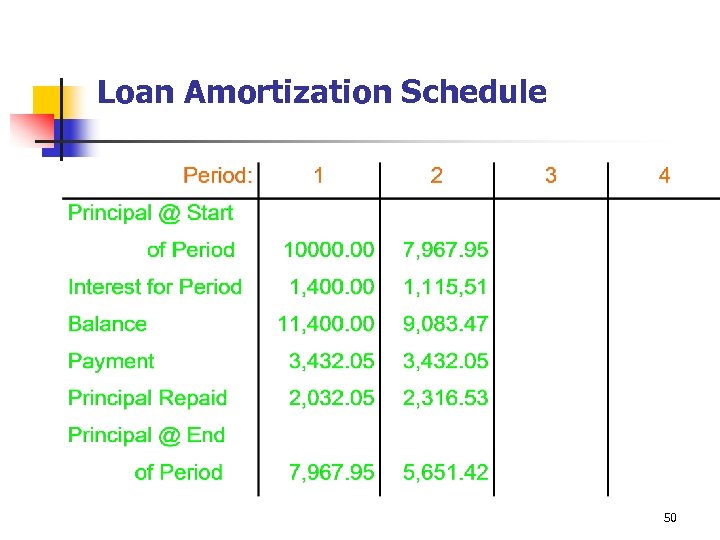Loan Amortization Schedule 50

Loan Amortization Schedule 51

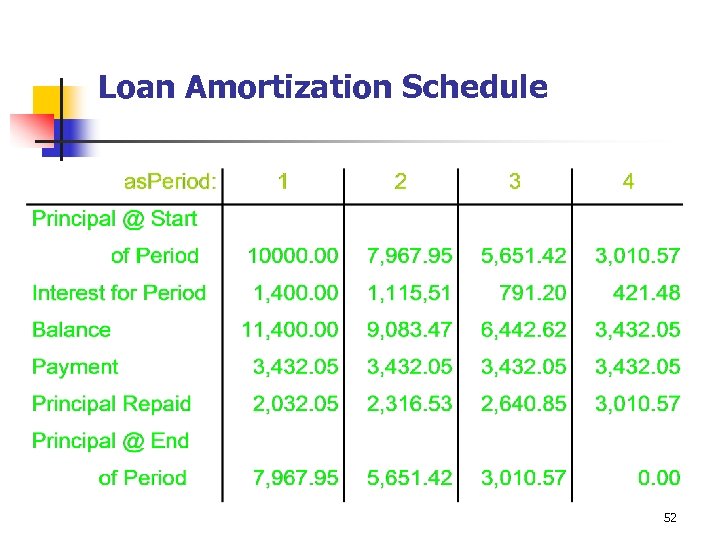Loan Amortization Schedule 52

APR and APY for an Installment Loan Suppose you borrow Rs. 5, 000 from the bank and promise to repay the loan in 12 equal monthly installments of Rs. 437. 25 each, with the first payment to be made one month from today. What is the APR? n What is the APY? n 53

Perpetuity n n n A perpetuity is an annuity with an infinite number of cash flows. The present value of cash flows occurring in the distant future is very close to zero. n At 10% interest, the PV of Rs. 100 cash flow occurring 50 years from today is Rs. 0. 85! n The PV of Rs. 100 cash flow occurring 100 years from today is less than one penny Suppose you will receive a fixed payment every period (month, year, etc. ) forever. This is an example of a perpetuity. 54

Mathematically, (PVIFA r, n ) = 1 - 1 n (1 + r) r We said that a perpetuity is an annuity where n = infinity. What happens to this formula when n gets very, very large? 55

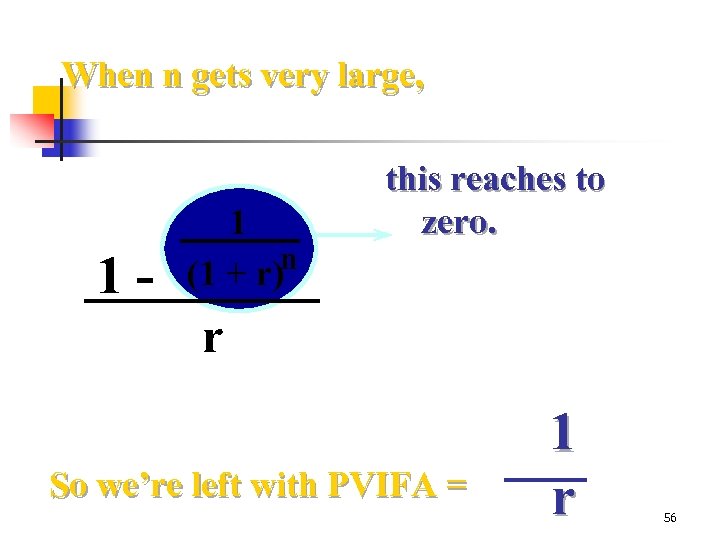When n gets very large, 1 - 1 n (1 + r) this reaches to zero. r So we’re left with PVIFA = 1 r 56

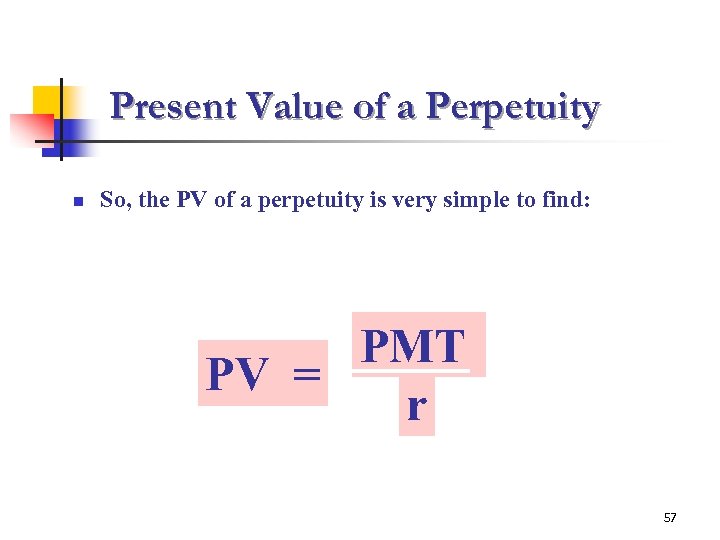Present Value of a Perpetuity n So, the PV of a perpetuity is very simple to find: PMT PV = r 57

Ex. n n n What should you be willing to pay in order to receive Rs. 10, 000 annually forever, if you require 8% per year on the investment? Find the present value of a perpetuity of Rs. 270 per year if the interest rate is 12% per year. The First Commerce Bank offers a Certificate of Deposit (CD) that pays you Rs. 5, 000 in four years. The CD can be purchased today for Rs. 3, 477. 87. Assuming you hold the CD to maturity, what annual interest rate is the bank paying on this CD? 58

Solving for an Unknown Interest Rate on a Loan Erin Clapton borrows Rs. 10, 000 from her bank, and agrees to repay the loan in six equal annual installments of Rs. 2, 100 each. The first payment will be made one year from today. What interest rate is the bank charging her? 59

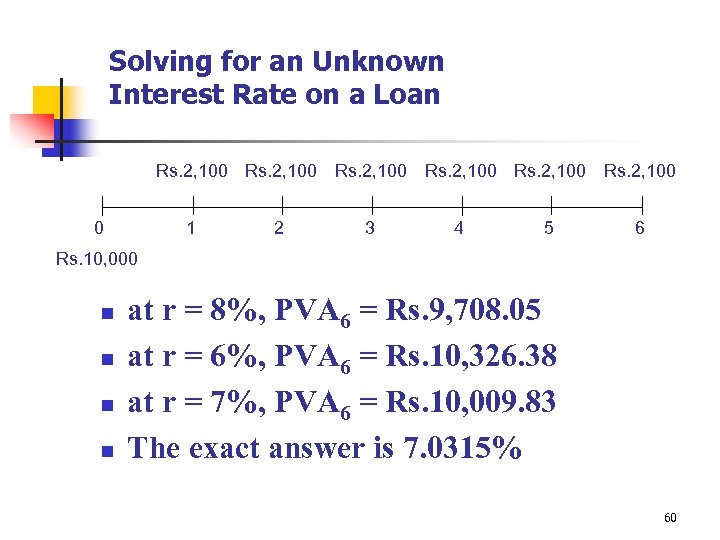Solving for an Unknown Interest Rate on a Loan Rs. 2, 100 0 1 2 3 Rs. 2, 100 4 5 6 Rs. 10, 000 n n at r = 8%, PVA 6 = Rs. 9, 708. 05 at r = 6%, PVA 6 = Rs. 10, 326. 38 at r = 7%, PVA 6 = Rs. 10, 009. 83 The exact answer is 7. 0315% 60