The Gaseous State of Matter Preparation for College

The Gaseous State of Matter Preparation for College Chemistry Columbia University Department of Chemistry

Chapter Outline KMT Gas Laws Ideal Gas Equation Gas Stoichiometry Air Pollution

Preliminary Observations Molar mass of water: 18 g /mole 6. 02 x 1023 molecules weigh 18 g Density of water: 1 g/cc 18 g liquid water occupies 18 m. L 18 g gaseous water occupies 22, 400 m. L

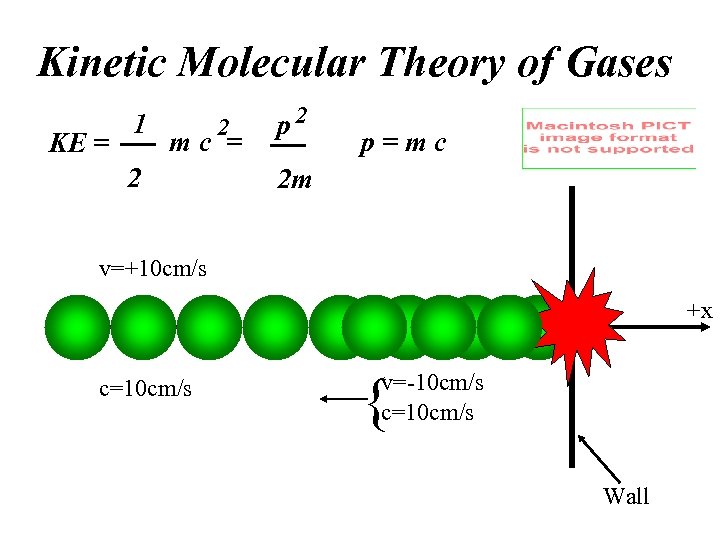Kinetic Molecular Theory of Gases KE = 1 2 mc = 2 p=mc 2 m v=+10 cm/s -x c=10 cm/s +x { v=-10 cm/s c=10 cm/s Wall

Kinetic Molecular Theory of Gases

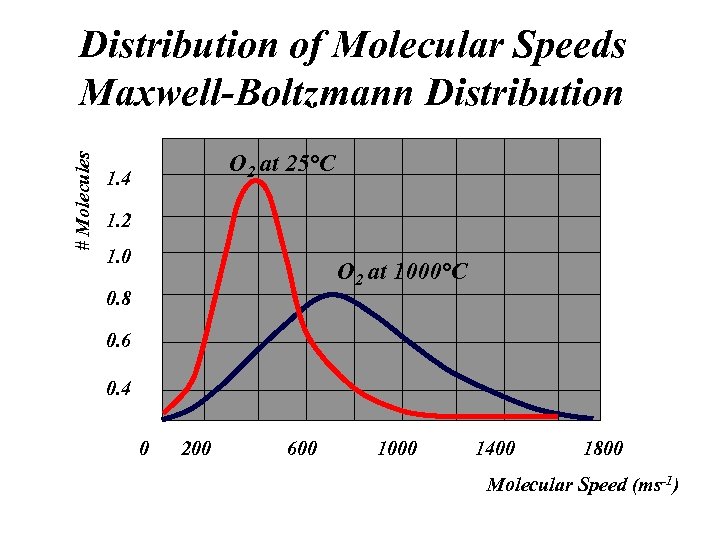# Molecules Distribution of Molecular Speeds Maxwell-Boltzmann Distribution O 2 at 25°C 1. 4 1. 2 1. 0 O 2 at 1000°C 0. 8 0. 6 0. 4 0 200 600 1000 1400 1800 Molecular Speed (ms-1)

Graham’s Law of Effusion At the same T and P, the rates of Effusion of two gases are inversely proportional to their densities or molar masses.

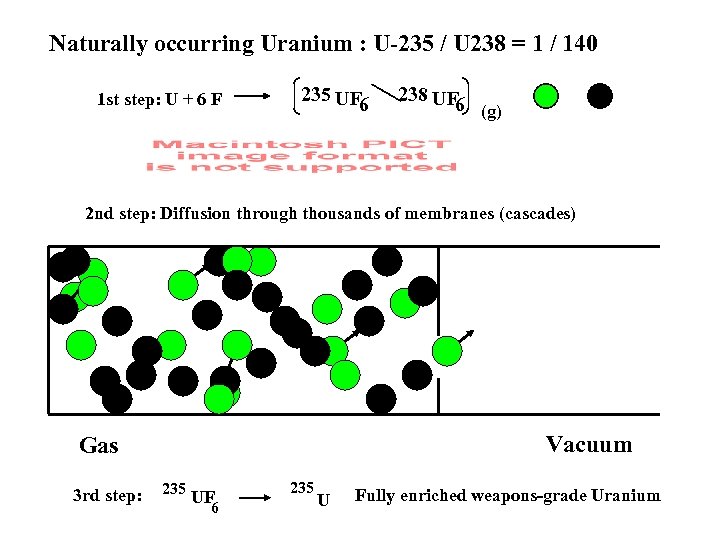Naturally occurring Uranium : U-235 / U 238 = 1 / 140 1 st step: U + 6 F 235 UF 6 238 UF 6 (g) 2 nd step: Diffusion through thousands of membranes (cascades) Vacuum Gas 3 rd step: 235 UF 6 235 U Fully enriched weapons-grade Uranium

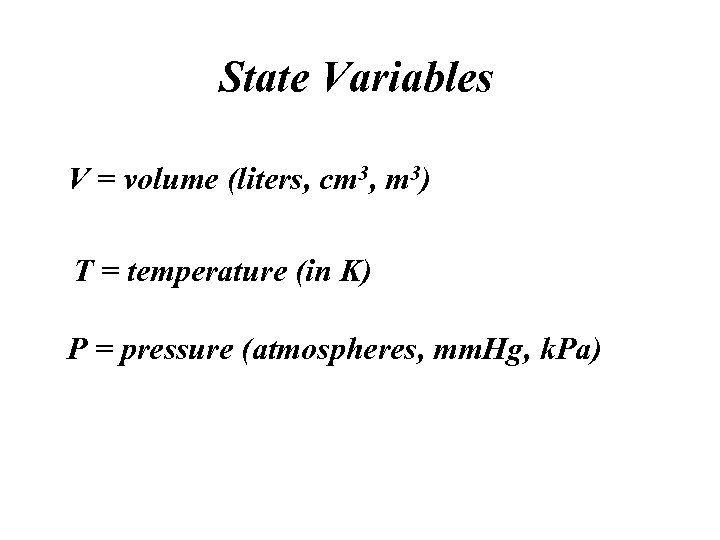State Variables V = volume (liters, cm 3, m 3) T = temperature (in K) P = pressure (atmospheres, mm. Hg, k. Pa)

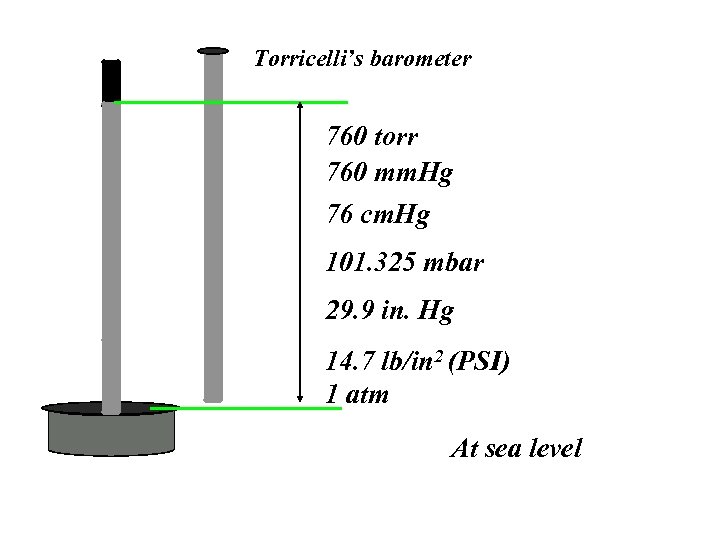Torricelli’s barometer 760 torr 760 mm. Hg 76 cm. Hg 101. 325 mbar 29. 9 in. Hg 14. 7 lb/in 2 (PSI) 1 atm At sea level

Hg height 150 km air Atmospheric Pressure

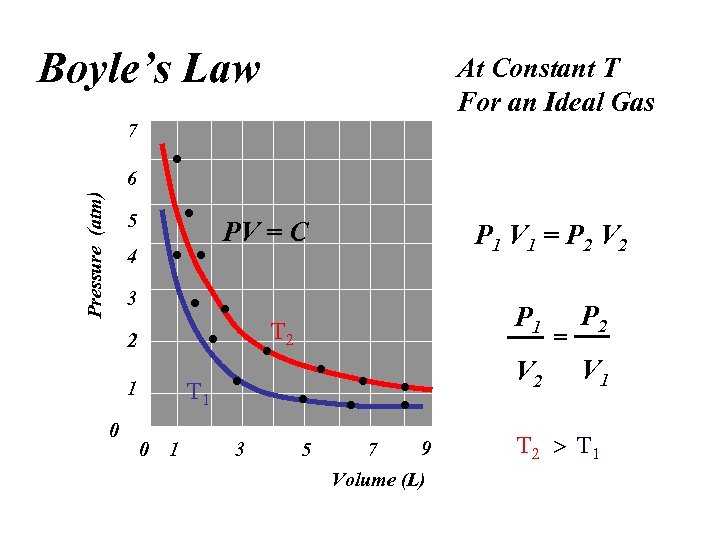Boyle’s Law At Constant T For an Ideal Gas 7 Pressure (atm) 6 5 PV = C 4 P 1 V 1 = P 2 V 2 3 2 V 2 1 0 P 1 0 1 3 5 9 7 Volume (L) = P 2 V 1

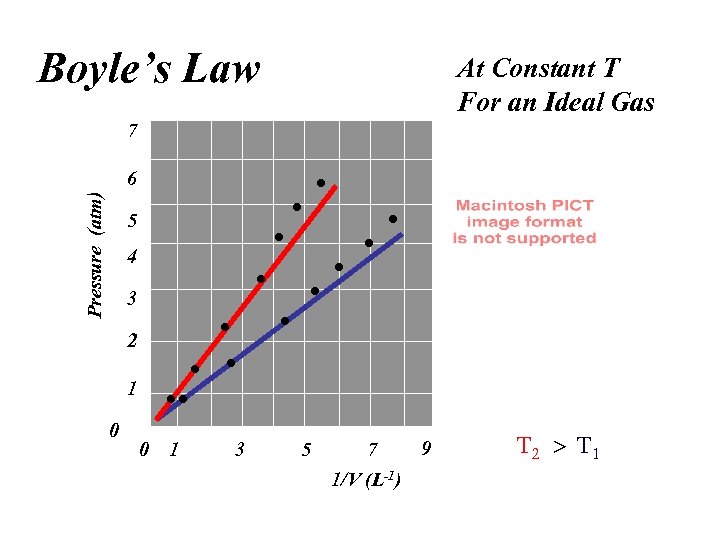Boyle’s Law At Constant T For an Ideal Gas 7 Pressure (atm) 6 5 4 3 2 1 0 0 1 3 5 9 7 1/V (L-1)

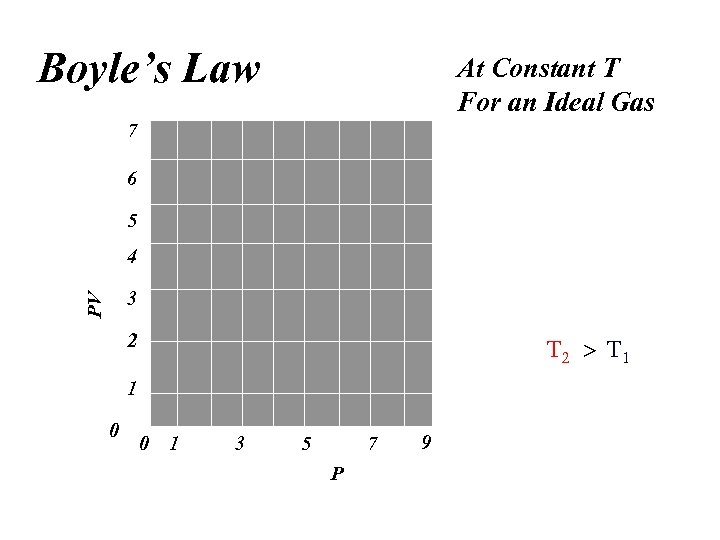Boyle’s Law At Constant T For an Ideal Gas 7 6 5 4 PV 3 2 1 0 0 1 3 5 7 P 9

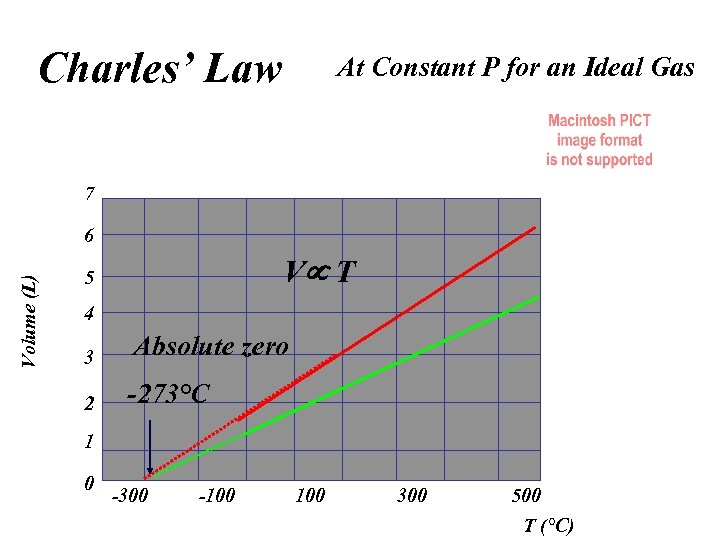Charles’ Law At Constant P for an Ideal Gas 7 Volume (L) 6 V T 5 4 3 2 Absolute zero -273°C 1 0 -300 -100 300 500 T (°C)

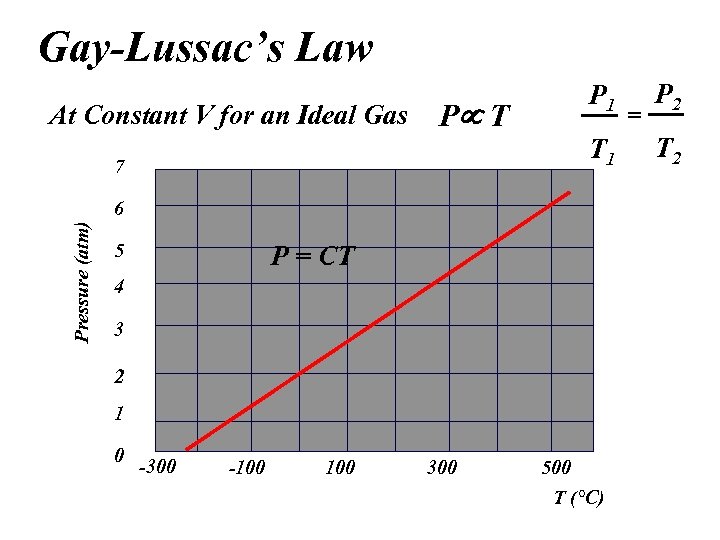Gay-Lussac’s Law At Constant V for an Ideal Gas P 1 P T T 1 7 Pressure (atm) 6 5 P = CT 4 3 2 1 0 -300 -100 300 500 T (°C) = P 2 T 2

Combined Gas Laws Charles’ V 1 T 1 = Boyle’s V 2 P 1 V 1 = P 2 V 2 T 2 P 1 V 1 T 1 V 2 = P 2 V 2 T 2 = V 1 P 1 T 2 P 2 T 1

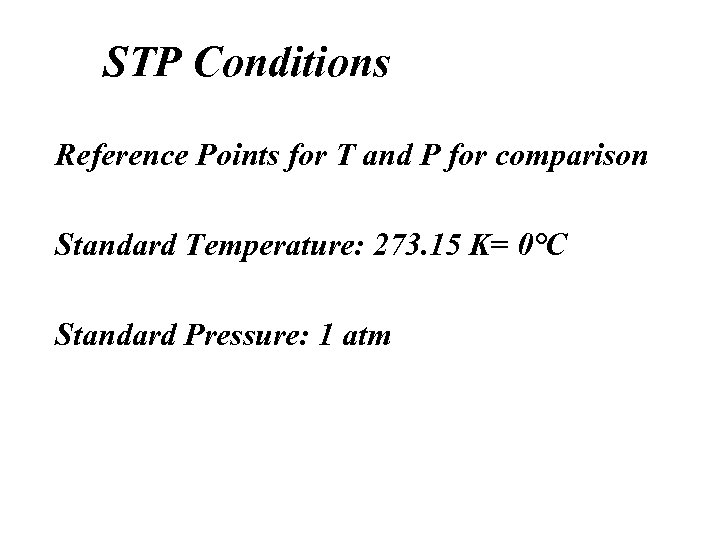STP Conditions Reference Points for T and P for comparison Standard Temperature: 273. 15 K= 0°C Standard Pressure: 1 atm

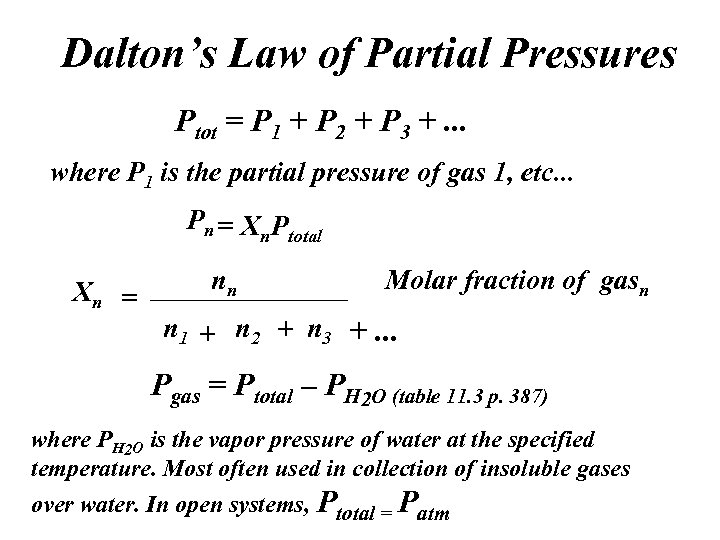Dalton’s Law of Partial Pressures Ptot = P 1 + P 2 + P 3 +. . . where P 1 is the partial pressure of gas 1, etc. . . Pn = Xn Ptotal Xn = nn Molar fraction of gasn n 1 + n 2 + n 3 +. . . Pgas = Ptotal – PH 2 O (table 11. 3 p. 387) where PH 2 O is the vapor pressure of water at the specified temperature. Most often used in collection of insoluble gases over water. In open systems, Ptotal = Patm

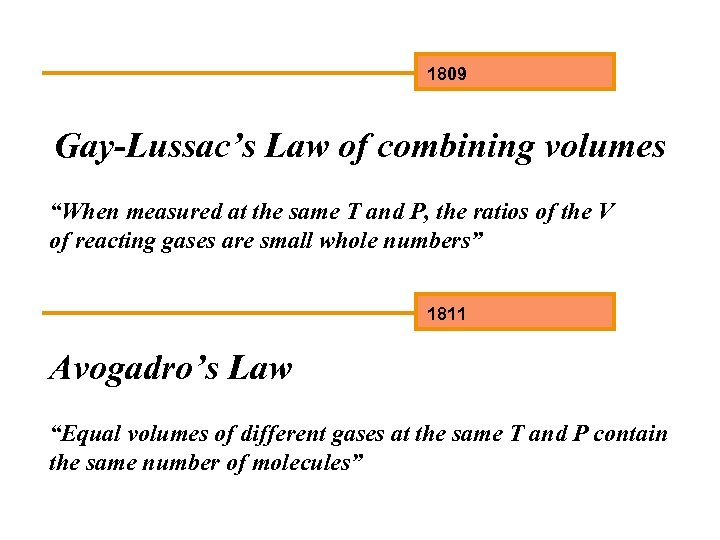1809 Gay-Lussac’s Law of combining volumes “When measured at the same T and P, the ratios of the V of reacting gases are small whole numbers” 1811 Avogadro’s Law “Equal volumes of different gases at the same T and P contain the same number of molecules”

Consequences of Avogadro’s Law 1. Explanation of Gay-Lussac’s combining volumes law. Diatomic nature of elemental gases. 2. Method for determining molar masses of gases. The molar Volume. 3. Firm foundation of KMT: gases consists of microscopic particles

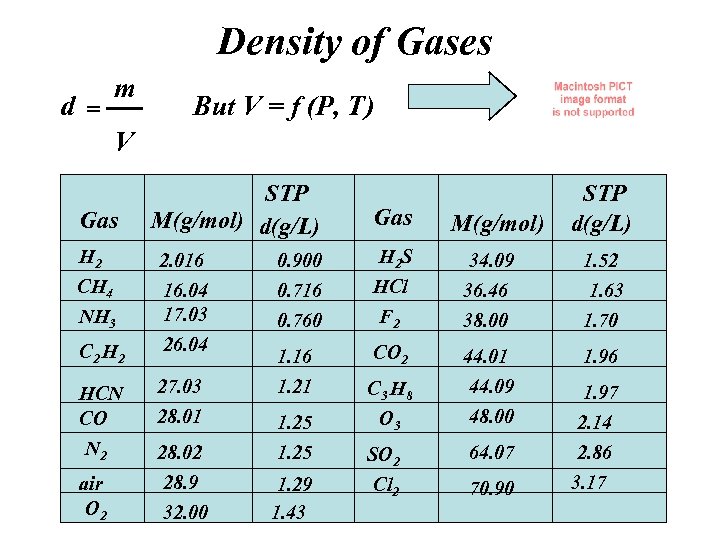Density of Gases d= m But V = f (P, T) V Gas H 2 CH 4 NH 3 C 2 H 2 STP M(g/mol) d(g/L) 2. 016 16. 04 17. 03 26. 04 0. 900 0. 716 0. 760 1. 16 1. 21 HCN CO 27. 03 28. 01 N 2 28. 02 1. 25 air O 2 28. 9 32. 00 1. 29 1. 43 Gas H 2 S HCl F 2 CO 2 C 3 H 8 O 3 SO 2 Cl 2 M(g/mol) STP d(g/L) 34. 09 36. 46 38. 00 1. 52 1. 63 1. 70 44. 01 44. 09 48. 00 1. 96 1. 97 64. 07 2. 14 2. 86 70. 90 3. 17

Ideal Gas Equation of State 1 V P V R = PV = n. RT P V n. T T PV = m RT M m. RT M = PV d= For one mole of a gas at STP, R constant: R = (1 atm)(22. 4 L) 273 K = 0. 082 L-atm mol-K PM RT

Ideal Gas Equation The ideal gas constant has energy/mol degrees dimensions [R] = [pressure][Volume] [temperature][mol] [R] = [force][length] [temperature][mol] = [force][volume] [area][temperature][mol] = [energy] [temperature][mol] R = 8. 134 J mol-1 K-1 ~ 2 Cal mol -1 K-1

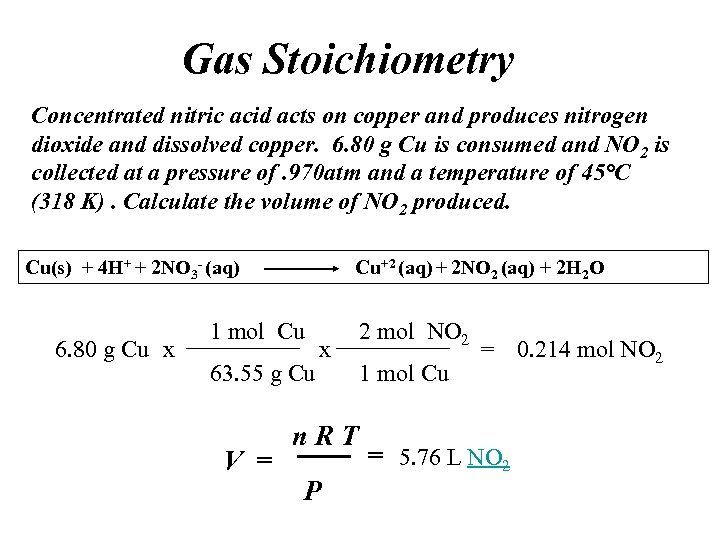Gas Stoichiometry Concentrated nitric acid acts on copper and produces nitrogen dioxide and dissolved copper. 6. 80 g Cu is consumed and NO 2 is collected at a pressure of. 970 atm and a temperature of 45°C (318 K). Calculate the volume of NO 2 produced. Cu(s) + 4 H+ + 2 NO 3 - (aq) 6. 80 g Cu x Cu+2 (aq) + 2 NO 2 (aq) + 2 H 2 O 1 mol Cu 63. 55 g Cu V = x n. RT P 2 mol NO 2 1 mol Cu = 0. 214 mol NO 2 = 5. 76 L NO 2

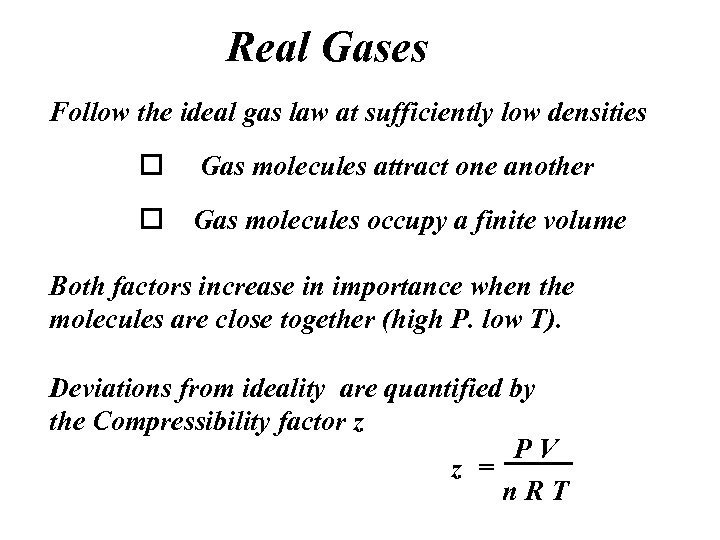Real Gases Follow the ideal gas law at sufficiently low densities o Gas molecules attract one another o Gas molecules occupy a finite volume Both factors increase in importance when the molecules are close together (high P. low T). Deviations from ideality are quantified by the Compressibility factor z PV z = n. RT

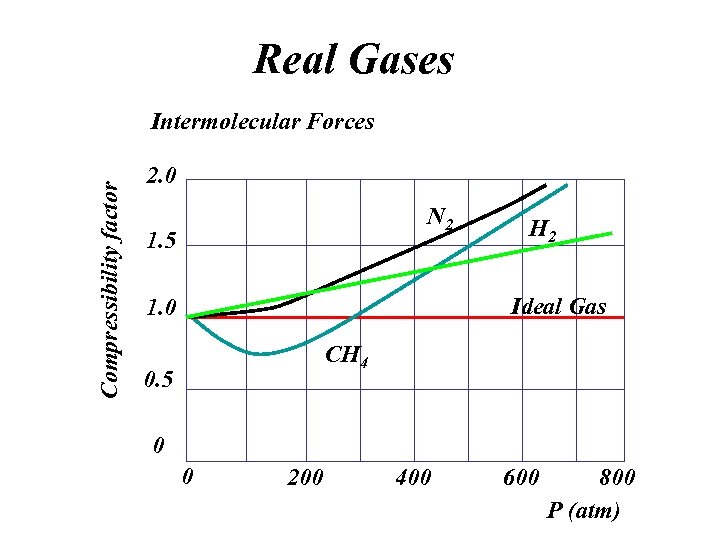Real Gases Compressibility factor Intermolecular Forces 2. 0 N 2 1. 5 H 2 Ideal Gas 1. 0 CH 4 0. 5 0 0 200 400 600 800 P (atm)

Compressibility factor Nitrogen at several T 2. 0 25 °C 1. 5 600 °C Ideal Gas 1. 0 -100 °C 0. 5 0 0 200 400 600 800 P (atm)

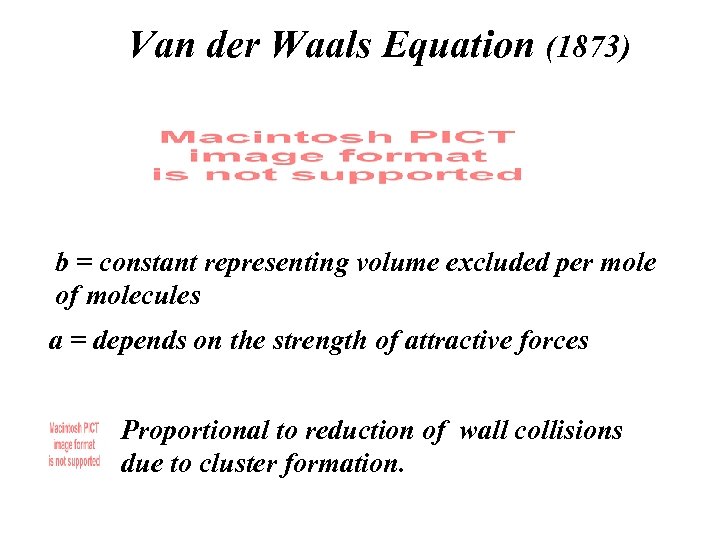Van der Waals Equation (1873) b = constant representing volume excluded per mole of molecules a = depends on the strength of attractive forces Proportional to reduction of wall collisions due to cluster formation.

Air Pollution Upper and Lower Atmosphere Ozone Sulfur Dioxide Nitrogen Oxides Green House Effect

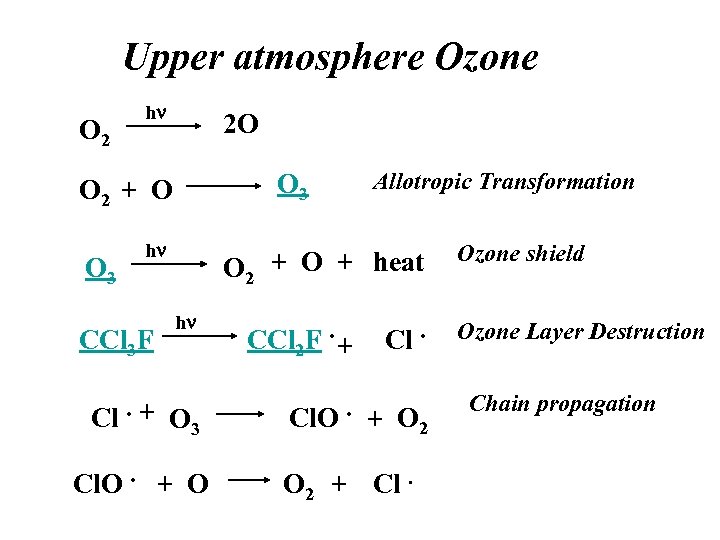Upper atmosphere Ozone O 2 hn 2 O O 3 O 2 + O O 3 hn CCl 3 F Cl. + Allotropic Transformation O 2 + O + heat hn CCl 2 F. + O 3 Cl. O. + O Cl. + O 2 + Cl. Ozone shield Ozone Layer Destruction Chain propagation

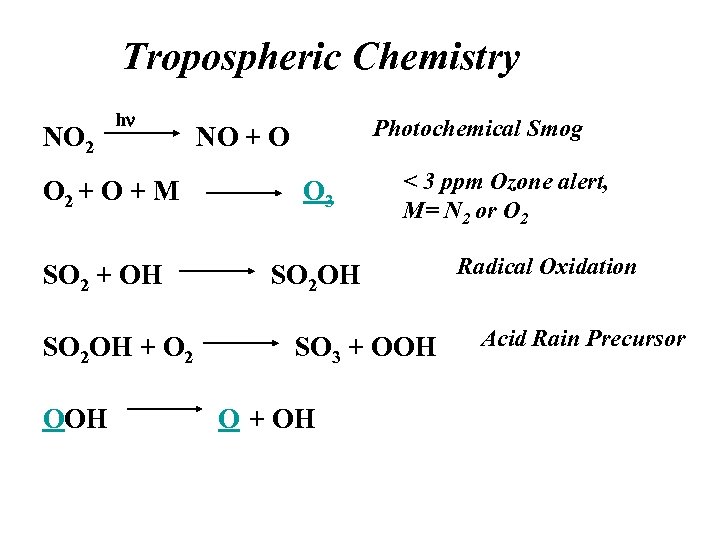Tropospheric Chemistry NO 2 hn Photochemical Smog NO + O O 2 + O + M O 3 SO 2 + OH < 3 ppm Ozone alert, M= N 2 or O 2 SO 2 OH + O 2 OOH SO 3 + OOH O + OH Radical Oxidation Acid Rain Precursor

http: //www. epa. gov/globalwarming/emissions/index. html

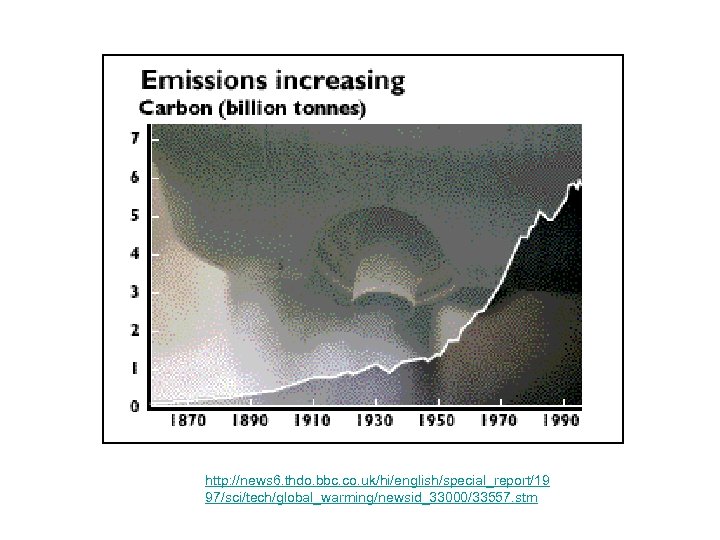http: //news 6. thdo. bbc. co. uk/hi/english/special_report/19 97/sci/tech/global_warming/newsid_33000/33557. stm