The Concept of Infinity In the History of

The Concept of Infinity In the History of Mathematics and its Implications

The Pythagoreans • Seemed to have some concept of infinity as they considered one the generator of all numbers. Any natural number no matter how big could be made bigger by adding one. • Later showed an intolerance for the concept that there were numbers outside the natural numbers when it was discovered that the hypotenuse of an isosceles right triangle is not commensurate with its side. (Hippasus thrown from boat) • *Curriculum connection – number systems - Gr. 11 U or M

Zeno`s Paradoxes • One such paradox involving the concept of infinity is that of crossing a room. Suppose you are trying to get from one end of a room to the other by going half the distance to the other side each time. As in the paradox by Zeno, the distance remaining will keep shrinking but you will never reach the other side in any number of finite steps. • Curriculum connection – asymptotes/limits – functions/calculus

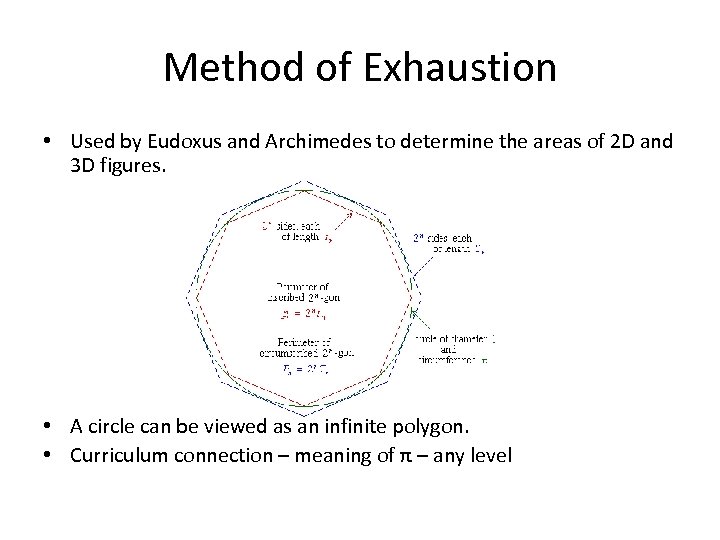Method of Exhaustion • Used by Eudoxus and Archimedes to determine the areas of 2 D and 3 D figures. • A circle can be viewed as an infinite polygon. • Curriculum connection – meaning of π – any level

Calculus • It would be approximately 2000 years until the use potential infinity combined with an extension of these methods was used to develop the concept of a limit and calculus in general. Why did it take so long?

Causes for Lack of Progress • Wars caused people to put their resources into surviving rather than advancing • Narrow mindedness of the Catholic Church

Church Doctrine - Aristotelian thinking became intertwined with Church doctrine due to St. Thomas Aquinas - Aristotle believed the natural numbers are potentially infinite because they have no greatest member. However, he would not allow that they are actually infinite, as he believed it impossible to imagine the entire collection of natural numbers as a completed thing. - Aquinas believed that nowhere in creation was there an actual mathematical infinite, either as a magnitude (a property of a single thing) or as a multitude (a property of a plurality of things). But he accepted the potential infinite, in essentially Aristotelian terms and relying on essentially Aristotelian arguments.

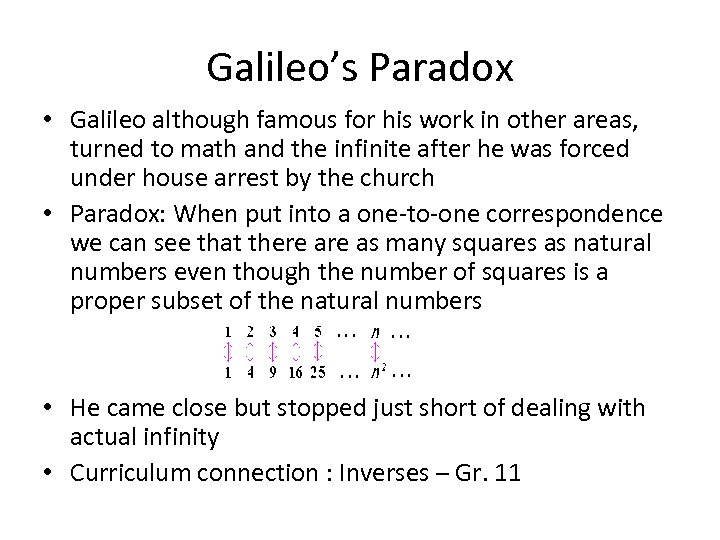Galileo’s Paradox • Galileo although famous for his work in other areas, turned to math and the infinite after he was forced under house arrest by the church • Paradox: When put into a one-to-one correspondence we can see that there as many squares as natural numbers even though the number of squares is a proper subset of the natural numbers • He came close but stopped just short of dealing with actual infinity • Curriculum connection : Inverses – Gr. 11

Bolzano • A one-to-one correspondence can be made for closed intervals of different sizes. For example, using the function y=2 x we can map every x in the domain 0 to 1 uniquely to a y in the range 0 to 2. This implies that there are many numbers on the closed interval 0 to 2 as there are in the closed interval 0 to 1 which is a proper subset. Similarly, it can be shown that there as many numbers in any two closed intervals of different sizes.

Cantor • The most significant figure in the history of mathematical infinity. Cantor completely contradicted the Aristotelian doctrine proscribing actual completed infinities. • The natural numbers are infinite but are considered countable. Cantor recognized the importance of one-to-one correspondence in showing whether any infinite set is countable.

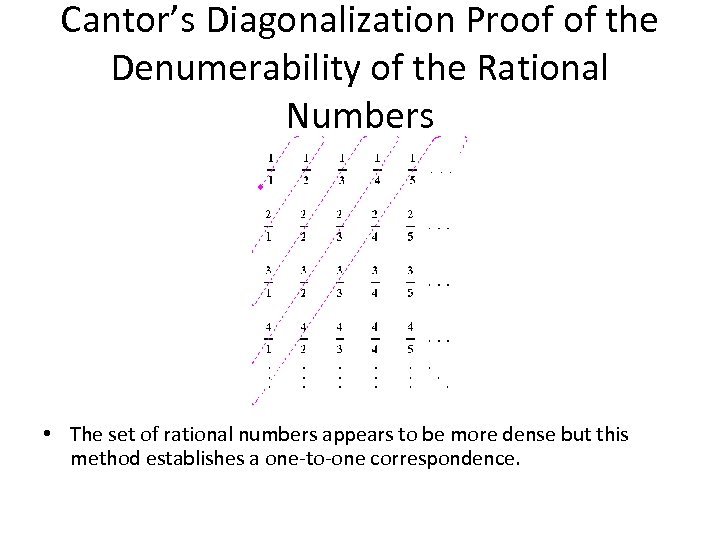Cantor’s Diagonalization Proof of the Denumerability of the Rational Numbers • The set of rational numbers appears to be more dense but this method establishes a one-to-one correspondence.

Cantor’s diagonalization argument • Cantor proved by contradiction that the real numbers are non-denumerable. • Assuming this list is complete leads to a contradiction as a new number can be created that is not on the list.

Transfinite Cardinals • We now have an infinite set of different cardinality than the other infinite sets we have examined and thus Cantor also showed the existence of different types of infinity. Cantor introduced the Jewish letter aleph, , א to denote his different orders of infinity. Cantor believed there were a series of alephs …, 3א , 2א , 1א , 0א to represent the transfinite cardinals. 0א was the lowest infinite cardinal therefore it was the cardinality of the natural numbers. A few questions remained: How many alephs are there? What was the cardinality of the continuum? Was it the next highest cardinal , 1א or some other cardinal?

Transfinite Arithmetic 1) א m + א n = א n for all n ≥ m. 2) א m x א n = א n for all n ≥ m. 3) א n < 2 ^ א n for all nɛN. The first two results show that addition and multiplication do not increase the cardinality of infinity. For example, adding the odd integers and even integers both of cardinality aleph null is equal to the set of all integers which still has cardinality aleph null. The interesting result above is that exponentiation increases the cardinality of a set. Put another way, the power set of a set, always has a higher cardinality than the set itself. This shows that there is no highest cardinal and therefore the set of infinite cardinals is itself infinite.

The Continuum Hypothesis • With this result for power sets, the question of the cardinality of the continuum could now be examined. Every number on the continuum has an infinite decimal expansion. Number systems using different bases are interchangeable therefore we can consider the binary expansion of any number on the continuum. At any position, there is either a 0 or a 1, and the number of positions is countably infinite, therefore the cardinality of the continuum, c, is 2^. 0א • Cantor believed that the continuum was the next cardinality after that of the natural numbers, or 2^. 1א = 0א

God’s Messenger • Mathematics, the role of infinity in particular, as seen in the light of Augustine’s writings(which were Neoplatonistic as opposed to Aristotelian), gained importance during this period as a way to understand God. Cantor believed in his different transfinite cardinals and thought the highest of these the Absolute, was God Himself. He also believed he needed no proof that these transfinite numbers existed because God told him so. • Cantor had made the continuum hypothesis a matter of dogma. He no longer requred the proof that had eluded him for so many years. To him the assumption that 2^ = 0א 1א was not a statement that had to be proved. It was the word of God.

Mental Illness • At first Cantor believed the continuum hypothesis was true but the flip flopped back and forth between trying to prove it true then false. This was likely a major cause of his mental breakdown. Other potential causes were: his relationship with his father, his realization of the forbidden nature of the knowledge he was seeking, opposition from Kronecker which cause him difficulties in the publishing and acceptance of his work.

Zermelo • Zermelo then went on to build on work and axiomatize set theory. To protect his continuum hypothesis from attack, Cantor had to prove every infinite cardinal was one of the alephs. To do this he needed to prove the well-ordering principle – that every set can be well ordered. A set is well ordered if every one of its nonempty subsets has a smallest element. Cantor was not able to prove this but luckily Zermelo came to the rescue and did. In his proof, Zermelo used what he called the axiom of choice. Other mathematicians had issues with this axiom but it turned out in the end that the axiom of choice was equivalent to the wellordering principle.

New Paradoxes • Hilbert’s Infinite Hotel: In an infinite hotel which is ‘full’, one more guest can be accomodated by moving everyone down one room and an infinite number of guests can be accomodated by moving all the occupants to the even rooms thus leaving the odd rooms vacant. • Russell’s paradox: Russell considered the set of all sets that are not members of themselves. Russell called this set R. Then he asked: Is R a member of itself? Here, Russell obtained a paradox. If the set R is a member of itself then it isn’t. And if R is not a member of itself, then it is.

Gödel and Cohen • Like Cantor, when Kurt Gödel began to touch the forbidden concepts of the alephs and actual infinity, he too became mentally ill. He then began trying to design a mathematical proof of the existence of God. Gödel was also half way to proving that the continuum hypothesis was independent of the rest of mathematics but gave that up in the midst of his mental illness. Paul Cohen completed the other half of the proof Gödel started and thus that the continuum hypothesis was independent from all the axioms within the current Zermelo-Fraenkel axiomatic system. The continuum hypothesis can neither be proven true or false.

Conclusion • The concept of infinity has profound philosophical implications, even when being dealt with as a purely mathematical concept. Throughout history, it has been surrounded by controversy and has been immensely difficult for those who dared to confront it.