9aebf79bf6358201095cd66e4eab674d.ppt
- Количество слайдов: 11
Steps Collecting like terms Multiplying numbers and letters Finding the value of expressions Mastering Mathematics © Hodder and Stoughton 2014 Combining variables – Developing Understanding

Collecting like terms Bruce knows that 2 r + r is the same as 3 r. He writes down some other sets of terms that add up to 3 r. 2 r + r = 3 r r + 2 r = 3 r 1. How many sets of terms can you find that add to 4 r? 2. Which expression is the odd one out? a) 3 m + 5 n + 2 n + m + 2 m b) 2 n – 3 m + 4 m + 2 n + 3 n + 5 m c) 10 m + 8 n – 4 m – 6 n + 2 n There were 3+ 2 npossible ways without 3 m + seven Term 2 m = 3 r + 7 n +m+ There are 5 n ways of splitting 6 minto like terms. subtraction. 3 n + 5 m = 6 m + 7 n 2 n. A term+is a single number or a variable. – 3 m 4 m +2 n + using There like 8 n 7 ways of splittingtogether 4 r into like If the were – 4 mbe 6 n +product of+ 4 n A 10 m + terms are collected 6 m term can also – the 2 n = terms. all equivalent to 4 r. they are or variables. numbers Investigate the number ofso it could 5 r. c) does not add to 6 m + 7 n ways for also Examples: be the odd one 5 3 n ab m 7 pq t out. What do you notice? Like terms are terms that use exactly Can you use a formula to describe your the same variable. rule? 5 T and 3 T are like terms as they use Test your formula with other values. the same letter. 6 w and 3 v are not like terms. You can also have different orders. + r is the odd one = 4 r b) r + r + r = 4 r as the out r + 2 r = 4 r r + 2 r + r = 4 r expression has six subtracted terms. 2 r + r = 4 r There are 4 different ways. terms. r + 3 r = 4 r 3 r + r = 4 r 2 r + 2 r = 4 r So there are 7 different ways. r + a) + rtherodd r r + 2 r r is + = 4 one 2 r out ras it r + 2 = 4 has no r + 3 r Menu Back Forward Cont/d More Vocabulary Mastering Mathematics © Hodder and Stoughton 2014 Q 1 Opinion 2 Answer Q 2 Opinion 1 Opinion 2 Answer Combining variables – Developing Understanding
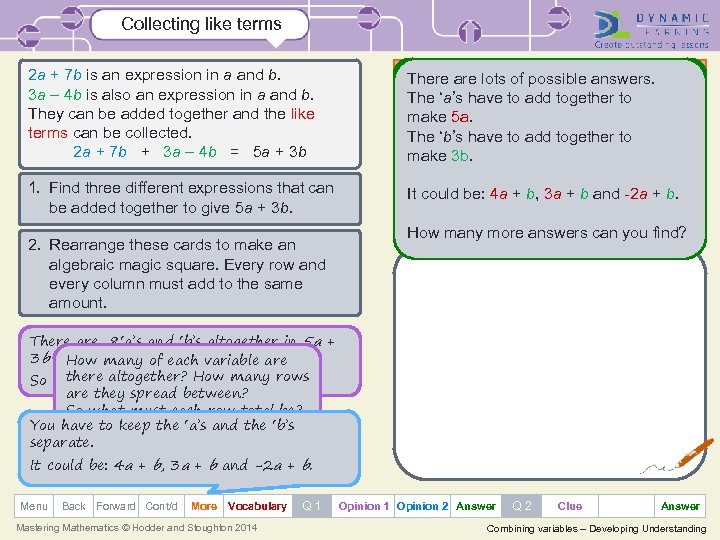
Collecting like terms 2 a + 7 b is an expression in a and b. 3 a – 4 b is also an expression in a and b. They can be added together and the like terms can be collected. 2 a + 7 b + 3 a – 4 b = 5 a + 3 b 1. Find three different expressions that can be added together to give 5 a + 3 b. 2. Rearrange these cards to make an algebraic magic square. Every row and every column must add to the same amount. Make your own of possible answers. There are lots algebraic magic square. Like+ 4 b a terms 5 a – Begin‘a’s + a 2 by add together to you work with 3 b 2 – 2 b The 2 a have to 9 asquare while 2 b Like terms use exactly the same letter out an efficient method. make 5 a. or combination of letters. 7 a have add+together + 3 b 3 b 3 a – a by 3 b + The ‘b’s + 3 b to -2 asquare. a to 4 b Now make be 3 combined into 7 asingle They can a make 3 b. term. 3 a + 3 b called– 2 b -4 aor 4 b This is 9 a + 3 b -4 a + 4 b 3 a collecting + b 3 a – Can you make one with more than two gathering terms. It could be: 4 acolumn adds -2 a b. Each row and+ + b, + 4 a + 2 bto 8 a ++5 b variables? 2 a 3 b 3 a + b and 6 a + 5 b. Example: = Did you find a different answer? How many more answers can you find? There are 8‘a’s and ‘b’s altogether in 5 a + 3 b so. How many same as variable are that’s the of each 8 ab. there altogether? How many rows So the answer could be: ab + 3 ab + 4 ab. are they spread between? So what must each row total be? You have to keep the ‘a’s and the ‘b’s separate. It could be: 4 a + b, 3 a + b and -2 a + b. Menu Back Forward Cont/d More Vocabulary Mastering Mathematics © Hodder and Stoughton 2014 Q 1 Opinion 2 Answer Q 2 Clue Answer Combining variables – Developing Understanding

Collecting like terms Liz is organising an evening at the Spotted Dragon for people at work. She knows how many people are going. She doesn’t know how much the tickets will cost yet. She writes £f for the cost of a full ticket. She writes £d for the cost of a disco ticket. The Spotted Dragon says that the cost will but they Cutting room: how you It doesn’t matter 2 f + 4 d add up the terms, be £ 15 for the Expression full evening and 3 f + for the disco. d terms can’t be £ 5 5 d Sewing room: separately. The f and must be kept How much Despatch: of 2 f + group have to pay? combined. does the 3 d and letters combined together A collection numbers How many different ways can you find to work out the using arithmetic signs. total question asks is the cost of cost? The correct answerfor 13 f + 13 d. the tickets, not the Which isof people who are going. number the easiest way? Why is it easier to gather like terms first? 1. The total cost in pounds of all the tickets for people in the office is 6 f + d. What are the cost in pounds of tickets for each of the other departments? 2. Write an expression for the cost of tickets for the whole group. Cutting + Total = Cutting room 4 d. Sewing room: 2 + 4 = 6 tickets of full tickets + cost of disco Cutting room: 2 f + + Total = cost Sewing room: 3 + 5 = 8 tickets Sewing Despatch + + 5 d room: 3 f Office tickets Despatch: = 2 f Despatch: + 4 d + 3+ + 5 d + 2 f + 3 d + 6 f + d 2 + 3 = 5 tickets 3 f + 2 f + 6 f + 4 d + 5 d + 3 d + d 2 f f 3 d = 2 f + = 13 fd = 13 f + 13 d Menu Back Forward More Vocabulary Mastering Mathematics © Hodder and Stoughton 2014 Q 1 Opinion 2 Answer Q 2 Opinion 1 Opinion 2 Answer Combining variables – Developing Understanding
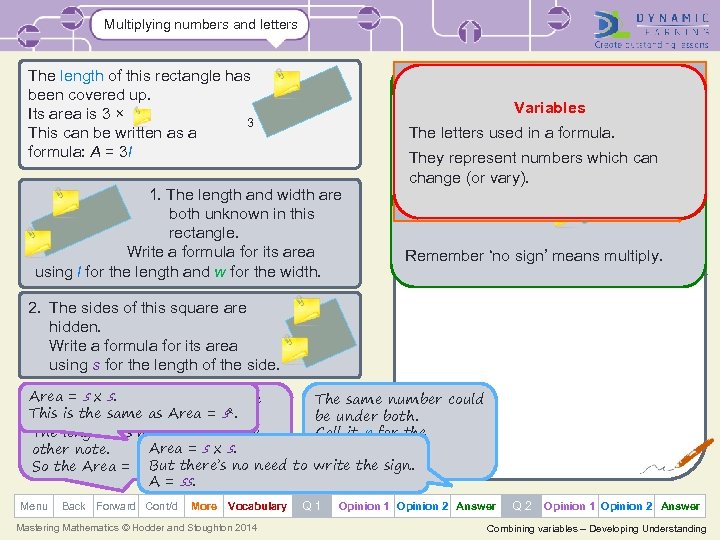
Multiplying numbers and letters The length of this rectangle has been covered up. Its area is 3 × 3 This can be written as a formula: A = 3 l m 1. The length and width are both unknown in this rectangle. 3 Write a formula for its area using l for the length and w for the width. m The length and width are different Variables formulae forin the same way What otherare squaredmust use do you 5 cm measurements Variables so we shapes two as numbers. know? variables. different The letters used in a formula. s 2 you find some Area = that × length Canmeans ls x s. w. formulaewidth can a use They represent l 2 Call them 3 and numbers which cm =2× 5 cube like (orinstead of a square? r change 2 vary). = 10 cm 2 So A = s. A = lw w Remember ‘no sign’ means multiply. 2. The sides of this square hidden. Write a formula for its area using s for the length of the side. Areawidth s. is under one of the The = s x w This is the same as Area = s 2. notes. The length l is hidden under the Area = s x s. other note. But there’s no need to So the Area = lw. A = ss. Menu Back Forward Cont/d More Vocabulary Mastering Mathematics © Hodder and Stoughton 2014 The same number could be under both. Call it n for the number. write = n x n. So A the sign. Q 1 Opinion 2 Answer Q 2 Opinion 1 Opinion 2 Answer Combining variables – Developing Understanding
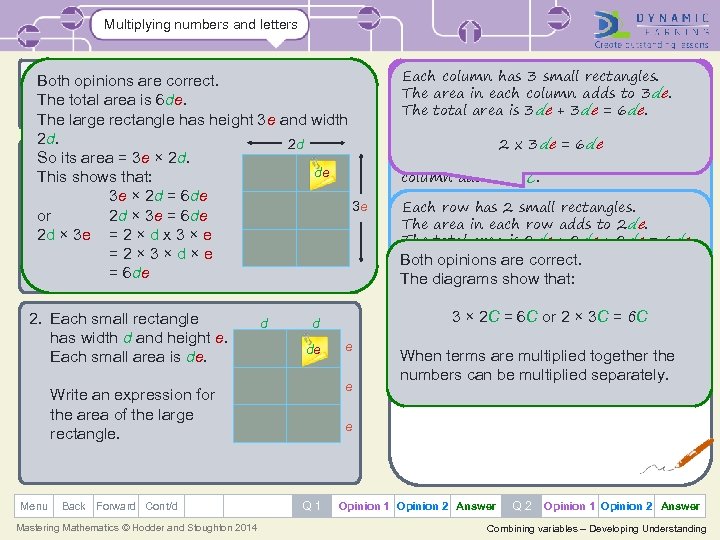
Multiplying numbers and letters Each small rectangle has an area B. Both opinions are correct. Threetotal area is 6 de. the large rectangle. The of them make up The diagram shows that B + 3 e= 3 B. width The large rectangle has height B and There column has 3 small rectangles. 2 C. Each are 3 rows. Each row adds to The area 6 ‘C’s column adds to 3 de. There are in eachaltogether. B The total area C = 6 + So 2 C + 2 is 3 de. C 3 de = 6 de. 2 d. 1. Howarea = ‘C’s×are So its many 3 e 2 d. C there in this This shows that: diagram? Write an 6 de 3 e × 2 d = C orexpression × 3 e = 6 de 2 d that shows = 2 × d 2 d × 3 e how theyx 3 × e C can be counted × e = 2 × 3 in groups. = 6 de B 2 x 3 de = 6 de They are set out in 2 columns. Each column adds to 3 C. B There are 6 ‘C’s altogether. Each row has 2 small rectangles. So 3 C + 3 C = 6 C The area in each row adds to 2 de. The total area is 2 de + 2 de = 6 de. 2. Each small rectangle has width d and height e. Each small area is de. 2 d C C+C=2 C de C C+C=2 C 3 e C C+C=2 C d Back Forward Cont/d Mastering Mathematics © Hodder and Stoughton 2014 e e Write an expression for the area of the large rectangle. Menu 3 × 2 C = 6 C or 2 × 3 C = 6 C d de Both opinions are correct. The diagrams 3 x 2 de = 6 de show that: When terms are multiplied together the numbers can be multiplied separately. e Q 1 Opinion 2 Answer Q 2 Opinion 1 Opinion 2 Answer Combining variables – Developing Understanding
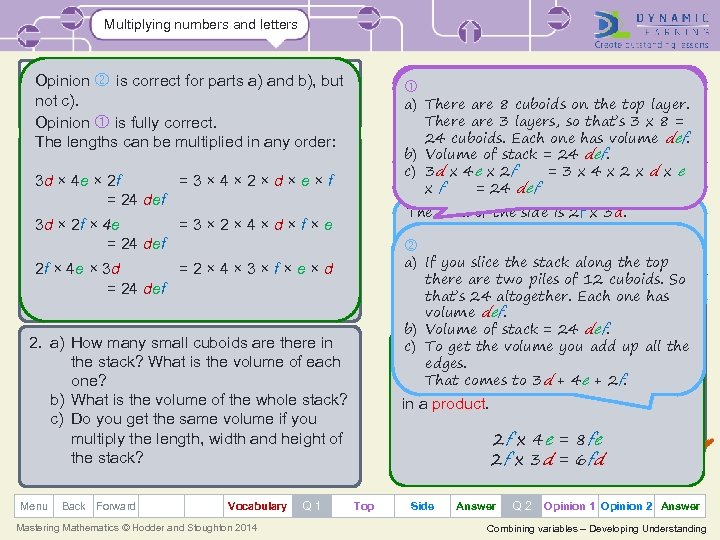
Multiplying numbers and letters The small is correctcm high, e cm wide and Opinion cuboid is d for parts a) and b), but f not long. Its volume is d × e × f cm 3. cm c). This is written as def cm 3. Opinion is fully correct. The lengths can front of the in any 1. The area of thebe multipliedstack is order: The area of the top is 2 f x 4 e. Thef face is made from 8 the top layer. a) There are 8 cuboids onrectangles. Product The area of each is fe. There are 3 layers, so that’s 3 x 8 = 24 cuboids. Each one has volume def. Two This e numbers 2 f = 4 e = 8 fethat are shows that or variables 3 d b) Volume of stack x 24 def. . together. c) 3 d x 4 emultiplied= 3 x 4 x 2 x d x 2 f e x f = 24 def 4 e d 3 d × 4 e. 3 d. The face is made = 3 × 4 × 2 × d × e × f × 4 e × 2 f de 3 d from 12 = 24 def small 3 drectangles. The = 3 × 2 × 4 × d × f × e × 2 f × 4 e 4 e = 24 is de. area of each def 2 f This shows that 3 d × 4 e 4 × 3 × f × e × d × 4 e × 3 d = 2 × = 12 de. Write a similar expression for the area of = 24 def top and side faces. The area of the side is 2 f x 3 d. The face is made from 6 rectangles. The area of each is fd. a) If you slice the stack along the top there are two piles of 12 cuboids. So This shows that 2 f x 3 d = 6 fd that’s 24 altogether. Each one has volume def. b) Volume of stack = 24 def. c) To get the volume you add up all Always multiply the numbers and the edges. variables separately. That numbers before + variables Write thecomes to 3 d + 4 ethe 2 f. 2. a) How many small cuboids are there in the stack? What is the volume of each one? b) What is the volume of the whole stack? c) Do you get the same volume if you multiply the length, width and height of the stack? Menu Back Forward Vocabulary Mastering Mathematics © Hodder and Stoughton 2014 Q 1 in a product. 2 f x 4 e = 8 fe 2 f x 3 d = 6 fd Top Side Answer Q 2 Opinion 1 Opinion 2 Answer Combining variables – Developing Understanding

Finding the value of expressions The statement compares two expressions involving the variable m. 1. Is the statement always true, sometimes true or never true? If you think ‘sometimes’, when is it true and when is it not true? It depends on the value of m. 8 m – 2 is larger than 4 m + 18 when m > 5. When m = 3 8 m – 2 =8× 3– 2 = 24 – 2 = 22 When m = 7 8 m – 2 =8× 7– 2 = 56 – 2 = 54 4 m+18 = 30 4 m+18 = 46 4 m+18 = 38 Smaller When m = 5 8 m – 2 =8× 5– 2 = 40 – 2 = 38 Expression Think of any number. A collection of numbers and letters The value of combined together using arithmetic Can you make 8 m two expressions that up – 2 signs. will bothis more than the value of your give the same value when 4 m + 18 number is substituted into them? Variables The letters used in a formula. Example: They represent numbers which can My number is 5. change (or vary). 8 m to find two expressions that are the I need – 2 is always larger as there are eight ‘m’s compared with only four same when 5 is substituted into them. in 4 m + 18. They could be 3 n + 2 and 22 – n These both have the value 17 when n = 5 is substituted. When m=3 4 m + 18 = 30 But 8 m – 2 = 22 So 8 m – 2 is smaller. Larger The same Menu Back Forward Cont/d More Vocabulary Mastering Mathematics © Hodder and Stoughton 2014 Q 1 Opinion 2 Answer Combining variables – Developing Understanding

Finding the value of expressions Here are three expressions in k. Remember that k 2 means k × k. Also k means × k. To work this out find half of k, or k ÷ 2. 1. Find values of k for which each of these expressions is negative. 2. The three expressions are to be put into order. Find a value of k for each one that gives it the middle value. When k = 1, k + 6 = × 1 in 6 Expressions + There are many values graph which Use graphing software orof k for paper to = that 2 A + 6 way of saying + 6 kthe k quickand of the are negative. draw graphs 8 – 3 k three expressions = variable Largest expression+uses this 6 k 6 above. Superimpose all three on the When k =21, 8 – 3 k = 8 – 3 × 1 But will always be positive whatever samekgraph. 8 – 3 k =8– 3 An value of k. the expression in terms of k would use only the variable k = 5 combined. Middle with Look at where the 2 graphs cross. Can you When k = 1, and arithmetic signs. k =1× 1 numbers Remember that two negative numbers see why each expression can have the =1 Smallest Example: give multiply to 3 k + 7 middle value? 2 a positive number. 4 k – 3 k + 5 What are the critical values of k when the order of the expressions changes? When k = -14, = x -14 When k = 4, kk++66 = × 4 + 6 When k = 2, k + 6 = × 2 + 6 I agree with the first two in =2++ 6 -7 6 = =1+6 = -1 [ k + 6 is negative] Opinion , but the value =8 Middle =7 Largest When k = 5, 8 – 3 k = 8 – 3 x 5 for k 2 is wrong. When k = 4, 8 – 3 k = 8 – 3154 When k = 2, 8 – 3 k = 8 – 3 × 2 =8– × = 8 – 12 – 3 k is negative] =8 6 = -7 [8 k 2 can’t ever be–negative. – 4 =2 Smallest When k = -4, k 2 = -4 x Smallest = -4 – 2 = 4 16 [k 2 is negative] 2 = 2 × 2 When k = 4, k = × 4 When k = 2, k = 16 Largest =4 Middle Menu Back Forward Cont/d More Vocabulary Mastering Mathematics © Hodder and Stoughton 2014 Q 1 Opinion 2 Answer Q 2 Answer 1 Answer 2 Answer 3 Combining variables – Developing Understanding
Finding the value of expressions Avonford Academy awards house points for achievement and voluntary service. House points are taken away if homework is not finished. The expression 3 a + 5 v – 2 h is used to calculate the number of points gained 1. What do the variables a, v and h represent? 2. Jo has volunteered 7 times this term. She thinks there’s been a mistake because her term total is zero points. What do you think? a is has gained 5 v points for achievement. Jo the points awarded for volunteering. v is the worth 5 x 7 = 35 points This is points awarded for volunteering. h is thean odd number. for no homework. That’s points taken off If 2 points are taken off for every missing homework it’ll never get to zero. The lowest a is the number of achievement awards given. it could get to is 1 point. v is the number of volunteering awards given. h is the number of times homework is missing. Menu Back More Mastering Mathematics © Hodder and Stoughton 2014 Q 1 Some possible answers are: Make avspreadsheet to help the House a = 5, = 7, h = 15 Leader keep 3 × 5 + 5 × 7 – 2 points. Points = track of the total × 25 House points = 15 + 35 – 50 Mike goes to 0 Achievement: 3 points = Avonford Academy. He= 25, v Voluntary 55 with 34 points. finishes thehterm a = 7, = service: 5 points He has = 3 No 25 + 5 3 times. × 55 Points volunteered × 7 – 2 points × homework: – 2 = 75 + 35 – 110 How many = 0 could he have missed his times homework? given 3 points for every A student is achievement. So the total points for achievement is 3 × the number of awards. Opinion is wrong because she could a is the number of achievement awards have given. gained some extra points for achievement. v is the number of volunteering awards That could bring her total up to an given. even number before any homework h is the number of times homework is points are taken off. missing. Opinion 1 Opinion 2 Answer Q 2 Opinion 1 Opinion 2 Answer Combining variables – Developing Understanding
Editable Teacher Template Information Vocabulary 1. Task – fixed More 2. Task – appears Q 1 Opinion 1 Q 1 Opinion 2 Q 1 Answer Q 2 Opinion 1 Q 2 Opinion 2 Q 2 Answer Menu Back Forward More Vocabulary Mastering Mathematics © Hodder and Stoughton 2014 Q 1 Opinion 2 Answer Q 2 Opinion 1 Opinion 2 Answer Combining variables – Developing Understanding
9aebf79bf6358201095cd66e4eab674d.ppt