Solving 1 st power equations in one variable

Solving 1 st power equations in one variable: 1. A. 5 x + 8 = 2(2 x + 4) + x B. 6 x + 3 = 2(3 x + 5) 6 x + 3 = 6 x + 10 5 x + 8 = 5 x + 8 { all reals } 3 10 { no reals } 2. A. ½ x + 8 = 12 B. 6 = x (2)( ½ x + 8) = 2 (12) 5 (6) = 5 ( x) x + 16 = 24 30 = 2 x 15 = x x = 8 3. A. + 8 = 17 B. + 4 = 33 1 + 16 x = 17 8 x = 32 x = 4 16 x = 16 x = 1

Addition Property (of Equality) Example: If a = b, then a + c = b + c Multiplication Property (of Equality) Example: If a = b, then ca = cb

Reflexive Property (of Equality) Example: If “a” is a real number, then a = a Symmetric Property (of Equality) Example: If a = b, then b = a. Transitive Property (of Equality) Example: If a = b, and b = c, then a = c.

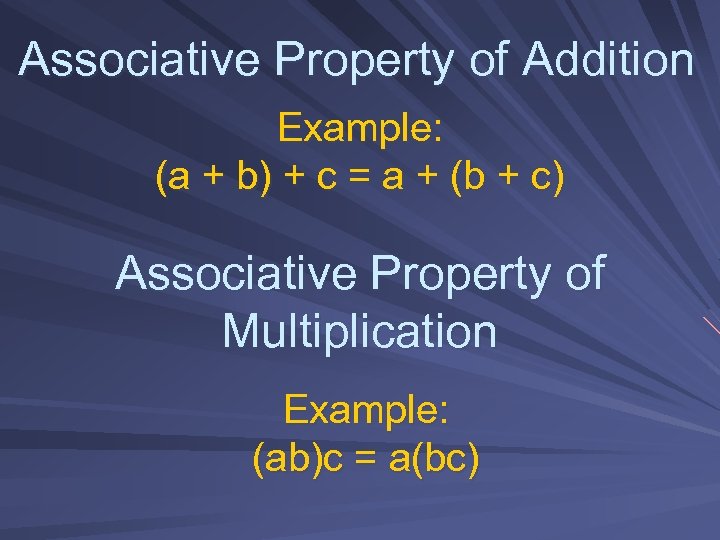
Associative Property of Addition Example: (a + b) + c = a + (b + c) Associative Property of Multiplication Example: (ab)c = a(bc)

Commutative Property of Addition Example: a + b = b + a Commutative Property of Multiplication Example: ab = ba

Distributive Property (of Multiplication over Addition) Example: a(b + c) = ab + ac

Prop of Opposites or Inverse Property of Addition Example: a – a = 1 Prop of Reciprocals or Inverse Prop. of Multiplication Example: a • 1/a = 1 and 1/a • a = 1

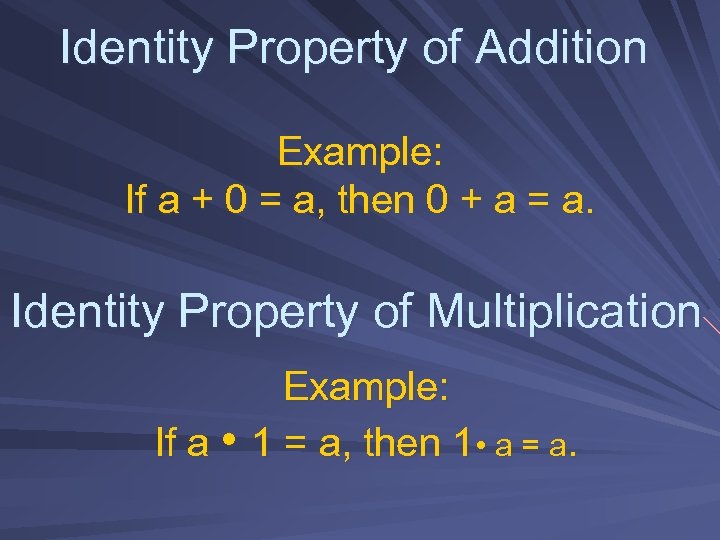
Identity Property of Addition Example: If a + 0 = a, then 0 + a = a. Identity Property of Multiplication Example: If a • 1 = a, then 1 • a = a.

Multiplicative Property of Zero Example: If a • 0 = 0, then 0 • a = 0. Closure Property of Addition Example: a + b is a unique real number Closure Property of Multiplication Example: ab is a unique real number

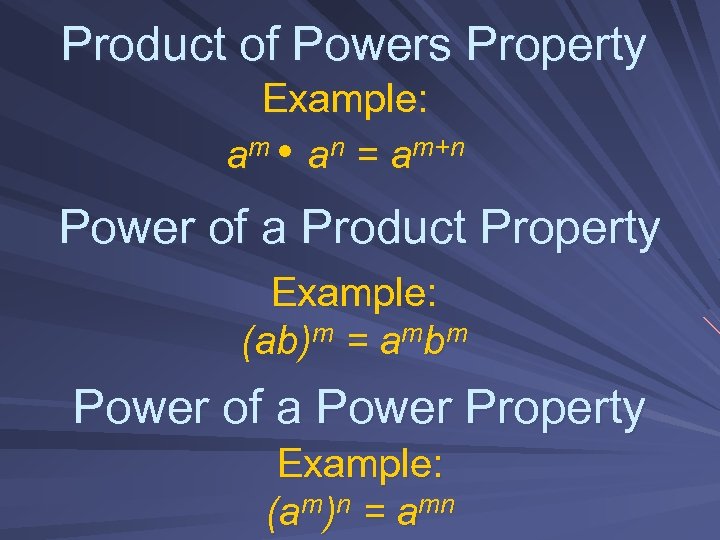
Product of Powers Property Example: am • an = am+n Power of a Product Property Example: (ab)m = ambm Power of a Power Property Example: (am)n = amn

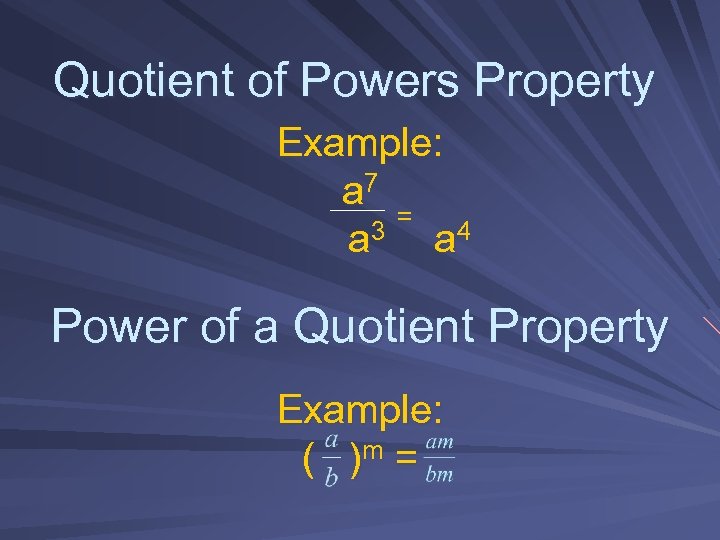
Quotient of Powers Property Example: a 7 = 3 a 4 a Power of a Quotient Property Example: ( )m =

Zero Power Property Example: x 0 =1 Negative Power Property Example: x-2 =1/x 2

Zero Product Property Example: If ab = 0, then a = 0 or b = 0.

Product of Roots Property Example: 20= 5. 4 Quotient of Roots Property Example: 16 = = 4 9 3

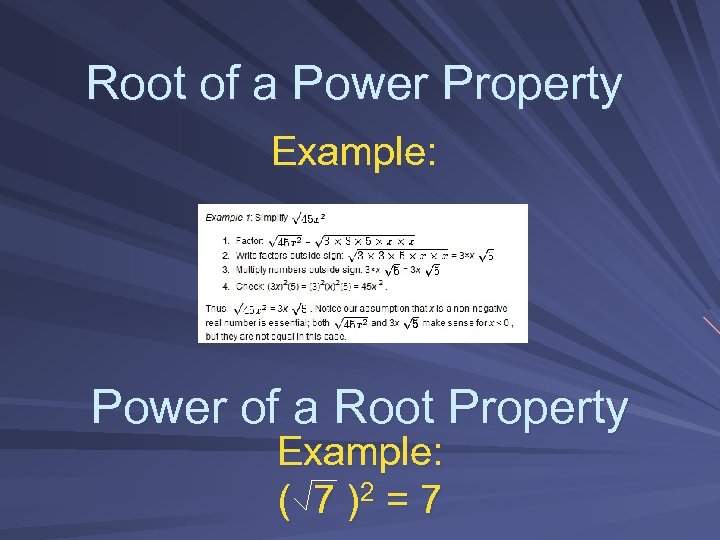
Root of a Power Property Example: Power of a Root Property Example: ( 7 )2 = 7

Quiz on Properties Click when you’re ready to see the answer. Question Answer 1. 2. 3. 4. 1. Commutative Property (of Addition) 2. Prop of Opposites or Inverse Property of Addition 3. Distributive Property (of Multiplication over Addition) 4. Zero Product Property 5. Zero Power Property 6. Negative Power Property 7. Symmetric Property (of Equality) a + b = b + a a – a = 1 a(b + c) = ab + ac If ab = 0, then a = 0 or b = 0. 5. x 0 =1 6. x-2 =1/x 2 7. If a = b, then b = a. 8. If a = b, then ca = 8. Multiplication Property (of Equality) cb 9. Commutative Property of Multiplication 9. ab = ba 10. Power of a Quotient Property 10. ( )m =

Solving 1 st power inequalities in one variable. (Watch for special cases of {all reals} and those which have no solution. ) A. With only one inequality sign 4 x > 28 x > 7 Click to view answer. B. Conjunction Whenever you see “and”, the inequality is always going to be a conjunction. 8 < 4 – 2 x > 12 -2 > x < -4 This problem is a conjunction although it does not have “and” in it. C. Disjunction Whenever you see “or”, the inequality is always going to be a disjunction. z < 4 OR z ≥ 4 { All Real Numbers}

Factoring out the Greatest Common Factor (GCF) is perhaps the most used type of factoring because it occurs as part of the process of factoring other types of products. Before you can factor trinomials, for example, you should check for any GCF. 1: Factor the following problem completely 2 x-14 2(x -7) Look for the greatest factor common to every term Often there is no factor common to all terms of a polynomial. However there WILL be factors common to some of the terms. A second technique of factoring called grouping is illustrated in the following examples. 2. Factor the following problem completely 3 ax+6 ay+4 x+8 y (3 a+4)(x+2 y) The glob (3 a + b) has now become the GCF. Factor out 3 a from the first 2 terms and 4 from the last 2 terms.

Factoring Cont. The definition of the difference in two perfect squares states that there must be two terms, the sign between the two terms is a minus sign, and each of the two terms MUST have perfect squares. The answer includes two binomials found after factoring the difference in two squares. One of the binomials contains the sum of two terms and the other contains the difference of two terms. A good example is: a 2 – b 2 = 9 a + b) (a- b) 6: Factor the following problem completely: 9 x 2 – 16 y 4 1. Examine the problem for a GCF. (There is none) 2. Take the square root of each term. 3 x and 4 y 2 (3 x+4 y)(3 x-4 y) 3. Use the square roots to fill in the parentheses. Be sure to check that neither factor can be factored again.

Factoring Cont. Factoring the sum or difference in two perfect cubes is our next technique. As with squares, the difference in two cubes means that there will be two terms and each will contain perfect cubes and the sign between the two terms will be negative. The sum of two cubes would, of course, contain a plus sign between the two perfect cube terms. The follow formulas are helpful for factoring cubes: Sum: Difference: Notice that the sum and the difference are exactly the same except for the signs in the factors. Many students have found the acronym SOAP extremely helpful for remembering the arrangement of the signs. S represents the fact that the sign between the two terms in the binomial portion of the answer will always be the same as the sign in the given problem. O implies that the sign between the first two terms of the trinomial portion of the answer will be the opposite of the sign in the problem. AP states that the sign between the final two terms in the trinomial will be always positive. Factor the following problem completely: x 3 - 27 (x 3)( ) (x 3)(x 2 9) (x 3)(x 2 3 x 9) (x-3)(x 2+3 x+9) In the first set, there will be a binomial containing the cube root of each term. In this problem, x and 3. In the second set there will be a trinomial. The first term of the trinomial is the square of the first term in the binomial. The last term is the square of the last term in the binomial. The middle term is the product of the two terms in the binomial.

Factoring Cont. Before factoring a trinomial, examine the trinomial to be sure that terms are arranged in descending order. Most of the time trinomials factor to two binomials in product form. Factor the following problem completely. 12 x 3 – 5 x 2 – 3 x x(12 x 2 – 5 x - 3) x(4 x )(3 x ) x(4 x + 3)(3 x – 1) x(12 x 2 – 4 x + 9 x – 3) x(12 x 2 + 5 x – 3)

Factoring Cont. A general trinomial is one whose first term has a coefficient that can not be factored out as a GCF. The method of trial and error will be used to mentally determine the factors that satisfy the trinomial. We will show you the steps to factor each of the following general trinomials completely. Factor the following problem completely. Factor out the GCF. In factoring the general trinomial, begin with the factors of 12. These include the following: 1, 12, 2, 6, 3, 4. As a general rule, the set of factors closest together on a number line should be tried first as possible factors for the trinomial. The only factors of the last term of the trinomial are 1 and 3, so there are not other choices to try. Because the last term is negative the signs of the factors 1 and 3 must be opposite. This is the first trial. The answer must be checked by multiplication, as follows:

Graphing To graph you will need to create a line graph and place it so x is equal or greater than five. To graph you will need to create the line graph and place it so that x will be smaller than -5 There is be a dark dot for “greater than or equal” and for “less than or equal”.

Graphing It is the same with greater than or less than but there is just a circle on the graph instead of the dot. If there are two equations and they use the word “and” then you shade in the overlapping area on the graph. However if the 2 equations has the word “or” used in connecting them then you know to graph the two on the same line.

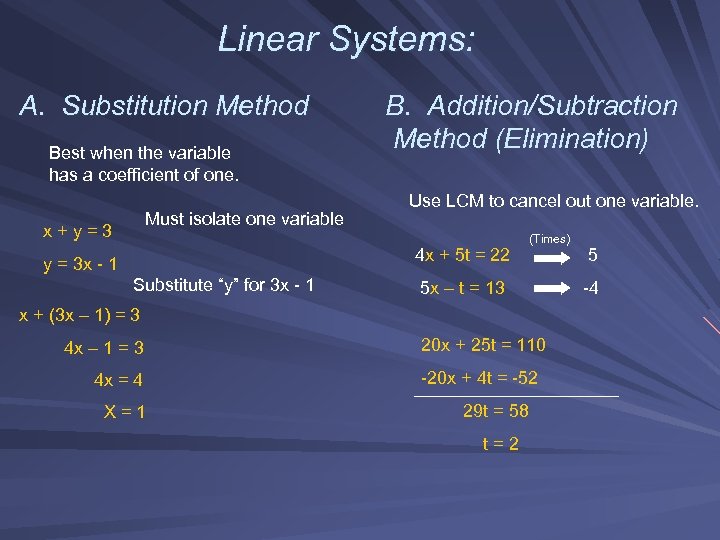
Linear Systems: A. Substitution Method Best when the variable has a coefficient of one. Must isolate one variable x + y = 3 B. Addition/Subtraction Method (Elimination) Use LCM to cancel out one variable. 4 x + 5 t = 22 y = 3 x - 1 Substitute “y” for 3 x - 1 (Times) 5 x – t = 13 x + (3 x – 1) = 3 4 x – 1 = 3 20 x + 25 t = 110 4 x = 4 -20 x + 4 t = -52 X = 1 29 t = 58 t = 2 5 -4

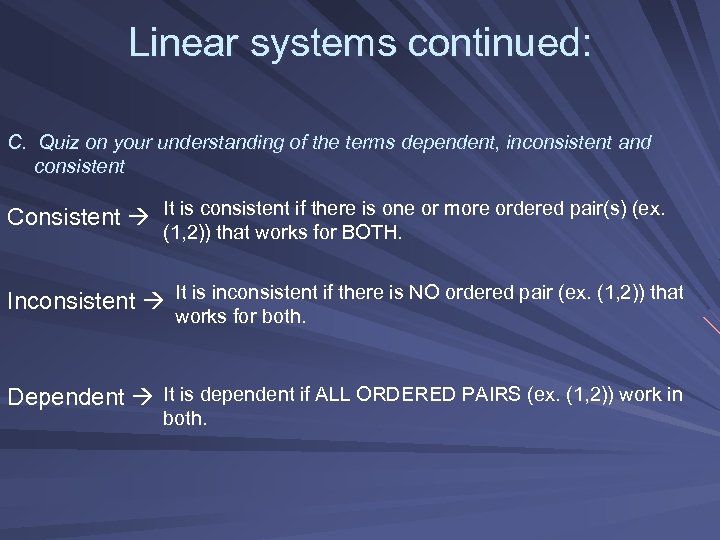
Linear systems continued: C. Quiz on your understanding of the terms dependent, inconsistent and consistent Consistent It is consistent if there is one or more ordered pair(s) (ex. (1, 2)) that works for BOTH. Inconsistent It is inconsistent if there is NO ordered pair (ex. (1, 2)) that works for both. Dependent It is dependent if ALL ORDERED PAIRS (ex. (1, 2)) work in both.

Rational expressions (a) A rational number is a number expressed as a quotient of two integers 16 = 8 2 3 a 1 = 6 a 2

Rational expressions (b) x +2 1 x - 1 = 3 x 6 2 x 2 + 4 x -6 = x 2 - x x 2 + 5 x – 6 = 0 (x + 6) (x – 1) = 0 x = -6 x = 1

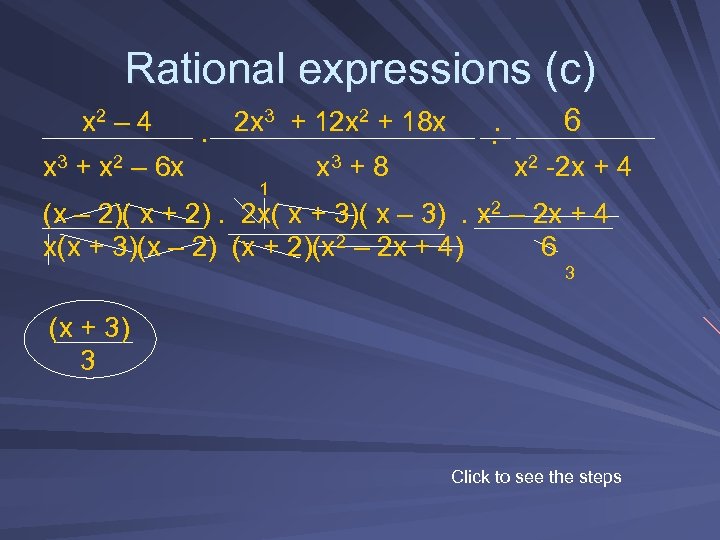
Rational expressions (c) x 2 – 4 . 2 x 3 + 12 x 2 + 18 x . . 6 x 3 + x 2 – 6 x x 3 + 8 x 2 -2 x + 4 1 (x – 2)( x + 2). 2 x( x + 3)( x – 3) . x 2 – 2 x + 4 x(x + 3)(x – 2) (x + 2)(x 2 – 2 x + 4) 6 3 (x + 3) 3 Click to see the steps

Functions (a) NOT ALL RELATIONS ARE FUNCTIONS!! F(x) is the same as y

Functions (b) The domain is the x-coordinates in a function. The range is the y-coordinates in a function.

Functions (c) If you are given two points on a graph you will use point slope formula to find a linear equation that works for the two given points.

Functions (d) Graphing the Parabola y = ax 2 + bx + c 1. Find whether the parabola opens upward or downward. a. If a > 0, it opens upward. b. If a < 0, it opens downward. -b 2. Find the vertex. 2 a a. The x-coordinate is . b. The y-coordinate is found by substituting the x-coordinate, from the previous step, in the equation y = ax 2 + bx + 3. Find the y-intercept by setting x = 0. 4. Find the x-intercepts (if any) by setting y = 0, and then solving the equation ax 2 + bx + c = 0. 5. Find two or three other points if there are no x-intercepts.

Quadratic Function The discriminant tells me if the equation will work or not The discriminant is b 2 -4 ac You will use the factoring methods we have talked about to solve these functions as well as any type of function.

Simplifying expressions with exponents a 7 Simply subtract the amount of the lower part from the higher part. = a 3 x 4 (a 2)3 = a 6 Simply multiply the exponents. a 3 a 3 Simply multiply the variables and keep it a division problem. b = b 3 (9 xy)0 = 1 Anything by the 0 exponent is ALWAYS going to be 1. A-2 = 1 When dealing with negative exponents simply make it a fraction with a 2 the exponent on the bottom of the fraction with the bottom number of the previous fraction (a 2/1) on top (the 1 goes on top).

Simplifying Expressions with Radicals 4 In a problem like , it doesn’t take too much brain power to know that the answer 2. Even a problem like 27 3 = 3 is easy once we realize 3 x 3 x 3 = 27. Our trouble usually occurs when the number under our radical sign is not a perfect square or a perfect cube. A problem like 24 may look difficult because there is no square root of 24. However, the problem can be simplified. So even though 24 is not a perfect square, it can be broken down into smaller pieces where one of those pieces might be perfect square. Simplifying a radical expression can involve variables as well as numbers. Just as you were able to break down a number into its smaller pieces, you can do the same with variables. When the radical is a square root, you should try to have terms raised to an even power (2, 4, 6, 8, and so on) When the radical is a cube root, you should try to have terms raised to a power of three (3, 6, 9, 12, and so on) For example; 24 = 4 x 6 = 2 6 These types of simplifications with variables will be helpful when doing operations with radical expressions.

Word problems: 1. A chemist mixes 12 L of a 45% acid solution with 8 L of a 70% acid solution. Find the % of acid in the water. Concentration: 1 st solution: 45% 2 nd solution: Final mixture: 70% x Volume: 12 L 8 L 20 L Fin. conc. x fin. vol. = conc 1 vol 1 conc 2 vol 2 Mult. By 100 x + 20 = (. 45)(12) + (. 70)(8) 2000 x = 540 + 560 2000 x = 1100 x = 1100/2000 or 11/20 x = 55%

Word problems: If Bob can paint a house in 4 hours, and Jimmy can paint the same house in 6 hours, how long will it take for both of them to paint the house together? a. 3 hours and 12 minutes b. 4 hours and 33 minutes c. 3 hours and 44 minutes d. 2 hours and 24 minutes If Sally can paint the house in 4 hours, then in 1 hour she can paint 1/4 of the house. If John can paint the house in 6 hours, then in 1 hour he can paint 1/6 of the house. Let x = number of hours it would take them together to paint the house, then working together, in 1 hour they can paint 1/x of the house. The equation is this: 1 1 1 + = 4 6 x Multiply both sides by the LCD (which is 12 x): (12 x) (1/4) + (12 x) (1/6) = (12 x) (1/x) 3 x + 2 x = 12 x 12 = = 2. 4 hours or d 5

Word Problem If 2 pens and 3 notebook cost 4. 55 and 3 pens and 2 note books cost 3. 70, find the price of each pen a. $1. 25 b. $0. 50 c. $0. 40 d. $1. 00 e. $0. 67 -2(2 p+3 n=4. 55) 3(3 p+2 n=3. 70) (Use elimination method) (9 p = 6 n) = 11. 1 + (-4 – 6 n) = - 9. 1 p=0. 40 (c)

Line of Best Fit or Regression Line A regression line is a line drawn through a scatter plot of two variables. The line is chosen so that it comes as close to the most amount of points as possible. A graphing calculator is very helpful for finding this because it has a program in it to do this. We have a handout that you should have kept about it.