Solar Radiation The energy emitted by the Sun

Solar Radiation The energy emitted by the Sun is called SOLAR RADIATION. It is the only source of energy for the Earth. Other sources: Earth’s surface – 5000 times less, Stars – 30. 000 times less. When arriving to Earth, the larger part of the solar radiation (SR) transforms to heat energy, and a small portion of it to electric energy (upper atmosphere). area receives annually of energy 1

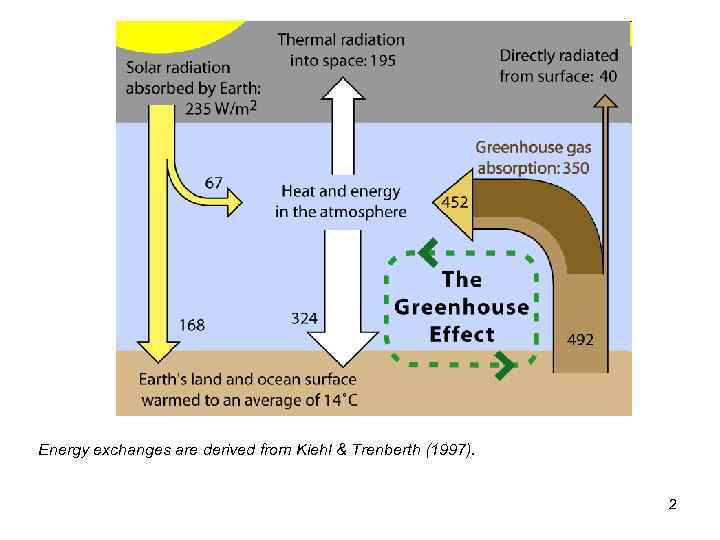
Energy exchanges are derived from Kiehl & Trenberth (1997). 2

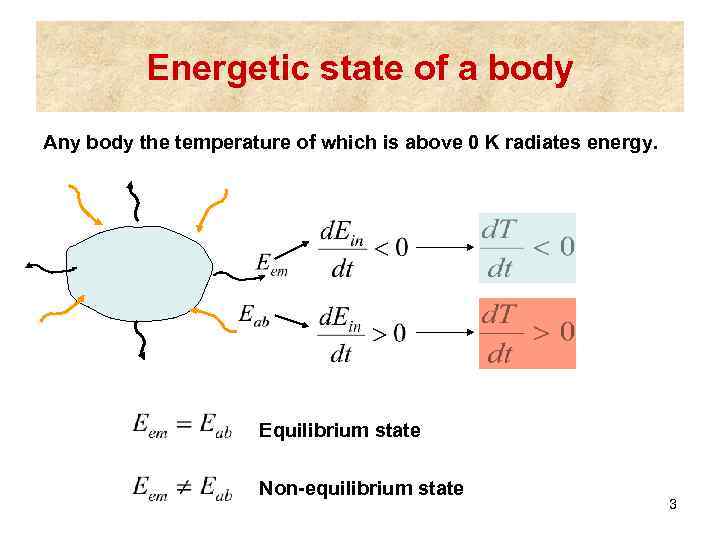
Energetic state of a body Any body the temperature of which is above 0 K radiates energy. Equilibrium state Non-equilibrium state 3

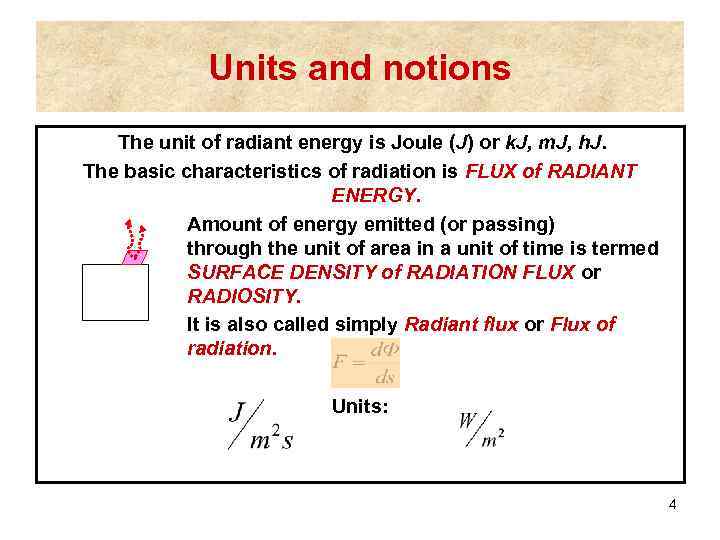
Units and notions The unit of radiant energy is Joule (J) or k. J, m. J, h. J. The basic characteristics of radiation is FLUX of RADIANT ENERGY. Amount of energy emitted (or passing) through the unit of area in a unit of time is termed SURFACE DENSITY of RADIATION FLUX or RADIOSITY. It is also called simply Radiant flux or Flux of radiation. Units: 4

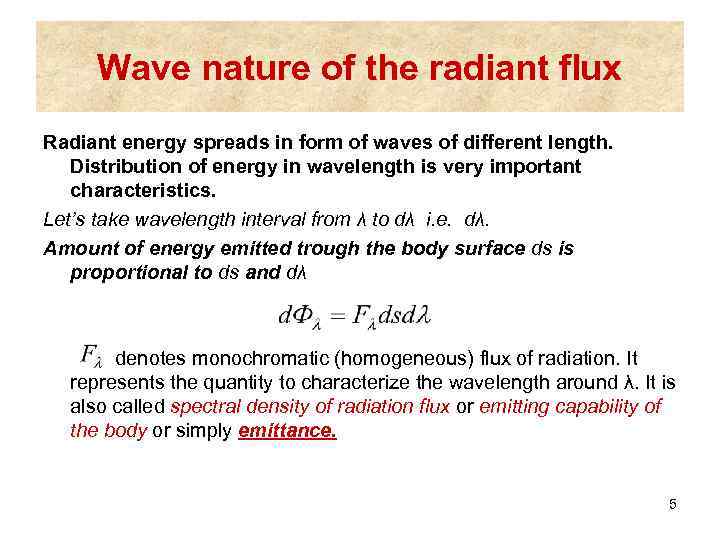
Wave nature of the radiant flux Radiant energy spreads in form of waves of different length. Distribution of energy in wavelength is very important characteristics. Let’s take wavelength interval from λ to dλ i. e. dλ. Amount of energy emitted trough the body surface ds is proportional to ds and dλ denotes monochromatic (homogeneous) flux of radiation. It represents the quantity to characterize the wavelength around λ. It is also called spectral density of radiation flux or emitting capability of the body or simply emittance. 5

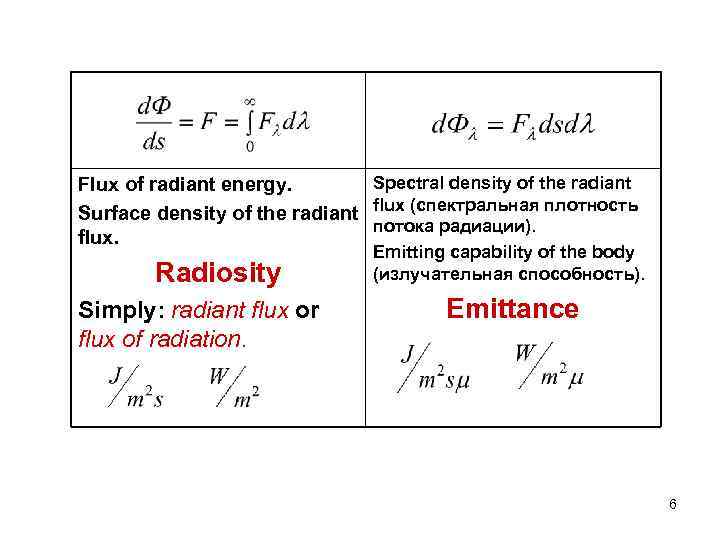
Spectral density of the radiant Flux of radiant energy. Surface density of the radiant flux (спектральная плотность потока радиации). flux. Radiosity Simply: radiant flux or flux of radiation. Emitting capability of the body (излучательная способность). Emittance 6

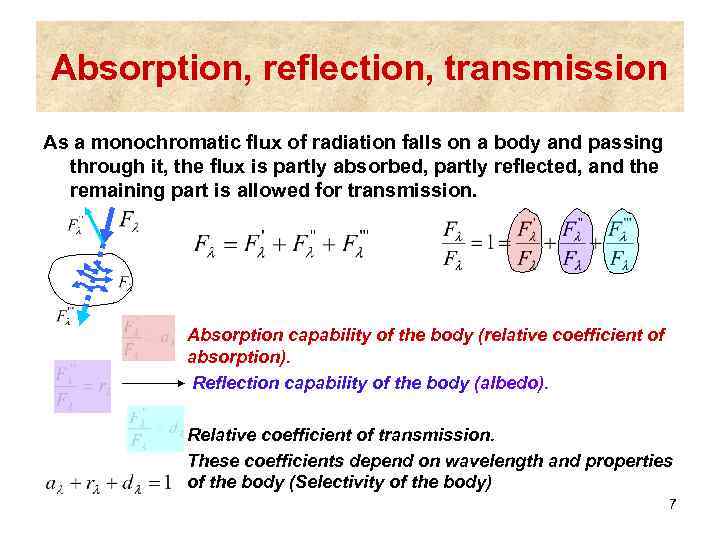
Absorption, reflection, transmission As a monochromatic flux of radiation falls on a body and passing through it, the flux is partly absorbed, partly reflected, and the remaining part is allowed for transmission. Absorption capability of the body (relative coefficient of absorption). Reflection capability of the body (albedo). Relative coefficient of transmission. These coefficients depend on wavelength and properties of the body (Selectivity of the body) 7

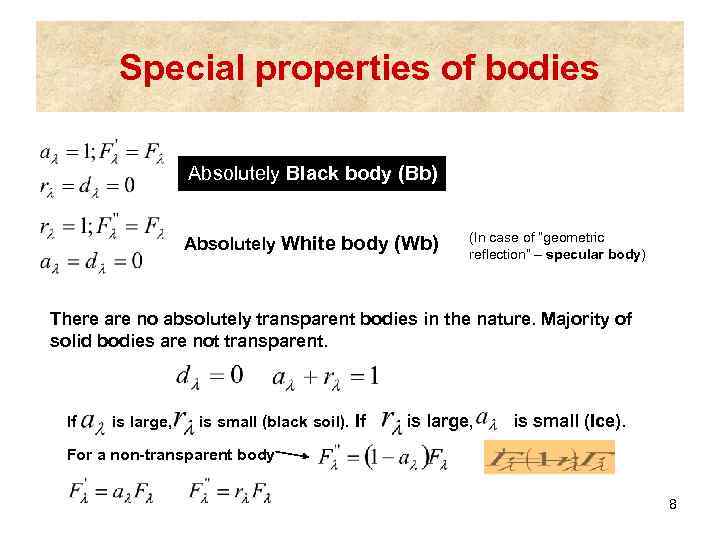
Special properties of bodies Absolutely Black body (Bb) Absolutely White body (Wb) (In case of “geometric reflection” – specular body) There are no absolutely transparent bodies in the nature. Majority of solid bodies are not transparent. If is large, is small (black soil). If is large, is small (Ice). For a non-transparent body 8

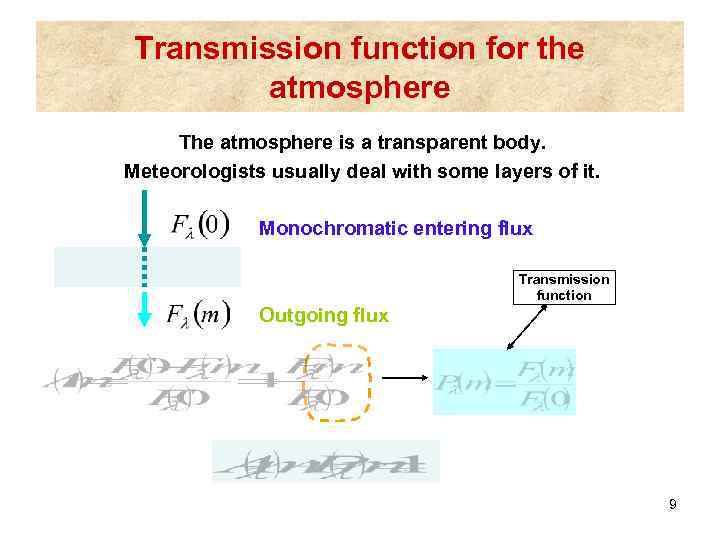
Transmission function for the atmosphere The atmosphere is a transparent body. Meteorologists usually deal with some layers of it. Monochromatic entering flux Transmission function Outgoing flux 9

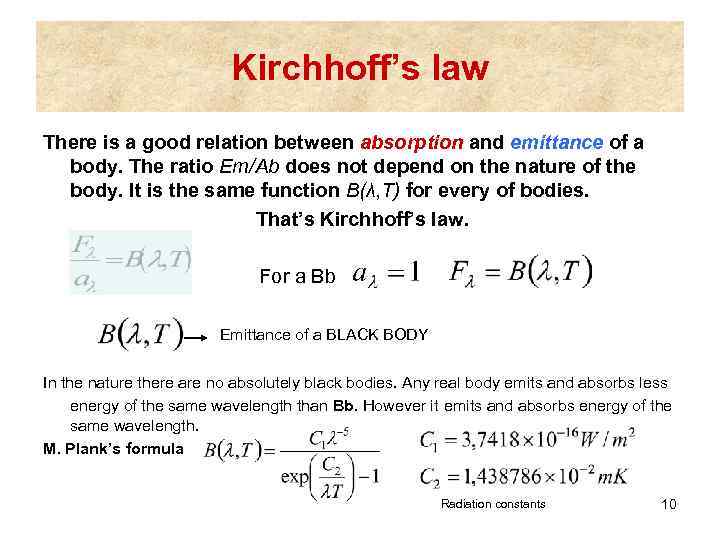
Kirchhoff’s law There is a good relation between absorption and emittance of a body. The ratio Em/Ab does not depend on the nature of the body. It is the same function B(λ, T) for every of bodies. That’s Kirchhoff’s law. For a Bb Emittance of a BLACK BODY In the nature there are no absolutely black bodies. Any real body emits and absorbs less energy of the same wavelength than Bb. However it emits and absorbs energy of the same wavelength. M. Plank’s formula Radiation constants 10

Gustav Robert Kirchhoff 1824 – 1887 Born Königsberg, Kingdom of Prussia He coined the term "black body" radiation in 1862 Wilhelm Wien 1864 – 1928 born at Gaffken near Fischhausen (Rybaki), Province of Prussia (now Primorsk, Russia) In 1896 Wien empirically determined a distribution law of blackbody radiation, later named after him: Wien's 11

Max Planck • 1858 – 1947 • Planck was gifted when it came to music. He took singing lessons and played piano, organ and cello(Violoncello ), and composed songs and operas. However, instead of music he chose to study physics. 12

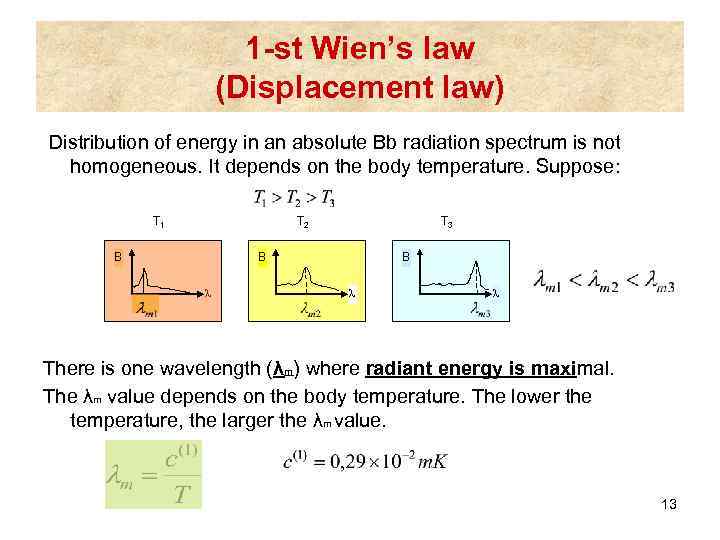
1 -st Wien’s law (Displacement law) Distribution of energy in an absolute Bb radiation spectrum is not homogeneous. It depends on the body temperature. Suppose: T 1 T 2 B T 3 B λ λ There is one wavelength (λm) where radiant energy is maximal. The λm value depends on the body temperature. The lower the temperature, the larger the λm value. 13

Practical application of the 1 Wien’s law? 14

• Much of a person's energy is radiated away in the form of infrared light. Some materials are transparent in the infrared, while opaque to visible light, as is the plastic bag in this infrared image (bottom). Other materials are transparent to visible light, while opaque or reflective in the infrared, noticeable by darkness of the man's glasses. http: //en. wikipedia. org/wiki/Black_body 15

Temperatures of flames by appearance The temperature of flames with carbon particles emitting light can be assessed by their color: • Red – – – • Just visible: 525 °C (980 °F) Dull: 700 °C (1, 300 °F) Cherry, dull: 800 °C (1, 500 °F) Cherry, full: 900 °C (1, 700 °F) Cherry, clear: 1, 000 °C (1, 800 °F) Orange – Deep: 1, 100 °C (2, 000 °F) – Clear: 1, 200 °C (2, 200 °F) • White – Whitish: 1, 300 °C (2, 400 °F) – Bright: 1, 400 °C (2, 600 °F) – Dazzling: 1, 500 °C (2, 700 °F) • http: //en. wikipedia. org/wiki/Fire#Typical_temperatures_of_fires_and_flames 16

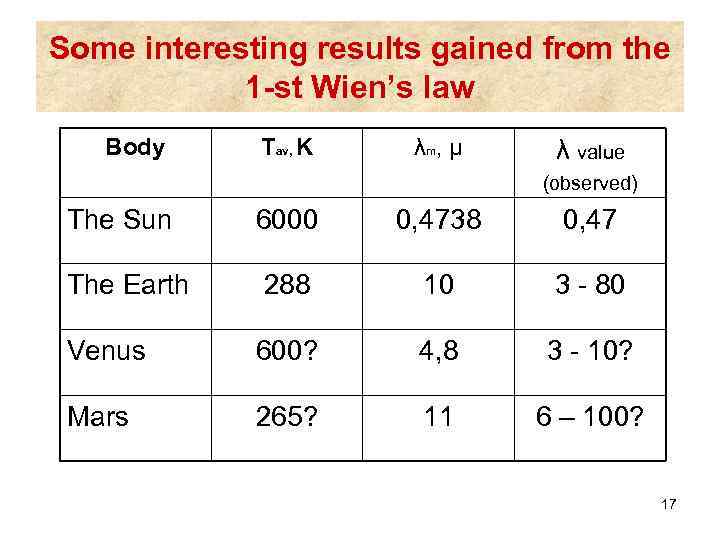
Some interesting results gained from the 1 -st Wien’s law Body Tav, K λm, μ λ value (observed) The Sun 6000 0, 4738 0, 47 The Earth 288 10 3 - 80 Venus 600? 4, 8 3 - 10? Mars 265? 11 6 – 100? 17

The total flux and 2 -nd Wien’s law The total flux of Bb radiation includes energy of all wavelengths emitted by the body. After integration Stephan-Boltzman constant 2 -nd Wien’s law 18

Grey body Since in the nature there are no absolutely black bodies, we may call of them grey bodies. The grey body is a body the absorption capability of which is the same for every wavelength. Radiation flux of any grey body can be presented as; 19

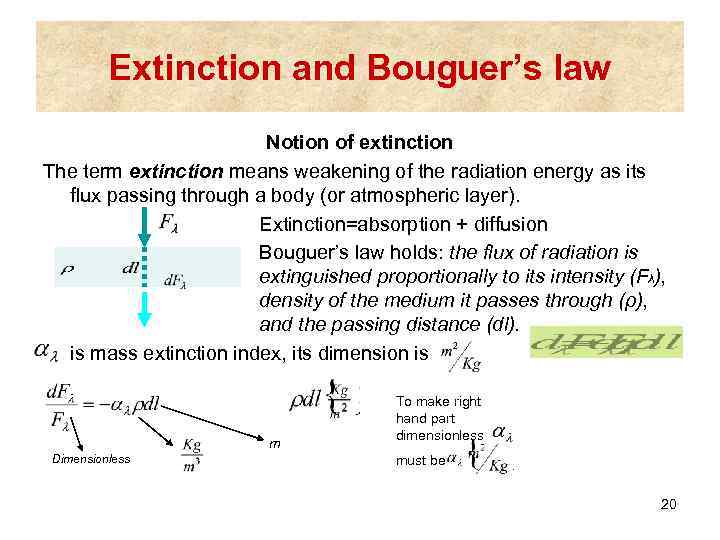
Extinction and Bouguer’s law Notion of extinction The term extinction means weakening of the radiation energy as its flux passing through a body (or atmospheric layer). Extinction=absorption + diffusion Bouguer’s law holds: the flux of radiation is extinguished proportionally to its intensity (Fλ), density of the medium it passes through (ρ), and the passing distance (dl). is mass extinction index, its dimension is m Dimensionless To make right hand part dimensionless must be 20

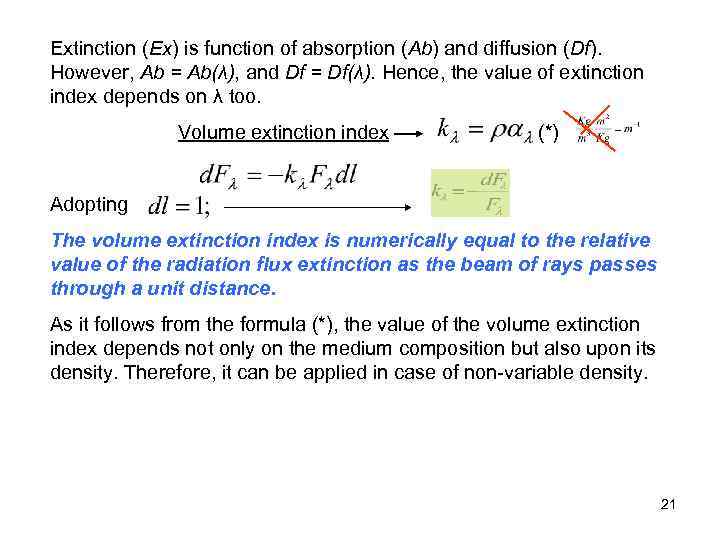
Extinction (Ex) is function of absorption (Ab) and diffusion (Df). However, Ab = Ab(λ), and Df = Df(λ). Hence, the value of extinction index depends on λ too. Volume extinction index (*) Adopting The volume extinction index is numerically equal to the relative value of the radiation flux extinction as the beam of rays passes through a unit distance. As it follows from the formula (*), the value of the volume extinction index depends not only on the medium composition but also upon its density. Therefore, it can be applied in case of non-variable density. 21

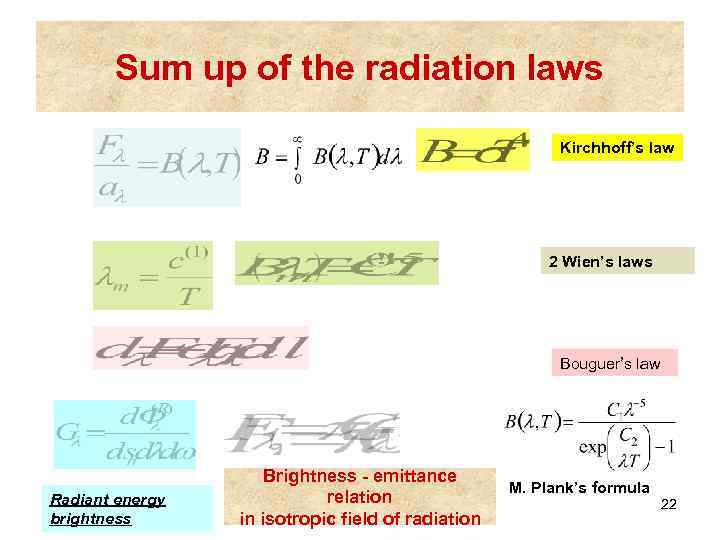
Sum up of the radiation laws Kirchhoff’s law 2 Wien’s laws Bouguer’s law Radiant energy brightness Brightness - emittance relation in isotropic field of radiation M. Plank’s formula 22

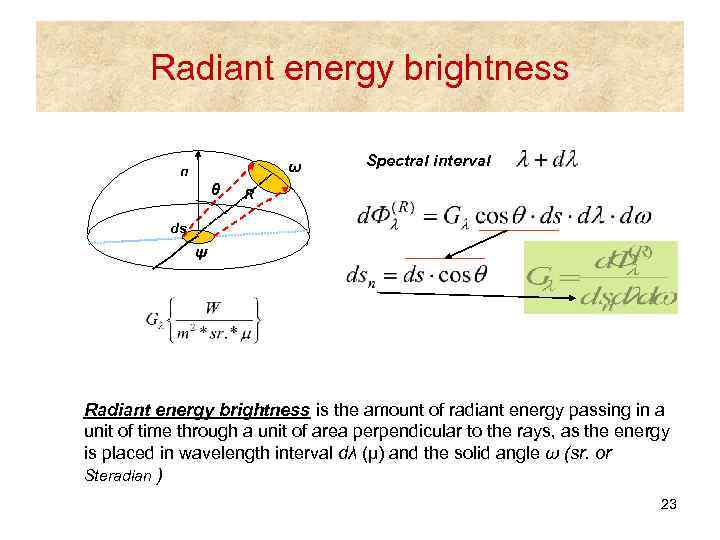
Radiant energy brightness ω n θ Spectral interval R ds ψ Radiant energy brightness is the amount of radiant energy passing in a unit of time through a unit of area perpendicular to the rays, as the energy is placed in wavelength interval dλ (μ) and the solid angle ω (sr. or Steradian ) 23

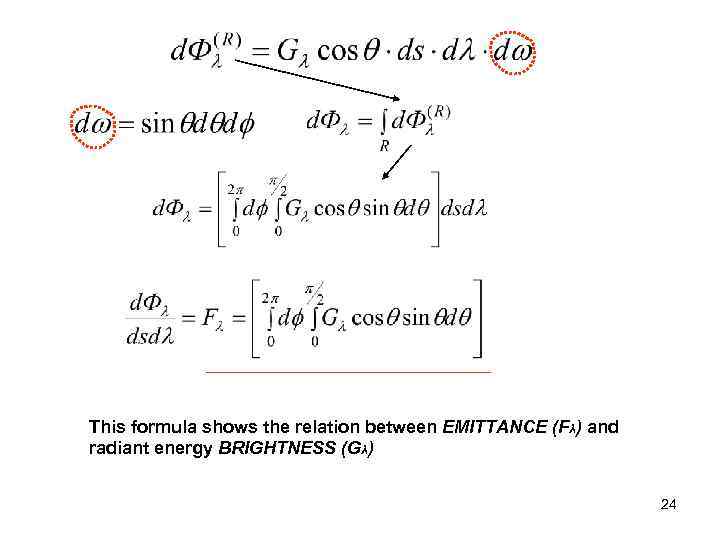
This formula shows the relation between EMITTANCE (Fλ) and radiant energy BRIGHTNESS (Gλ) 24

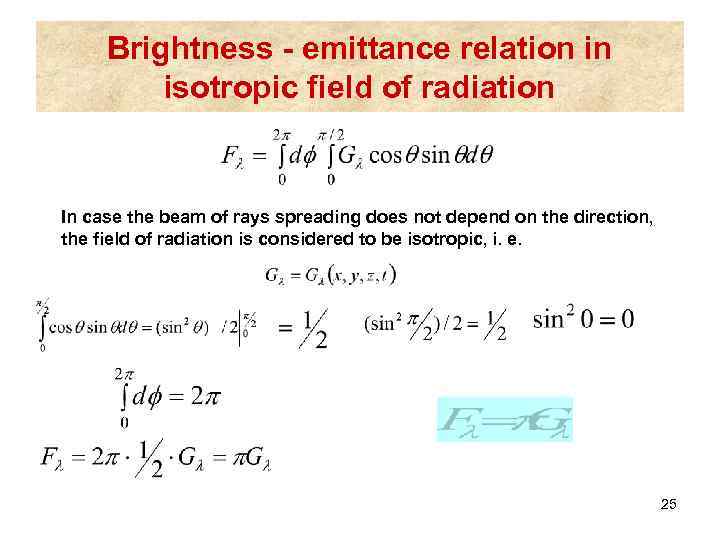
Brightness - emittance relation in isotropic field of radiation In case the beam of rays spreading does not depend on the direction, the field of radiation is considered to be isotropic, i. e. 25

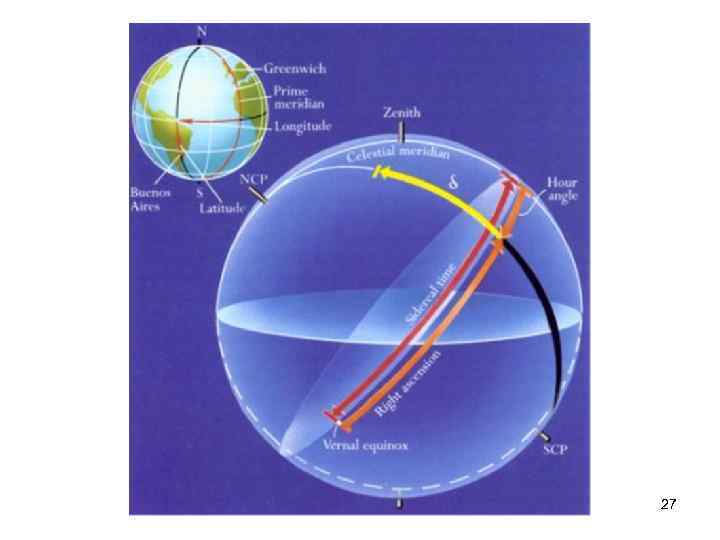
Definitions Hour Angle of the Sun The Solar Hour Angle of the Sun for any local location on the Earth is zero° when the sun is straight overhead, at the zenith, and negative before local solar noon and positive after solar noon. In one 24 -hour period, the Solar Hour Angle changes by 360 degrees (i. e. one revolution). Solar Noon (and Solar Time) Solar Time is based on the motion of the sun around the Earth. The apparent sun's motion, and position in the sky, can vary due to a few things such as: the elliptical orbits of the Earth and Sun, the inclination of the axis of the Earth's rotation, the perturbations of the moon and other planets, and of course, your latitude and longitude of observation. Solar Noon is when the sun is at the highest in the sky, and is defined when the Hour Angle is 0°. Solar Noon is also the midpoint between Sunrise and Sunset. Sun Declination The Declination of the sun is how many degrees North (positive) or South (negative) of the equator that the sun is when viewed from the center of the earth. The range of the declination of the sun ranges from approximately +23. 5° (North) in June to -23. 5° (South) in December. 26

27

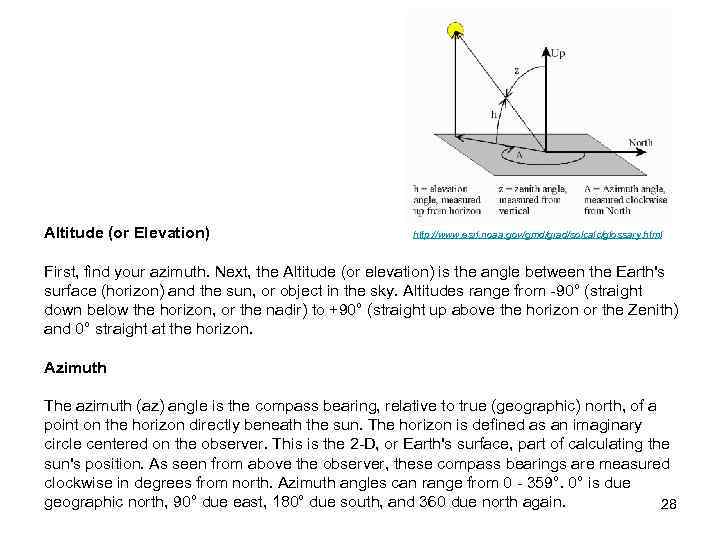
Altitude (or Elevation) http: //www. esrl. noaa. gov/gmd/grad/solcalc/glossary. html First, find your azimuth. Next, the Altitude (or elevation) is the angle between the Earth's surface (horizon) and the sun, or object in the sky. Altitudes range from -90° (straight down below the horizon, or the nadir) to +90° (straight up above the horizon or the Zenith) and 0° straight at the horizon. Azimuth The azimuth (az) angle is the compass bearing, relative to true (geographic) north, of a point on the horizon directly beneath the sun. The horizon is defined as an imaginary circle centered on the observer. This is the 2 -D, or Earth's surface, part of calculating the sun's position. As seen from above the observer, these compass bearings are measured clockwise in degrees from north. Azimuth angles can range from 0 - 359°. 0° is due geographic north, 90° due east, 180° due south, and 360 due north again. 28