7857c89d413d3ce9e5dbef0b80cda0dc.ppt
- Количество слайдов: 29
 Simultaneous Heat and Mass Transfer during Evaporation/Condensation on the Surface of a Stagnant Droplet in the Presence of Inert Admixtures Containing Non-condensable Solvable Gas: Application for the In-cloud Scavenging of Polluted Gases T. Elperin, A. Fominykh and B. Krasovitov Department of Mechanical Engineering The Pearlstone Center for Aeronautical Engineering Studies Ben-Gurion University of the Negev P. O. B. 653, Beer Sheva 84105, ISRAEL
Simultaneous Heat and Mass Transfer during Evaporation/Condensation on the Surface of a Stagnant Droplet in the Presence of Inert Admixtures Containing Non-condensable Solvable Gas: Application for the In-cloud Scavenging of Polluted Gases T. Elperin, A. Fominykh and B. Krasovitov Department of Mechanical Engineering The Pearlstone Center for Aeronautical Engineering Studies Ben-Gurion University of the Negev P. O. B. 653, Beer Sheva 84105, ISRAEL
 Laboratory of Turbulent Multiphase Flows http: //www. bgu. ac. il/me/laboratories/tmf/turbulent. Multiphase. Flow. html Head - Professor Tov Elperin People Dr. Alexander Eidelman Dr. Andrew Fominykh Mr. Ilia Golubev Dr. Nathan Kleeorin Dr. Boris Krasovitov Mr. Alexander Krein Mr. Andrew Markovich Dr. Igor Rogachevskii Mr. Itsik Sapir-Katiraie
Laboratory of Turbulent Multiphase Flows http: //www. bgu. ac. il/me/laboratories/tmf/turbulent. Multiphase. Flow. html Head - Professor Tov Elperin People Dr. Alexander Eidelman Dr. Andrew Fominykh Mr. Ilia Golubev Dr. Nathan Kleeorin Dr. Boris Krasovitov Mr. Alexander Krein Mr. Andrew Markovich Dr. Igor Rogachevskii Mr. Itsik Sapir-Katiraie
 Outline of the presentation Motivation and goals Description of the model Gas absorption by stagnant evaporating/growing droplets Gas absorption by moving droplets Results and discussion: Application for the In -cloud Scavenging of Polluted Gases Conclusions
Outline of the presentation Motivation and goals Description of the model Gas absorption by stagnant evaporating/growing droplets Gas absorption by moving droplets Results and discussion: Application for the In -cloud Scavenging of Polluted Gases Conclusions
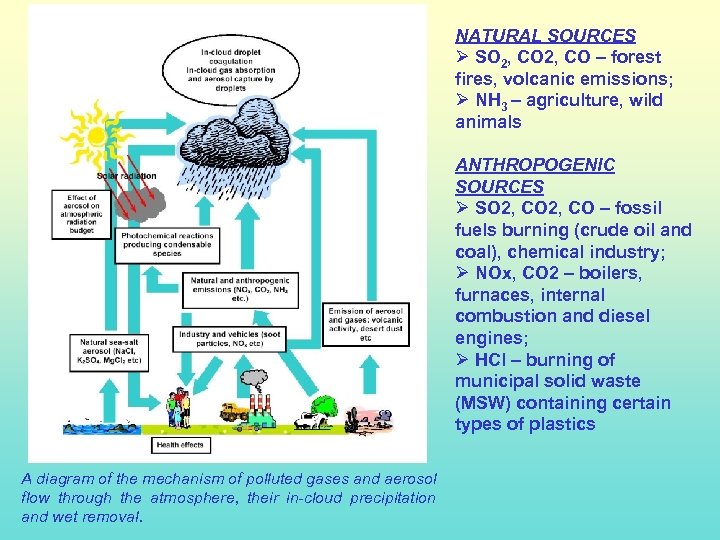 NATURAL SOURCES Ø SO 2, CO – forest fires, volcanic emissions; Ø NH 3 – agriculture, wild animals ANTHROPOGENIC SOURCES Ø SO 2, CO – fossil fuels burning (crude oil and coal), chemical industry; Ø NOx, CO 2 – boilers, furnaces, internal combustion and diesel engines; Ø HCl – burning of municipal solid waste (MSW) containing certain types of plastics A diagram of the mechanism of polluted gases and aerosol flow through the atmosphere, their in-cloud precipitation and wet removal.
NATURAL SOURCES Ø SO 2, CO – forest fires, volcanic emissions; Ø NH 3 – agriculture, wild animals ANTHROPOGENIC SOURCES Ø SO 2, CO – fossil fuels burning (crude oil and coal), chemical industry; Ø NOx, CO 2 – boilers, furnaces, internal combustion and diesel engines; Ø HCl – burning of municipal solid waste (MSW) containing certain types of plastics A diagram of the mechanism of polluted gases and aerosol flow through the atmosphere, their in-cloud precipitation and wet removal.
 Gas absorption by stagnant droplets: Scientific background Dispersed-phase controlled isothermal absorption of a pure gas by stagnant liquid droplet (see e. g. , Newman A. B. , 1931); Gas absorption in the presence of inert admixtures (see e. g. , Plocker U. J. , Schmidt-Traub H. , 1972); Effect of vapor condensation at the surface of stagnant droplets on the rate of mass transfer during gas absorption by growing droplets uniform temperature distribution in both phases was assumed (see e. g. , Karamchandani, P. , Ray, A. K. and Das, N. , 1984) liquid-phase controlled mass transfer during absorption was investigated when the system consisted of liquid droplet, its vapor and solvable gas (see e. g. , Ray A. K. , Huckaby J. L. and Shah T. , 1987, 1989) Simultaneous heat and mass transfer during evaporation/condensation on the surface of a stagnant droplet in the presence of inert admixtures containing non-condensable solvable gas (Elperin T. , Fominykh A. and Krasovitov B. , 2005)
Gas absorption by stagnant droplets: Scientific background Dispersed-phase controlled isothermal absorption of a pure gas by stagnant liquid droplet (see e. g. , Newman A. B. , 1931); Gas absorption in the presence of inert admixtures (see e. g. , Plocker U. J. , Schmidt-Traub H. , 1972); Effect of vapor condensation at the surface of stagnant droplets on the rate of mass transfer during gas absorption by growing droplets uniform temperature distribution in both phases was assumed (see e. g. , Karamchandani, P. , Ray, A. K. and Das, N. , 1984) liquid-phase controlled mass transfer during absorption was investigated when the system consisted of liquid droplet, its vapor and solvable gas (see e. g. , Ray A. K. , Huckaby J. L. and Shah T. , 1987, 1989) Simultaneous heat and mass transfer during evaporation/condensation on the surface of a stagnant droplet in the presence of inert admixtures containing non-condensable solvable gas (Elperin T. , Fominykh A. and Krasovitov B. , 2005)
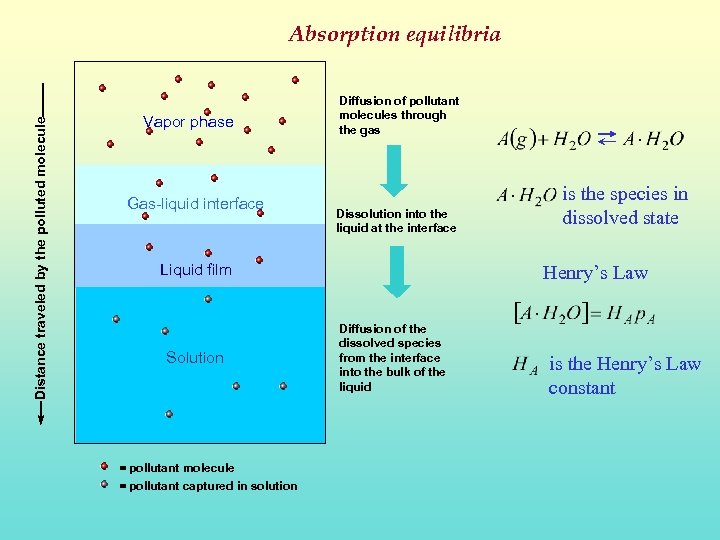 Distance traveled by the polluted molecule Absorption equilibria Vapor phase Gas-liquid interface Diffusion of pollutant molecules through the gas Dissolution into the liquid at the interface Liquid film Solution = pollutant molecule = pollutant captured in solution is the species in dissolved state Henry’s Law Diffusion of the dissolved species from the interface into the bulk of the liquid is the Henry’s Law constant
Distance traveled by the polluted molecule Absorption equilibria Vapor phase Gas-liquid interface Diffusion of pollutant molecules through the gas Dissolution into the liquid at the interface Liquid film Solution = pollutant molecule = pollutant captured in solution is the species in dissolved state Henry’s Law Diffusion of the dissolved species from the interface into the bulk of the liquid is the Henry’s Law constant
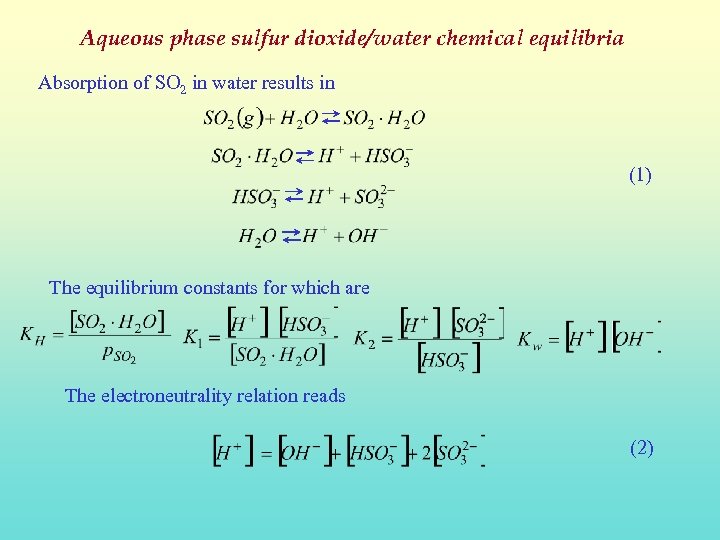 Aqueous phase sulfur dioxide/water chemical equilibria Absorption of SO 2 in water results in (1) The equilibrium constants for which are The electroneutrality relation reads (2)
Aqueous phase sulfur dioxide/water chemical equilibria Absorption of SO 2 in water results in (1) The equilibrium constants for which are The electroneutrality relation reads (2)
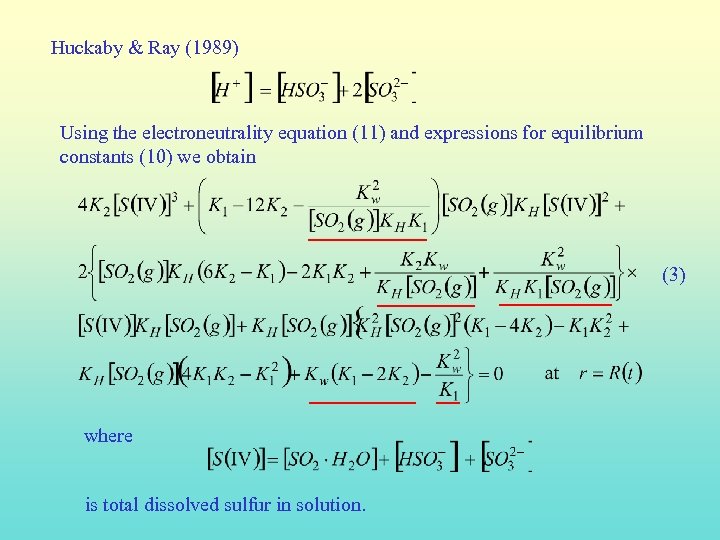 Huckaby & Ray (1989) Using the electroneutrality equation (11) and expressions for equilibrium constants (10) we obtain (3) where is total dissolved sulfur in solution.
Huckaby & Ray (1989) Using the electroneutrality equation (11) and expressions for equilibrium constants (10) we obtain (3) where is total dissolved sulfur in solution.
 Gas absorption by stagnant droplet Description of the model Governing equations 1. gaseous phase r > R (t) Droplet Far field (4) Gaseous phase Z (5) q (6) R Gas-liquid interface Y 2. liquid phase 0 < r < R (t) (7) (8) X j In Eqs. (5)
Gas absorption by stagnant droplet Description of the model Governing equations 1. gaseous phase r > R (t) Droplet Far field (4) Gaseous phase Z (5) q (6) R Gas-liquid interface Y 2. liquid phase 0 < r < R (t) (7) (8) X j In Eqs. (5)
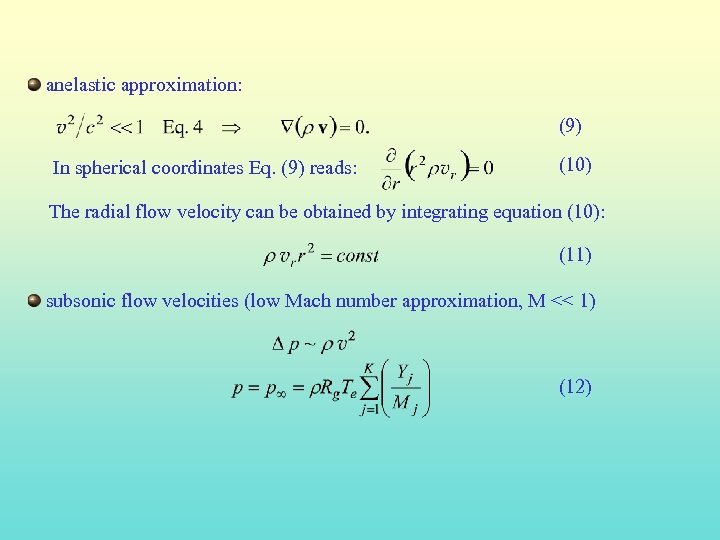 anelastic approximation: (9) In spherical coordinates Eq. (9) reads: (10) The radial flow velocity can be obtained by integrating equation (10): (11) subsonic flow velocities (low Mach number approximation, M << 1) (12)
anelastic approximation: (9) In spherical coordinates Eq. (9) reads: (10) The radial flow velocity can be obtained by integrating equation (10): (11) subsonic flow velocities (low Mach number approximation, M << 1) (12)
 Stefan velocity and droplet vaporization rate The continuity condition for the radial flux of the absorbate at the droplet surface reads: (13) Other non-solvable components of the inert admixtures are not absorbed in the liquid (14) Taking into account this condition and using Eq. (10) we can obtain the expression for Stefan velocity: (15) where subscript “ 1” denotes water vapor species
Stefan velocity and droplet vaporization rate The continuity condition for the radial flux of the absorbate at the droplet surface reads: (13) Other non-solvable components of the inert admixtures are not absorbed in the liquid (14) Taking into account this condition and using Eq. (10) we can obtain the expression for Stefan velocity: (15) where subscript “ 1” denotes water vapor species
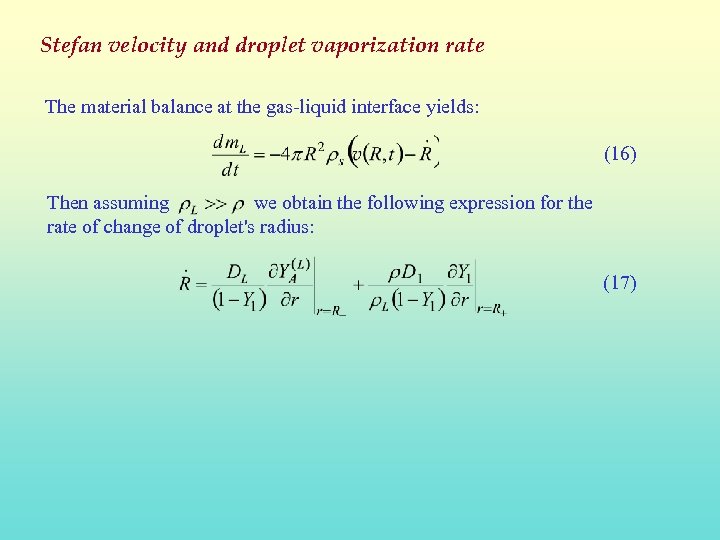 Stefan velocity and droplet vaporization rate The material balance at the gas-liquid interface yields: (16) Then assuming we obtain the following expression for the rate of change of droplet's radius: (17)
Stefan velocity and droplet vaporization rate The material balance at the gas-liquid interface yields: (16) Then assuming we obtain the following expression for the rate of change of droplet's radius: (17)
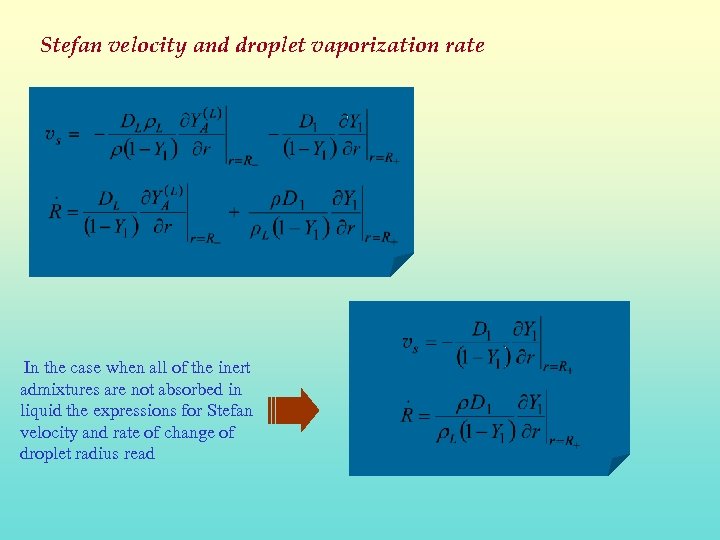 Stefan velocity and droplet vaporization rate In the case when all of the inert admixtures are not absorbed in liquid the expressions for Stefan velocity and rate of change of droplet radius read
Stefan velocity and droplet vaporization rate In the case when all of the inert admixtures are not absorbed in liquid the expressions for Stefan velocity and rate of change of droplet radius read
 Initial and boundary conditions The initial conditions for the system of equations (1)–(5) read: At t = 0, (18) At the droplet surface the continuity conditions for the radial flux of nonsolvable gaseous species yield: (19) For the absorbate boundary condition reads: (20) The droplet temperature can be found from the following equation: (21)
Initial and boundary conditions The initial conditions for the system of equations (1)–(5) read: At t = 0, (18) At the droplet surface the continuity conditions for the radial flux of nonsolvable gaseous species yield: (19) For the absorbate boundary condition reads: (20) The droplet temperature can be found from the following equation: (21)
 Initial and boundary conditions The equilibrium between solvable gaseous and dissolved in liquid species can be expressed using the Henry's law (22) At the gas-liquid interface (23) In the center of the droplet symmetry conditions yields: (24) At and the ‘soft’ boundary conditions at infinity are imposed (25)
Initial and boundary conditions The equilibrium between solvable gaseous and dissolved in liquid species can be expressed using the Henry's law (22) At the gas-liquid interface (23) In the center of the droplet symmetry conditions yields: (24) At and the ‘soft’ boundary conditions at infinity are imposed (25)
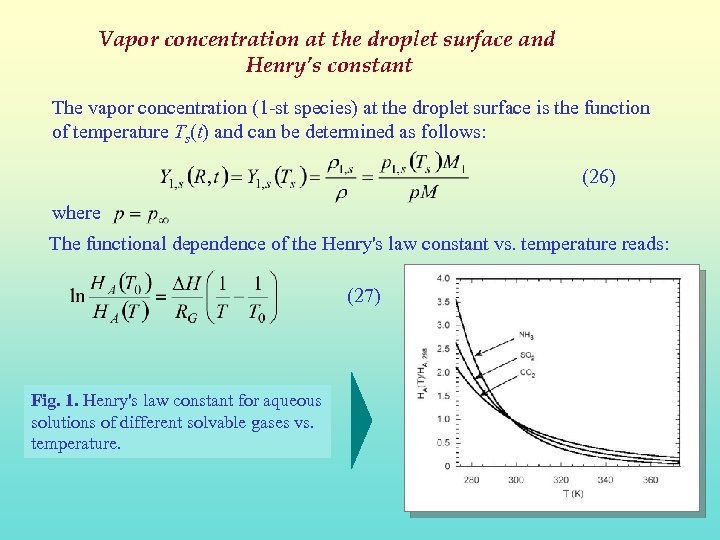 Vapor concentration at the droplet surface and Henry’s constant The vapor concentration (1 -st species) at the droplet surface is the function of temperature Ts(t) and can be determined as follows: (26) where The functional dependence of the Henry's law constant vs. temperature reads: (27) Fig. 1. Henry's law constant for aqueous solutions of different solvable gases vs. temperature.
Vapor concentration at the droplet surface and Henry’s constant The vapor concentration (1 -st species) at the droplet surface is the function of temperature Ts(t) and can be determined as follows: (26) where The functional dependence of the Henry's law constant vs. temperature reads: (27) Fig. 1. Henry's law constant for aqueous solutions of different solvable gases vs. temperature.
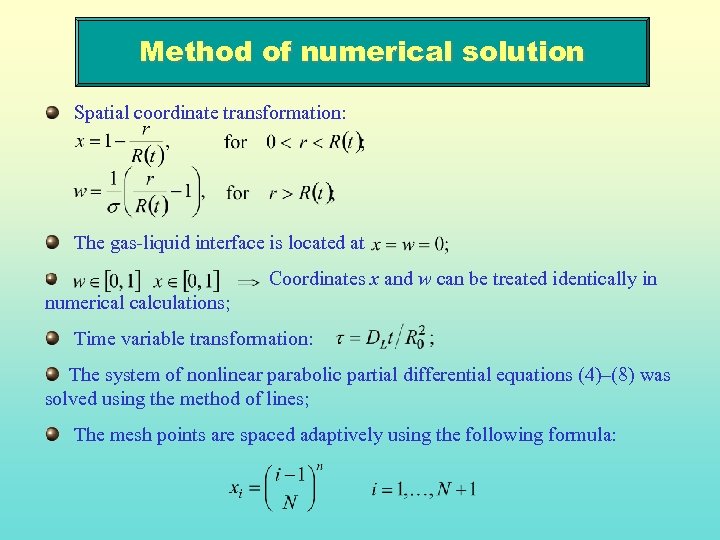 Method of numerical solution Spatial coordinate transformation: The gas-liquid interface is located at Coordinates x and w can be treated identically in numerical calculations; Time variable transformation: The system of nonlinear parabolic partial differential equations (4)–(8) was solved using the method of lines; The mesh points are spaced adaptively using the following formula:
Method of numerical solution Spatial coordinate transformation: The gas-liquid interface is located at Coordinates x and w can be treated identically in numerical calculations; Time variable transformation: The system of nonlinear parabolic partial differential equations (4)–(8) was solved using the method of lines; The mesh points are spaced adaptively using the following formula:
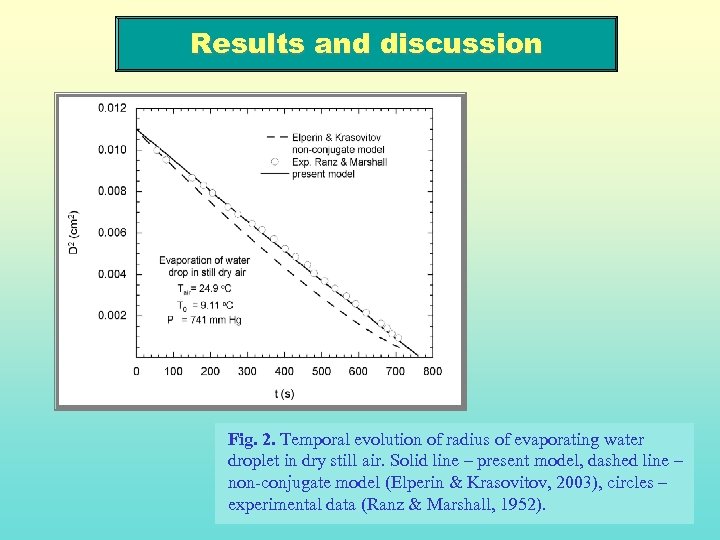 Results and discussion Fig. 2. Temporal evolution of radius of evaporating water droplet in dry still air. Solid line – present model, dashed line – non-conjugate model (Elperin & Krasovitov, 2003), circles – experimental data (Ranz & Marshall, 1952).
Results and discussion Fig. 2. Temporal evolution of radius of evaporating water droplet in dry still air. Solid line – present model, dashed line – non-conjugate model (Elperin & Krasovitov, 2003), circles – experimental data (Ranz & Marshall, 1952).
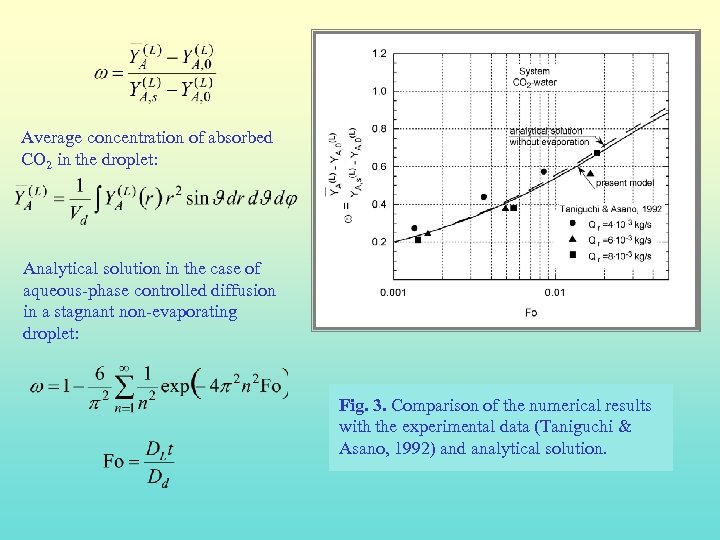 Average concentration of absorbed CO 2 in the droplet: Analytical solution in the case of aqueous-phase controlled diffusion in a stagnant non-evaporating droplet: Fig. 3. Comparison of the numerical results with the experimental data (Taniguchi & Asano, 1992) and analytical solution.
Average concentration of absorbed CO 2 in the droplet: Analytical solution in the case of aqueous-phase controlled diffusion in a stagnant non-evaporating droplet: Fig. 3. Comparison of the numerical results with the experimental data (Taniguchi & Asano, 1992) and analytical solution.
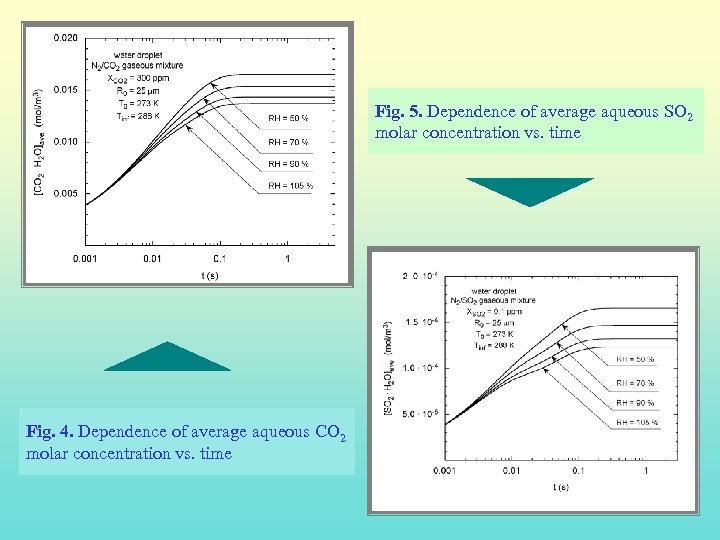 Fig. 5. Dependence of average aqueous SO 2 molar concentration vs. time Fig. 4. Dependence of average aqueous CO 2 molar concentration vs. time
Fig. 5. Dependence of average aqueous SO 2 molar concentration vs. time Fig. 4. Dependence of average aqueous CO 2 molar concentration vs. time
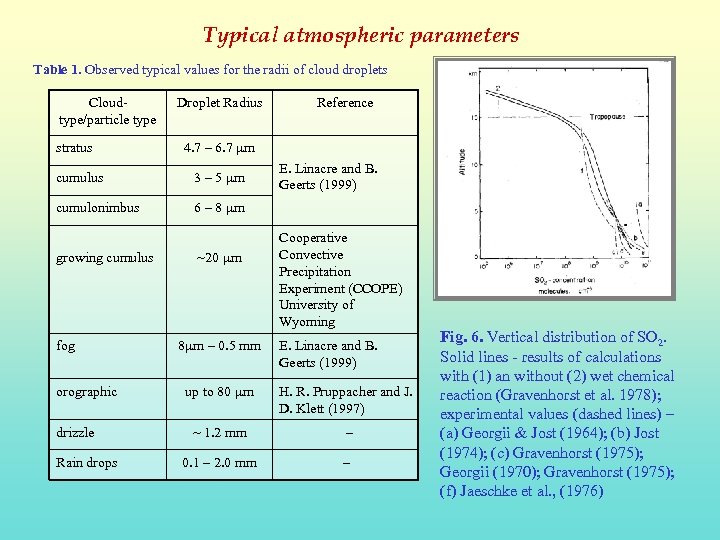 Typical atmospheric parameters Table 1. Observed typical values for the radii of cloud droplets Cloudtype/particle type stratus Droplet Radius Reference 4. 7 – 6. 7 mm cumulus 3 – 5 mm cumulonimbus E. Linacre and B. Geerts (1999) 6 – 8 mm growing cumulus fog orographic drizzle Rain drops ~20 mm 8 mm – 0. 5 mm Cooperative Convective Precipitation Experiment (CCOPE) University of Wyoming E. Linacre and B. Geerts (1999) up to 80 mm H. R. Pruppacher and J. D. Klett (1997) ~ 1. 2 mm – 0. 1 – 2. 0 mm – Fig. 6. Vertical distribution of SO 2. Solid lines - results of calculations with (1) an without (2) wet chemical reaction (Gravenhorst et al. 1978); experimental values (dashed lines) – (a) Georgii & Jost (1964); (b) Jost (1974); (c) Gravenhorst (1975); Georgii (1970); Gravenhorst (1975); (f) Jaeschke et al. , (1976)
Typical atmospheric parameters Table 1. Observed typical values for the radii of cloud droplets Cloudtype/particle type stratus Droplet Radius Reference 4. 7 – 6. 7 mm cumulus 3 – 5 mm cumulonimbus E. Linacre and B. Geerts (1999) 6 – 8 mm growing cumulus fog orographic drizzle Rain drops ~20 mm 8 mm – 0. 5 mm Cooperative Convective Precipitation Experiment (CCOPE) University of Wyoming E. Linacre and B. Geerts (1999) up to 80 mm H. R. Pruppacher and J. D. Klett (1997) ~ 1. 2 mm – 0. 1 – 2. 0 mm – Fig. 6. Vertical distribution of SO 2. Solid lines - results of calculations with (1) an without (2) wet chemical reaction (Gravenhorst et al. 1978); experimental values (dashed lines) – (a) Georgii & Jost (1964); (b) Jost (1974); (c) Gravenhorst (1975); Georgii (1970); Gravenhorst (1975); (f) Jaeschke et al. , (1976)
 Fig. 7. Dependence of dimensionless average aqueous CO 2 concentration vs. time (RH = 0%). Fig. 8. Dependence of dimensionless average aqueous SO 2 concentration vs. time (RH = 0%). Fig. 9. Dependence of dimensionless average aqueous CO 2 concentration vs. time (R 0 = 25 mm).
Fig. 7. Dependence of dimensionless average aqueous CO 2 concentration vs. time (RH = 0%). Fig. 8. Dependence of dimensionless average aqueous SO 2 concentration vs. time (RH = 0%). Fig. 9. Dependence of dimensionless average aqueous CO 2 concentration vs. time (R 0 = 25 mm).
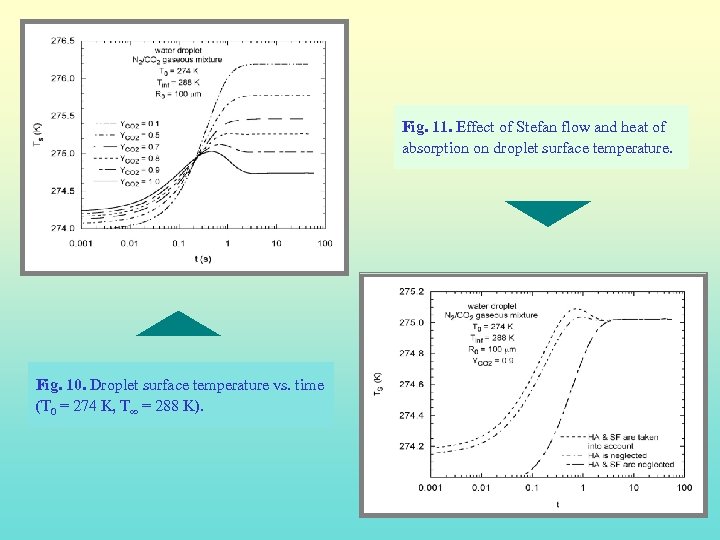 Fig. 11. Effect of Stefan flow and heat of absorption on droplet surface temperature. Fig. 10. Droplet surface temperature vs. time (T 0 = 274 K, T∞ = 288 K).
Fig. 11. Effect of Stefan flow and heat of absorption on droplet surface temperature. Fig. 10. Droplet surface temperature vs. time (T 0 = 274 K, T∞ = 288 K).
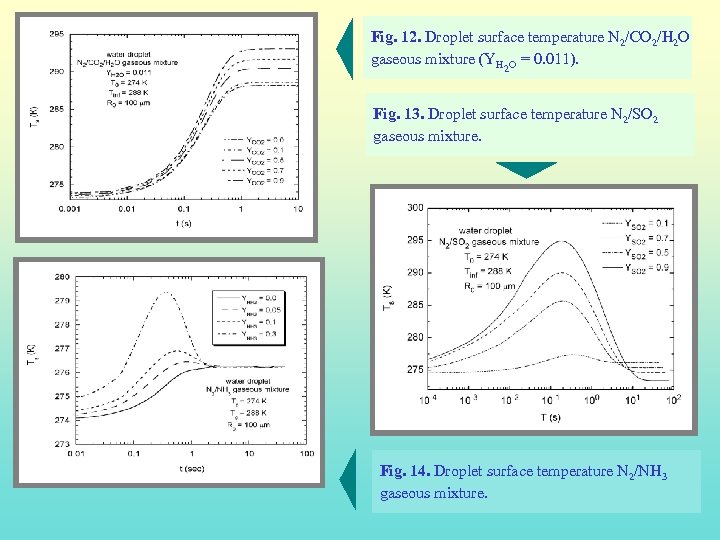 Fig. 12. Droplet surface temperature N 2/CO 2/H 2 O gaseous mixture (YH O = 0. 011). 2 Fig. 13. Droplet surface temperature N 2/SO 2 gaseous mixture. Fig. 14. Droplet surface temperature N 2/NH 3 gaseous mixture.
Fig. 12. Droplet surface temperature N 2/CO 2/H 2 O gaseous mixture (YH O = 0. 011). 2 Fig. 13. Droplet surface temperature N 2/SO 2 gaseous mixture. Fig. 14. Droplet surface temperature N 2/NH 3 gaseous mixture.
 Fig. 15. Dimensionless droplet radius vs. time R 0 = 25 mm, XSO 2 = 0. 1 ppm. Fig. 17. Dimensionless droplet radius vs. time N 2/CO 2/H 2 O gaseous mixture YH 2 O = 0. 011. Fig. 16. Dimensionless droplet radius vs. time R 0 = 100 mm, N 2/CO 2 gaseous mixture. Fig. 18. Dimensionless droplet radius vs. time N 2/CO 2/H 2 O gaseous mixture.
Fig. 15. Dimensionless droplet radius vs. time R 0 = 25 mm, XSO 2 = 0. 1 ppm. Fig. 17. Dimensionless droplet radius vs. time N 2/CO 2/H 2 O gaseous mixture YH 2 O = 0. 011. Fig. 16. Dimensionless droplet radius vs. time R 0 = 100 mm, N 2/CO 2 gaseous mixture. Fig. 18. Dimensionless droplet radius vs. time N 2/CO 2/H 2 O gaseous mixture.
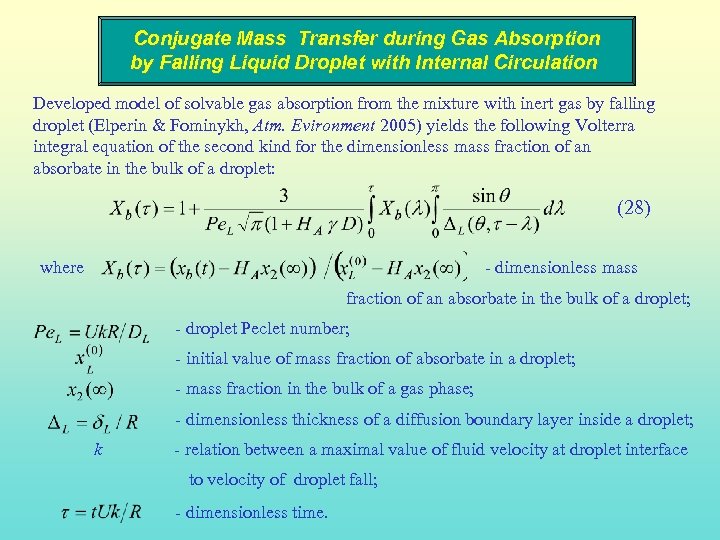 Conjugate Mass Transfer during Gas Absorption by Falling Liquid Droplet with Internal Circulation Developed model of solvable gas absorption from the mixture with inert gas by falling droplet (Elperin & Fominykh, Atm. Evironment 2005) yields the following Volterra integral equation of the second kind for the dimensionless mass fraction of an absorbate in the bulk of a droplet: (28) where - dimensionless mass fraction of an absorbate in the bulk of a droplet; - droplet Peclet number; - initial value of mass fraction of absorbate in a droplet; - mass fraction in the bulk of a gas phase; - dimensionless thickness of a diffusion boundary layer inside a droplet; k - relation between a maximal value of fluid velocity at droplet interface to velocity of droplet fall; - dimensionless time.
Conjugate Mass Transfer during Gas Absorption by Falling Liquid Droplet with Internal Circulation Developed model of solvable gas absorption from the mixture with inert gas by falling droplet (Elperin & Fominykh, Atm. Evironment 2005) yields the following Volterra integral equation of the second kind for the dimensionless mass fraction of an absorbate in the bulk of a droplet: (28) where - dimensionless mass fraction of an absorbate in the bulk of a droplet; - droplet Peclet number; - initial value of mass fraction of absorbate in a droplet; - mass fraction in the bulk of a gas phase; - dimensionless thickness of a diffusion boundary layer inside a droplet; k - relation between a maximal value of fluid velocity at droplet interface to velocity of droplet fall; - dimensionless time.
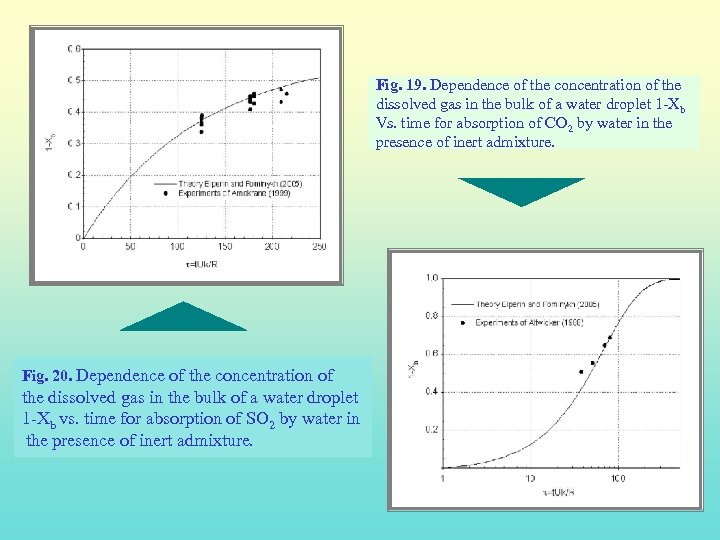 Fig. 19. Dependence of the concentration of the dissolved gas in the bulk of a water droplet 1 -Xb Vs. time for absorption of CO 2 by water in the presence of inert admixture. Fig. 20. Dependence of the concentration of the dissolved gas in the bulk of a water droplet 1 -Xb vs. time for absorption of SO 2 by water in the presence of inert admixture.
Fig. 19. Dependence of the concentration of the dissolved gas in the bulk of a water droplet 1 -Xb Vs. time for absorption of CO 2 by water in the presence of inert admixture. Fig. 20. Dependence of the concentration of the dissolved gas in the bulk of a water droplet 1 -Xb vs. time for absorption of SO 2 by water in the presence of inert admixture.
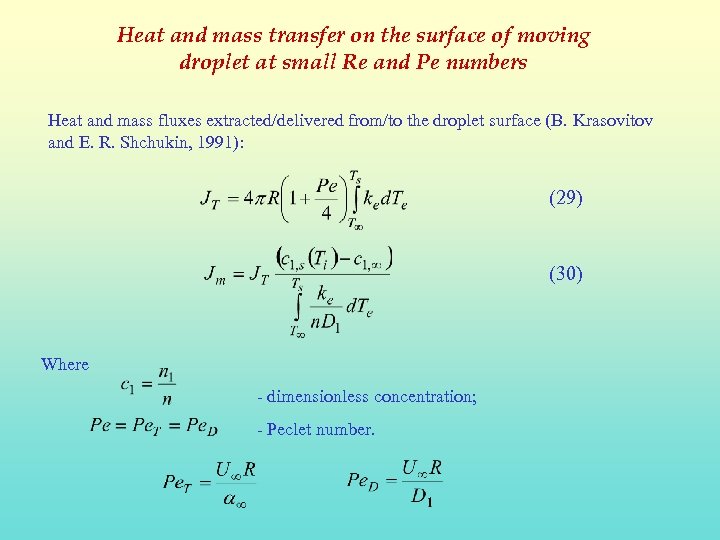 Heat and mass transfer on the surface of moving droplet at small Re and Pe numbers Heat and mass fluxes extracted/delivered from/to the droplet surface (B. Krasovitov and E. R. Shchukin, 1991): (29) (30) Where - dimensionless concentration; - Peclet number.
Heat and mass transfer on the surface of moving droplet at small Re and Pe numbers Heat and mass fluxes extracted/delivered from/to the droplet surface (B. Krasovitov and E. R. Shchukin, 1991): (29) (30) Where - dimensionless concentration; - Peclet number.
 Conclusions In this study we developed a model that takes into account the simultaneous effect of gas absorption and evaporation (condensation) for a system consisting of liquid droplet - vapor of liquid droplet - inert noncondensable and nonabsorbable gasnoncondensable solvable gas. Droplet evaporation rate, droplet temperature, interfacial absorbate concentration and the rate of mass transfer during gas absorption are highly interdependent. Thermal effect of gas dissolution in a droplet and Stefan flow increases droplet temperature and mass flux of a volatile species from the droplet temperature at the initial stage of evaporation. The obtained results show good agreement with the experimental data. The performed analysis of gas absorption by liquid droplets accompanied by droplets evaporation and vapor condensation on the surface of liquid droplets can be used in calculations of scavenging of hazardous gases in atmosphere by rain, atmospheric cloud evolution.
Conclusions In this study we developed a model that takes into account the simultaneous effect of gas absorption and evaporation (condensation) for a system consisting of liquid droplet - vapor of liquid droplet - inert noncondensable and nonabsorbable gasnoncondensable solvable gas. Droplet evaporation rate, droplet temperature, interfacial absorbate concentration and the rate of mass transfer during gas absorption are highly interdependent. Thermal effect of gas dissolution in a droplet and Stefan flow increases droplet temperature and mass flux of a volatile species from the droplet temperature at the initial stage of evaporation. The obtained results show good agreement with the experimental data. The performed analysis of gas absorption by liquid droplets accompanied by droplets evaporation and vapor condensation on the surface of liquid droplets can be used in calculations of scavenging of hazardous gases in atmosphere by rain, atmospheric cloud evolution.


