 Signs of equality of triangles
Signs of equality of triangles
 • Type of lesson: study and the initial consolidation of new knowledge. • Lesson Objectives. • Enter the concept of theorem and proof of theorem; • To prove signs of equality of triangles; • To learn to solve problems in the application of the signs of equality of triangles.
• Type of lesson: study and the initial consolidation of new knowledge. • Lesson Objectives. • Enter the concept of theorem and proof of theorem; • To prove signs of equality of triangles; • To learn to solve problems in the application of the signs of equality of triangles.
 • Lesson Plan : • • • Organizing time. Signs of equality of triangles, and the proof of theorem. Questions Give homework. Summing up the results of the lesson.
• Lesson Plan : • • • Organizing time. Signs of equality of triangles, and the proof of theorem. Questions Give homework. Summing up the results of the lesson.
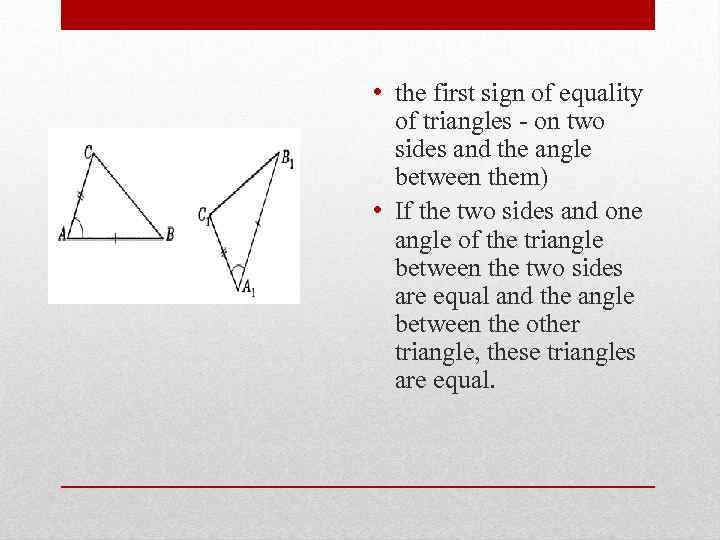 • the first sign of equality of triangles - on two sides and the angle between them) • If the two sides and one angle of the triangle between the two sides are equal and the angle between the other triangle, these triangles are equal.
• the first sign of equality of triangles - on two sides and the angle between them) • If the two sides and one angle of the triangle between the two sides are equal and the angle between the other triangle, these triangles are equal.
 the second sign of the equality of triangles • If the side and adjacent to it the angles of a triangle are equal respectively to the side of and adjacent to it the other corners of the triangle, these triangles are equal.
the second sign of the equality of triangles • If the side and adjacent to it the angles of a triangle are equal respectively to the side of and adjacent to it the other corners of the triangle, these triangles are equal.
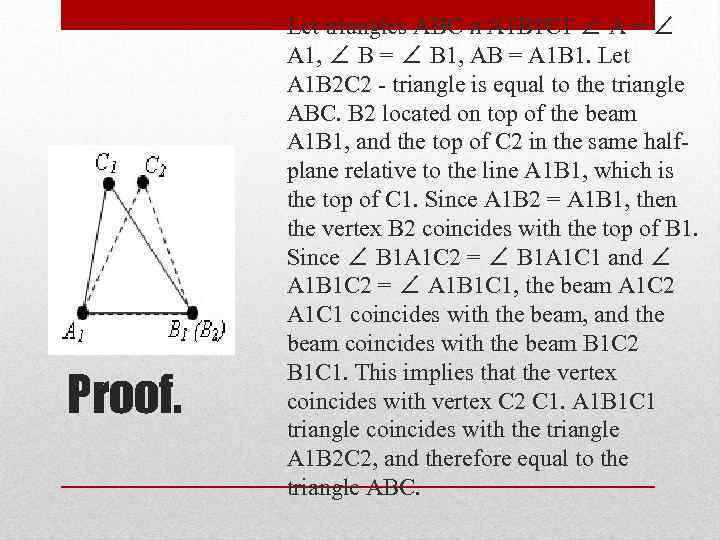 Proof. • Let triangles ABC и A 1 B 1 C 1 ∠ A = ∠ A 1, ∠ B = ∠ B 1, AB = A 1 B 1. Let A 1 B 2 C 2 - triangle is equal to the triangle ABC. B 2 located on top of the beam A 1 B 1, and the top of C 2 in the same halfplane relative to the line A 1 B 1, which is the top of C 1. Since A 1 B 2 = A 1 B 1, then the vertex B 2 coincides with the top of B 1. Since ∠ B 1 A 1 C 2 = ∠ B 1 A 1 C 1 and ∠ A 1 B 1 C 2 = ∠ A 1 B 1 C 1, the beam A 1 C 2 A 1 C 1 coincides with the beam, and the beam coincides with the beam B 1 C 2 B 1 C 1. This implies that the vertex coincides with vertex C 2 C 1. A 1 B 1 C 1 triangle coincides with the triangle A 1 B 2 C 2, and therefore equal to the triangle ABC.
Proof. • Let triangles ABC и A 1 B 1 C 1 ∠ A = ∠ A 1, ∠ B = ∠ B 1, AB = A 1 B 1. Let A 1 B 2 C 2 - triangle is equal to the triangle ABC. B 2 located on top of the beam A 1 B 1, and the top of C 2 in the same halfplane relative to the line A 1 B 1, which is the top of C 1. Since A 1 B 2 = A 1 B 1, then the vertex B 2 coincides with the top of B 1. Since ∠ B 1 A 1 C 2 = ∠ B 1 A 1 C 1 and ∠ A 1 B 1 C 2 = ∠ A 1 B 1 C 1, the beam A 1 C 2 A 1 C 1 coincides with the beam, and the beam coincides with the beam B 1 C 2 B 1 C 1. This implies that the vertex coincides with vertex C 2 C 1. A 1 B 1 C 1 triangle coincides with the triangle A 1 B 2 C 2, and therefore equal to the triangle ABC.
 The third sign of equality of triangles • Theorem. If, then these triangles are equal to three sides of a triangle are equal to the other three sides of the triangle. • Proof. Consider triangles ABC and A 1 B 1 C 1 in which AB = A 1 B 1, BC = B 1 C 1, CA = C 1 A 1 (Fig. 84), and prove that the triangles are equal.
The third sign of equality of triangles • Theorem. If, then these triangles are equal to three sides of a triangle are equal to the other three sides of the triangle. • Proof. Consider triangles ABC and A 1 B 1 C 1 in which AB = A 1 B 1, BC = B 1 C 1, CA = C 1 A 1 (Fig. 84), and prove that the triangles are equal.
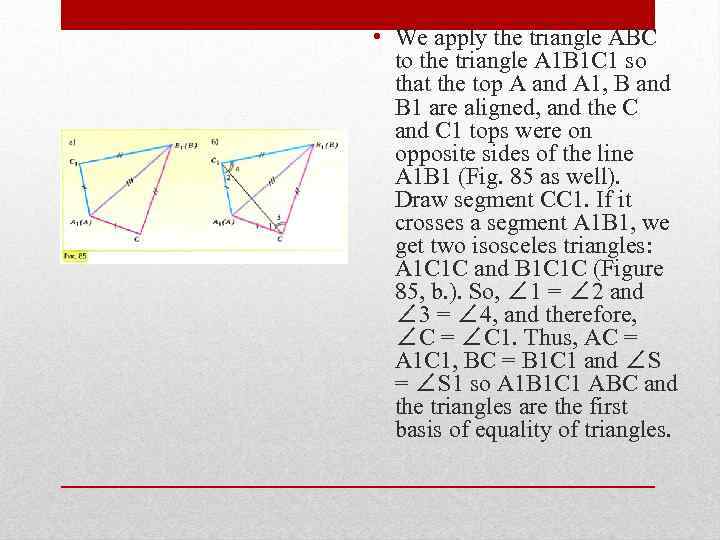 • We apply the triangle ABC to the triangle A 1 B 1 C 1 so that the top A and A 1, B and B 1 are aligned, and the C and C 1 tops were on opposite sides of the line A 1 B 1 (Fig. 85 as well). Draw segment CC 1. If it crosses a segment A 1 B 1, we get two isosceles triangles: A 1 C 1 C and B 1 C 1 C (Figure 85, b. ). So, ∠ 1 = ∠ 2 and ∠ 3 = ∠ 4, and therefore, ∠C = ∠C 1. Thus, AC = A 1 C 1, BC = B 1 C 1 and ∠S = ∠S 1 so A 1 B 1 C 1 ABC and the triangles are the first basis of equality of triangles.
• We apply the triangle ABC to the triangle A 1 B 1 C 1 so that the top A and A 1, B and B 1 are aligned, and the C and C 1 tops were on opposite sides of the line A 1 B 1 (Fig. 85 as well). Draw segment CC 1. If it crosses a segment A 1 B 1, we get two isosceles triangles: A 1 C 1 C and B 1 C 1 C (Figure 85, b. ). So, ∠ 1 = ∠ 2 and ∠ 3 = ∠ 4, and therefore, ∠C = ∠C 1. Thus, AC = A 1 C 1, BC = B 1 C 1 and ∠S = ∠S 1 so A 1 B 1 C 1 ABC and the triangles are the first basis of equality of triangles.
 Questions • • • What is the first sign of the equality of triangles? What is the second sign of the equality of triangles? What is the third sign of the equality of triangles? What are the symptoms? What is a "sign of equality of triangles"?
Questions • • • What is the first sign of the equality of triangles? What is the second sign of the equality of triangles? What is the third sign of the equality of triangles? What are the symptoms? What is a "sign of equality of triangles"?