Short- and long-ranged Coulomb interactions in models for

Short- and long-ranged Coulomb interactions in models for ionic solutions and water John D. Weeks Institute for Physical Science and Technology and Department of Chemistry and Biochemistry University of Maryland Water Jocelyn Rodgers Polar Molecular Liquids Zhonghan Hu

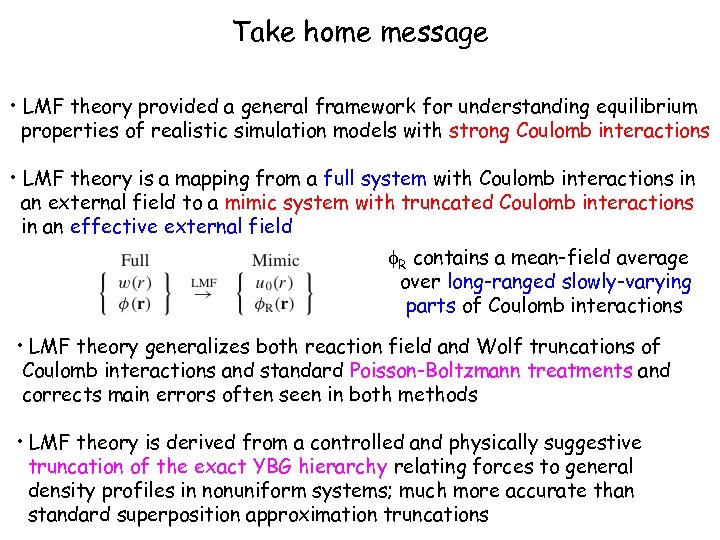
Take home message • LMF theory provided a general framework for understanding equilibrium properties of realistic simulation models with strong Coulomb interactions • LMF theory is a mapping from a full system with Coulomb interactions in an external field to a mimic system with truncated Coulomb interactions in an effective external field R contains a mean-field average over long-ranged slowly-varying parts of Coulomb interactions • LMF theory generalizes both reaction field and Wolf truncations of Coulomb interactions and standard Poisson-Boltzmann treatments and corrects main errors often seen in both methods • LMF theory is derived from a controlled and physically suggestive truncation of the exact YBG hierarchy relating forces to general density profiles in nonuniform systems; much more accurate than standard superposition approximation truncations

Coulomb interactions in molecular simulation models Molecular models: strong Coulomb interactions at short distances compete with other strong intermolecular interactions -- LJ cores, covalent bonds … HC CT YC YN • Want total force on molecular charged site at r and not just Coulomb force on infinitesimal test charge considered in classical electrostatics • short-ranged Coulomb forces dominate wide class of interesting Strong phenomena: H-bonds in water, effective attraction between like-charged walls, ion pairing and chain formation near ionic fluid critical point … Simplest idea: Truncate Coulomb interactions and hope for the best! Cf. Ion reaction field methods (Hummer), Wolf truncations, Force-matching truncations (Voth)

Truncation captures local liquid structure in uniform LJ fluid Map from long to short in uniform LJ system w(r) = u 0(r) + u 1(r) Attractive forces cancel w(r) u 0(r) u 1(r) 1 Soft-sphere u 0(r) accurate everywhere Hard-sphere ud(r) accurate except in first peak near contact J. D. Weeks, D. Chandler, and H. C. Andersen, J. Chem. Phys. 54, 5237 (1971).

Classical water models use point charges to describe both short-ranged H-bonds and long-ranged dipolar forces Extended Simple Point Charge (SPC/E) Model Long range of Coulomb forces causes problems LJ= 3. 166 A q. H = 0. 424 l. OH=1 A H-bonds in SPC/E water result from frustrated ion pairing Properly truncated Coulomb interactions can describe local H-bonds well but not longranged dipolar forces Max g. OO =2. 75 A

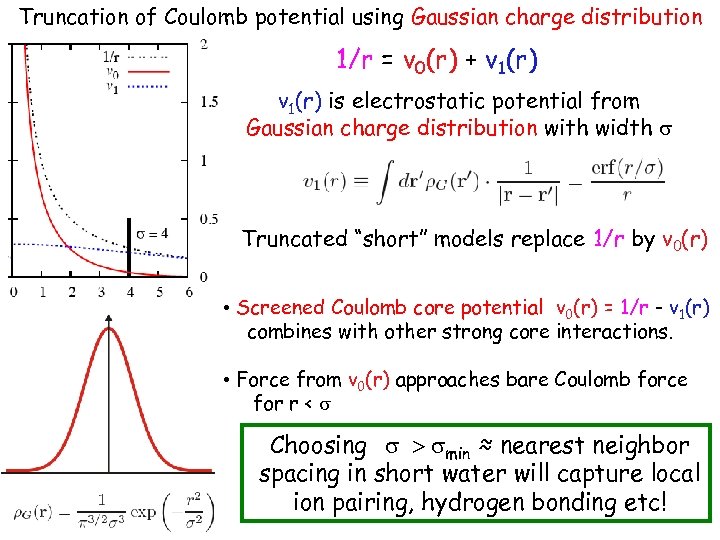
Truncation of Coulomb potential using Gaussian charge distribution 1/r = v 0(r) + v 1(r) is electrostatic potential from Gaussian charge distribution with width Truncated “short” models replace 1/r by v 0(r) • Screened Coulomb core potential v 0(r) = 1/r - v 1(r) combines with other strong core interactions. • Force from v 0(r) approaches bare Coulomb force for r < Choosing min ≈ nearest neighbor spacing in short water will capture local ion pairing, hydrogen bonding etc!

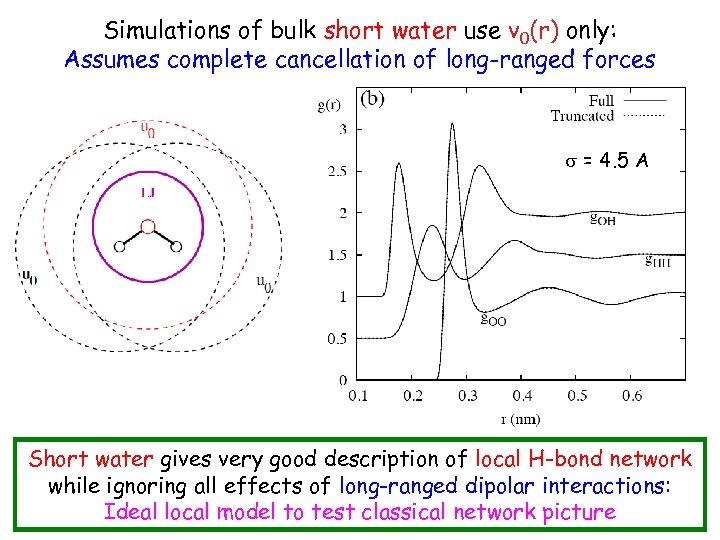
Simulations of bulk short water use v 0(r) only: Assumes complete cancellation of long-ranged forces = 4. 5 A Short water gives very good description of local H-bond network while ignoring all effects of long-ranged dipolar interactions: Ideal local model to test classical network picture

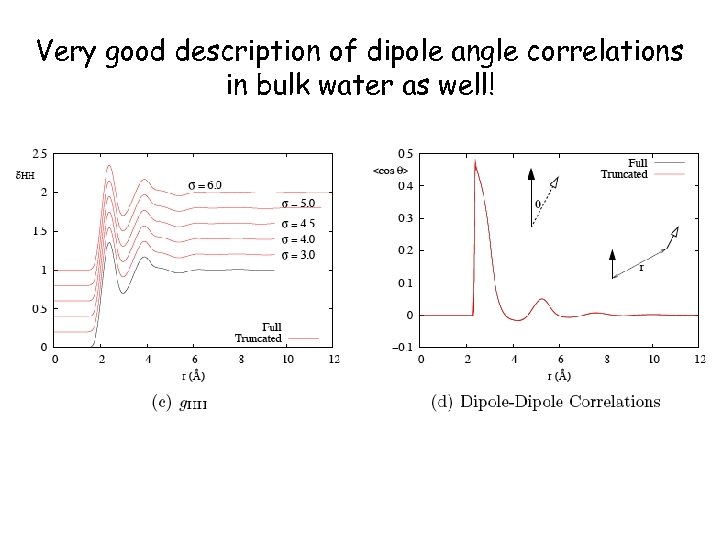
Very good description of dipole angle correlations in bulk water as well!

Site-site radial distribution functions for Acetonitrile HC CT YC YN

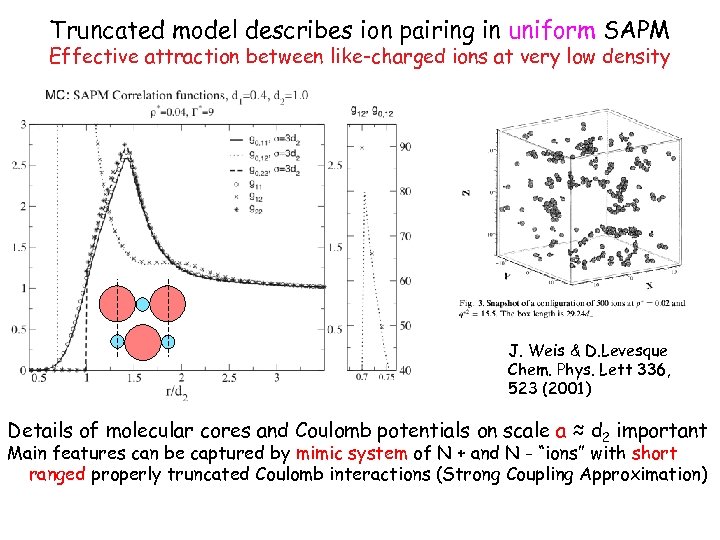
Truncated model describes ion pairing in uniform SAPM Effective attraction between like-charged ions at very low density J. Weis & D. Levesque Chem. Phys. Lett 336, 523 (2001) Details of molecular cores and Coulomb potentials on scale a ≈ d 2 important Main features can be captured by mimic system of N + and N - “ions” with short ranged properly truncated Coulomb interactions (Strong Coupling Approximation)

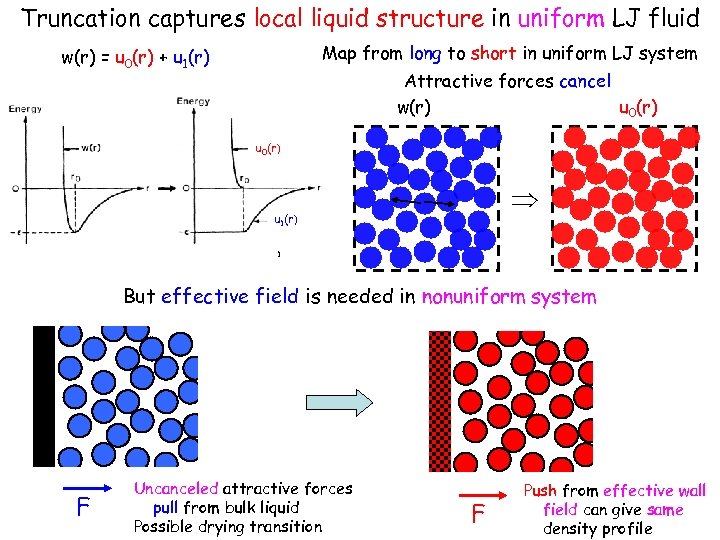
Truncation captures local liquid structure in uniform LJ fluid Map from long to short in uniform LJ system w(r) = u 0(r) + u 1(r) Attractive forces cancel w(r) u 0(r) u 1(r) 1 But effective field is needed in nonuniform system F Uncanceled attractive forces pull from bulk liquid Possible drying transition F Push from effective wall field can give same density profile

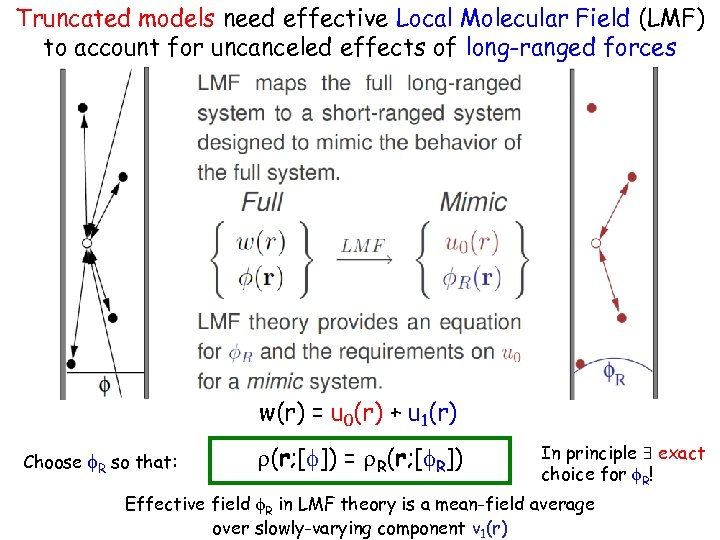
Truncated models need effective Local Molecular Field (LMF) to account for uncanceled effects of long-ranged forces w(r) = u 0(r) + u 1(r) Choose R so that: (r; [ ]) = R(r; [ R]) In principle exact choice for R! Effective field R in LMF theory is a mean-field average over slowly-varying component v 1(r)

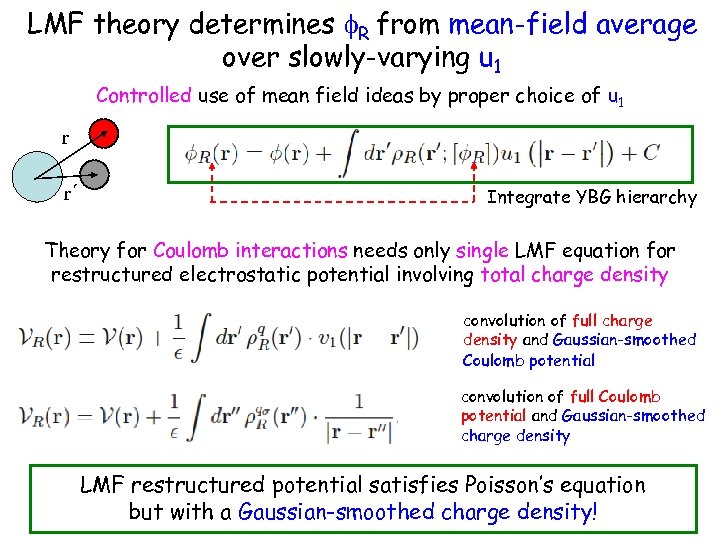
LMF theory determines R from mean-field average over slowly-varying u 1 Controlled use of mean field ideas by proper choice of u 1 r r´ Integrate YBG hierarchy Theory for Coulomb interactions needs only single LMF equation for restructured electrostatic potential involving total charge density convolution of full charge density and Gaussian-smoothed Coulomb potential convolution of full Coulomb potential and Gaussian-smoothed charge density LMF restructured potential satisfies Poisson’s equation but with a Gaussian-smoothed charge density!

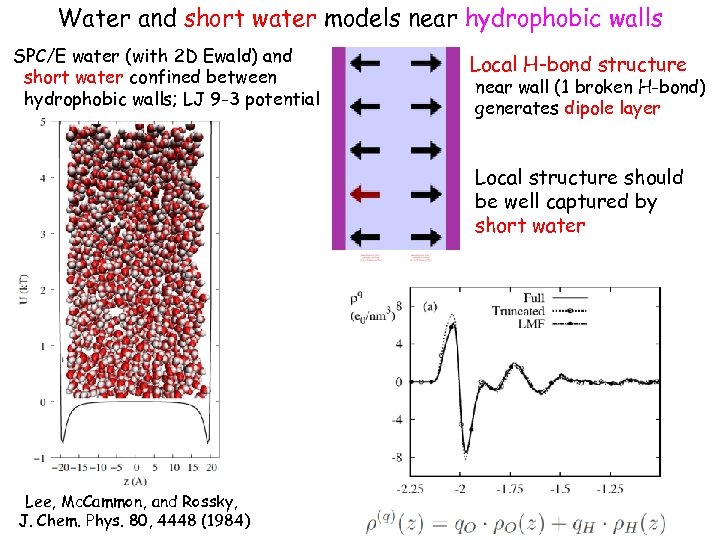
Water and short water models near hydrophobic walls SPC/E water (with 2 D Ewald) and short water confined between hydrophobic walls; LJ 9 -3 potential Local H-bond structure near wall (1 broken H-bond) generates dipole layer Local structure should be well captured by short water Lee, Mc. Cammon, and Rossky, J. Chem. Phys. 80, 4448 (1984)

Competition between local H-bond structure and long-ranged dipolar forces important for electrostatic properties • Short system accounts only for local H-bonds • Neglects competing long-ranged effects of dipole layers out to ∞ in x- and y directions • This is precisely what an effective LMF can capture! LMF affects long-wavelength orientations of H-bond network

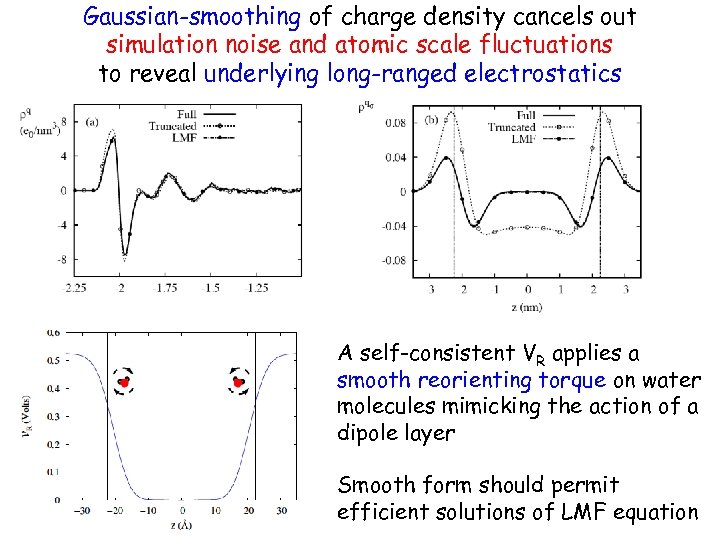
Gaussian-smoothing of charge density cancels out simulation noise and atomic scale fluctuations to reveal underlying long-ranged electrostatics A self-consistent VR applies a smooth reorienting torque on water molecules mimicking the action of a dipole layer Smooth form should permit efficient solutions of LMF equation

LMF theory and classical electrostatics: why does it work so well? We show effective field R in LMF theory satisfies Poisson’s equation but with a Gaussian-smoothed (over scale ) charge density • Classical electrostatics smoothes over molecular scale fluctuations in deriving basic equations for polarization field P and other dielectric properties = Purcell: Electricity And Magnetism 1963 • LMF theory provides a general conceptual framework that shows how to carry out such smoothing in general environments and using realistic molecular models. • may be a fundamental length scale in molecular electrostatics

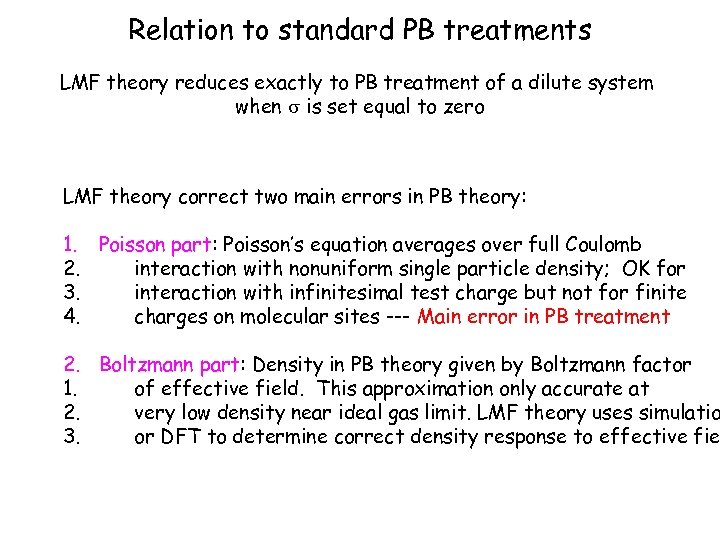
Relation to standard PB treatments LMF theory reduces exactly to PB treatment of a dilute system when is set equal to zero LMF theory correct two main errors in PB theory: 1. 2. 3. 4. Poisson part: Poisson’s equation averages over full Coulomb interaction with nonuniform single particle density; OK for interaction with infinitesimal test charge but not for finite charges on molecular sites --- Main error in PB treatment 2. 1. 2. 3. Boltzmann part: Density in PB theory given by Boltzmann factor of effective field. This approximation only accurate at very low density near ideal gas limit. LMF theory uses simulatio or DFT to determine correct density response to effective fie

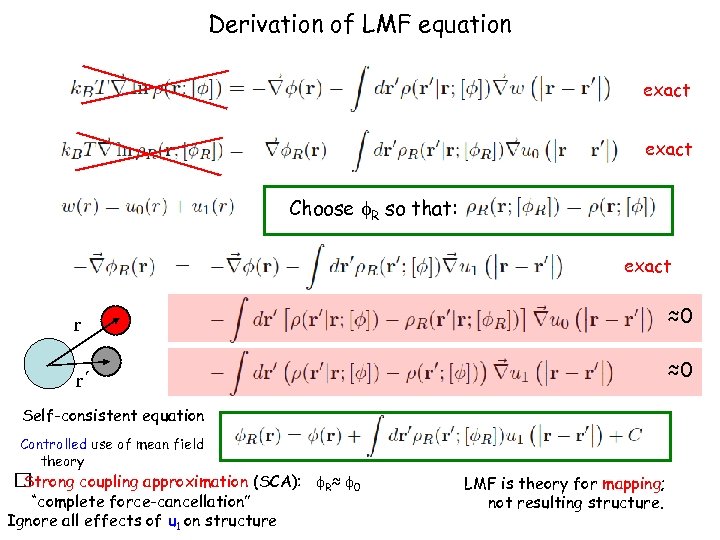
Derivation of LMF equation exact Choose R so that: exact r ≈0 r´ ≈0 Self-consistent equation Controlled use of mean field theory Strong coupling approximation (SCA): “complete force-cancellation” Ignore all effects of u 1 on structure R≈ 0 LMF is theory for mapping; not resulting structure.

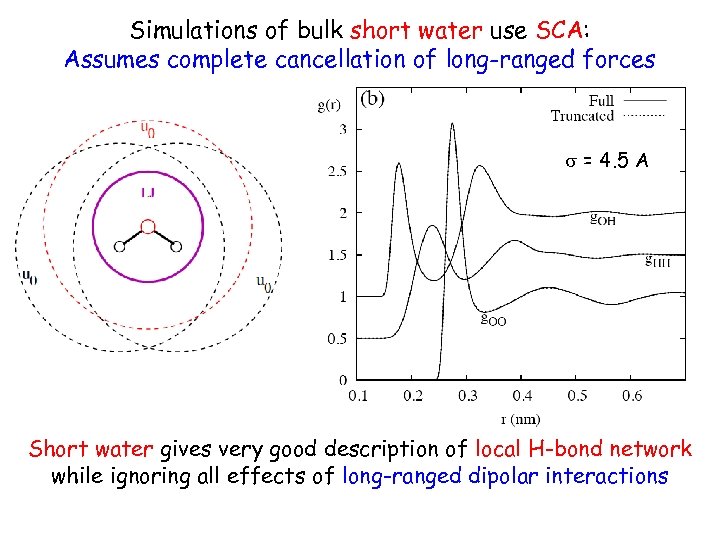
Simulations of bulk short water use SCA: Assumes complete cancellation of long-ranged forces = 4. 5 A Short water gives very good description of local H-bond network while ignoring all effects of long-ranged dipolar interactions

Very good description of dipole angle correlations in bulk water as well

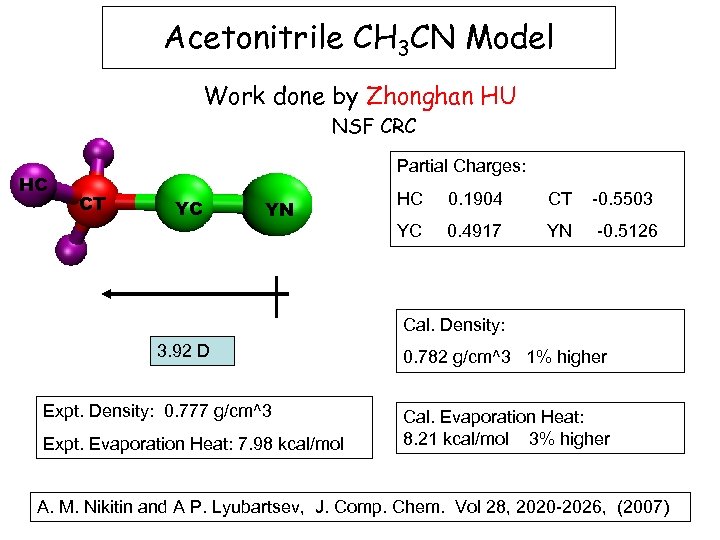
Acetonitrile CH 3 CN Model Work done by Zhonghan HU NSF CRC HC Partial Charges: CT YC YN HC 0. 1904 CT -0. 5503 YC 0. 4917 YN -0. 5126 Cal. Density: 3. 92 D Expt. Density: 0. 777 g/cm^3 Expt. Evaporation Heat: 7. 98 kcal/mol 0. 782 g/cm^3 1% higher Cal. Evaporation Heat: 8. 21 kcal/mol 3% higher A. M. Nikitin and A P. Lyubartsev, J. Comp. Chem. Vol 28, 2020 -2026, (2007)

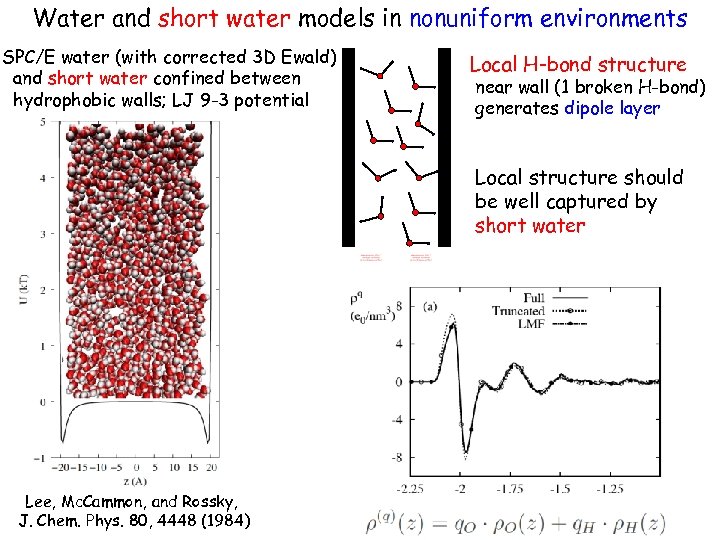
Water and short water models in nonuniform environments SPC/E water (with corrected 3 D Ewald) and short water confined between hydrophobic walls; LJ 9 -3 potential Local H-bond structure near wall (1 broken H-bond) generates dipole layer Local structure should be well captured by short water Lee, Mc. Cammon, and Rossky, J. Chem. Phys. 80, 4448 (1984)

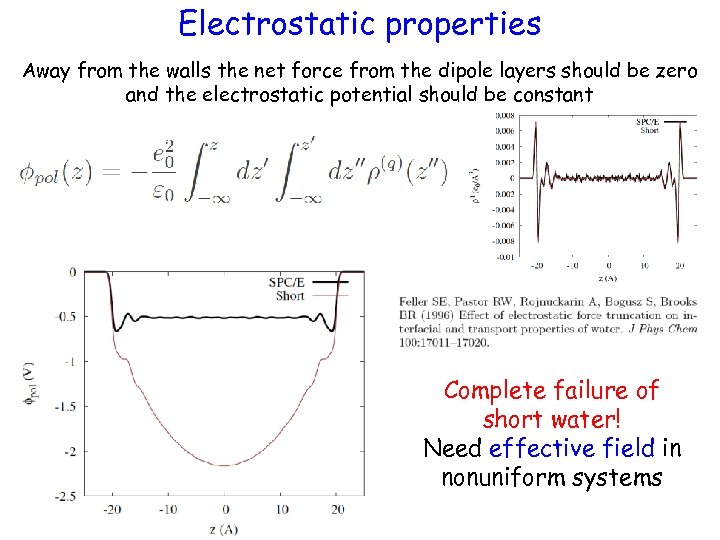
Electrostatic properties Away from the walls the net force from the dipole layers should be zero and the electrostatic potential should be constant Complete failure of short water! Need effective field in nonuniform systems

Competition between local H-bond structure and long-ranged dipolar forces important for electrostatic properties. • Short system accounts only for local H-bonds • Neglects competing long-ranged effects of dipole layers out to ∞ in x- and y directions • This is precisely what an effective LMF can capture!

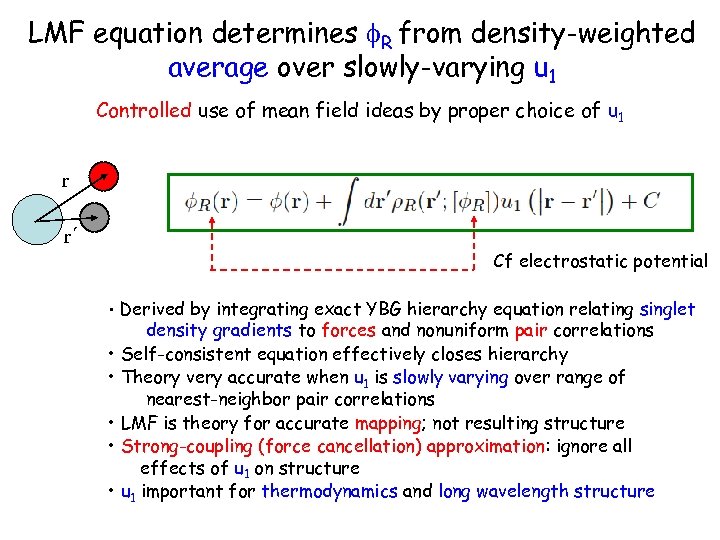
LMF equation determines R from density-weighted average over slowly-varying u 1 Controlled use of mean field ideas by proper choice of u 1 r r´ Cf electrostatic potential • Derived by integrating exact YBG hierarchy equation relating singlet density gradients to forces and nonuniform pair correlations • Self-consistent equation effectively closes hierarchy • Theory very accurate when u 1 is slowly varying over range of nearest-neighbor pair correlations • LMF is theory for accurate mapping; not resulting structure • Strong-coupling (force cancellation) approximation: ignore all effects of u 1 on structure • u 1 important for thermodynamics and long wavelength structure

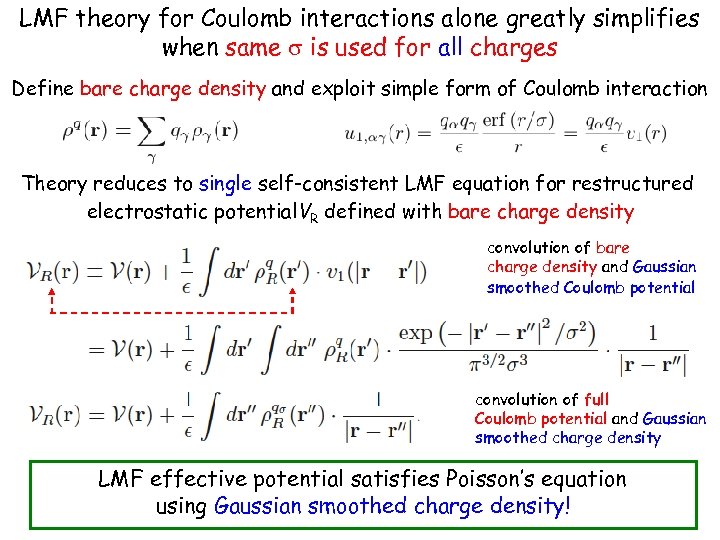
LMF theory for Coulomb interactions alone greatly simplifies when same is used for all charges Define bare charge density and exploit simple form of Coulomb interaction Theory reduces to single self-consistent LMF equation for restructured electrostatic potential. VR defined with bare charge density convolution of bare charge density and Gaussian smoothed Coulomb potential convolution of full Coulomb potential and Gaussian smoothed charge density LMF effective potential satisfies Poisson’s equation using Gaussian smoothed charge density!

Gaussian-smoothing of charge density in LMF theory cancels out simulation noise and atomic scale fluctuations to reveal underlying long-ranged electrostatics A self-consistent VR applies a smooth reorienting torque on water molecules, mimicking the action of a dipole layer Smooth form should permit efficient solutions of LMF equation

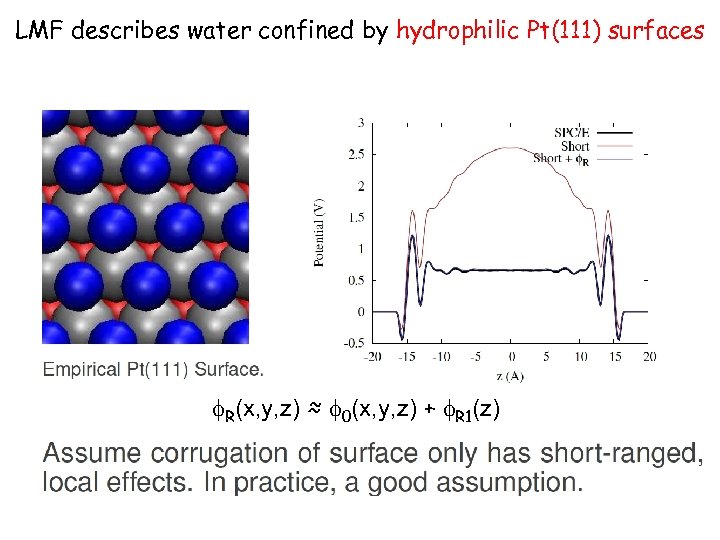
LMF describes water confined by hydrophilic Pt(111) surfaces R(x, y, z) ≈ 0(x, y, z) + R 1(z)

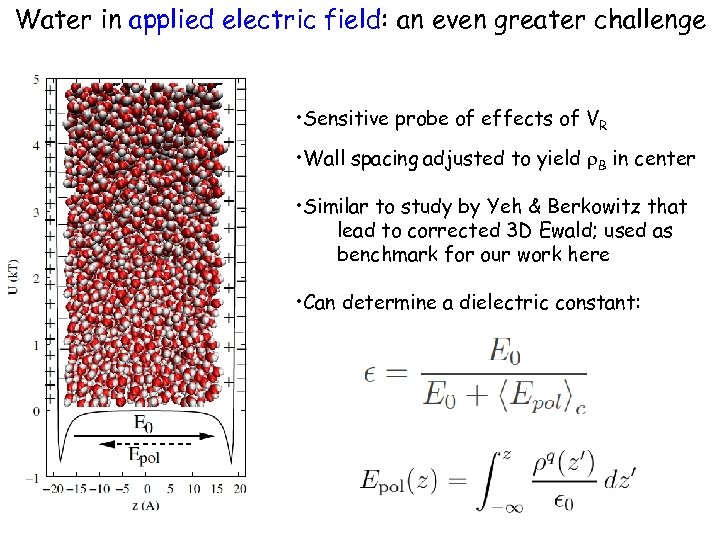
Water in applied electric field: an even greater challenge • Sensitive probe of effects of VR • Wall spacing adjusted to yield B in center • Similar to study by Yeh & Berkowitz that lead to corrected 3 D Ewald; used as benchmark for our work here • Can determine a dielectric constant:

LMF theory corrects very poor results for short water in electric field Negative dielectric constant predicted for short water for using standard formula! LMF theory in excellent agreement with full Ewald.

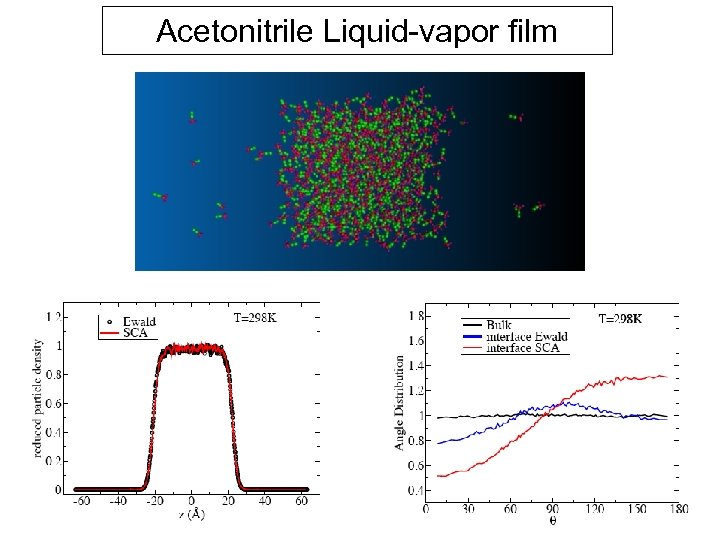
Acetonitrile Liquid-vapor film

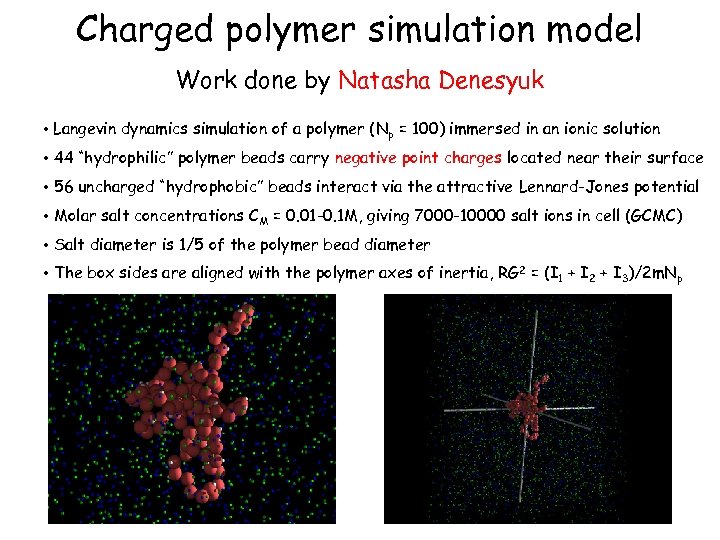
Charged polymer simulation model Work done by Natasha Denesyuk • Langevin dynamics simulation of a polymer (Np = 100) immersed in an ionic solution • 44 “hydrophilic” polymer beads carry negative point charges located near their surface • 56 uncharged “hydrophobic” beads interact via the attractive Lennard-Jones potential • Molar salt concentrations CM = 0. 01 -0. 1 M, giving 7000 -10000 salt ions in cell (GCMC) • Salt diameter is 1/5 of the polymer bead diameter • The box sides are aligned with the polymer axes of inertia, RG 2 = (I 1 + I 2 + I 3)/2 m. Np

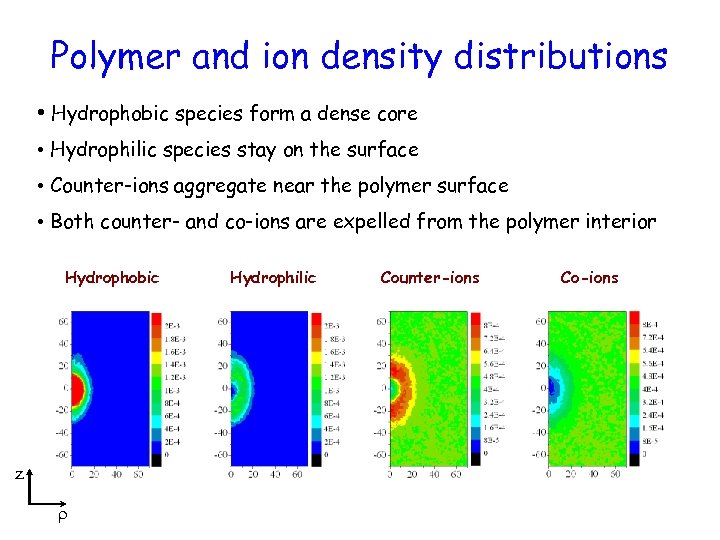
Polymer and ion density distributions • Hydrophobic species form a dense core • Hydrophilic species stay on the surface • Counter-ions aggregate near the polymer surface • Both counter- and co-ions are expelled from the polymer interior Hydrophobic z Hydrophilic Counter-ions Co-ions

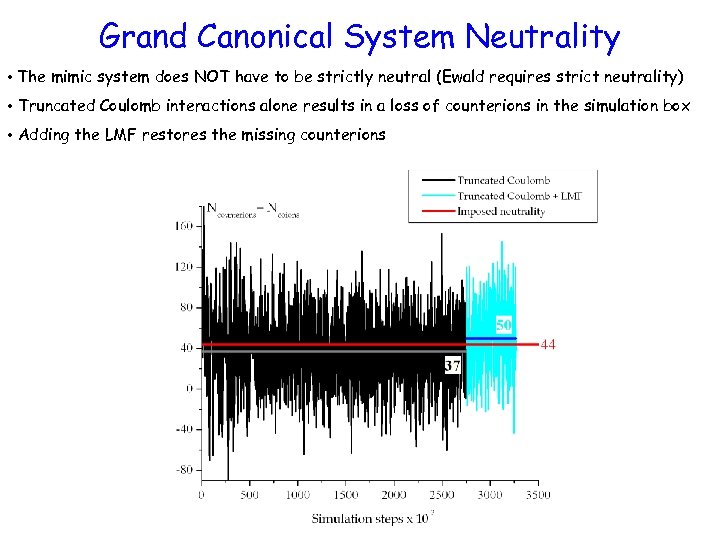
Grand Canonical System Neutrality • The mimic system does NOT have to be strictly neutral (Ewald requires strict neutrality) • Truncated Coulomb interactions alone results in a loss of counterions in the simulation box • Adding the LMF restores the missing counterions

Conclusions • SPC/E water and CH 3 CN can be very accurately described by short-ranged mimic system in effective external field • Effective field accounts for mean field average of special long-ranged slowly varying component of Coulomb interactions • Effective field satisfies Poisson’s equation with Gaussian-smoothed charge density • Effective field corrects major errors in electrostatic properties of nonuniform systems from simple truncations of long-ranged forces. • No Ewald sums etc. needed in mimic simulations • LMF method adapted to open-source DL-Poly MD code and in-house Langevin MD polymer simulation code • Further work on ions, water, dipolar fluids near silica surfaces, charged polymers, etc. in progress • LMF theory provides a unified conceptual framework for wide class of nonuniform molecular fluids: ions, polymer and water models, …

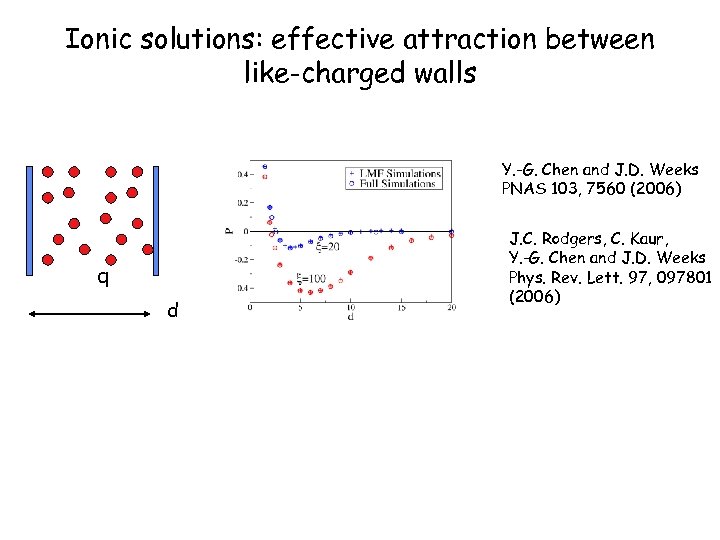
Ionic solutions: effective attraction between like-charged walls Y. -G. Chen and J. D. Weeks PNAS 103, 7560 (2006) q d J. C. Rodgers, C. Kaur, Y. -G. Chen and J. D. Weeks Phys. Rev. Lett. 97, 097801 (2006)

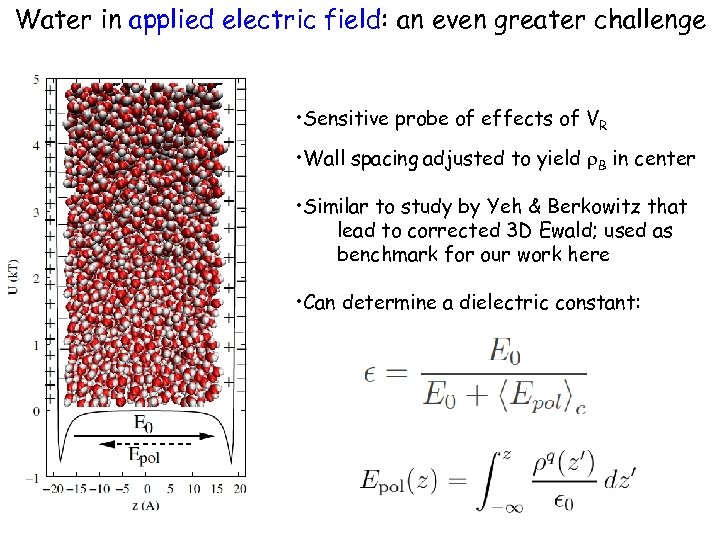
Water in applied electric field: an even greater challenge • Sensitive probe of effects of VR • Wall spacing adjusted to yield B in center • Similar to study by Yeh & Berkowitz that lead to corrected 3 D Ewald; used as benchmark for our work here • Can determine a dielectric constant:

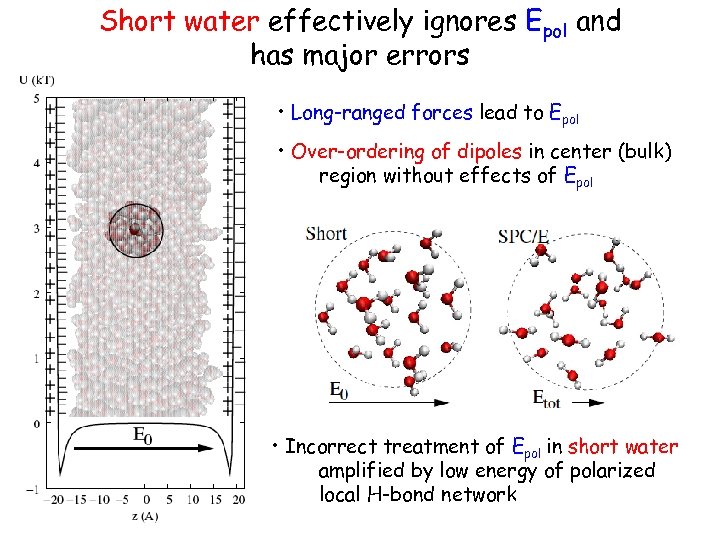
Short water effectively ignores Epol and has major errors • Long-ranged forces lead to Epol • Over-ordering of dipoles in center (bulk) region without effects of Epol • Incorrect treatment of Epol in short water amplified by low energy of polarized local H-bond network

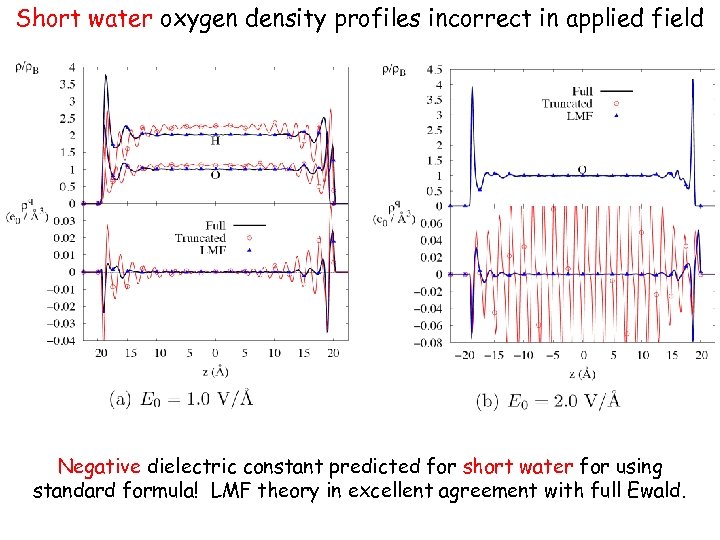
Short water oxygen density profiles incorrect in applied field Negative dielectric constant predicted for short water for using standard formula! LMF theory in excellent agreement with full Ewald.

LMF tames applied field systems as well Self-consistent VR generates weak force on molecules at center Short water feels nearly full force from bare V everywhere