01 Introduction + Arithmetic Progressions.pptx
- Количество слайдов: 23

Series Introduction Arithmetic Progressions

Today’s Objectives • Definition of a sequence and a series • Series notation • Definition of an arithmetic progression and the nth term • Sum of an arithmetic progression
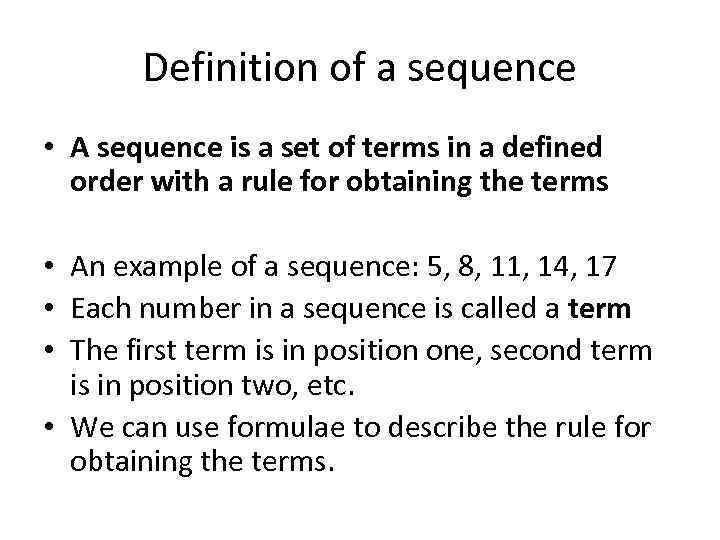
Definition of a sequence • A sequence is a set of terms in a defined order with a rule for obtaining the terms • An example of a sequence: 5, 8, 11, 14, 17 • Each number in a sequence is called a term • The first term is in position one, second term is in position two, etc. • We can use formulae to describe the rule for obtaining the terms.
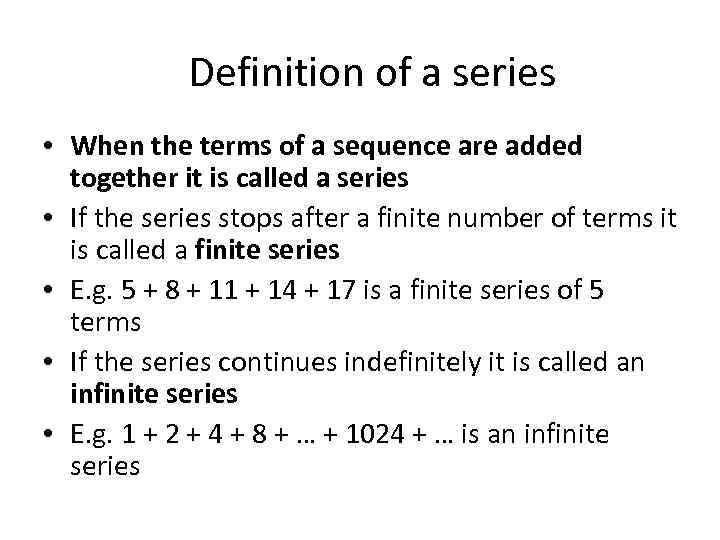
Definition of a series • When the terms of a sequence are added together it is called a series • If the series stops after a finite number of terms it is called a finite series • E. g. 5 + 8 + 11 + 14 + 17 is a finite series of 5 terms • If the series continues indefinitely it is called an infinite series • E. g. 1 + 2 + 4 + 8 + … + 1024 + … is an infinite series
Formulae • We have said that the rule for obtaining the terms of a sequence can be expressed with a formula. • So we need to be able to use the formula for a given sequence to find the terms and vice versa.
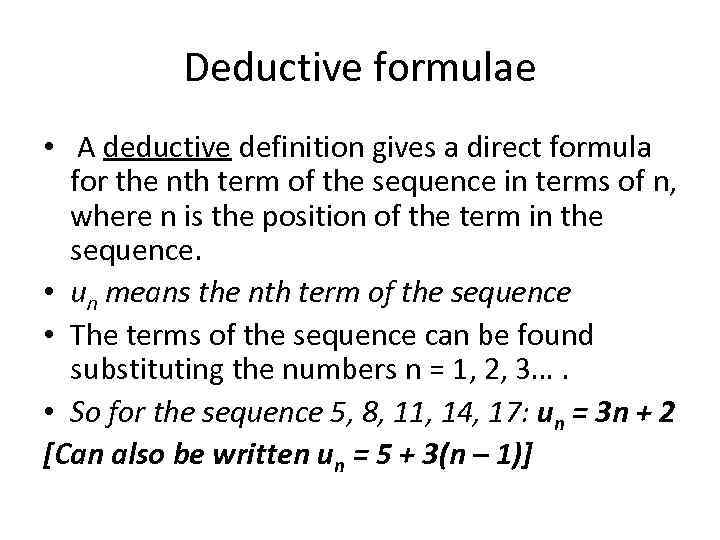
Deductive formulae • A deductive definition gives a direct formula for the nth term of the sequence in terms of n, where n is the position of the term in the sequence. • un means the nth term of the sequence • The terms of the sequence can be found substituting the numbers n = 1, 2, 3…. • So for the sequence 5, 8, 11, 14, 17: un = 3 n + 2 [Can also be written un = 5 + 3(n – 1)]

Deductive formulae • For example, if the nth term is given by un = 3 n + 2 • Then substituting n = 1, 2, 3, 4, 5, … gives the sequence 5, 8, 11, 14, 17, …. [We could also have written un = 5 + 3(n – 1). Notice that 5 is the first term and 3 is the difference between each term. ]

Finding the formula • To find the formula for a sequence we first look at the difference between the terms and from this decide what category the sequence is in. • If the difference (d) is always the same then the sequence is like a times table and the formula is a version of ‘dn’ where n is the position. • Find the formula for: – 6, 10, 14, 18, … – -2, -6, -10, -14, … (See hand written solutions)

Finding the formula • If the difference is not always the same but the difference of the differences (the second difference d 2) is the same then the sequence is a quadratic and the formula is a version of ‘n 2(d 2)/2’ where n is the position. • Find the formula for 2, 9, 20, 35, … • See hand written solution

Finding the formula • If the second difference is not the same then there is no set method to find the formula. Instead we use trial and error considering cubes, higher powers of n, n as the index and so on. • Find the formula for: – 1, 8, 27, 64, 125, … – 2, 8, 26, 62, 122, … – 2, 4, 8, 16, 32, … – 3, 6, 11, 20, 37, … (See hand written solutions)
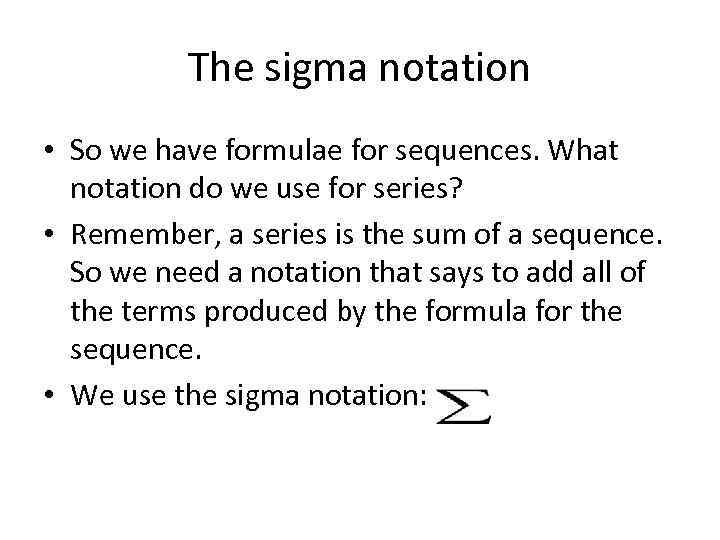
The sigma notation • So we have formulae for sequences. What notation do we use for series? • Remember, a series is the sum of a sequence. So we need a notation that says to add all of the terms produced by the formula for the sequence. • We use the sigma notation:
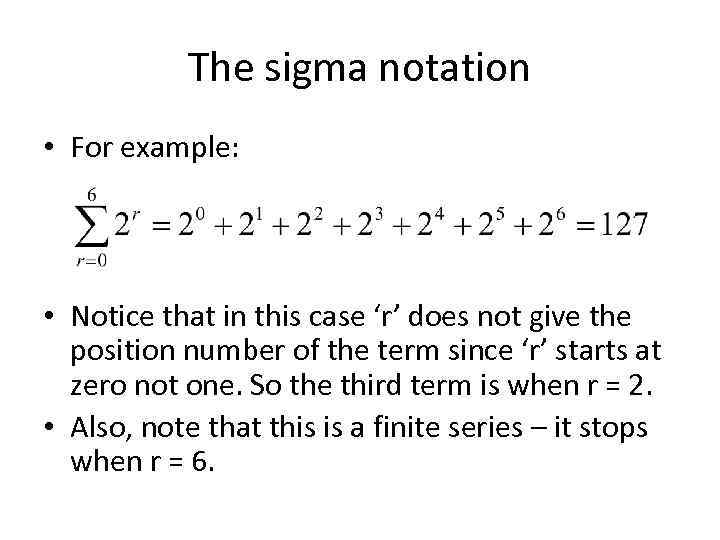
The sigma notation • For example: • Notice that in this case ‘r’ does not give the position number of the term since ‘r’ starts at zero not one. So the third term is when r = 2. • Also, note that this is a finite series – it stops when r = 6.
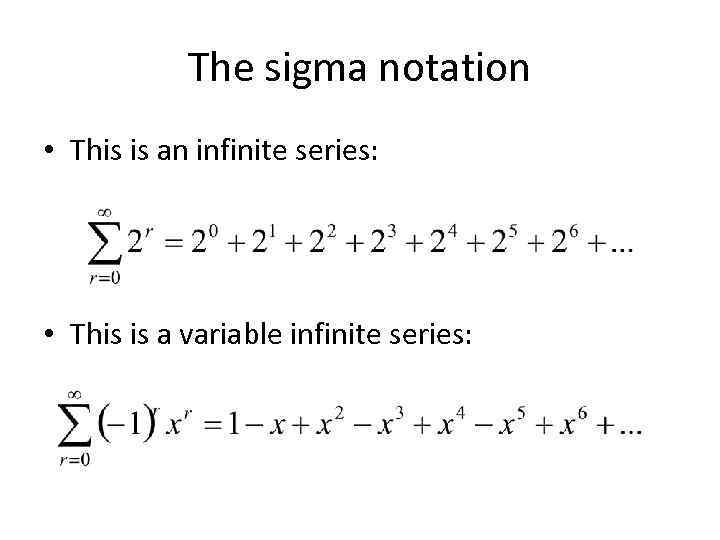
The sigma notation • This is an infinite series: • This is a variable infinite series:
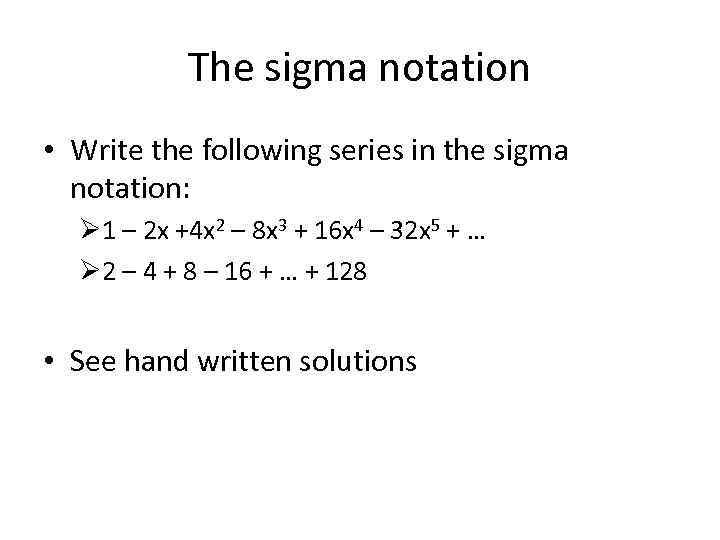
The sigma notation • Write the following series in the sigma notation: Ø 1 – 2 x +4 x 2 – 8 x 3 + 16 x 4 – 32 x 5 + … Ø 2 – 4 + 8 – 16 + … + 128 • See hand written solutions
Arithmetic Progressions (APs) • An arithmetic progression is when the difference between each term is a fixed amount. • This difference is known as the common difference and we use the letter d. • The first and second examples above arithmetic progressions; Ø 6, 10, 14, 18, … (un = 6 + (n – 1)4) Øand – 2, – 6, – 10, – 14, … (un = – 2 + (n – 1)(– 4)) ØSee hand written solutions
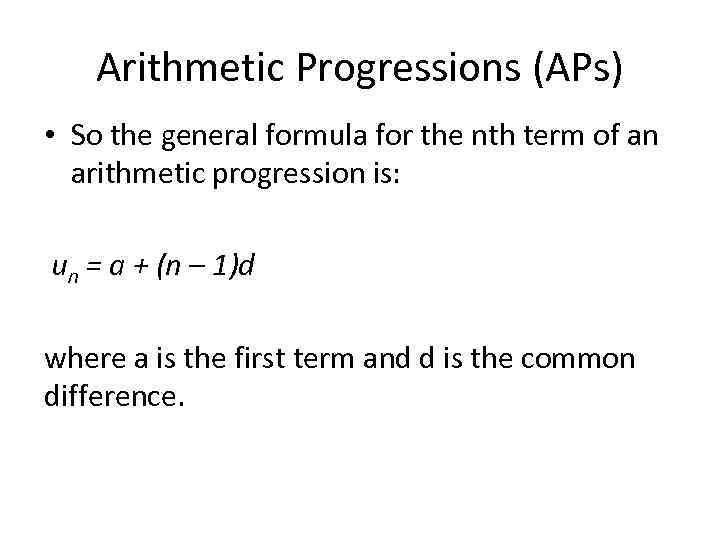
Arithmetic Progressions (APs) • So the general formula for the nth term of an arithmetic progression is: un = a + (n – 1)d where a is the first term and d is the common difference.

AP Examples • See handwritten examples with solutions

Sum of an AP • Considering the sum of the first n terms of the sequence (i. e. the series), where a is the first term, d is the common difference and l is the last term: • But this is rather long.
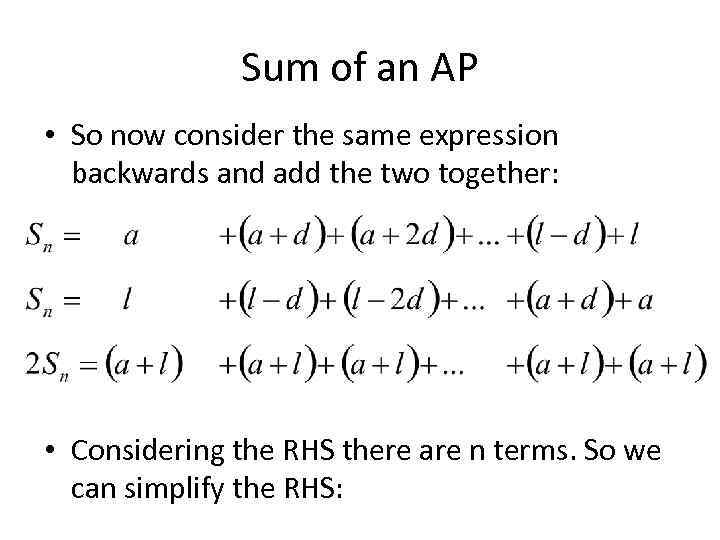
Sum of an AP • So now consider the same expression backwards and add the two together: • Considering the RHS there are n terms. So we can simplify the RHS:
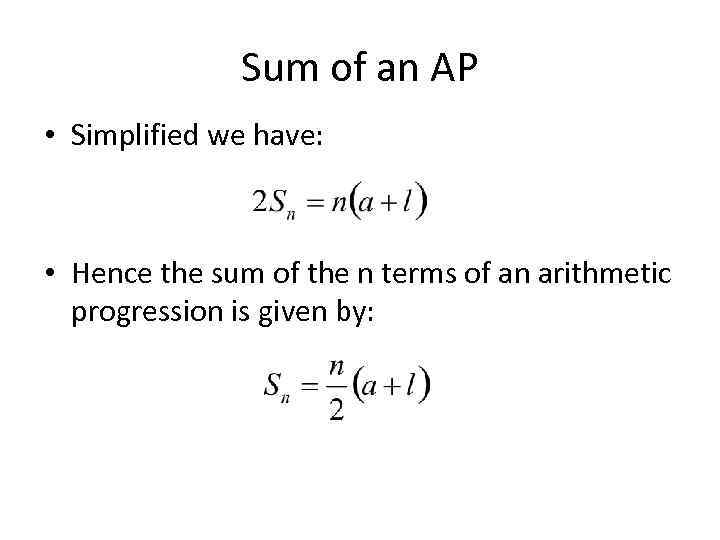
Sum of an AP • Simplified we have: • Hence the sum of the n terms of an arithmetic progression is given by:
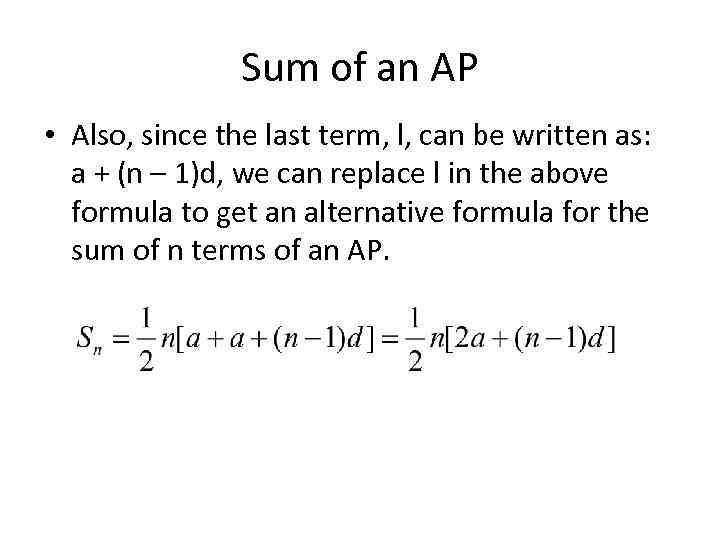
Sum of an AP • Also, since the last term, l, can be written as: a + (n – 1)d, we can replace l in the above formula to get an alternative formula for the sum of n terms of an AP.

Sum of an AP Examples • See handwritten examples with solutions
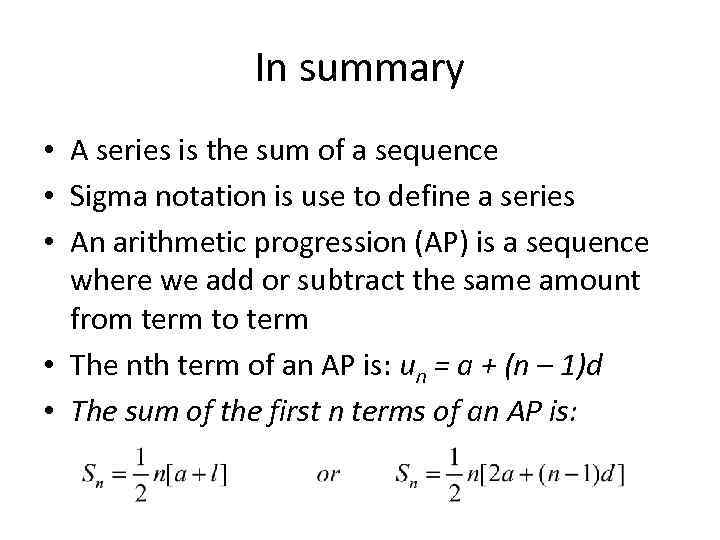
In summary • A series is the sum of a sequence • Sigma notation is use to define a series • An arithmetic progression (AP) is a sequence where we add or subtract the same amount from term to term • The nth term of an AP is: un = a + (n – 1)d • The sum of the first n terms of an AP is:
01 Introduction + Arithmetic Progressions.pptx