RBEs and MPCs in MSC. Nastran ARipRoarin’Reviewof RigidElements











rbe_and_mpc_in_nastran.ppt
- Размер: 1.8 Mегабайта
- Количество слайдов: 94
Описание презентации RBEs and MPCs in MSC. Nastran ARipRoarin’Reviewof RigidElements по слайдам
 RBEs and MPCs in MSC. Nastran ARip. Roarin’Reviewof Rigid. Elements
RBEs and MPCs in MSC. Nastran ARip. Roarin’Reviewof Rigid. Elements
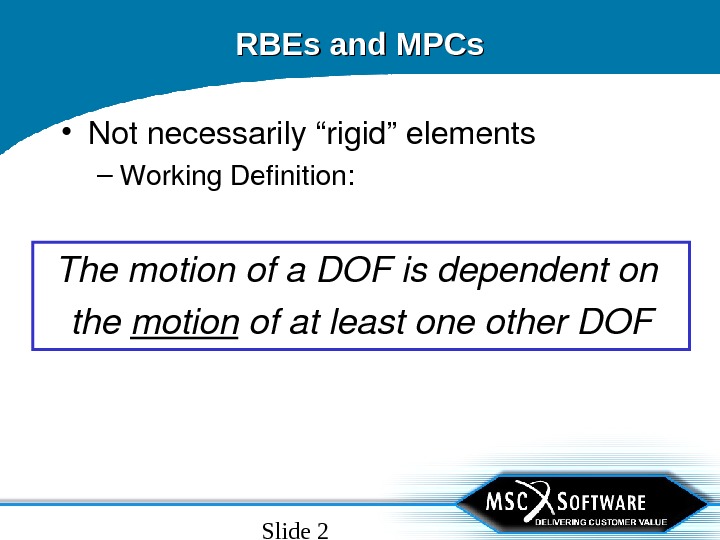
 Slide 2 RBEs and MPCs • Notnecessarily“rigid”elements – Working. Definition: Themotionofa. DOFisdependenton the motion ofatleastoneother. DO
Slide 2 RBEs and MPCs • Notnecessarily“rigid”elements – Working. Definition: Themotionofa. DOFisdependenton the motion ofatleastoneother. DO
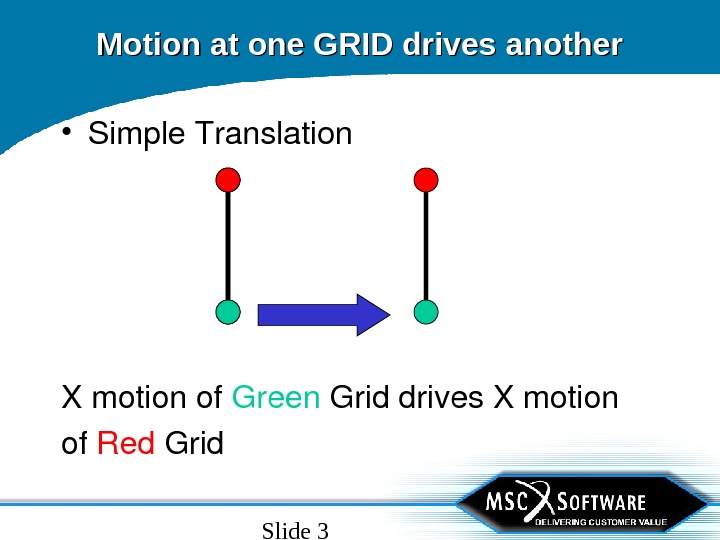
 Slide 3 Motion at one GRID drives another • Simple. Translation Xmotionof Green Griddrives. Xmotion of Red Grid
Slide 3 Motion at one GRID drives another • Simple. Translation Xmotionof Green Griddrives. Xmotion of Red Grid
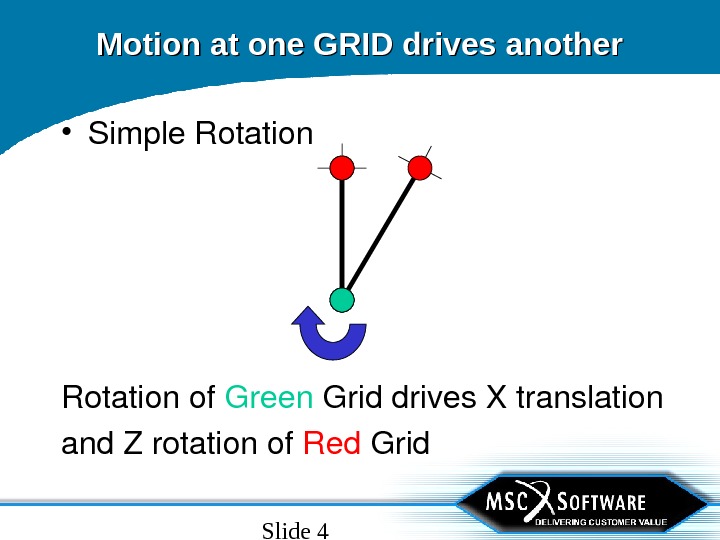
 Slide 4 Motion at one GRID drives another • Simple. Rotationof Green Griddrives. Xtranslation and. Zrotationof Red Grid
Slide 4 Motion at one GRID drives another • Simple. Rotationof Green Griddrives. Xtranslation and. Zrotationof Red Grid
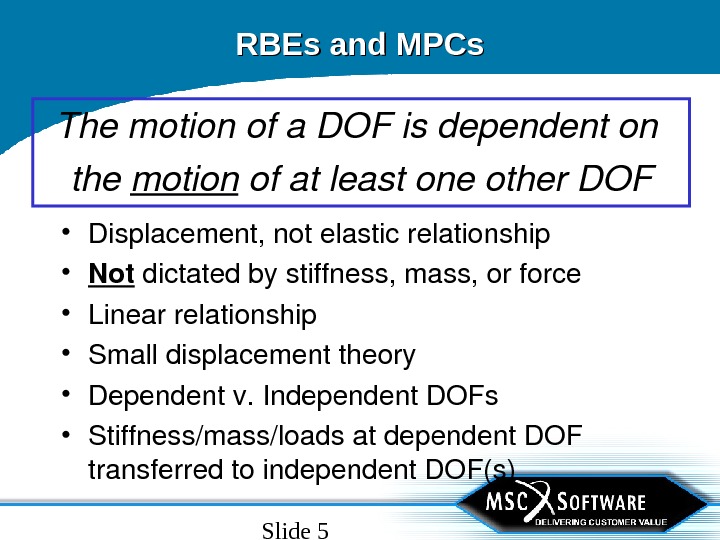
 Slide 5 RBEs and MPCs Themotionofa. DOFisdependenton the motion ofatleastoneother. DOF • Displacement, notelasticrelationship • Not dictatedbystiffness, mass, orforce • Linearrelationship • Smalldisplacementtheory • Dependentv. Independent. DOFs • Stiffness/mass/loadsatdependent. DOF transferredtoindependent. DOF(s)
Slide 5 RBEs and MPCs Themotionofa. DOFisdependenton the motion ofatleastoneother. DOF • Displacement, notelasticrelationship • Not dictatedbystiffness, mass, orforce • Linearrelationship • Smalldisplacementtheory • Dependentv. Independent. DOFs • Stiffness/mass/loadsatdependent. DOF transferredtoindependent. DOF(s)
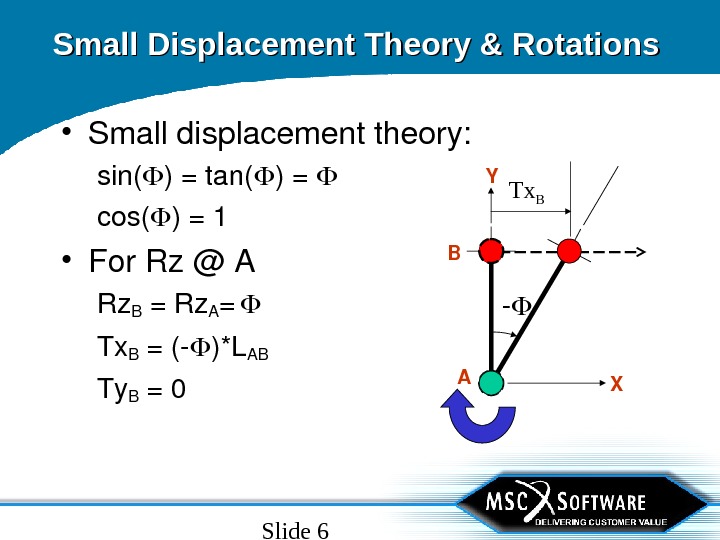
 Slide 6 Small Displacement Theory & Rotations • Smalldisplacementtheory: sin( )=tan( )= cos( )=1 • For. Rz@A Rz. B =Rz. A = Tx B =( )*LAB Ty B =0 XY AB Tx
Slide 6 Small Displacement Theory & Rotations • Smalldisplacementtheory: sin( )=tan( )= cos( )=1 • For. Rz@A Rz. B =Rz. A = Tx B =( )*LAB Ty B =0 XY AB Tx
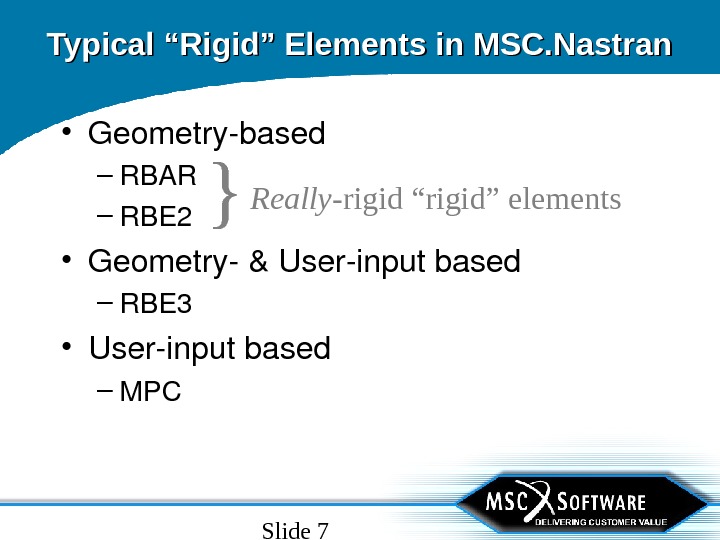
 Slide 7 • Geometrybased – RBAR – RBE 2 • Geometry&Userinputbased – RBE 3 • Userinputbased – MPCTypical “Rigid” Elements in MSC. Nastran } Really- rigid “rigid” elements
Slide 7 • Geometrybased – RBAR – RBE 2 • Geometry&Userinputbased – RBE 3 • Userinputbased – MPCTypical “Rigid” Elements in MSC. Nastran } Really- rigid “rigid” elements
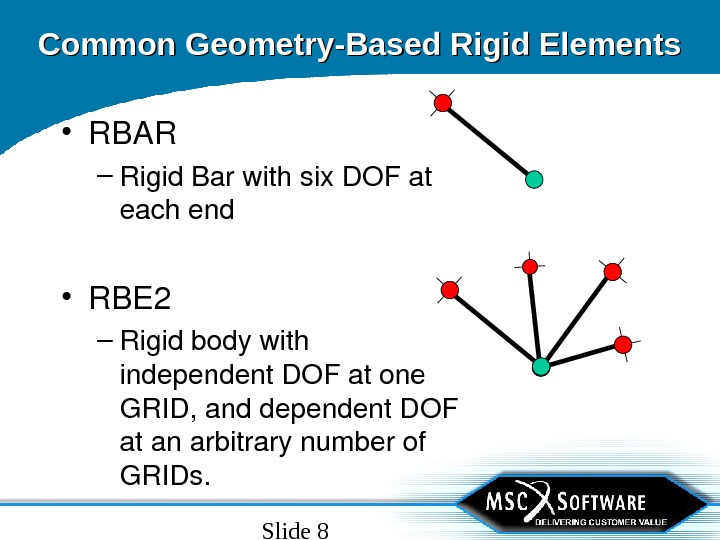
 Slide 8 Common Geometry-Based Rigid Elements • RBAR – Rigid. Barwithsix. DOFat eachend • RBE 2 – Rigidbodywith independent. DOFatone GRID, anddependent. DOF atanarbitrarynumberof GRIDs.
Slide 8 Common Geometry-Based Rigid Elements • RBAR – Rigid. Barwithsix. DOFat eachend • RBE 2 – Rigidbodywith independent. DOFatone GRID, anddependent. DOF atanarbitrarynumberof GRIDs.
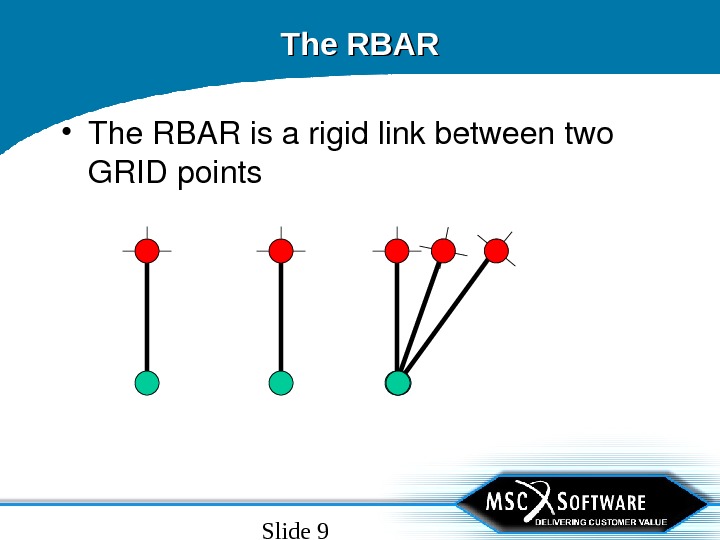
 Slide 9 The RBAR • The. RBARisarigidlinkbetweentwo GRIDpoints
Slide 9 The RBAR • The. RBARisarigidlinkbetweentwo GRIDpoints
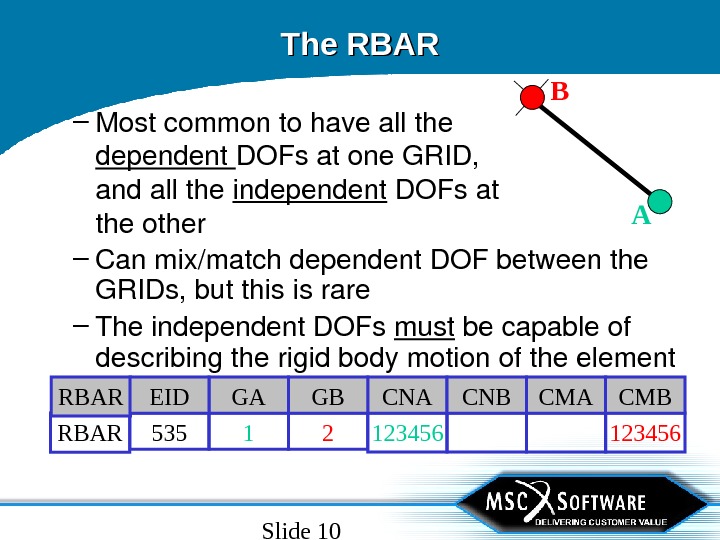
 Slide 10 The RBAR – Canmix/matchdependent. DOFbetweenthe GRIDs, butthisisrare – Theindependent. DOFs must becapableof describingtherigidbodymotionoftheelement 1234561 2 RBAR 535 CMA CMBCNA CNBGA GBRBAR EID– Mostcommontohaveallthe dependent DOFsatone. GRID, andallthe independent DOFsat theother
Slide 10 The RBAR – Canmix/matchdependent. DOFbetweenthe GRIDs, butthisisrare – Theindependent. DOFs must becapableof describingtherigidbodymotionoftheelement 1234561 2 RBAR 535 CMA CMBCNA CNBGA GBRBAR EID– Mostcommontohaveallthe dependent DOFsatone. GRID, andallthe independent DOFsat theother
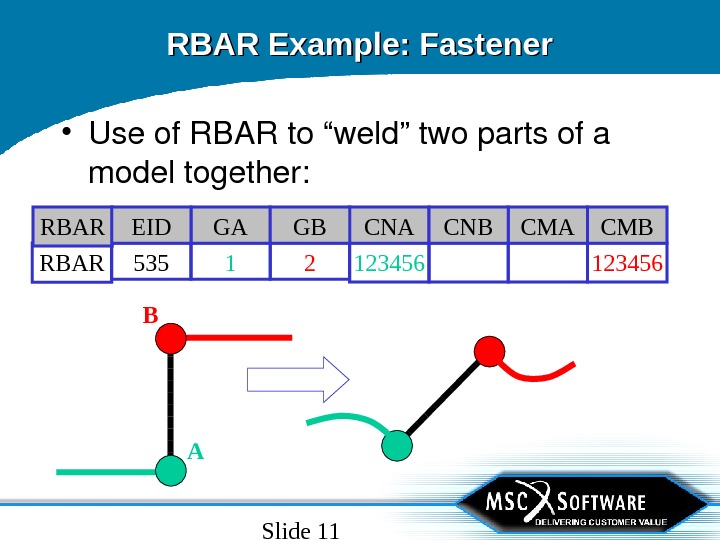
 Slide 11 RBAR Example: Fastener • Useof. RBARto“weld”twopartsofa modeltogether: 1234561 2 RBAR 535 CMA CMBCNA CNBGA GBRBAR EI
Slide 11 RBAR Example: Fastener • Useof. RBARto“weld”twopartsofa modeltogether: 1234561 2 RBAR 535 CMA CMBCNA CNBGA GBRBAR EI
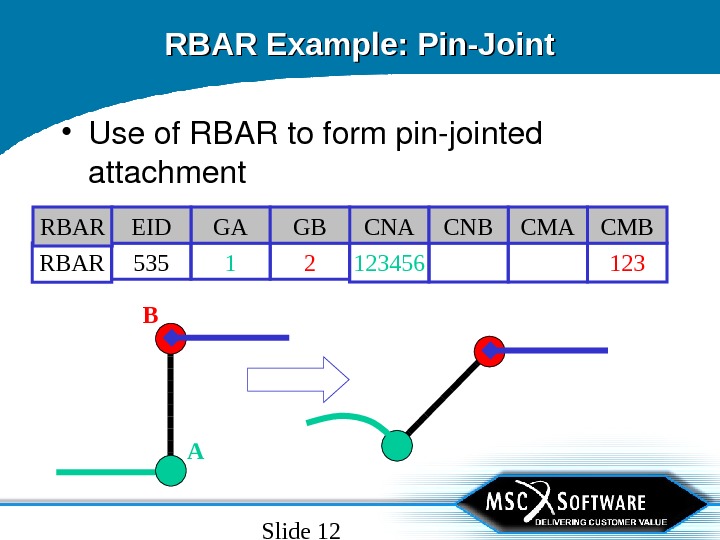
 Slide 12 RBAR Example: Pin-Joint • Useof. RBARtoformpinjointed attachment 1231234561 2 RBAR 535 CMA CMBCNA CNBGA GBRBAR EI
Slide 12 RBAR Example: Pin-Joint • Useof. RBARtoformpinjointed attachment 1231234561 2 RBAR 535 CMA CMBCNA CNBGA GBRBAR EI
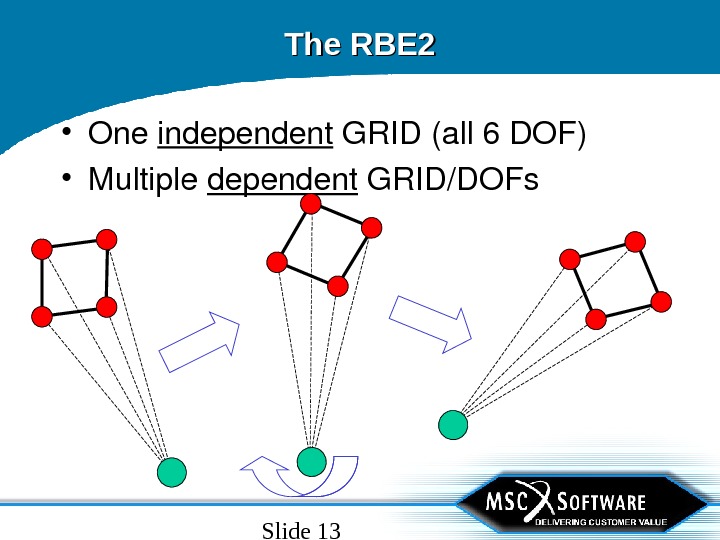
 Slide 13 The RBE 2 • One independent GRID(all 6 DOF) • Multiple dependent GRID/DOFs
Slide 13 The RBE 2 • One independent GRID(all 6 DOF) • Multiple dependent GRID/DOFs
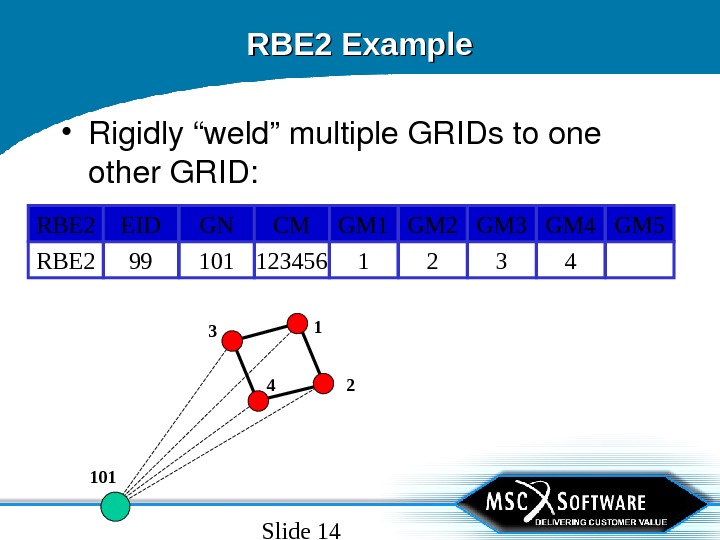
 Slide 14 RBE 2 Example • Rigidly“weld”multiple. GRIDstoone other. GRID: 32 RBE 2 4110199 123456 GM 5 GM 3 GM 2 RBE 2 GM 4 GM 1 GNEID CM
Slide 14 RBE 2 Example • Rigidly“weld”multiple. GRIDstoone other. GRID: 32 RBE 2 4110199 123456 GM 5 GM 3 GM 2 RBE 2 GM 4 GM 1 GNEID CM
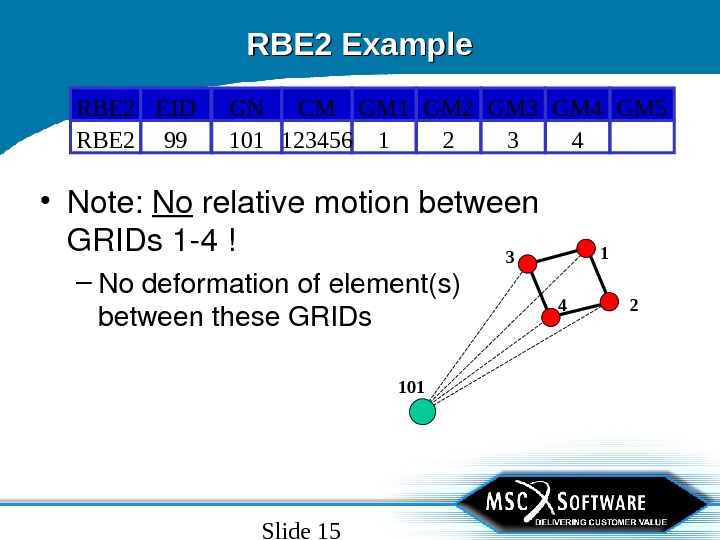
 Slide 15 RBE 2 Example • Note: No relativemotionbetween GRIDs 14! – Nodeformationofelement(s) betweenthese. GRIDs 32 RBE 2 4110199 123456 GM 5 GM 3 GM 2 RBE 2 GM 4 GM 1 GNEID CM
Slide 15 RBE 2 Example • Note: No relativemotionbetween GRIDs 14! – Nodeformationofelement(s) betweenthese. GRIDs 32 RBE 2 4110199 123456 GM 5 GM 3 GM 2 RBE 2 GM 4 GM 1 GNEID CM
 Slide 16 Common RBE 2/RBAR Uses • RBE 2 or. RBARbetween 2 GRIDs – “ Weld” 2 differentpartstogether • 6 DOFconnection – “ Bolt” 2 differentpartstogether • 3 DOFconnection • RBE 2 – “ Spider”or“wagonwheel”connections – Largemass/basedriveconnection
Slide 16 Common RBE 2/RBAR Uses • RBE 2 or. RBARbetween 2 GRIDs – “ Weld” 2 differentpartstogether • 6 DOFconnection – “ Bolt” 2 differentpartstogether • 3 DOFconnection • RBE 2 – “ Spider”or“wagonwheel”connections – Largemass/basedriveconnection
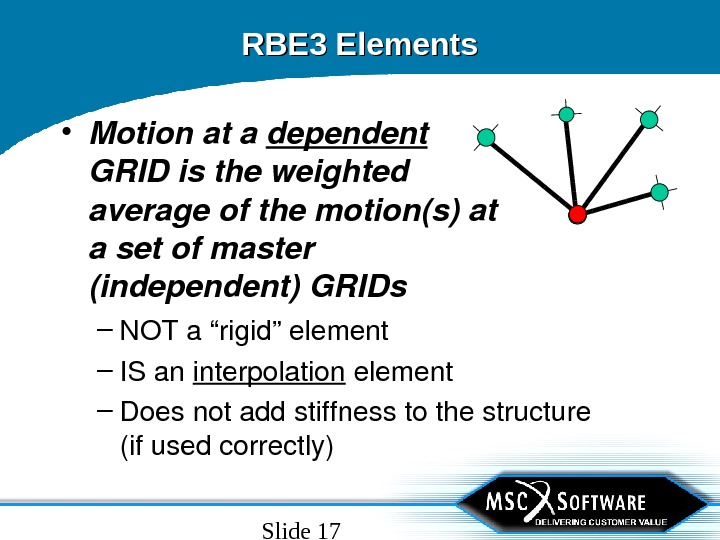
 Slide 17 RBE 3 Elements – NOTa“rigid”element – ISan interpolation element – Doesnotaddstiffnesstothestructure (ifusedcorrectly) • Motionata dependent GRIDistheweighted averageofthemotion(s)at asetofmaster (independent)GRIDs
Slide 17 RBE 3 Elements – NOTa“rigid”element – ISan interpolation element – Doesnotaddstiffnesstothestructure (ifusedcorrectly) • Motionata dependent GRIDistheweighted averageofthemotion(s)at asetofmaster (independent)GRIDs
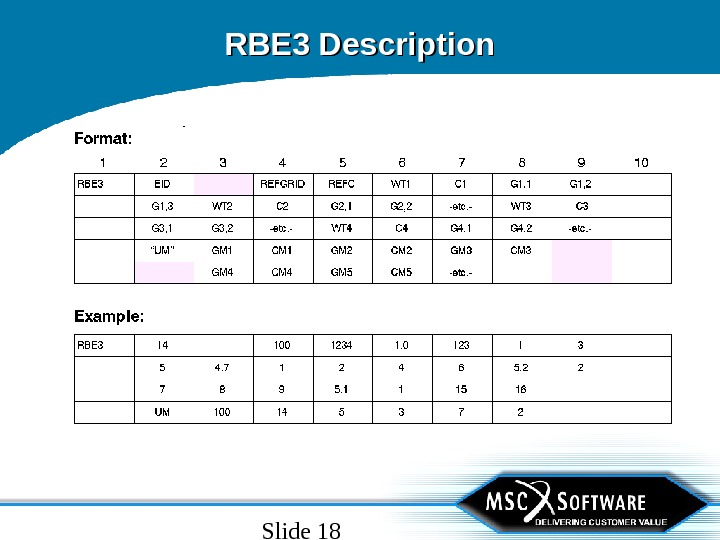
 Slide 18 RBE 3 Description
Slide 18 RBE 3 Description
 Slide 19 RBE 3 Description • Bydefault, thereferencegrid. DOFwill bethedependent. DOF • Numberofdependent. DOFisequalto thenumberof. DOFonthe. REFCfield • Dependent. DOFcannotbe. SPC’d, OMITted, SUPORTedorbedependent onother. RBE/MPCelements
Slide 19 RBE 3 Description • Bydefault, thereferencegrid. DOFwill bethedependent. DOF • Numberofdependent. DOFisequalto thenumberof. DOFonthe. REFCfield • Dependent. DOFcannotbe. SPC’d, OMITted, SUPORTedorbedependent onother. RBE/MPCelements
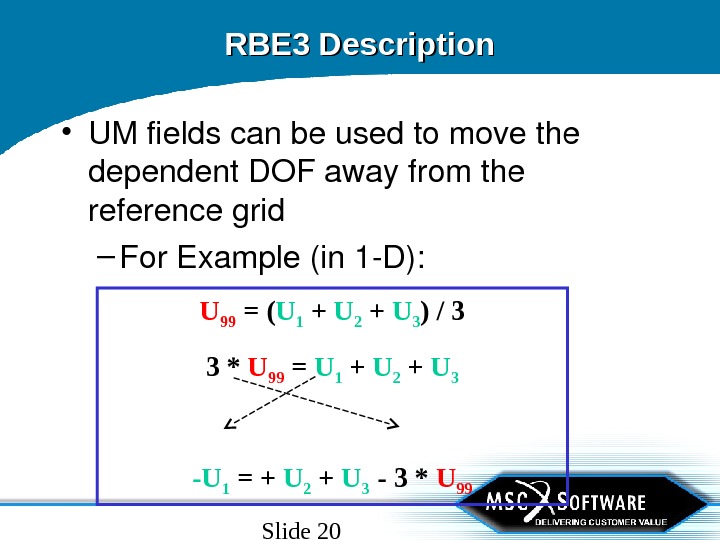
 Slide 20 U 99 = ( U 1 + U 2 + U 3 ) / 3 3 * U 99 = U 1 + U 2 + U 3 -U 1 = + U 2 + U 3 — 3 * U 99 RBE 3 Description • UMfieldscanbeusedtomovethe dependent. DOFawayfromthe referencegrid – For. Example(in 1 D):
Slide 20 U 99 = ( U 1 + U 2 + U 3 ) / 3 3 * U 99 = U 1 + U 2 + U 3 -U 1 = + U 2 + U 3 — 3 * U 99 RBE 3 Description • UMfieldscanbeusedtomovethe dependent. DOFawayfromthe referencegrid – For. Example(in 1 D):
 Slide 21 RBE 3 Is Not Rigid! • RBE 3 vs. RBE 2 – RBE 3 allowswarping and 3 Deffects – Inthisexample, RBE 2 enforcesbeam theory(planesectionsremainplanar) RBE 3 R
Slide 21 RBE 3 Is Not Rigid! • RBE 3 vs. RBE 2 – RBE 3 allowswarping and 3 Deffects – Inthisexample, RBE 2 enforcesbeam theory(planesectionsremainplanar) RBE 3 R
 Slide 22 RBE 3: How it Works? • Forces/momentsappliedatreference gridaredistributedtothemastergrids insamemannerasclassicalboltpattern analysis – Step 1: Appliedloadsaretransferredtothe CGoftheweightedgridgroupusingan equivalent. Force/Moment – Step 2: Appliedloadsat. CGtransferredto mastergridsaccordingtoeachgrid’s weightingfactor
Slide 22 RBE 3: How it Works? • Forces/momentsappliedatreference gridaredistributedtothemastergrids insamemannerasclassicalboltpattern analysis – Step 1: Appliedloadsaretransferredtothe CGoftheweightedgridgroupusingan equivalent. Force/Moment – Step 2: Appliedloadsat. CGtransferredto mastergridsaccordingtoeachgrid’s weightingfactor
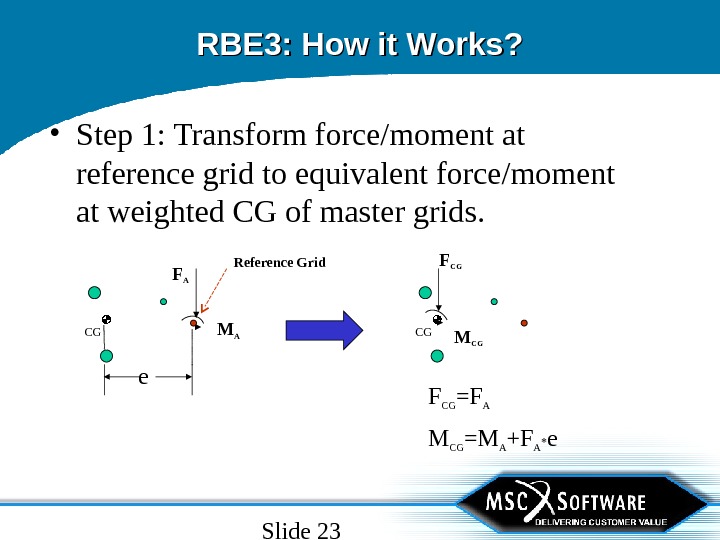
 Slide 23 RBE 3: How it Works? • Step 1: Transform force/moment at reference grid to equivalent force/moment at weighted CG of master grids. M CG =M A +F A * e. F CG =F ACG F CG M CGF A M AReference Grid e. CG
Slide 23 RBE 3: How it Works? • Step 1: Transform force/moment at reference grid to equivalent force/moment at weighted CG of master grids. M CG =M A +F A * e. F CG =F ACG F CG M CGF A M AReference Grid e. CG
 Slide 24 RBE 3: How it Works? • Step 2: Moveloadsat. CGtomaster gridsaccordingtotheirweighting values. – Forceat. CGdividedamongstmastergrids accordingtoweightingfactors. Wi – Momentat. CGmappedasequivalentforce couplesonmastergridsaccordingto weightingfactors. W i
Slide 24 RBE 3: How it Works? • Step 2: Moveloadsat. CGtomaster gridsaccordingtotheirweighting values. – Forceat. CGdividedamongstmastergrids accordingtoweightingfactors. Wi – Momentat. CGmappedasequivalentforce couplesonmastergridsaccordingto weightingfactors. W i
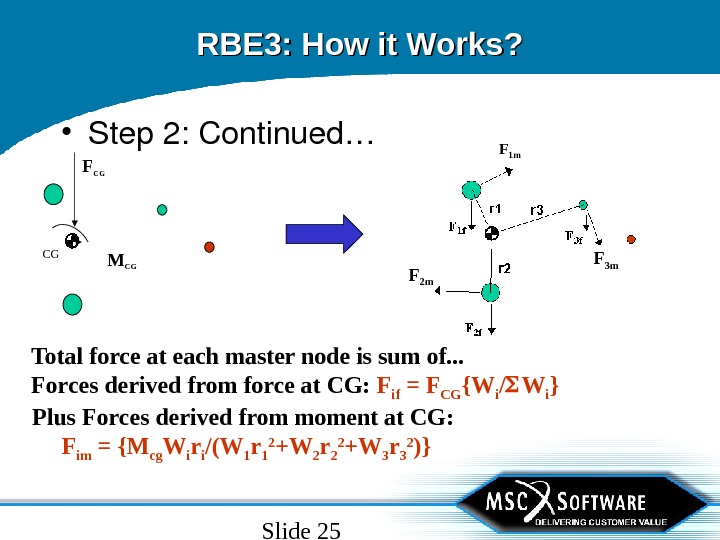
 Slide 25 RBE 3: How it Works? • Step 2: Continued… CG F CG M CG Total force at each master node is sum of. . . Forces derived from force at CG: F if = F CG {W i / W i }F 1 m F 3 m F 2 m Plus Forces derived from moment at CG: F im = {M cg W i r i /(W 1 r 1 2 +W 2 r 2 2 +W 3 r 3 2 )}
Slide 25 RBE 3: How it Works? • Step 2: Continued… CG F CG M CG Total force at each master node is sum of. . . Forces derived from force at CG: F if = F CG {W i / W i }F 1 m F 3 m F 2 m Plus Forces derived from moment at CG: F im = {M cg W i r i /(W 1 r 1 2 +W 2 r 2 2 +W 3 r 3 2 )}
 Slide 26 RBE 3: How it Works? • Massesonreferencegridaresmeared tothemastergridssimilartohowforces aredistributed – Massisdistributedtothemastergridsaccording totheirweightingfactors – Motionofreferencemassresultsininertialforce thatgetstransferredtomastergrids – Referencenodeinertialforceisdistributedin samemanneraswhenstaticforceisappliedto thereferencegrid.
Slide 26 RBE 3: How it Works? • Massesonreferencegridaresmeared tothemastergridssimilartohowforces aredistributed – Massisdistributedtothemastergridsaccording totheirweightingfactors – Motionofreferencemassresultsininertialforce thatgetstransferredtomastergrids – Referencenodeinertialforceisdistributedin samemanneraswhenstaticforceisappliedto thereferencegrid.
 Slide 27 Example 1 • RBE 3 distributionofloadswhenforceat referencegridat. CGpassesthrough CGofmastergrids
Slide 27 Example 1 • RBE 3 distributionofloadswhenforceat referencegridat. CGpassesthrough CGofmastergrids
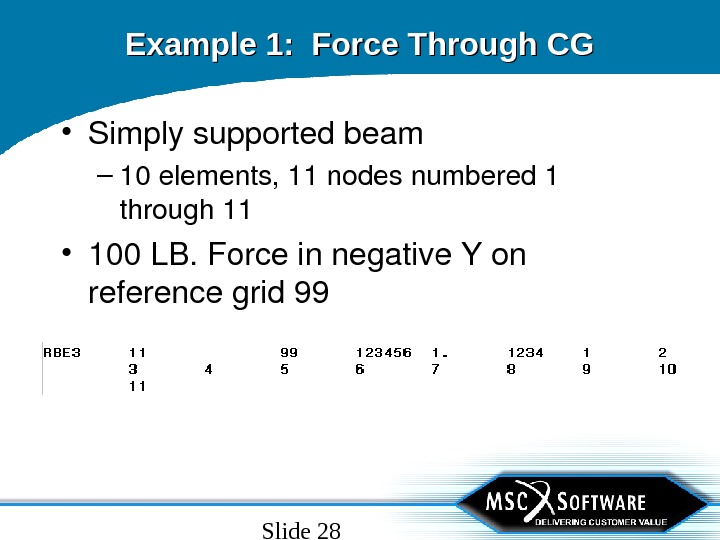
 Slide 28 Example 1: Force Through CG • Simplysupportedbeam – 10 elements, 11 nodesnumbered 1 through 11 • 100 LB. Forceinnegative. Yon referencegrid
Slide 28 Example 1: Force Through CG • Simplysupportedbeam – 10 elements, 11 nodesnumbered 1 through 11 • 100 LB. Forceinnegative. Yon referencegrid
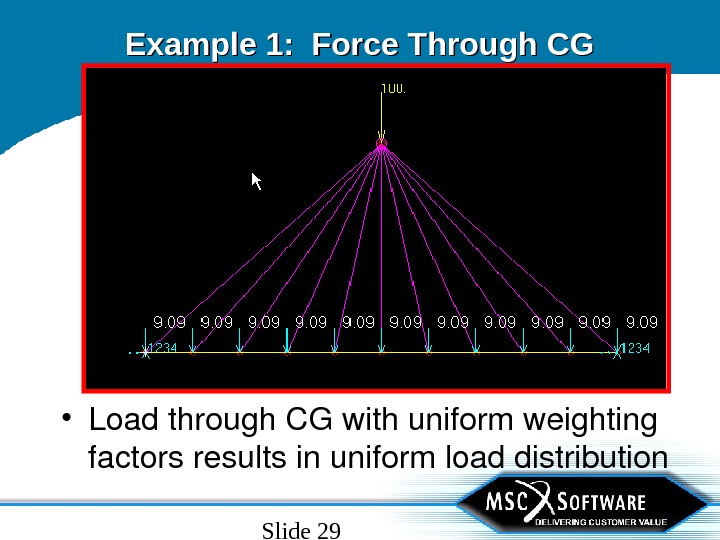
 Slide 29 Example 1: Force Through CG • Loadthrough. CGwithuniformweighting factorsresultsinuniformloaddistribution
Slide 29 Example 1: Force Through CG • Loadthrough. CGwithuniformweighting factorsresultsinuniformloaddistribution
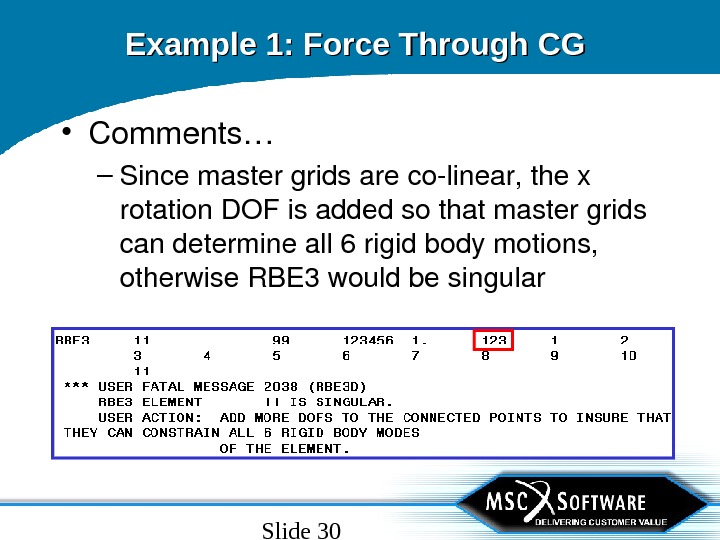
 Slide 30 Example 1: Force Through CG • Comments… – Sincemastergridsarecolinear, thex rotation. DOFisaddedsothatmastergrids candetermineall 6 rigidbodymotions, otherwise. RBE 3 wouldbesingular
Slide 30 Example 1: Force Through CG • Comments… – Sincemastergridsarecolinear, thex rotation. DOFisaddedsothatmastergrids candetermineall 6 rigidbodymotions, otherwise. RBE 3 wouldbesingular
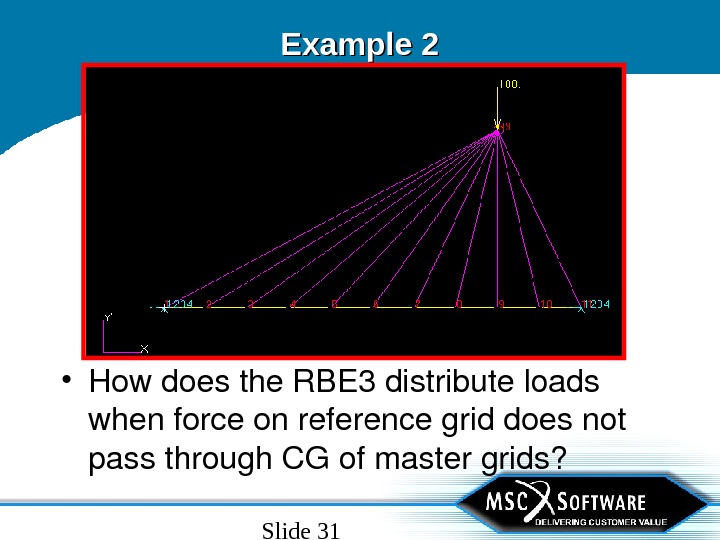
 Slide 31 Example 2 • Howdoesthe. RBE 3 distributeloads whenforceonreferencegriddoesnot passthrough. CGofmastergrids?
Slide 31 Example 2 • Howdoesthe. RBE 3 distributeloads whenforceonreferencegriddoesnot passthrough. CGofmastergrids?
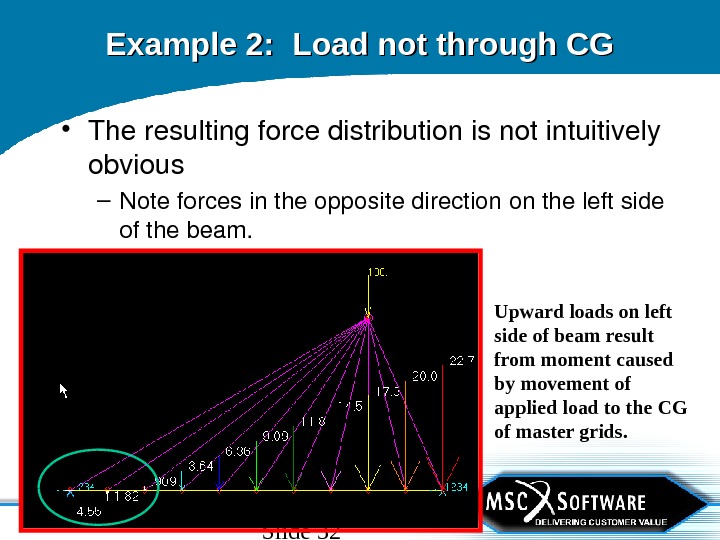
 Slide 32 Example 2: Load not through CG • Theresultingforcedistributionisnotintuitively obvious – Noteforcesintheoppositedirectionontheleftside ofthebeam. Upward loads on left side of beam result from moment caused by movement of applied load to the CG of master grids.
Slide 32 Example 2: Load not through CG • Theresultingforcedistributionisnotintuitively obvious – Noteforcesintheoppositedirectionontheleftside ofthebeam. Upward loads on left side of beam result from moment caused by movement of applied load to the CG of master grids.
 Slide 33 Example 3 • Useofweightingfactorstogenerate realisticloaddistribution: 100 LB. transverseloadon 3 Dbeam.
Slide 33 Example 3 • Useofweightingfactorstogenerate realisticloaddistribution: 100 LB. transverseloadon 3 Dbeam.
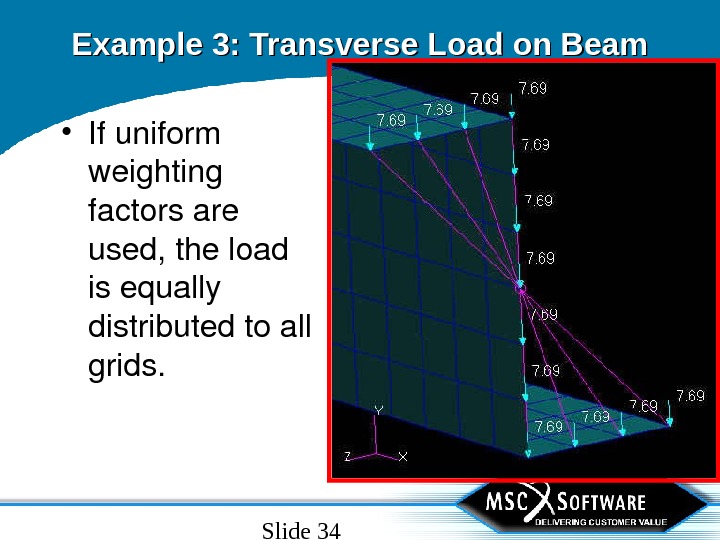
 Slide 34 Example 3: Transverse Load on Beam • Ifuniform weighting factorsare used, theload isequally distributedtoall grids.
Slide 34 Example 3: Transverse Load on Beam • Ifuniform weighting factorsare used, theload isequally distributedtoall grids.
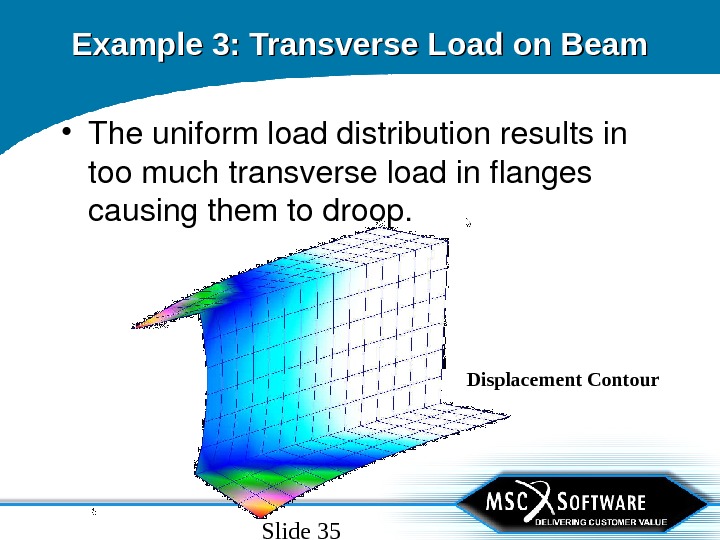
 Slide 35 Example 3: Transverse Load on Beam Displacement Contour • Theuniformloaddistributionresultsin toomuchtransverseloadinflanges causingthemtodroop.
Slide 35 Example 3: Transverse Load on Beam Displacement Contour • Theuniformloaddistributionresultsin toomuchtransverseloadinflanges causingthemtodroop.
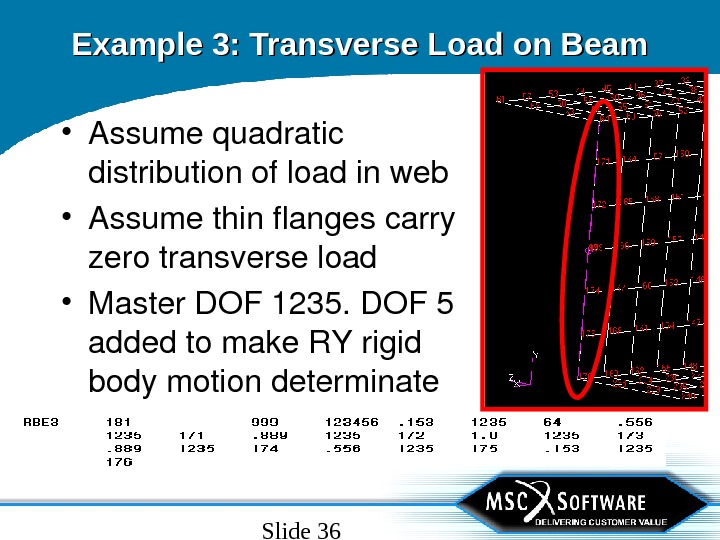
 Slide 36 Example 3: Transverse Load on Beam • Assumequadratic distributionofloadinweb • Assumethinflangescarry zerotransverseload • Master. DOF 1235. DOF 5 addedtomake. RYrigid bodymotiondeterminate
Slide 36 Example 3: Transverse Load on Beam • Assumequadratic distributionofloadinweb • Assumethinflangescarry zerotransverseload • Master. DOF 1235. DOF 5 addedtomake. RYrigid bodymotiondeterminate
 Slide 37 • Displacementswithquadraticweighting factorsvirtuallyequivalenttothosefrom RBE 2(Beam. Theory), butdonot impose“planesectionsremainplanar” asdoes. RBE 2. Example 3: Transverse Load on Beam
Slide 37 • Displacementswithquadraticweighting factorsvirtuallyequivalenttothosefrom RBE 2(Beam. Theory), butdonot impose“planesectionsremainplanar” asdoes. RBE 2. Example 3: Transverse Load on Beam
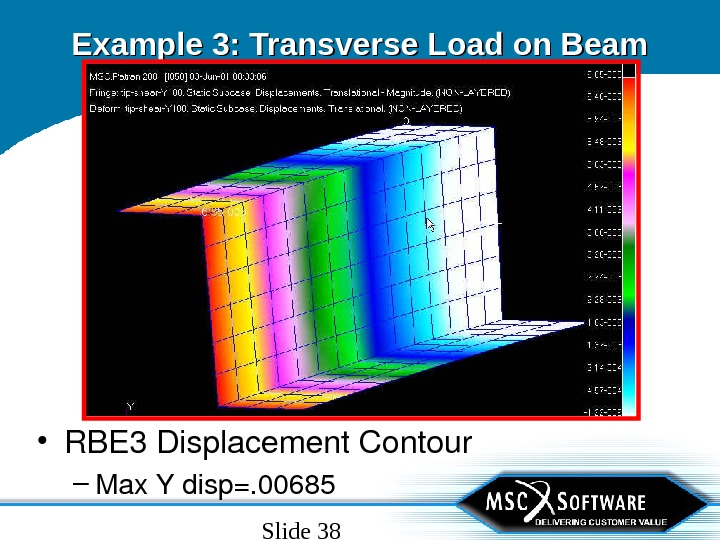
 Slide 38 Example 3: Transverse Load on Beam • RBE 3 Displacement. Contour – Max. Ydisp=.
Slide 38 Example 3: Transverse Load on Beam • RBE 3 Displacement. Contour – Max. Ydisp=.
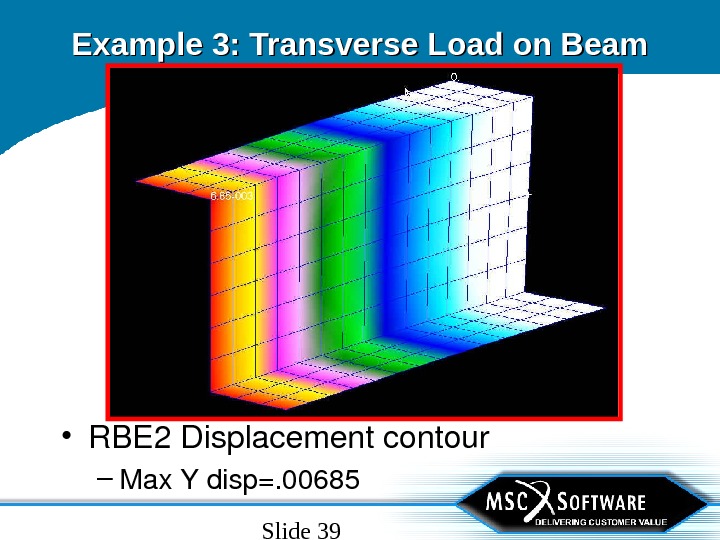
 Slide 39 Example 3: Transverse Load on Beam • RBE 2 Displacementcontour – Max. Ydisp=.
Slide 39 Example 3: Transverse Load on Beam • RBE 2 Displacementcontour – Max. Ydisp=.
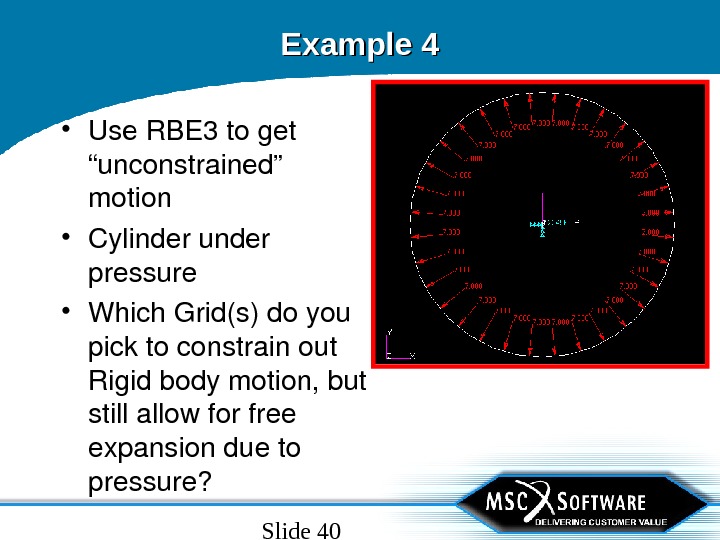
 Slide 40 Example 4 • Use. RBE 3 toget “unconstrained” motion • Cylinderunder pressure • Which. Grid(s)doyou picktoconstrainout Rigidbodymotion, but stillallowforfree expansiondueto pressure?
Slide 40 Example 4 • Use. RBE 3 toget “unconstrained” motion • Cylinderunder pressure • Which. Grid(s)doyou picktoconstrainout Rigidbodymotion, but stillallowforfree expansiondueto pressure?
 Slide 41 Example 4: Use RBE 3 for Unconstrained Motion • Solution: – Use. RBE 3 – Movedependent. DOFfromreferencegridtoselectedmaster gridswith. UMoptionon. RBE 3(otherwise, referencegrid cannotbe. SPC’d) – Apply. SPCtoreferencegrid
Slide 41 Example 4: Use RBE 3 for Unconstrained Motion • Solution: – Use. RBE 3 – Movedependent. DOFfromreferencegridtoselectedmaster gridswith. UMoptionon. RBE 3(otherwise, referencegrid cannotbe. SPC’d) – Apply. SPCtoreferencegrid
 Slide 42 Example 4: Use RBE 3 for Unconstrained Motion • Sincereferencegridhas 6 DOF, we mustassign 6“UM”DOFtoasetof mastergrids – Pick 3 points, forminganicetrianglefor bestnumericalconditioning – Selectatotalof 6 DOFoverthethree. UM gridstodeterminethe 6 rigidbodymotions ofthe. RBE 3 – Note: “M”isthe. NASTRANDOFsetname fordependent. DO
Slide 42 Example 4: Use RBE 3 for Unconstrained Motion • Sincereferencegridhas 6 DOF, we mustassign 6“UM”DOFtoasetof mastergrids – Pick 3 points, forminganicetrianglefor bestnumericalconditioning – Selectatotalof 6 DOFoverthethree. UM gridstodeterminethe 6 rigidbodymotions ofthe. RBE 3 – Note: “M”isthe. NASTRANDOFsetname fordependent. DO
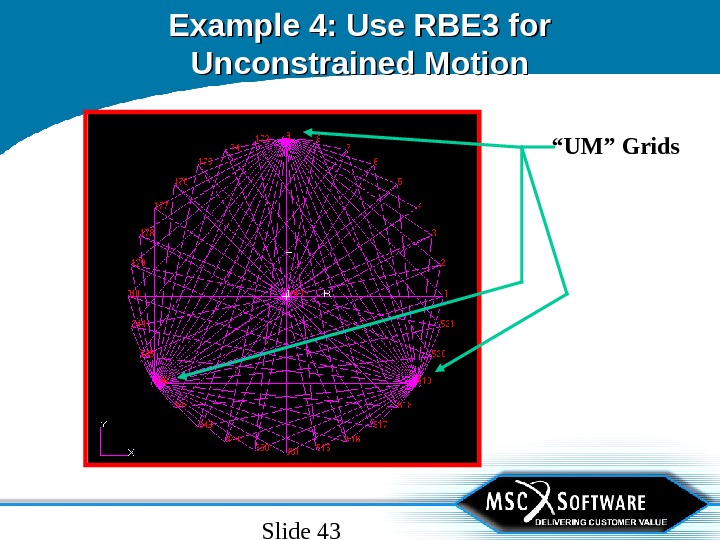
 Slide 43 Example 4: Use RBE 3 for Unconstrained Motion “ UM” Grids
Slide 43 Example 4: Use RBE 3 for Unconstrained Motion “ UM” Grids
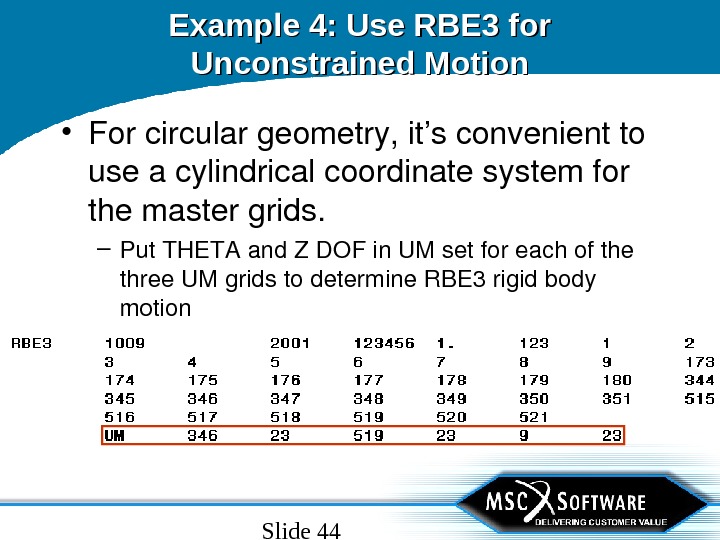
 Slide 44 Example 4: Use RBE 3 for Unconstrained Motion • Forcirculargeometry, it’sconvenientto useacylindricalcoordinatesystemfor themastergrids. – Put. THETAand. ZDOFin. UMsetforeachofthe three. UMgridstodetermine. RBE 3 rigidbody motion
Slide 44 Example 4: Use RBE 3 for Unconstrained Motion • Forcirculargeometry, it’sconvenientto useacylindricalcoordinatesystemfor themastergrids. – Put. THETAand. ZDOFin. UMsetforeachofthe three. UMgridstodetermine. RBE 3 rigidbody motion
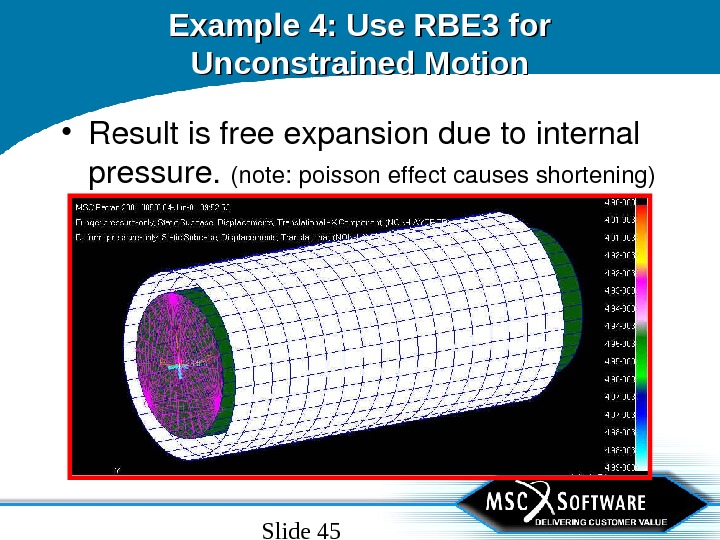
 Slide 45 Example 4: Use RBE 3 for Unconstrained Motion • Resultisfreeexpansionduetointernal pressure. (note: poissoneffectcausesshortening)
Slide 45 Example 4: Use RBE 3 for Unconstrained Motion • Resultisfreeexpansionduetointernal pressure. (note: poissoneffectcausesshortening)
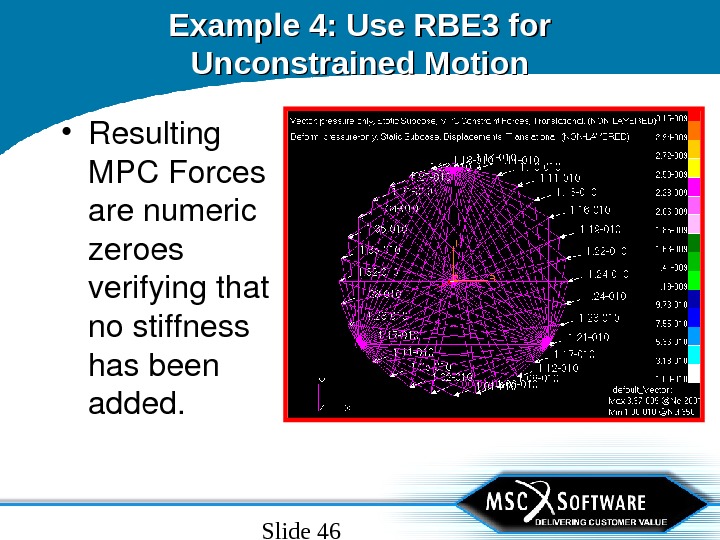
 Slide 46 Example 4: Use RBE 3 for Unconstrained Motion • Resulting MPCForces arenumeric zeroes verifyingthat nostiffness hasbeen added.
Slide 46 Example 4: Use RBE 3 for Unconstrained Motion • Resulting MPCForces arenumeric zeroes verifyingthat nostiffness hasbeen added.
 Slide 47 Example 5 • Connect 3 Dmodeltostickmodel • 3 Dmodelwith 7 psiinternalpressure • Use. RBE 3 insteadof. RBE 2 sothat 3 D modelcanexpandnaturallyatinterface. – RBE 3 willalsoallowwarpingandother 3 D effectsattheinterface.
Slide 47 Example 5 • Connect 3 Dmodeltostickmodel • 3 Dmodelwith 7 psiinternalpressure • Use. RBE 3 insteadof. RBE 2 sothat 3 D modelcanexpandnaturallyatinterface. – RBE 3 willalsoallowwarpingandother 3 D effectsattheinterface.
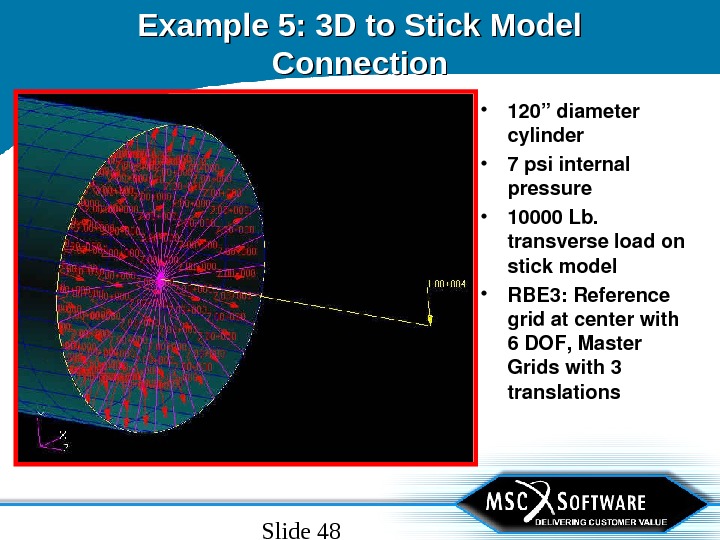
 Slide 48 Example 5: 3 D to Stick Model Connection • 120”diameter cylinder • 7 psiinternal pressure • 10000 Lb. transverseloadon stickmodel • RBE 3: Reference gridatcenterwith 6 DOF, Master Gridswith 3 translations
Slide 48 Example 5: 3 D to Stick Model Connection • 120”diameter cylinder • 7 psiinternal pressure • 10000 Lb. transverseloadon stickmodel • RBE 3: Reference gridatcenterwith 6 DOF, Master Gridswith 3 translations
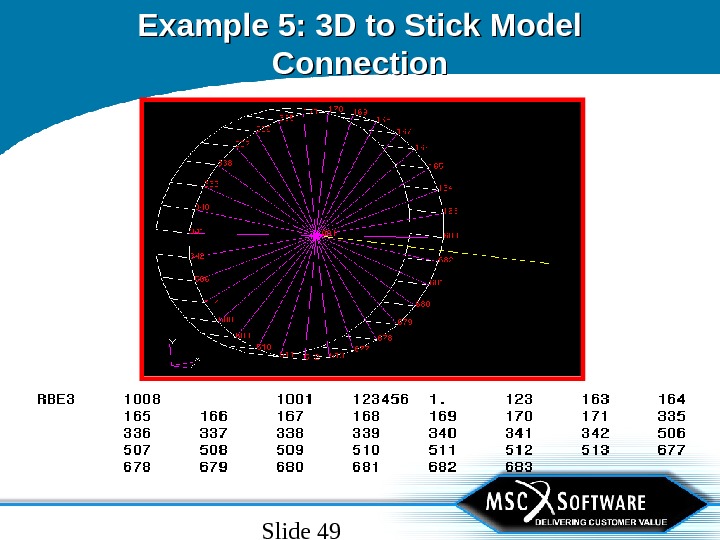
 Slide 49 Example 5: 3 D to Stick Model Connection
Slide 49 Example 5: 3 D to Stick Model Connection
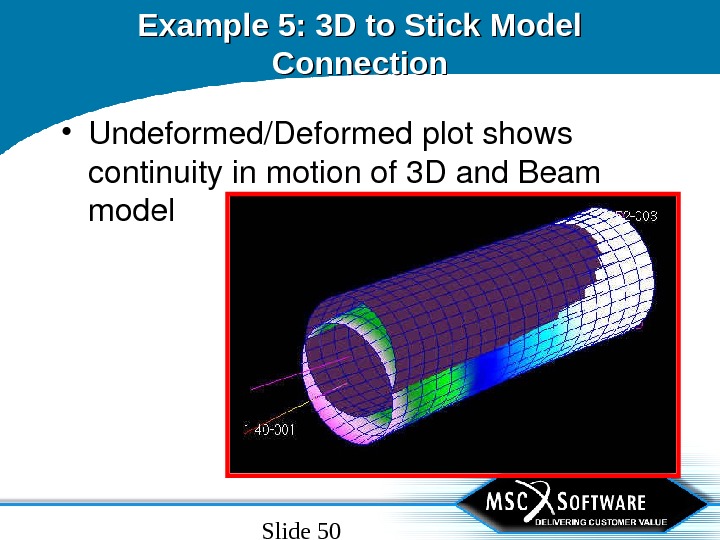
 Slide 50 Example 5: 3 D to Stick Model Connection • Undeformed/Deformedplotshows continuityinmotionof 3 Dand. Beam model
Slide 50 Example 5: 3 D to Stick Model Connection • Undeformed/Deformedplotshows continuityinmotionof 3 Dand. Beam model
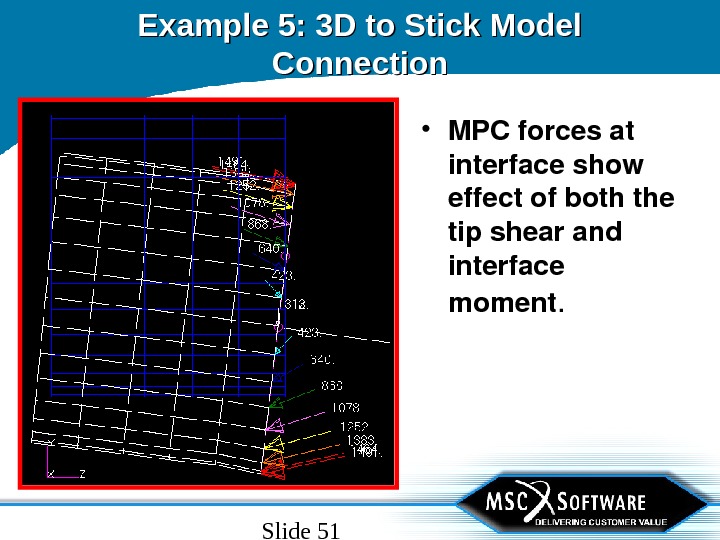
 Slide 51 Example 5: 3 D to Stick Model Connection • MPCforcesat interfaceshow effectofboththe tipshearand interface moment.
Slide 51 Example 5: 3 D to Stick Model Connection • MPCforcesat interfaceshow effectofboththe tipshearand interface moment.
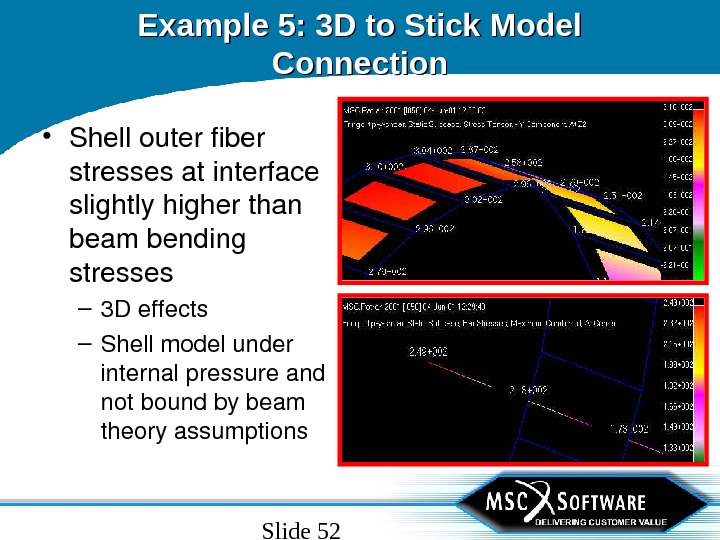
 Slide 52 Example 5: 3 D to Stick Model Connection • Shellouterfiber stressesatinterface slightlyhigherthan beambending stresses – 3 Deffects – Shellmodelunder internalpressureand notboundbybeam theoryassumptions
Slide 52 Example 5: 3 D to Stick Model Connection • Shellouterfiber stressesatinterface slightlyhigherthan beambending stresses – 3 Deffects – Shellmodelunder internalpressureand notboundbybeam theoryassumptions
 Slide 53 Example 6 • Use. RBE 3 tosee“beam”typemodes fromacomplexmodel • Sometimesit’sdifficulttoidentifyand describemodesofcomplexstructures • Solution: – Connectcomplexstructuredownto centerlinegridswith. RBE 3. – Connectcenterlinegridswith. PLOTELs
Slide 53 Example 6 • Use. RBE 3 tosee“beam”typemodes fromacomplexmodel • Sometimesit’sdifficulttoidentifyand describemodesofcomplexstructures • Solution: – Connectcomplexstructuredownto centerlinegridswith. RBE 3. – Connectcenterlinegridswith. PLOTELs
 Slide 54 Example 6: Using RBE 3 to Visualize “Beam” Modes • Genericenginecourtesyof. Pratt& Whitney
Slide 54 Example 6: Using RBE 3 to Visualize “Beam” Modes • Genericenginecourtesyof. Pratt& Whitney
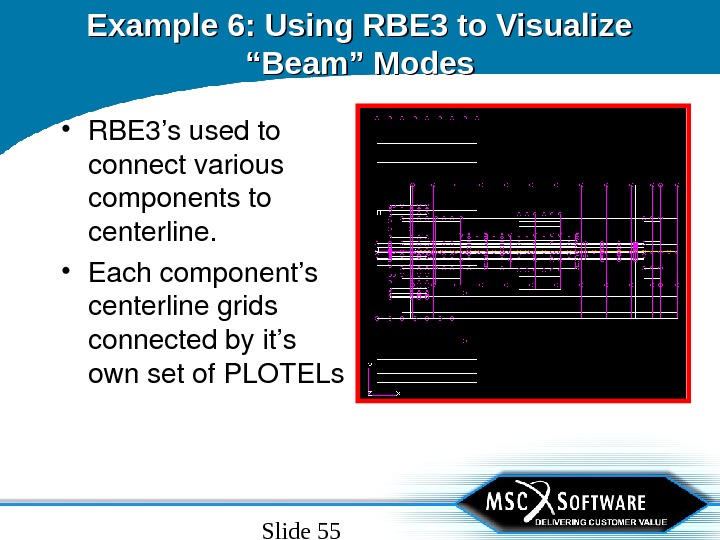
 Slide 55 Example 6: Using RBE 3 to Visualize “Beam” Modes • RBE 3’susedto connectvarious componentsto centerline. • Eachcomponent’s centerlinegrids connectedbyit’s ownsetof. PLOTELs
Slide 55 Example 6: Using RBE 3 to Visualize “Beam” Modes • RBE 3’susedto connectvarious componentsto centerline. • Eachcomponent’s centerlinegrids connectedbyit’s ownsetof. PLOTELs
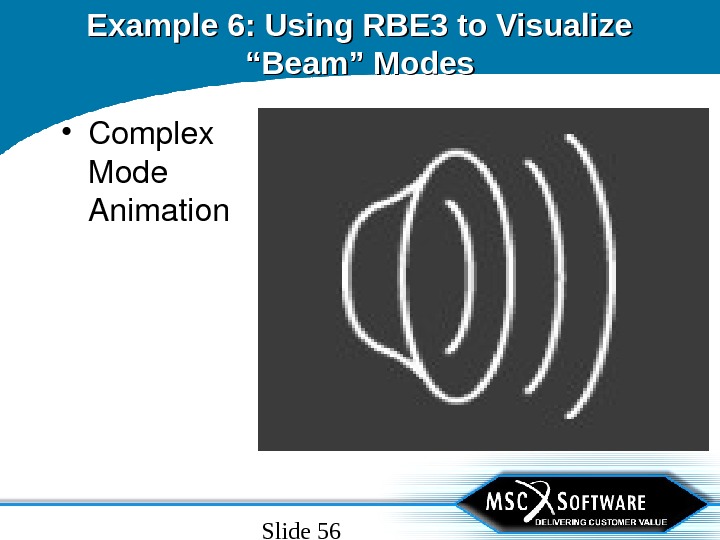
 Slide 56 Example 6: Using RBE 3 to Visualize “Beam” Modes • Complex Mode Animation
Slide 56 Example 6: Using RBE 3 to Visualize “Beam” Modes • Complex Mode Animation
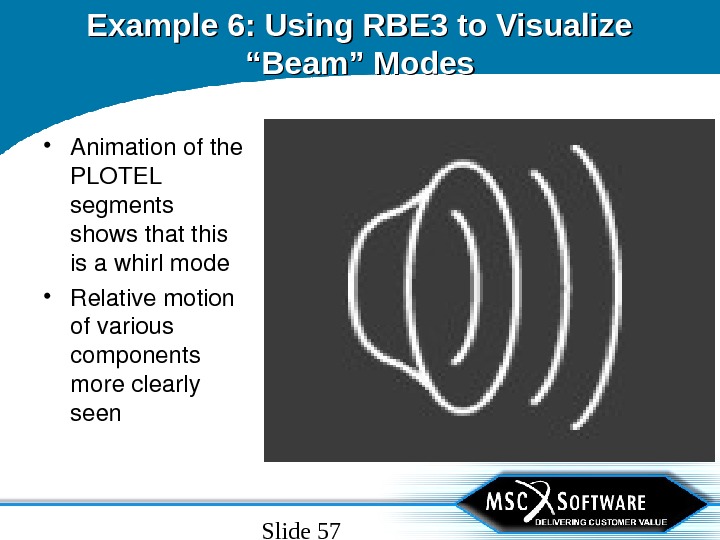
 Slide 57 Example 6: Using RBE 3 to Visualize “Beam” Modes • Animationofthe PLOTEL segments showsthatthis isawhirlmode • Relativemotion ofvarious components moreclearly seen
Slide 57 Example 6: Using RBE 3 to Visualize “Beam” Modes • Animationofthe PLOTEL segments showsthatthis isawhirlmode • Relativemotion ofvarious components moreclearly seen
 Slide 58 Example 7 • Use. RBE 3 toconnectincompatible elements – Beamtoplate – Beamtosolid – Platetosolid • Alternativeto. RSSCON
Slide 58 Example 7 • Use. RBE 3 toconnectincompatible elements – Beamtoplate – Beamtosolid – Platetosolid • Alternativeto. RSSCON
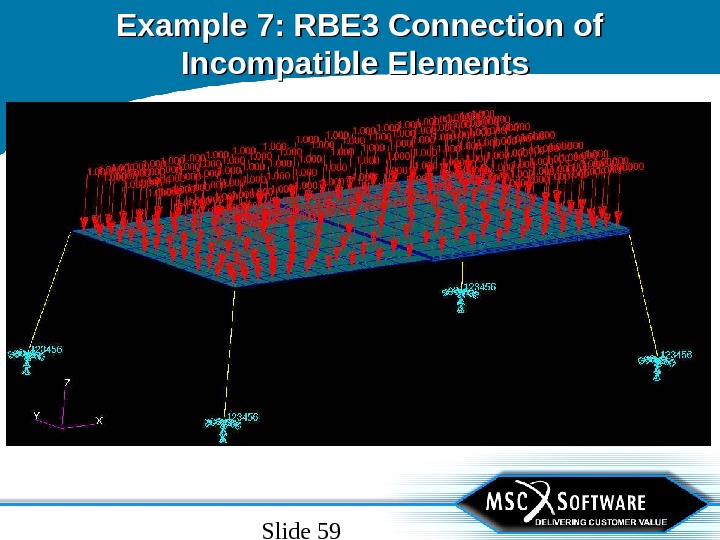
 Slide 59 Example 7: RBE 3 Connection of Incompatible Elements
Slide 59 Example 7: RBE 3 Connection of Incompatible Elements
 Slide 60 Example 7: RBE 3 Connection of Incompatible Elements • Use. RBE 3 toconnectbeamstoplates attwocorners • Use. RBE 3 toconnectbeamstosolids attwocorners • Use. RBE 3 toconnectplatestosolid – Platethicknessissameassolidthickness inthisexample
Slide 60 Example 7: RBE 3 Connection of Incompatible Elements • Use. RBE 3 toconnectbeamstoplates attwocorners • Use. RBE 3 toconnectbeamstosolids attwocorners • Use. RBE 3 toconnectplatestosolid – Platethicknessissameassolidthickness inthisexample
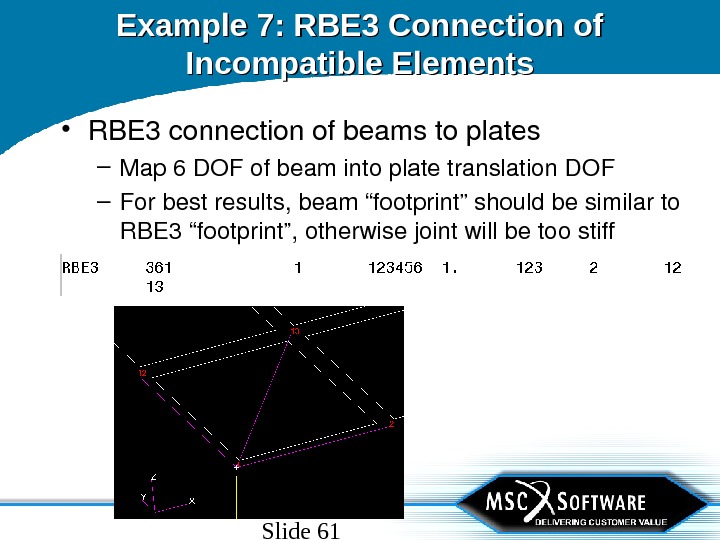
 Slide 61 Example 7: RBE 3 Connection of Incompatible Elements • RBE 3 connectionofbeamstoplates – Map 6 DOFofbeamintoplatetranslation. DOF – Forbestresults, beam“footprint”shouldbesimilarto RBE 3“footprint”, otherwisejointwillbetoostiff
Slide 61 Example 7: RBE 3 Connection of Incompatible Elements • RBE 3 connectionofbeamstoplates – Map 6 DOFofbeamintoplatetranslation. DOF – Forbestresults, beam“footprint”shouldbesimilarto RBE 3“footprint”, otherwisejointwillbetoostiff
 Slide 62 Example 7: RBE 3 Connection of Incompatible Elements • RBE 3 connectionof beamstosolids – Map 6 DOFofbeaminto solidtranslation. DOF – Forbestresults, beam “footprint”shouldbesimilar to. RBE 3“footprint”, otherwisejointwillbetoo stiff
Slide 62 Example 7: RBE 3 Connection of Incompatible Elements • RBE 3 connectionof beamstosolids – Map 6 DOFofbeaminto solidtranslation. DOF – Forbestresults, beam “footprint”shouldbesimilar to. RBE 3“footprint”, otherwisejointwillbetoo stiff
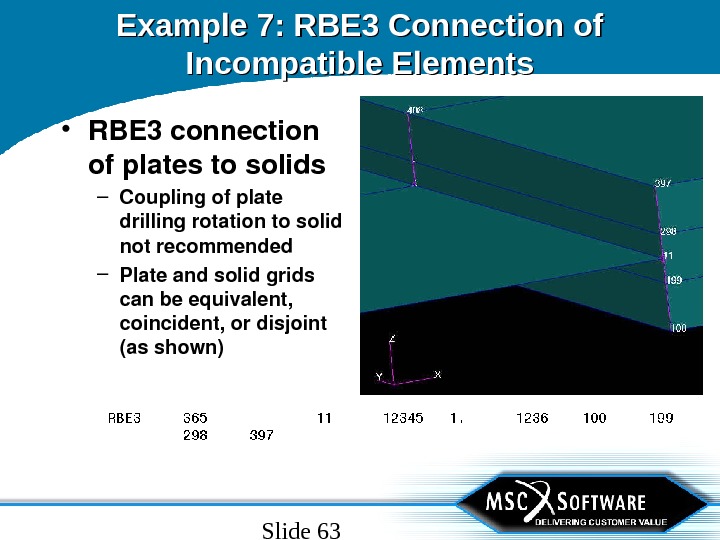
 Slide 63 Example 7: RBE 3 Connection of Incompatible Elements • RBE 3 connection ofplatestosolids – Couplingofplate drillingrotationtosolid notrecommended – Plateandsolidgrids canbeequivalent, coincident, ordisjoint (asshown)
Slide 63 Example 7: RBE 3 Connection of Incompatible Elements • RBE 3 connection ofplatestosolids – Couplingofplate drillingrotationtosolid notrecommended – Plateandsolidgrids canbeequivalent, coincident, ordisjoint (asshown)
 Slide 64 Example 7: RBE 3 Connection of Incompatible Elements • Deformationcontoursshowcontinuityat RBE 3 interfaces
Slide 64 Example 7: RBE 3 Connection of Incompatible Elements • Deformationcontoursshowcontinuityat RBE 3 interfaces
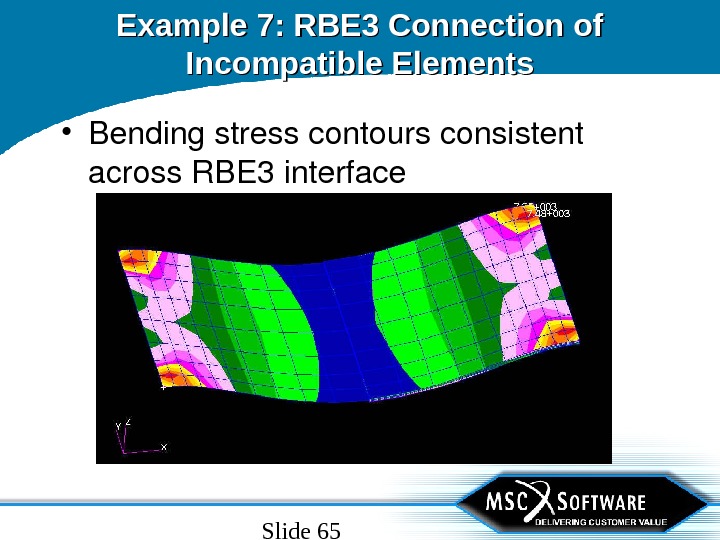
 Slide 65 Example 7: RBE 3 Connection of Incompatible Elements • Bendingstresscontoursconsistent across. RBE 3 interface
Slide 65 Example 7: RBE 3 Connection of Incompatible Elements • Bendingstresscontoursconsistent across. RBE 3 interface
 Slide 66 RBE 3 Usage Guidelines • Donotspecifyrotational. DOFfor mastergridsexceptwhennecessaryto avoidsingularitycausedbyalinearset ofmastergrids • Usingrotational. DOFonmastergrids canresultinimplausibleresults(see nexttwoslides)
Slide 66 RBE 3 Usage Guidelines • Donotspecifyrotational. DOFfor mastergridsexceptwhennecessaryto avoidsingularitycausedbyalinearset ofmastergrids • Usingrotational. DOFonmastergrids canresultinimplausibleresults(see nexttwoslides)
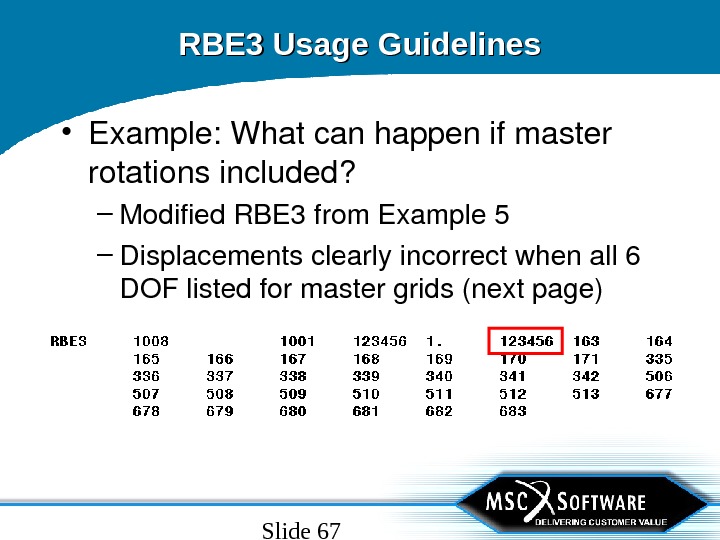
 Slide 67 RBE 3 Usage Guidelines • Example: Whatcanhappenifmaster rotationsincluded? – Modified. RBE 3 from. Example 5 – Displacementsclearlyincorrectwhenall 6 DOFlistedformastergrids(nextpage)
Slide 67 RBE 3 Usage Guidelines • Example: Whatcanhappenifmaster rotationsincluded? – Modified. RBE 3 from. Example 5 – Displacementsclearlyincorrectwhenall 6 DOFlistedformastergrids(nextpage)
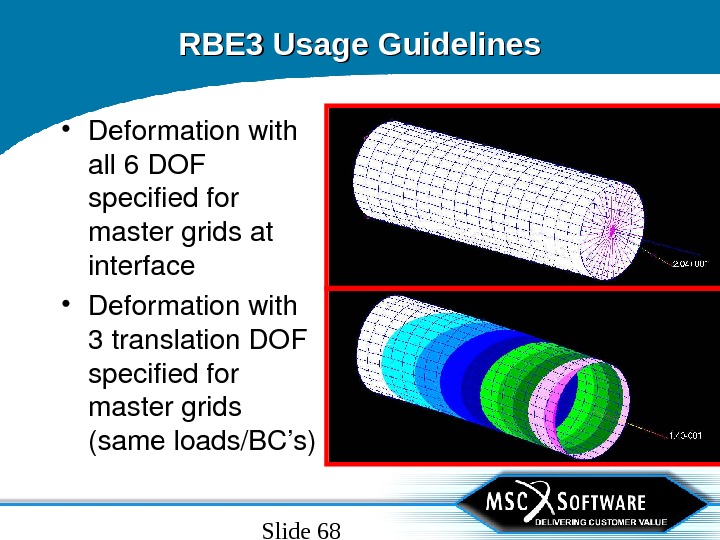
 Slide 68 RBE 3 Usage Guidelines • Deformationwith all 6 DOF specifiedfor mastergridsat interface • Deformationwith 3 translation. DOF specifiedfor mastergrids (sameloads/BC’s)
Slide 68 RBE 3 Usage Guidelines • Deformationwith all 6 DOF specifiedfor mastergridsat interface • Deformationwith 3 translation. DOF specifiedfor mastergrids (sameloads/BC’s)
 Slide 69 RBE 3 Usage Guidelines • Makecheckrunwith PARAM, CHECKOUT, YES – Section 9. 4. 1 of. MSC. Nastran. Reference. Manual(V 68) – EMHprintoutshouldbenumericzeroes(nogrounding) – No. MAXRATIOerrormessagesfromdecompositionof. R g mm and. R m mm matrices(numericallystable) • Performgroundingcheckofatleast. K GG and. K NN matrix – V 2001: Casecontrolcommand • GROUNDCHECK(SET=(G, N))=YES – V 70. 7 andearlier: • Use. CHECKAaltersfrom. SSSALTERlibrary
Slide 69 RBE 3 Usage Guidelines • Makecheckrunwith PARAM, CHECKOUT, YES – Section 9. 4. 1 of. MSC. Nastran. Reference. Manual(V 68) – EMHprintoutshouldbenumericzeroes(nogrounding) – No. MAXRATIOerrormessagesfromdecompositionof. R g mm and. R m mm matrices(numericallystable) • Performgroundingcheckofatleast. K GG and. K NN matrix – V 2001: Casecontrolcommand • GROUNDCHECK(SET=(G, N))=YES – V 70. 7 andearlier: • Use. CHECKAaltersfrom. SSSALTERlibrary
 Slide 70 RBE 3: Additional Reading • Much. RBE 3 informationhasbeenpostedon MSC’s. Knowledge. Base – http: //www. mechsolutions. com/support/ knowbase /index. html
Slide 70 RBE 3: Additional Reading • Much. RBE 3 informationhasbeenpostedon MSC’s. Knowledge. Base – http: //www. mechsolutions. com/support/ knowbase /index. html
 Slide 71 RBE 3: Additional Reading • Recommended. TANs – TAN#: 2402 RBE 3 The. Interpolation. Element. – TAN#: 3280 RBE 3 ELEMENTCHANGESINVERSION 70. 5, improveddiagnostics – TAN#: 4155 RBE 3 ELEMENTCHANGESINVERSION 70. 7 – TAN#: 4494 Mathematical. Specificationofthe. Modern RBE 3 Element – TAN#: 4497 ANECONOMICALMETHODTOEVALUATE RBE 3 ELEMENTSINLARGESIZEMODELS
Slide 71 RBE 3: Additional Reading • Recommended. TANs – TAN#: 2402 RBE 3 The. Interpolation. Element. – TAN#: 3280 RBE 3 ELEMENTCHANGESINVERSION 70. 5, improveddiagnostics – TAN#: 4155 RBE 3 ELEMENTCHANGESINVERSION 70. 7 – TAN#: 4494 Mathematical. Specificationofthe. Modern RBE 3 Element – TAN#: 4497 ANECONOMICALMETHODTOEVALUATE RBE 3 ELEMENTSINLARGESIZEMODELS
 Slide 72 User-Input based “Rigid” Elements • MPCs – Mostgeneralpurposewaytodefine motionbasedrelationships – Could beusedinplaceof. ALLother. RBEi • Lackofgeometrymakesthisimpractical – Canbechangedbetween. SUBCASEs
Slide 72 User-Input based “Rigid” Elements • MPCs – Mostgeneralpurposewaytodefine motionbasedrelationships – Could beusedinplaceof. ALLother. RBEi • Lackofgeometrymakesthisimpractical – Canbechangedbetween. SUBCASEs
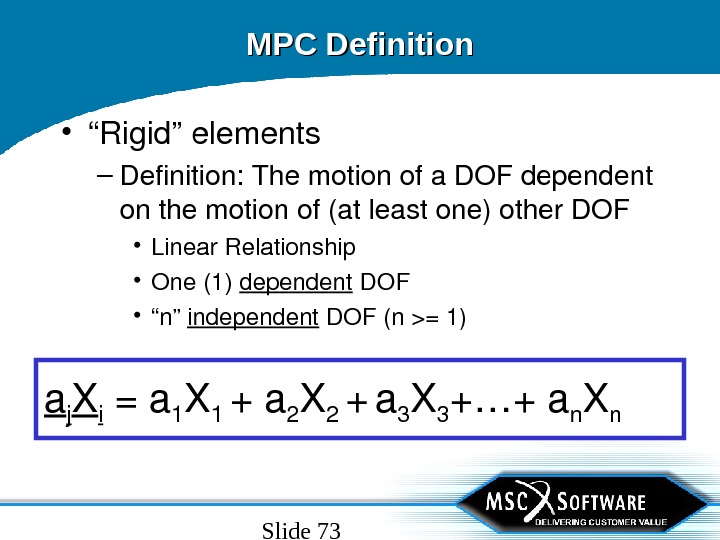
 Slide 73 MPC Definition • “ Rigid”elements – Definition: Themotionofa. DOFdependent onthemotionof(atleastone)other. DOF • Linear. Relationship • One(1) dependent DOF • “ n” independent DOF(n>=1) a j X i =a 1 X 1 +a 2 X 2 + a 3 X 3 +…+a n X n
Slide 73 MPC Definition • “ Rigid”elements – Definition: Themotionofa. DOFdependent onthemotionof(atleastone)other. DOF • Linear. Relationship • One(1) dependent DOF • “ n” independent DOF(n>=1) a j X i =a 1 X 1 +a 2 X 2 + a 3 X 3 +…+a n X n
 Slide 74 General Approach For Use of MPCs • Writeoutdesireddisplacementequality relationshipona per. DOF level – Dependentmotion=( yourequationgoeshere ) 0= U x 2 + U x 1 • Rearrangesolefthandsideiszero • Listdependenttermfirst U x 2 = U x
Slide 74 General Approach For Use of MPCs • Writeoutdesireddisplacementequality relationshipona per. DOF level – Dependentmotion=( yourequationgoeshere ) 0= U x 2 + U x 1 • Rearrangesolefthandsideiszero • Listdependenttermfirst U x 2 = U x
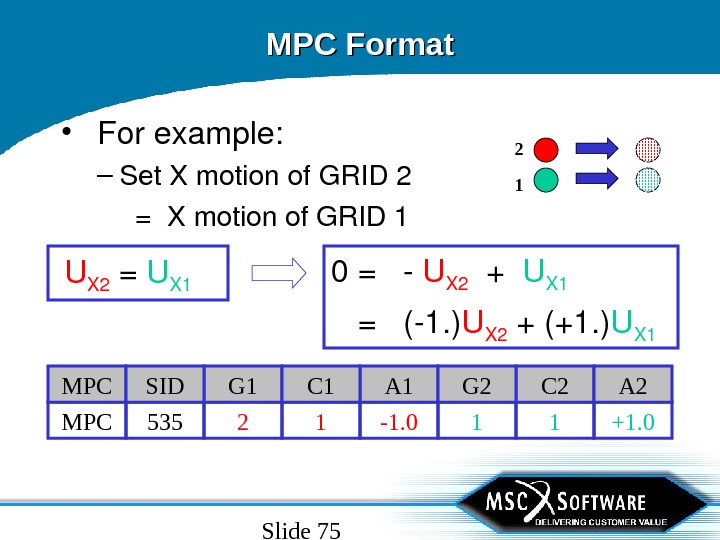
 Slide 75 MPC Format • Forexample: – Set. Xmotionof. GRID 2 =Xmotionof. GRID 1 U X 2 = U X 1 0 = U X 2 + U X 1 = (1. ) U X 2 +(+1. ) U X 1 1 +1. 0 -1. 0 12 1 MPC 535 C 2 A 2 A 1 G 2 G 1 C 1 MPC SI
Slide 75 MPC Format • Forexample: – Set. Xmotionof. GRID 2 =Xmotionof. GRID 1 U X 2 = U X 1 0 = U X 2 + U X 1 = (1. ) U X 2 +(+1. ) U X 1 1 +1. 0 -1. 0 12 1 MPC 535 C 2 A 2 A 1 G 2 G 1 C 1 MPC SI
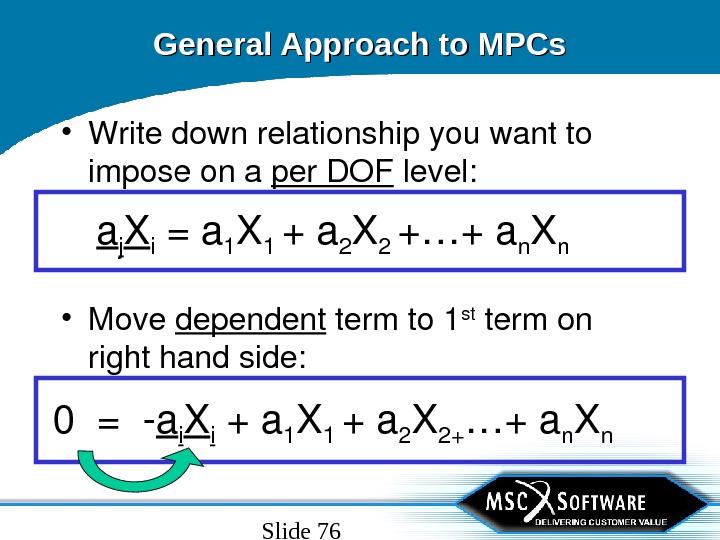
 Slide 76 General Approach to MPCs • Writedownrelationshipyouwantto imposeona per. DOF level: a j X i =a 1 X 1 +a 2 X 2 +…+a n X n 0= a i X i +a 1 X 1 +a 2 X 2+ …+a n X n • Move dependent termto 1 st termon righthandside:
Slide 76 General Approach to MPCs • Writedownrelationshipyouwantto imposeona per. DOF level: a j X i =a 1 X 1 +a 2 X 2 +…+a n X n 0= a i X i +a 1 X 1 +a 2 X 2+ …+a n X n • Move dependent termto 1 st termon righthandside:
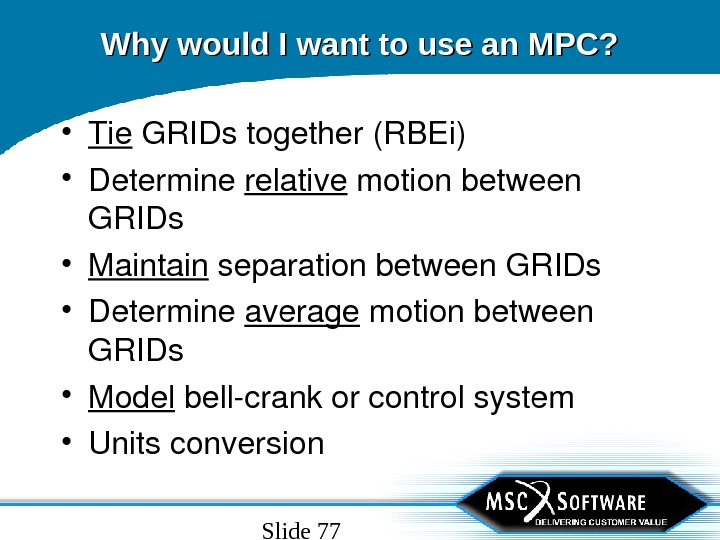
 Slide 77 Why would I want to use an MPC? • Tie GRIDstogether(RBEi) • Determine relative motionbetween GRIDs • Maintain separationbetween. GRIDs • Determine average motionbetween GRIDs • Model bellcrankorcontrolsystem • Unitsconversion
Slide 77 Why would I want to use an MPC? • Tie GRIDstogether(RBEi) • Determine relative motionbetween GRIDs • Maintain separationbetween. GRIDs • Determine average motionbetween GRIDs • Model bellcrankorcontrolsystem • Unitsconversion
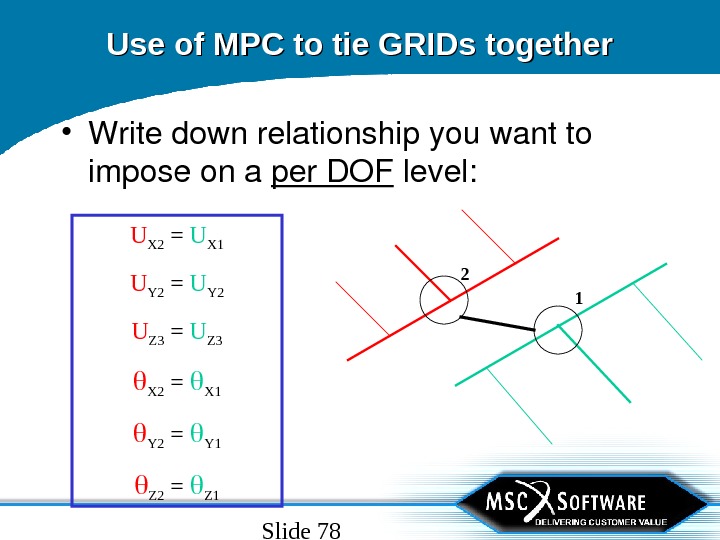
 Slide 78 Use of MPC to tie GRIDs together • Writedownrelationshipyouwantto imposeona per. DOF level: U X 2 = U X 1 U Y 2 = U Y 2 U Z 3 = U Z 3 X 2 = X 1 Y 2 = Y 1 Z 2 = Z
Slide 78 Use of MPC to tie GRIDs together • Writedownrelationshipyouwantto imposeona per. DOF level: U X 2 = U X 1 U Y 2 = U Y 2 U Z 3 = U Z 3 X 2 = X 1 Y 2 = Y 1 Z 2 = Z
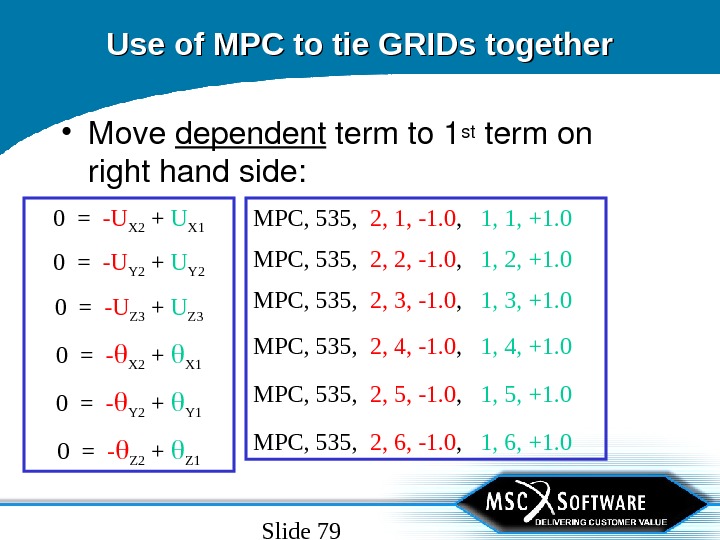
 Slide 79 MPC, 535, 2, 1, -1. 0 , 1, 1, +1. 0 MPC, 535, 2, 2, -1. 0 , 1, 2, +1. 0 MPC, 535, 2, 3, -1. 0 , 1, 3, +1. 0 MPC, 535, 2, 4, -1. 0 , 1, 4, +1. 0 MPC, 535, 2, 5, -1. 0 , 1, 5, +1. 0 MPC, 535, 2, 6, -1. 0 , 1, 6, +1. 0 Use of MPC to tie GRIDs together • Move dependent termto 1 st termon righthandside: 0 = -U X 2 + U X 1 0 = -U Y 2 + U Y 2 0 = -U Z 3 + U Z 3 0 = — X 2 + X 1 0 = — Y 2 + Y 1 0 = — Z 2 + Z
Slide 79 MPC, 535, 2, 1, -1. 0 , 1, 1, +1. 0 MPC, 535, 2, 2, -1. 0 , 1, 2, +1. 0 MPC, 535, 2, 3, -1. 0 , 1, 3, +1. 0 MPC, 535, 2, 4, -1. 0 , 1, 4, +1. 0 MPC, 535, 2, 5, -1. 0 , 1, 5, +1. 0 MPC, 535, 2, 6, -1. 0 , 1, 6, +1. 0 Use of MPC to tie GRIDs together • Move dependent termto 1 st termon righthandside: 0 = -U X 2 + U X 1 0 = -U Y 2 + U Y 2 0 = -U Z 3 + U Z 3 0 = — X 2 + X 1 0 = — Y 2 + Y 1 0 = — Z 2 + Z
 Slide 80 Use of MPC to tie GRIDs together • Use CAUTION whentyingnoncoincident GRIDstogether! • Watchforhowthose rotationsand translationscouple!2 1 U X 2 = U X 1 Z 2 = Z
Slide 80 Use of MPC to tie GRIDs together • Use CAUTION whentyingnoncoincident GRIDstogether! • Watchforhowthose rotationsand translationscouple!2 1 U X 2 = U X 1 Z 2 = Z
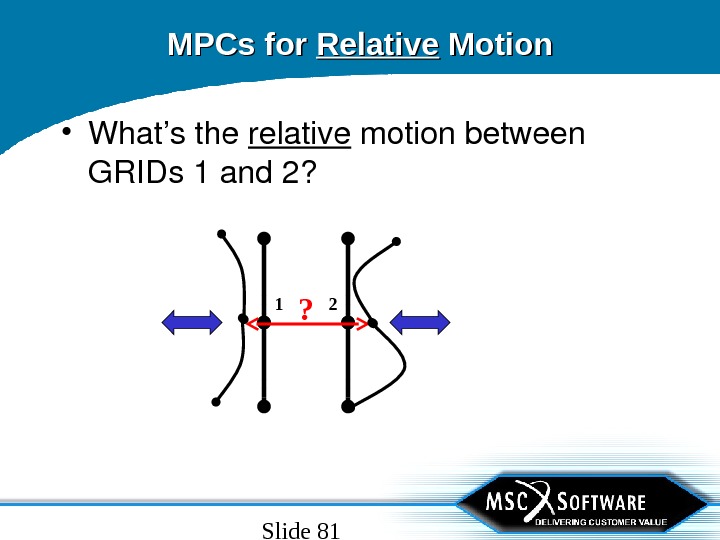
 Slide 81 MPCs for Relative Motion • What’sthe relative motionbetween GRIDs 1 and 2? 1 2 ?
Slide 81 MPCs for Relative Motion • What’sthe relative motionbetween GRIDs 1 and 2? 1 2 ?
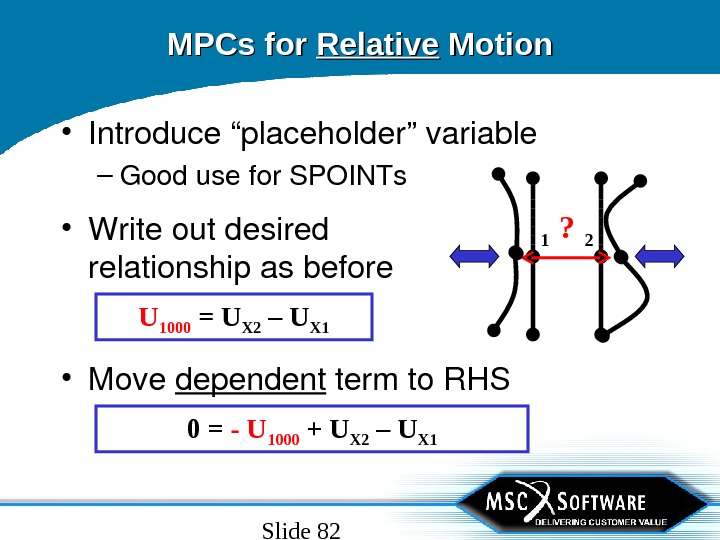
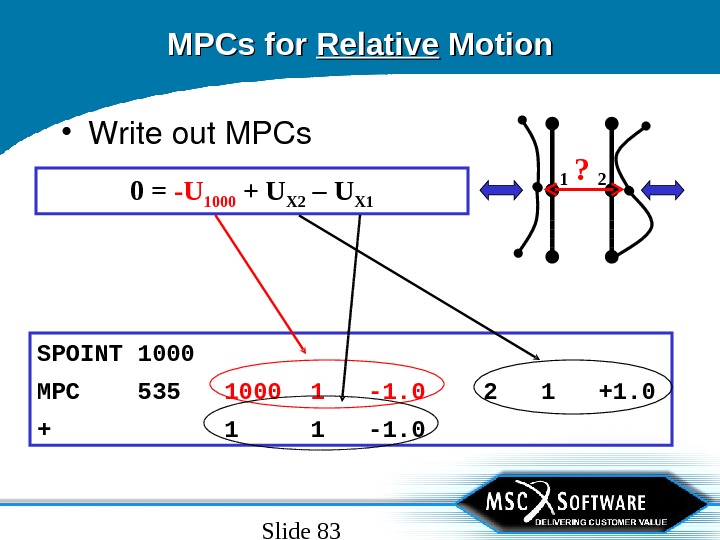
 Slide 82 MPCs for Relative Motion • Introduce“placeholder”variable – Goodusefor. SPOINTs 1 2? • Move dependent termto. RHS 0 = — U 1000 + U X 2 – U X 1 • Writeoutdesired relationshipasbefore U 1000 = U X 2 – U X
Slide 82 MPCs for Relative Motion • Introduce“placeholder”variable – Goodusefor. SPOINTs 1 2? • Move dependent termto. RHS 0 = — U 1000 + U X 2 – U X 1 • Writeoutdesired relationshipasbefore U 1000 = U X 2 – U X
 Slide 83 MPCs for Relative Motion • Writeout. MPCs 1 2? 0 = -U 1000 + U X 2 – U X 1 SPOINT 1000 MPC 535 1000 1 -1. 0 2 1 +1. 0 + 1 1 -1.
Slide 83 MPCs for Relative Motion • Writeout. MPCs 1 2? 0 = -U 1000 + U X 2 – U X 1 SPOINT 1000 MPC 535 1000 1 -1. 0 2 1 +1. 0 + 1 1 -1.
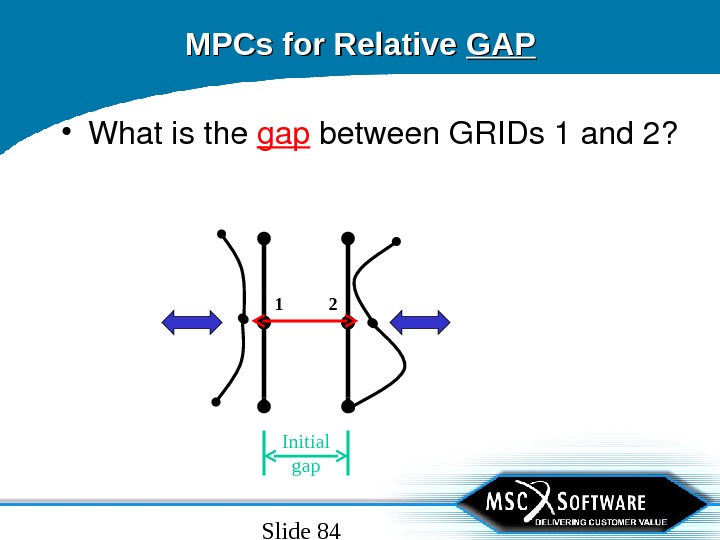
 Slide 84 Initial gap. MPCs for Relative GAPGAP • Whatisthe gap between. GRIDs 1 and 2?
Slide 84 Initial gap. MPCs for Relative GAPGAP • Whatisthe gap between. GRIDs 1 and 2?
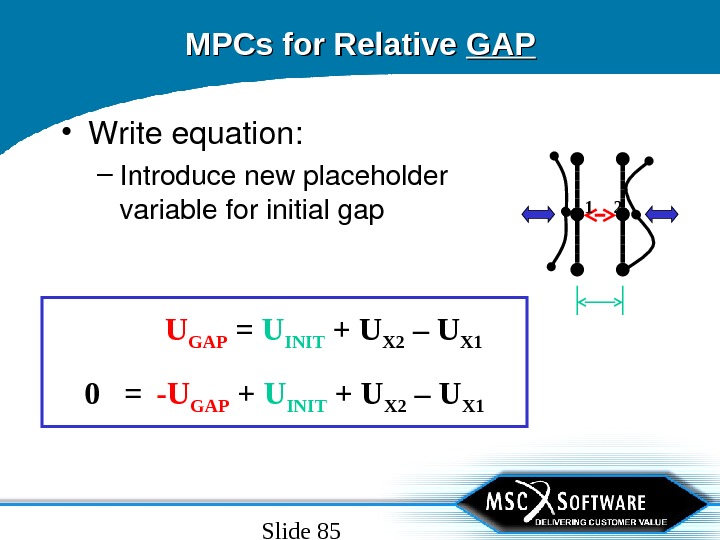
 Slide 85 MPCs for Relative GAPGAP 1 2 U GAP = U INIT + U X 2 – U X 1 0 = -U GAP + U INIT + U X 2 – U X 1 • Writeequation: – Introducenewplaceholder variableforinitialgap
Slide 85 MPCs for Relative GAPGAP 1 2 U GAP = U INIT + U X 2 – U X 1 0 = -U GAP + U INIT + U X 2 – U X 1 • Writeequation: – Introducenewplaceholder variableforinitialgap
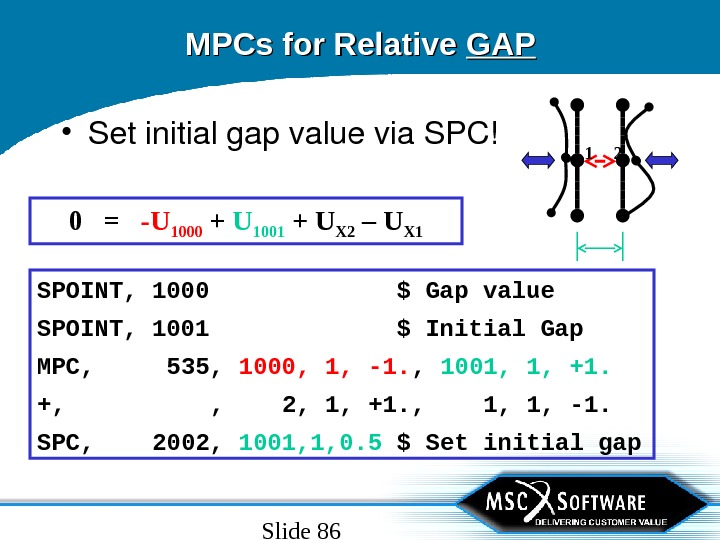
 Slide 86 MPCs for Relative GAPGAP • Setinitialgapvaluevia. SPC! 1 2 SPOINT, 1000 $ Gap value SPOINT, 1001 $ Initial Gap MPC, 535, 1000, 1, -1. , 1001, 1, +1. +, , 2, 1, +1. , 1, 1, -1. SPC, 2002, 1001, 1, 0. 5 $ Set initial gap 0 = -U 1000 + U 1001 + U X 2 – U X
Slide 86 MPCs for Relative GAPGAP • Setinitialgapvaluevia. SPC! 1 2 SPOINT, 1000 $ Gap value SPOINT, 1001 $ Initial Gap MPC, 535, 1000, 1, -1. , 1001, 1, +1. +, , 2, 1, +1. , 1, 1, -1. SPC, 2002, 1001, 1, 0. 5 $ Set initial gap 0 = -U 1000 + U 1001 + U X 2 – U X
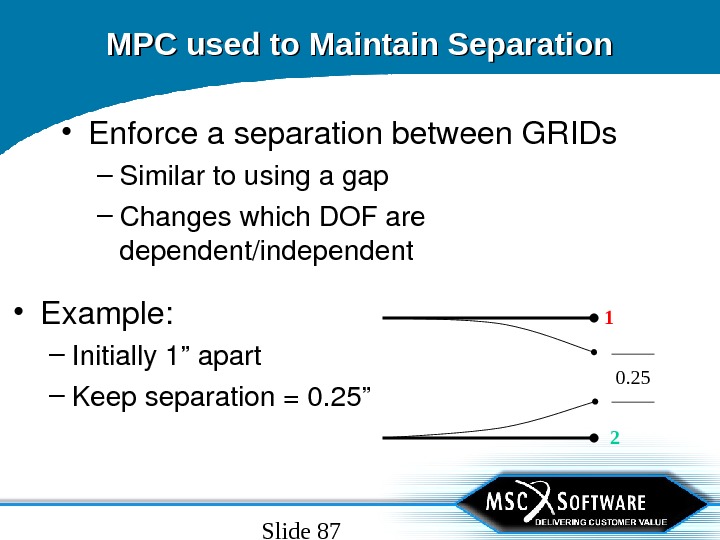
 Slide 87 MPC used to Maintain Separation • Enforceaseparationbetween. GRIDs – Similartousingagap – Changeswhich. DOFare dependent/independent • Example: – Initially 1”apart – Keepseparation=0. 25” 1 2 0.
Slide 87 MPC used to Maintain Separation • Enforceaseparationbetween. GRIDs – Similartousingagap – Changeswhich. DOFare dependent/independent • Example: – Initially 1”apart – Keepseparation=0. 25” 1 2 0.
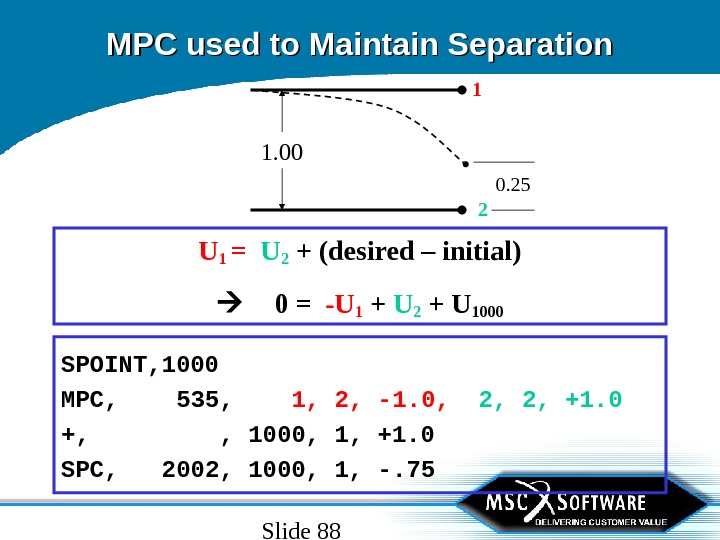
 Slide 88 MPC used to Maintain Separation 1 2 0. 25 U 1 = U 2 + (desired – initial) 0 = -U 1 + U 2 + U 1000 SPOINT, 1000 MPC, 535, 1, 2, -1. 0, 2, 2, +1. 0 +, , 1000, 1, +1. 0 SPC, 2002, 1000, 1, -. 75 1.
Slide 88 MPC used to Maintain Separation 1 2 0. 25 U 1 = U 2 + (desired – initial) 0 = -U 1 + U 2 + U 1000 SPOINT, 1000 MPC, 535, 1, 2, -1. 0, 2, 2, +1. 0 +, , 1000, 1, +1. 0 SPC, 2002, 1000, 1, -. 75 1.
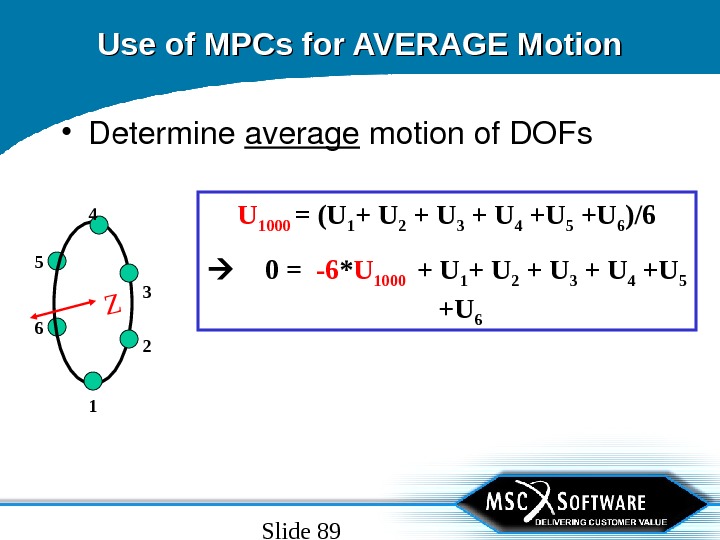
 Slide 89 Use of MPCs for AVERAGE Motion • Determine average motionof. DOFs U 1000 = (U 1 + U 2 + U 3 + U 4 +U 5 +U 6 )/6 0 = -6 * U 1000 + U 1 + U 2 + U 3 + U 4 +U 5 +U 6 Z
Slide 89 Use of MPCs for AVERAGE Motion • Determine average motionof. DOFs U 1000 = (U 1 + U 2 + U 3 + U 4 +U 5 +U 6 )/6 0 = -6 * U 1000 + U 1 + U 2 + U 3 + U 4 +U 5 +U 6 Z
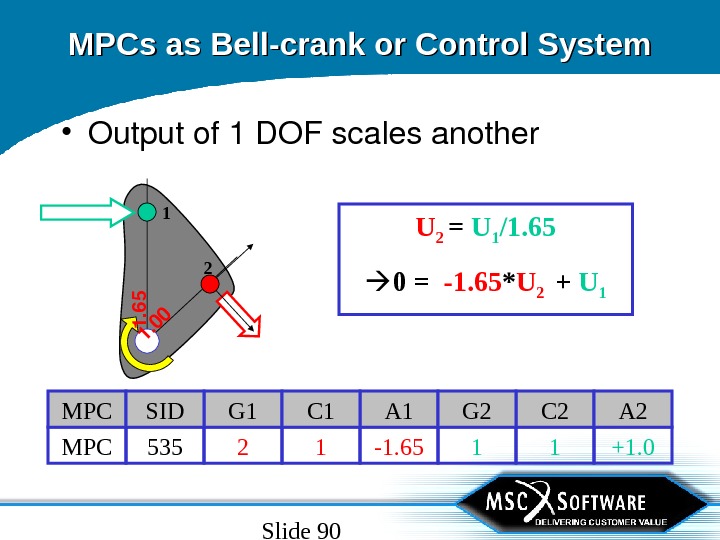
 Slide 90 MPCs as Bell-crank or Control System • Outputof 1 DOFscalesanother U 2 = U 1 /1. 65 0 = -1. 65 * U 2 + U 121 1 +1. 0 -1. 65 12 1 MPC 535 C 2 A 2 A 1 G 2 G 1 C 1 MPC SID 1. 6 5 1.
Slide 90 MPCs as Bell-crank or Control System • Outputof 1 DOFscalesanother U 2 = U 1 /1. 65 0 = -1. 65 * U 2 + U 121 1 +1. 0 -1. 65 12 1 MPC 535 C 2 A 2 A 1 G 2 G 1 C 1 MPC SID 1. 6 5 1.
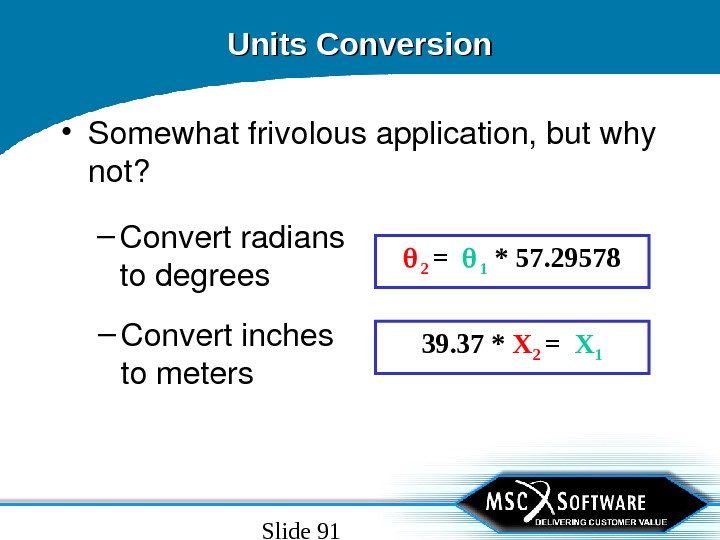
 Slide 91 Units Conversion • Somewhatfrivolousapplication, butwhy not? – Convertradians todegrees 2 = 1 * 57. 29578 – Convertinches tometers 39. 37 * X 2 = X
Slide 91 Units Conversion • Somewhatfrivolousapplication, butwhy not? – Convertradians todegrees 2 = 1 * 57. 29578 – Convertinches tometers 39. 37 * X 2 = X
 Slide 92 Rigid Element Output • Since. Rigidelementsareaspecialized inputof. MPCequations, theoutputis requestedby. MPCFORCEcasecontrol command. – COMMONERROR • The. MPCFORCEsareassociatedwith. GRID IDs, not. Element. IDs. Sowhenselectinga SETforoutput, besurethesetisfor. GRIDIDs, not. Element. IDs.
Slide 92 Rigid Element Output • Since. Rigidelementsareaspecialized inputof. MPCequations, theoutputis requestedby. MPCFORCEcasecontrol command. – COMMONERROR • The. MPCFORCEsareassociatedwith. GRID IDs, not. Element. IDs. Sowhenselectinga SETforoutput, besurethesetisfor. GRIDIDs, not. Element. IDs.
 Slide 93 Guidelines for “Rigid” Elements • Linear. ONLY – Relationshipscalculatedbasedon initial geometry • Cancauseinternalconstraintsfor thermalconditions • Becarefulthatindependent. GRIDhas 6 DO
Slide 93 Guidelines for “Rigid” Elements • Linear. ONLY – Relationshipscalculatedbasedon initial geometry • Cancauseinternalconstraintsfor thermalconditions • Becarefulthatindependent. GRIDhas 6 DO
 Slide 94 MPCs and RBEs • Offtheshelf – RBAR – RBE 2 • Customizable – RBE 3 • Handmade – MPC Add them to your modeling arsenal today!
Slide 94 MPCs and RBEs • Offtheshelf – RBAR – RBE 2 • Customizable – RBE 3 • Handmade – MPC Add them to your modeling arsenal today!

