PYTHAGOREAN THEOREM Daniela Stoikeviča Aļona Tarasova DEFINITION

PYTHAGOREAN THEOREM Daniela Stoikeviča Aļona Tarasova

DEFINITION The Pythagorean theorem, also known as Pythagoras' theorem, is a relation in Euclidean geometry among the three sides of a right triangle. It states that the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. The theorem can be written as an equation relating the lengths of the sides a, b and c, often called the "Pythagorean equation".

HISTORY Just Who Was This Pythagoras, Anyway? Pythagoras (569 -500 B. C. E. ) was born on the island of Samos in Greece, and did much traveling through Egypt, learning, among other things, mathematics. Pythagoras gained his famous status by founding a group, the Brotherhood of Pythagoreans, which was devoted to the study of mathematics. Legend has it that upon completion of his famous theorem, Pythagoras sacrificed 100 oxen. The Pythagoreans had discovered irrational numbers!

OTHER NAMES • Bartel Leendert van der Waerden (1903– 1996) conjectured that Pythagorean triples were discovered algebraically by the Babylonians. • In India, the Baudhayana Sulba Sutra, the dates of which are given variously as between the 8 th and 2 nd century BC, contains a list of Pythagorean triples discovered algebraically, a statement of the Pythagorean theorem, and a geometrical proof of the Pythagorean theorem for an isosceles right triangle.

THE SUM OF THE SQUARES OF THE SIDES OF A RIGHT TRIANGLE IS EQUAL TO THE SQUARE OF THE HYPOTENUSE. This relationship has been known since the days of the ancient Babylonians and Egyptians, although it may not have been stated as explicitly as above. A portion of a 4000 year old Babylonian tablet (c. 1900 B. C. E. ), now known as Plimpton 322, (in the collection of Columbia University, New York), lists columns of numbers showing what we now call Pythagorean Triples--sets of numbers that satisfy the equation: a 2 + b 2 = c 2

HANDS ON ACTIVITY It is known that the Egyptians used a knotted rope as an aid to constructing right angles in their buildings. The rope had 12 evenly spaced knots, which could be formed into a 3 -4 -5 right triangle, thus giving an angle of exactly 90 degrees. Here is further evidence that the Pythagorean relationship was known before the first written proof of it. The pattern of tiles shown here is typical of those seen in the Orient. Can you see the proof of the Pythagorean Theorem in the tile pattern?

Mathematical constructions made from crackers

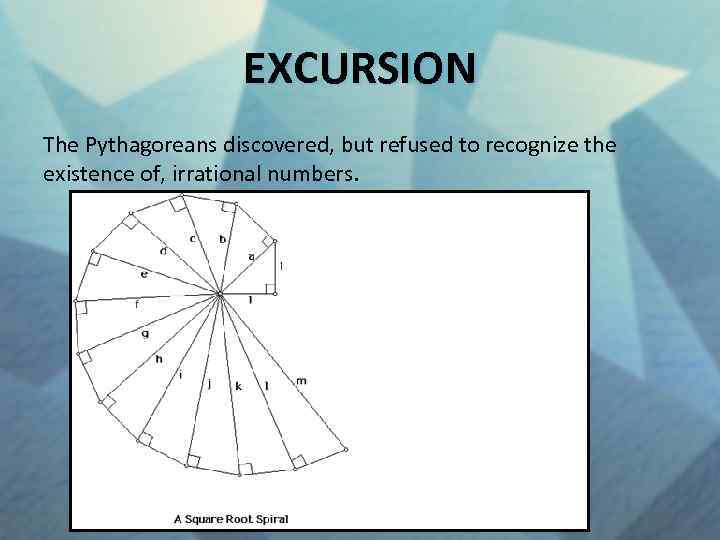EXCURSION The Pythagoreans discovered, but refused to recognize the existence of, irrational numbers.

PYTHAGOREAN PROOF Click here

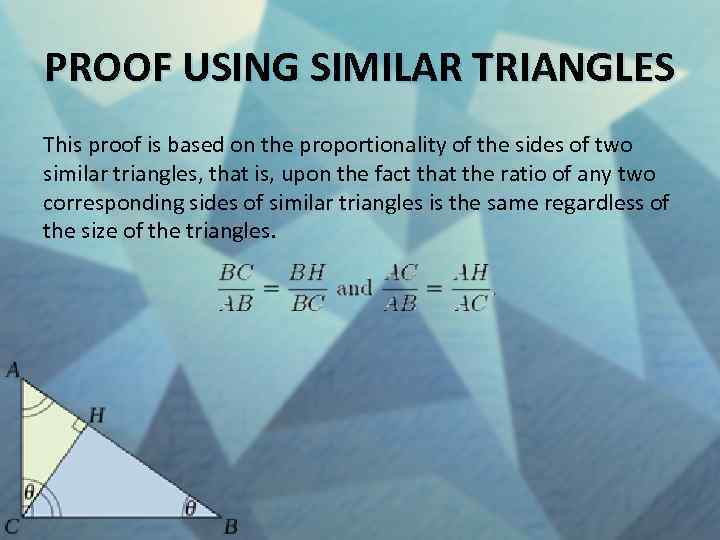PROOF USING SIMILAR TRIANGLES This proof is based on the proportionality of the sides of two similar triangles, that is, upon the fact that the ratio of any two corresponding sides of similar triangles is the same regardless of the size of the triangles.

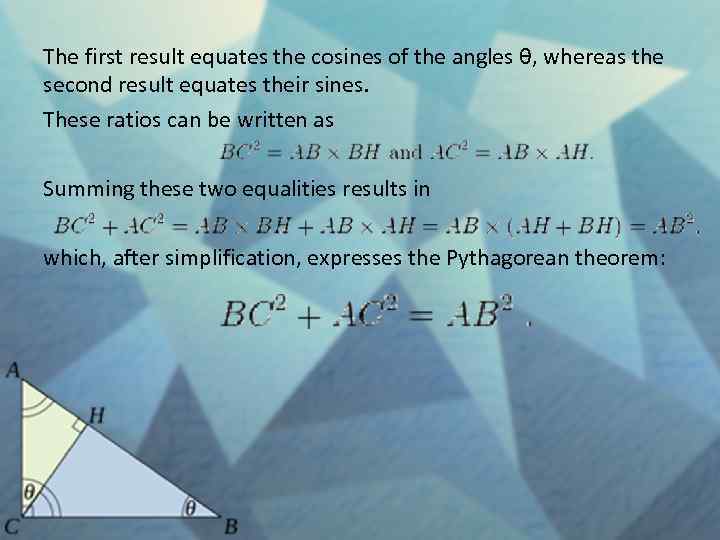The first result equates the cosines of the angles θ, whereas the second result equates their sines. These ratios can be written as Summing these two equalities results in which, after simplification, expresses the Pythagorean theorem:

PROOF BY REARRANGEMENT Click to view animation: 1 st proof 2 nd proof

HOW IS THE PYTHAGOREAN THEOREM USED TODAY? The Pythagorean theorem is used often in construction, in engineering, in architecture, in design, in art and in aeronautics. How do architects use the Pythagorean theorem? Architects use the Pythagorean theorem, which is expressed by the equation: a 2 + b 2 = c 2, in designing and computing the measurements of building structures and bridges.

Sydney Harbour Bridge

SCIENCE OF NFL FOOTBALL: THE PYTHAGOREAN THEOREM Click here

THANK YOU FOR YOUR ATTENTION! References: Ø http: //www. geom. uiuc. edu/~demo 533 7/Group 3/hist. html Ø Stephen W. Hawking (2005). God created the integers: the mathematical breakthroughs that changed history. Philadelphia: Running Press Book Publishers. p. 12. Ø Animations from: https: //en. wikipedia. org/wiki/Pythagor ean_theorem#cite_note-11 Ø Video from: http: //science 360. gov/obj/video/d 37 d d 34 c-b 721 -4230 -993127 c 663 c 208 df/science-nfl-footballpythagorean-theorem