Properties of Triangles Objectives: Identify isosceles, equilateral and right-angled triangles. Use the word ‘congruent’ when triangles are identical. Show that the angles of a triangle add up to 180 o and use this to find angles. Show that the exterior angle of a triangle is equal to the sum of the interior opposite angles. .
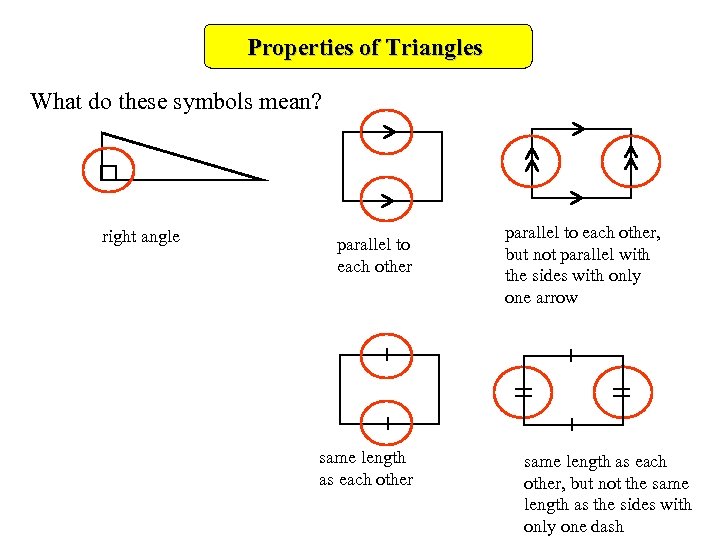
Properties of Triangles What do these symbols mean? right angle parallel to each other same length as each other parallel to each other, but not parallel with the sides with only one arrow same length as each other, but not the same length as the sides with only one dash
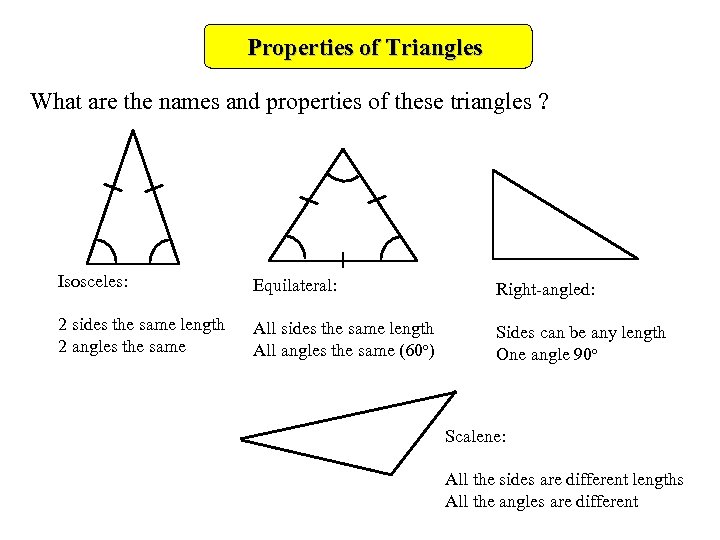
Properties of Triangles What are the names and properties of these triangles ? Isosceles: Equilateral: Right-angled: 2 sides the same length 2 angles the same All sides the same length All angles the same (60 o) Sides can be any length One angle 90 o Scalene: All the sides are different lengths All the angles are different
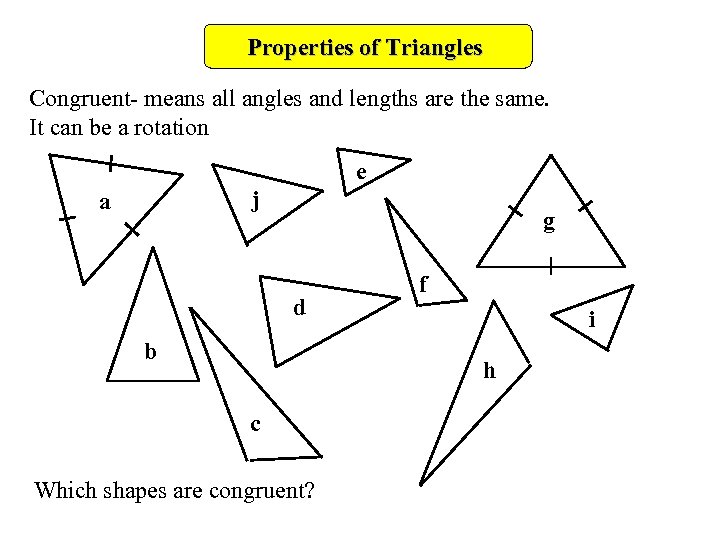
Properties of Triangles Congruent- means all angles and lengths are the same. It can be a rotation e j a g d b f i h c Which shapes are congruent?
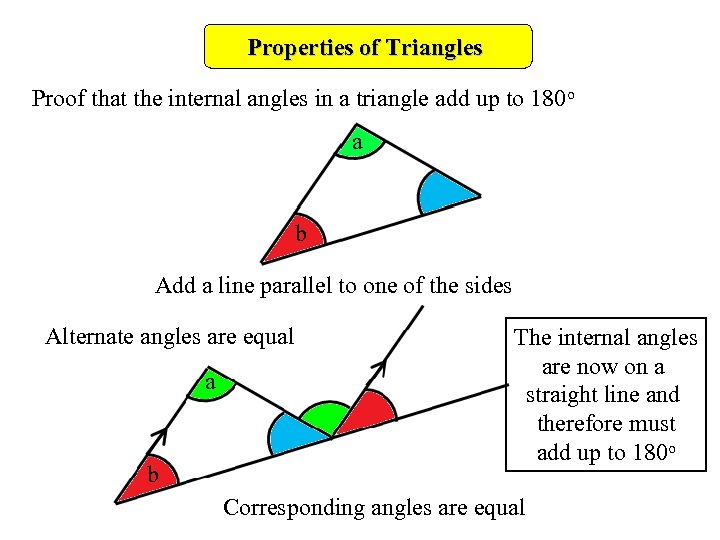
Properties of Triangles Proof that the internal angles in a triangle add up to 180 o a b Add a line parallel to one of the sides Alternate angles are equal a b The internal angles are now on a straight line and therefore must add up to 180 o Corresponding angles are equal
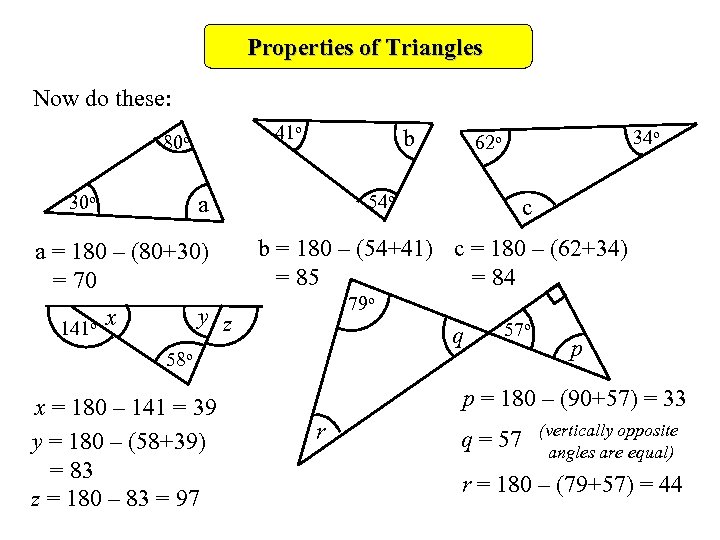
Properties of Triangles Now do these: 41 o 80 o a = 180 – (80+30) = 70 c b = 180 – (54+41) c = 180 – (62+34) = 85 = 84 79 o y z x 34 o 62 o 54 o a 30 o 141 o b q 57 o 58 o x = 180 – 141 = 39 y = 180 – (58+39) = 83 z = 180 – 83 = 97 p p = 180 – (90+57) = 33 r q = 57 (vertically opposite angles are equal) r = 180 – (79+57) = 44
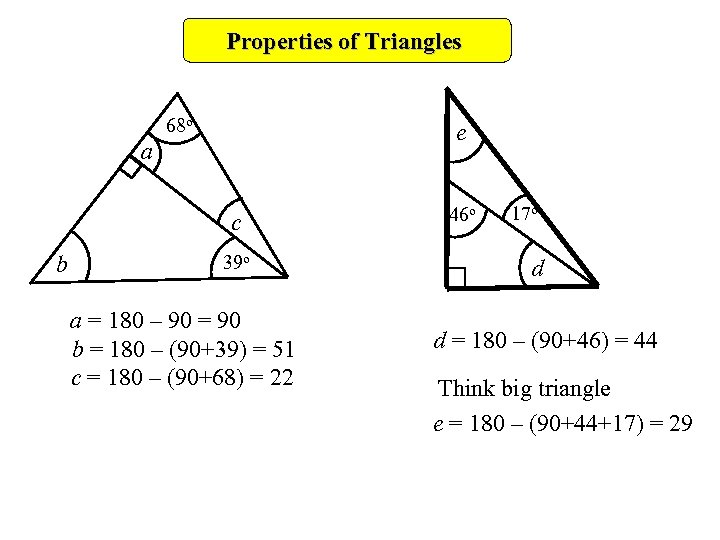
Properties of Triangles 68 o e a c b 39 o a = 180 – 90 = 90 b = 180 – (90+39) = 51 c = 180 – (90+68) = 22 46 o 17 o d d = 180 – (90+46) = 44 Think big triangle e = 180 – (90+44+17) = 29
Скачать презентацию Properties of Triangles Objectives Identify isosceles equilateral and
154b689eb950e64aa4d1533b08581100.ppt