1f2068e916aeaa948e76d79794c6eb9f.ppt
- Количество слайдов: 29

Option pricing models

Objective Learn to estimate the market value of option contracts.

Outline • The Binomial Model • The Black-Scholes pricing model
The Binomial Model A very simple to use and understand lattice model that provides theoretical foundation for other, more advanced models

The one-period binomial model Exemplification Assume the stock of ABC currently sells for $100/share. A call option on the ABC is struck at $120/share and expires in one year. In one year, the stock can either go up by 50% or down by 25%. How much should you pay for the call today if the risk free rate is 10%?
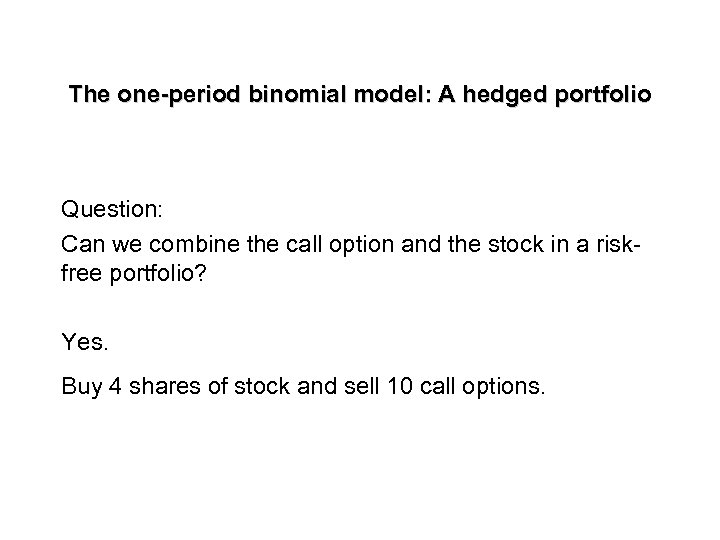
The one-period binomial model: A hedged portfolio Question: Can we combine the call option and the stock in a riskfree portfolio? Yes. Buy 4 shares of stock and sell 10 call options.

A hedged portfolio 1+ u = 1. 5 Su = $150 Portfolio value: $150(4) - $30(10) = $300 S 0 = $100 Buy 4 shares and sell 10 calls Cash flow = 10 C 0 - $400 1+ d = 0. 75 Sd = $75 Portfolio value: $75(4) = $300

A hedged portfolio: Summary Our portfolio is risk-free. We invested 10 C 0 - $400 and we obtained $300 over one year Our portfolio must earn the risk-free rate. (10 C 0 - $400)(1. 1) = $300 C 0 = $12. 73 The call option on stock ABC, struck at $120 and expiring in one year, should sell today for $12. 73
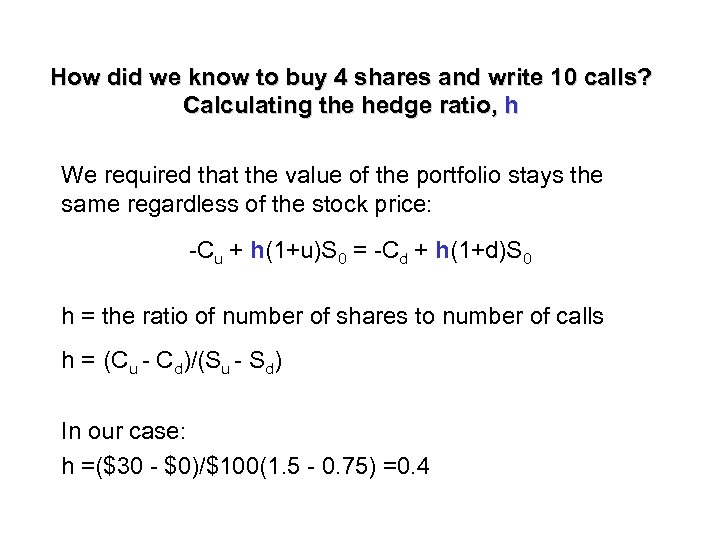
How did we know to buy 4 shares and write 10 calls? Calculating the hedge ratio, h We required that the value of the portfolio stays the same regardless of the stock price: -Cu + h(1+u)S 0 = -Cd + h(1+d)S 0 h = the ratio of number of shares to number of calls h = (Cu - Cd)/(Su - Sd) In our case: h =($30 - $0)/$100(1. 5 - 0. 75) =0. 4
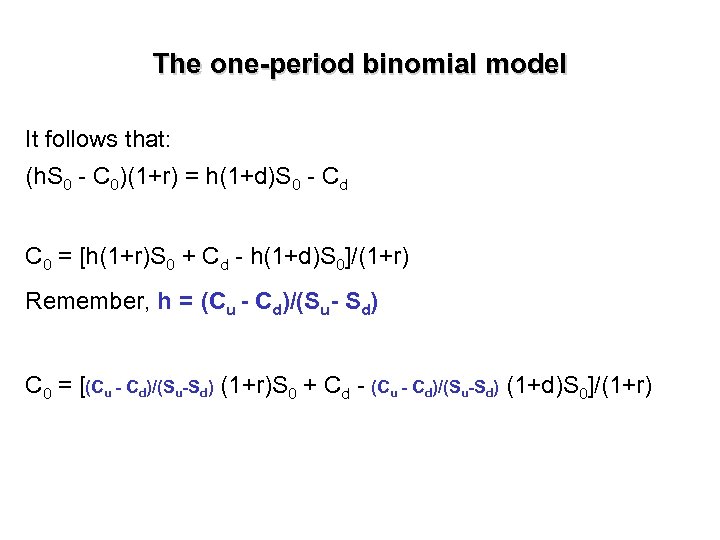
The one-period binomial model It follows that: (h. S 0 - C 0)(1+r) = h(1+d)S 0 - Cd C 0 = [h(1+r)S 0 + Cd - h(1+d)S 0]/(1+r) Remember, h = (Cu - Cd)/(Su- Sd) C 0 = [(Cu - Cd)/(Su-Sd) (1+r)S 0 + Cd - (Cu - Cd)/(Su-Sd) (1+d)S 0]/(1+r)
![The one-period binomial model C 0 = [p. Cu + (1 -p)Cd]/(1+r) where p The one-period binomial model C 0 = [p. Cu + (1 -p)Cd]/(1+r) where p](https://present5.com/presentation/1f2068e916aeaa948e76d79794c6eb9f/image-11.jpg)
The one-period binomial model C 0 = [p. Cu + (1 -p)Cd]/(1+r) where p = (r-d)/(u-d) 1 - p = (u-r)/(u-d)

The two-period binomial model Exemplification Assume the stock of ABC currently sells for $100/share. A call option on the ABC is struck at $120/share and expires in two years. Each year, the stock can either go up by 50% or down by 25%. How much should you pay for the call today if the risk free rate is 10%?
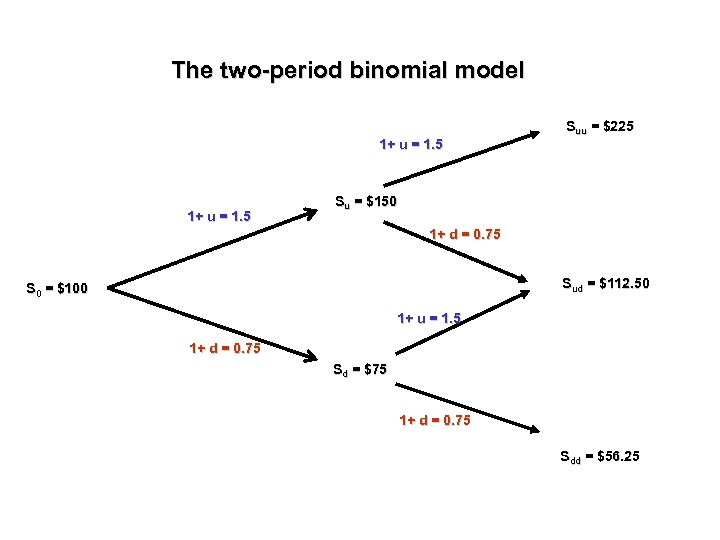
The two-period binomial model Suu = $225 1+ u = 1. 5 Su = $150 1+ d = 0. 75 Sud = $112. 50 S 0 = $100 1+ u = 1. 5 1+ d = 0. 75 Sd = $75 1+ d = 0. 75 Sdd = $56. 25
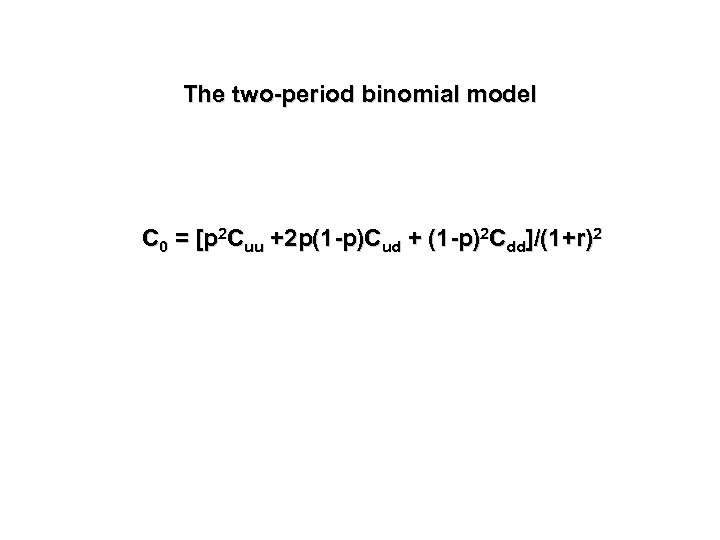
The two-period binomial model C 0 = [p 2 Cuu +2 p(1 -p)Cud + (1 -p)2 Cdd]/(1+r)2

What if we have n-periods? Assume Returns are log-normal distributed The risk-free rate and the returns volatility are constant Each period becomes extremely short Interest is compounded continuously No transaction costs The options are European and/or American but no dividend is paid on the stock until after expiration

The Black-Scholes formula C = SN(d 1) - Ee-r. T N(d 2) S = current stock price E = exercise price d 1 = [ln(S/E) + (r + 2/2)T]/ T 1/2 d 2 = d 1 - T 1/2 N(d 1), N(d 2) = cumulative normal probabilities = annualized standard deviation of stock’s return r = continuously compounded risk-free rate T = time to maturity

The Black-Scholes formula: A numerical example Digital Equipment July 165 call has 35 days to maturity (assume 365 days in a year) The current stock price is $164 The risk-free rate is 5. 35% The standard deviation of the return is 29% Calculate theoretical value of the call option

Step 1 Express the risk-free rate as a continuously compounded yield (find out that continuously compounded rate that would yield 5. 35%): (1 + r*/n)n = 1. 0535 er* = 1. 0535 r* = ln(1. 0535) = 0. 0521

Step 2 Calculate d 1 and d 2: d 1 = [ln(164/165) + (0. 0521 + 0. 0841/2)0. 0959]/(0. 29)(0. 309677) d 1 = [ - 0. 006079 + 0. 009029 ]/(0. 0898063) = 0. 0328485 d 2 = 0. 0328485 - 0. 0898063 = - 0. 0569578
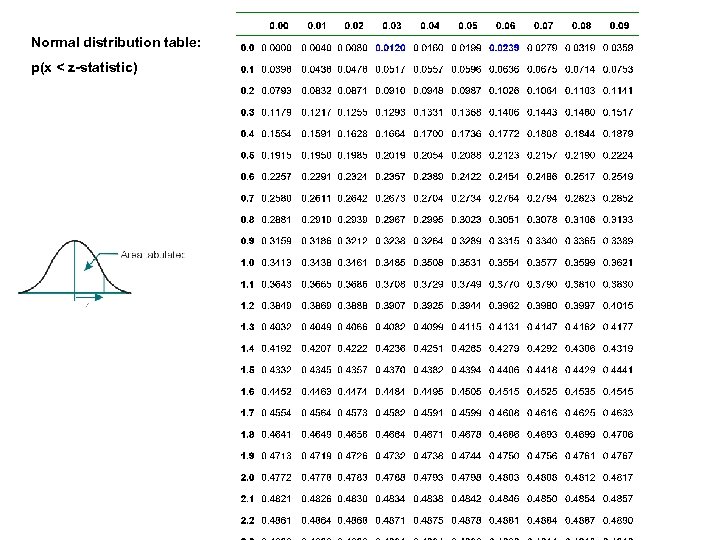
Normal distribution table: p(x < z-statistic)

Step 3 N(0. 03) = 0. 5 + 0. 012 = 0. 512 N(-0. 06) = 0. 5 - 0. 0239 = 0. 4761

Step 4 C = 164(0. 512) - 165 e-(0. 521)(0. 0959)(0. 4761) C = 83. 968 - 165(0. 995)(0. 4761) = $5. 803
Discussion Right now, the stock is at $164 The call is out-of-the-money (intrinsic value is zero) The market value should be $5. 803, reflecting the probability that, by expiration, the stock price might increase This probability calculation is based on observing the volatility of return

Relationship between several variables and the market value of options
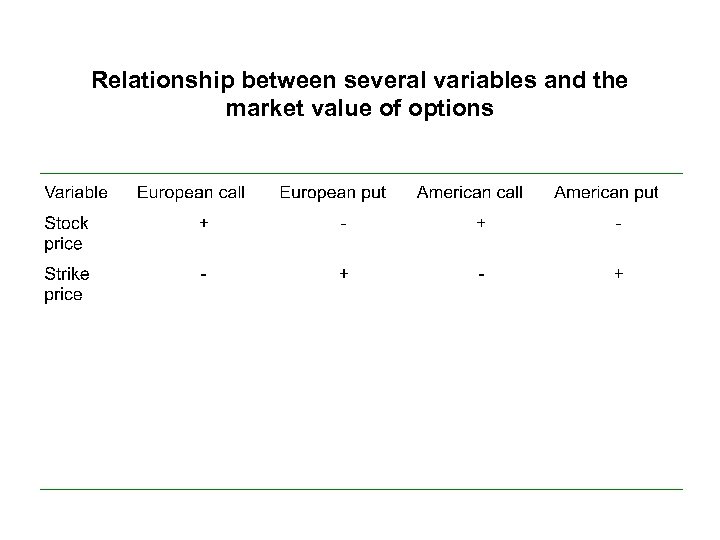
Relationship between several variables and the market value of options
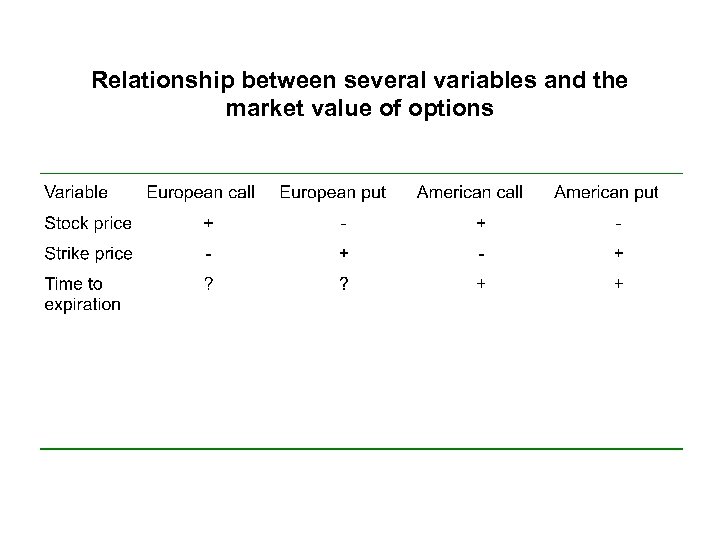
Relationship between several variables and the market value of options

Relationship between several variables and the market value of options
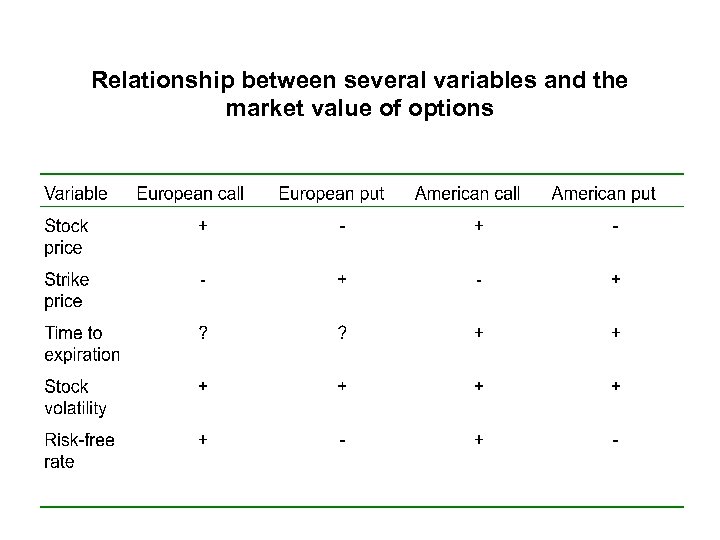
Relationship between several variables and the market value of options
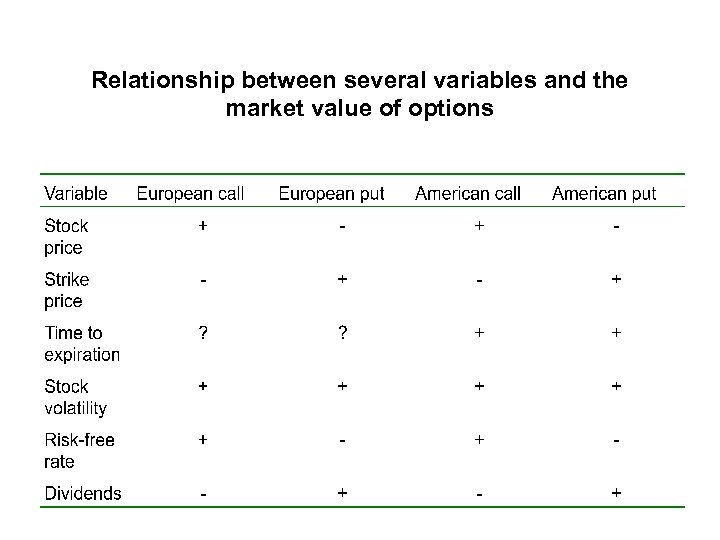
Relationship between several variables and the market value of options
1f2068e916aeaa948e76d79794c6eb9f.ppt