Methods in Chemistry III Part 1 Modul

Methods in Chemistry III – Part 1 Modul M. Che. 1101 WS 2010/11 – 1 Modern Methods of Inorganic Chemistry Mi 10: 15 -12: 00, Hörsaal II George Sheldrick gsheldr@shelx. uni-ac. gwdg. de

Part 1. Crystal structure determination The lectures will each take about 50 minutes, followed by exercises in the same lecture room (about 40 minutes). Each exercise will be rewarded with 0 to 2 points. These points constitute 24% of the total mark for the practical. A maximum of 24 such points will be counted (theoretically 26 would be possible for the 13 exercises). The practical class takes one week (5 days, 1 pm until 6 pm). The work should be performed in pairs and a maximum of 10 students may take part each week. This course will be offered for two weeks in March and four in the summer term. Registration takes place via Stud -IP. Each group starts on a Monday and each participant should make a short presentation of his or her results on the Friday and answer a few questions. The marks will be based on this presentation and the written report of the work performed in the practical. Part 2 (Meyer/Demeshko, Spectroscopic Methods) takes place each Thursday in the winter term between 10: 15 and 12: 00, followed by a separate practical class. The written exam for both parts together is scheduled for Wednesday, March 2 nd and the repeat exam for Wednesday April 6 th 2011.

Why crystal structure determination? The line diagram of an organic molecule can usually be determined by NMR, but without the help of a force field, NMR cannot determine bond lengths and angles. X-ray diffraction is not always the most accurate way of determining a structure. For very small molecules, rotational spectroscopy or even gas phase electron diffraction can be more precise. X-ray crystal structure determination is however also suitable for determining very large structures such as proteins, DNA, viruses etc. and the method is very generally applicable, for example for minerals, organometallic compounds and natural products. Crystal structures are objective and are still valid 50 years after their determination. Inorganic and organometallic journals often require a crystal structure as a proof that the compound claimed has actually been synthesized. But: In order to determine a crystal structure, crystals are required!

Nobel prizes for crystallographers 1914 M. von Laue 1915 W. H. Bragg and W. L. Bragg 1954 L. Pauling 1962 J. C. Kendrew, M. Perutz, F. Crick, J. D. Watson and M. H. F. Wilkins 1964 Dorothy C. Hodgkin 1976 W. N. Lipscomb 1982 A. Klug 1985 H. A. Hauptman and J. Karle 1988 J. Deisenhofer, R. Huber and H. Michel 1997 J. E. Walker 2003 P. Agre und R. Mac. Kinnon 2006 R. Kornberg 2009 V. Ramakrishnan, T. A. Steiz and Ada Yonath

RNA-Polymerase II The chemistry Nobel prize for 2006 was awarded to Roger Kornberg (Stanford, USA) for his crystal structures of RNA-Polymerase II in action: RNA is synthesized DNA (blueprint) Enzyme (protein catalyst)

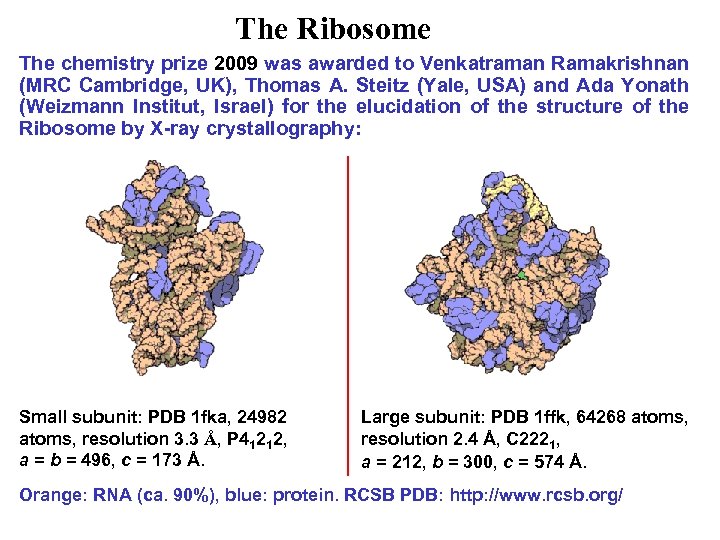The Ribosome The chemistry prize 2009 was awarded to Venkatraman Ramakrishnan (MRC Cambridge, UK), Thomas A. Steitz (Yale, USA) and Ada Yonath (Weizmann Institut, Israel) for the elucidation of the structure of the Ribosome by X-ray crystallography: Small subunit: PDB 1 fka, 24982 atoms, resolution 3. 3 Å, P 41212, a = b = 496, c = 173 Å. Large subunit: PDB 1 ffk, 64268 atoms, resolution 2. 4 Å, C 2221, a = 212, b = 300, c = 574 Å. Orange: RNA (ca. 90%), blue: protein. RCSB PDB: http: //www. rcsb. org/

X-ray diffraction: recommended reading The most suitable book for this course is actually in German: W. Massa, Kristallstrukturbestimmung, 6. Auflage Sept. 2009, Teubner Verlag, ISBN 978 -3834806499, ca. 36 Euro. There are copies in the inorganic library; the librarian, Herr Wöske, can help you to find them. Biomolecular crystallography, 1 st Edition 2010, by Bernhard Rupp, Garland Science, ISBN 978 -0815340812 (ca. 90 Euro) is a most comprehensive, up-to-date and readable account of macromolecular crystallography. For the first two lectures (point groups) there is a very nice tutorial on the internet: http: //symmetry. otterbein. edu/ Consulting hours: 8: 30 -9: 30 in room 333 of the inorganic chemistry.

Symmetry and point groups The symmetry of a molecule is described by a point group. Only two-, three-, four- and six-fold axes are compatible with an infinite crystal lattice, so that only 32 of these point groups (the so-called crystallographic point groups) form the basis of the 230 space groups that describe periodic three-dimensional structures. It is not possible to arrange five-fold axes in a periodic and self-consistent manner.

Symmetry elements O H 180º H C 2 N H H 120º C 3 H The molecule H 2 O possesses a C 2 rotation axis along the angle bisector and two mirror planes that intersect each other along the rotation axis. The point group is called C 2 v or 2 mm. Each combination of two of these elements would generate third. The molecule NH 3 possesses a C 3 rotation axis and three mirror planes that intersect one another along the rotation axis. The point group is called C 3 v or 3 m.

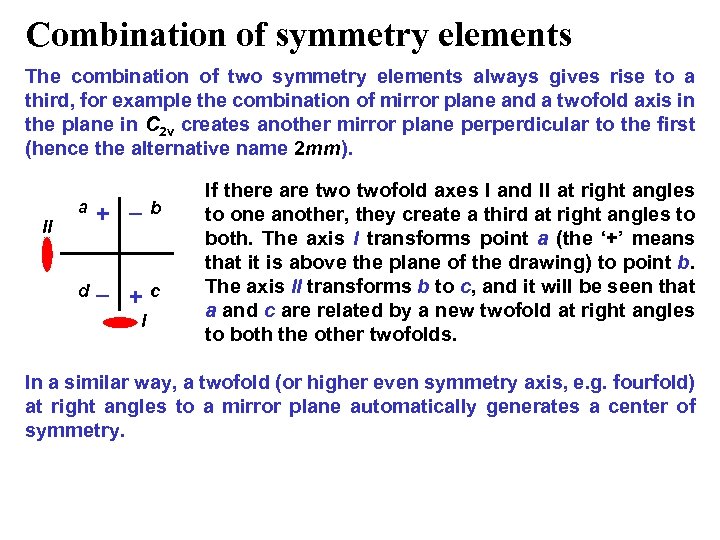Combination of symmetry elements The combination of two symmetry elements always gives rise to a third, for example the combination of mirror plane and a twofold axis in the plane in C 2 v creates another mirror plane perperdicular to the first (hence the alternative name 2 mm). a + – d – +c II I b If there are twofold axes I and II at right angles to one another, they create a third at right angles to both. The axis I transforms point a (the ‘+’ means that it is above the plane of the drawing) to point b. The axis II transforms b to c, and it will be seen that a and c are related by a new twofold at right angles to both the other twofolds. In a similar way, a twofold (or higher even symmetry axis, e. g. fourfold) at right angles to a mirror plane automatically generates a center of symmetry.

Schönflies and Hermann-Mauguin The Schönflies system is mainly used by spektroscopists and the Hermann-Mauguin system by crystallographers. Both systems have their idiosyncrasies. An inversion center is called ”i” (S) or 1 (HM). In the presence of a vertical rotation axis, vertical mirror planes are called “v” and “m” respectively; horizontal mirror planes are “h” and “/m”. An N-fold rotation axis is CN (S) or N (H-M). Examples: C 1=1; C 2=2; C 3=3; C 4=4; Ci=1; Cs=m; C 2 v=2 mm; C 2 h=2/m [Cs is also called C 1 v and 2 mm can be named mm 2].

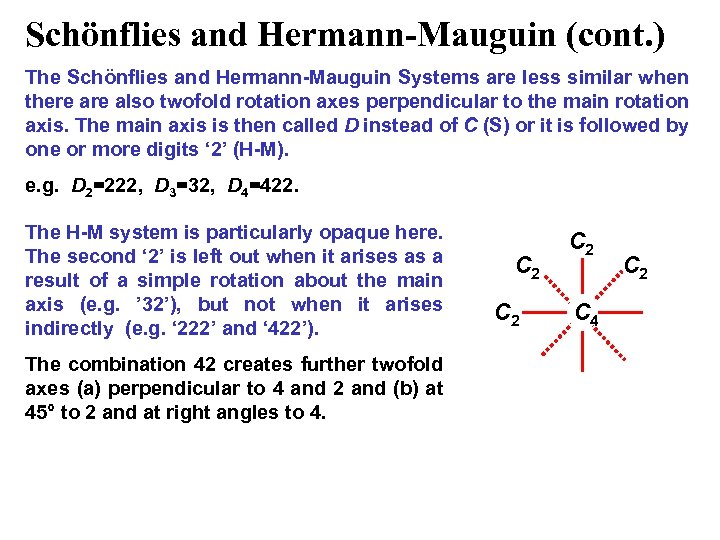Schönflies and Hermann-Mauguin (cont. ) The Schönflies and Hermann-Mauguin Systems are less similar when there also twofold rotation axes perpendicular to the main rotation axis. The main axis is then called D instead of C (S) or it is followed by one or more digits ‘ 2’ (H-M). e. g. D 2=222, D 3=32, D 4=422. The H-M system is particularly opaque here. The second ‘ 2’ is left out when it arises as a result of a simple rotation about the main axis (e. g. ’ 32’), but not when it arises indirectly (e. g. ‘ 222’ and ‘ 422’). The combination 42 creates further twofold axes (a) perpendicular to 4 and 2 and (b) at 45° to 2 and at right angles to 4. C 2 C 2 C 4 C 2

Further D point groups (1) A point group DN possesses both a CN axis and twofold axes perpendicular to it. If vertical mirror planes are also present (between the twofold axes) but there are no horizontal mirror planes, the point group is called DNd. If both vertical and horizontal mirror planes are present, it is called DNh. Examples: CH 2 HC 2 F O O Fe O F O O O [Fe(ox)3]3– D 3=32 F H 2 C P F F H 2 C PF 5 D 3 h=6 m 2 CH 2 C 6 H 12 (cyclohexane) D 3 d=3 m

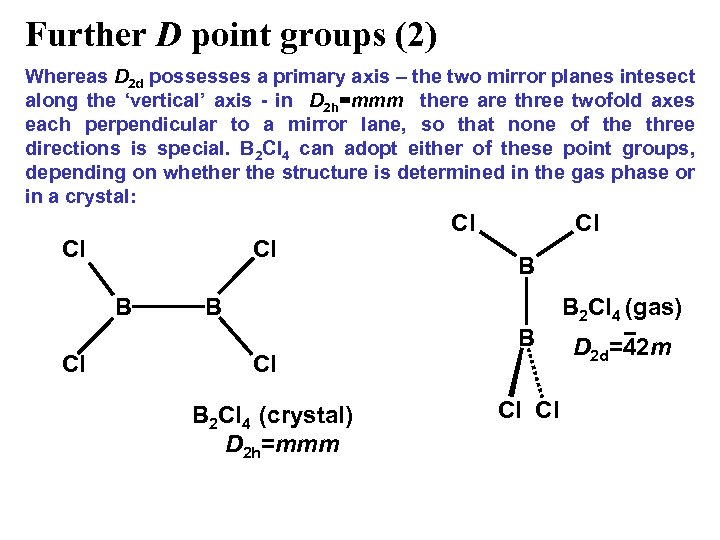Further D point groups (2) Whereas D 2 d possesses a primary axis – the two mirror planes intesect along the ‘vertical’ axis - in D 2 h=mmm there are three twofold axes each perpendicular to a mirror lane, so that none of the three directions is special. B 2 Cl 4 can adopt either of these point groups, depending on whether the structure is determined in the gas phase or in a crystal: Cl Cl B Cl Cl Cl B B B 2 Cl 4 (gas) Cl B 2 Cl 4 (crystal) D 2 h=mmm B Cl Cl D 2 d=42 m

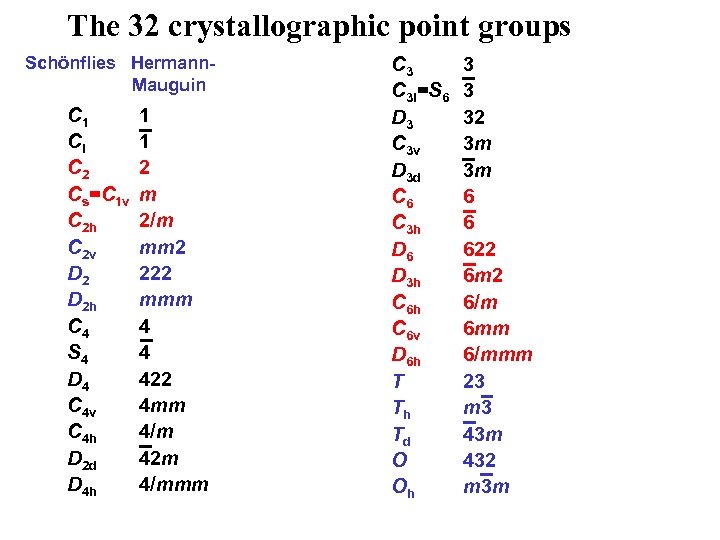The 32 crystallographic point groups Schönflies Hermann. Mauguin C 1 Ci C 2 Cs=C 1 v C 2 h C 2 v D 2 h C 4 S 4 D 4 C 4 v C 4 h D 2 d D 4 h 1 1 2 m 2/m mm 2 222 mmm 4 4 422 4 mm 4/m 42 m 4/mmm C 3 i=S 6 D 3 C 3 v D 3 d C 6 C 3 h D 6 D 3 h C 6 v D 6 h T Th Td O Oh 3 3 32 3 m 3 m 6 6 622 6 m 2 6/m 6 mm 6/mmm 23 m 3 43 m 432 m 3 m

Exercises Which symmetry elements are present in the following, and to which point groups do they belong (in both systems)? 1) H 2 O 2 (gauche) 2) SF 4 3) B 2 H 6 4) C 2 H 6 (staggered and eclipsed) 5) H 2 C=C=CH 2