ME 31 B CHAPTER FOUR DESIGN OF STRUCTURAL

ME 31 B: CHAPTER FOUR DESIGN OF STRUCTURAL MEMBERS

DESIGN OF MEMBERS IN DIRECT STRESS: n Structural members under direct stress are mainly ties, cables, and short columns.

Solution Concluded

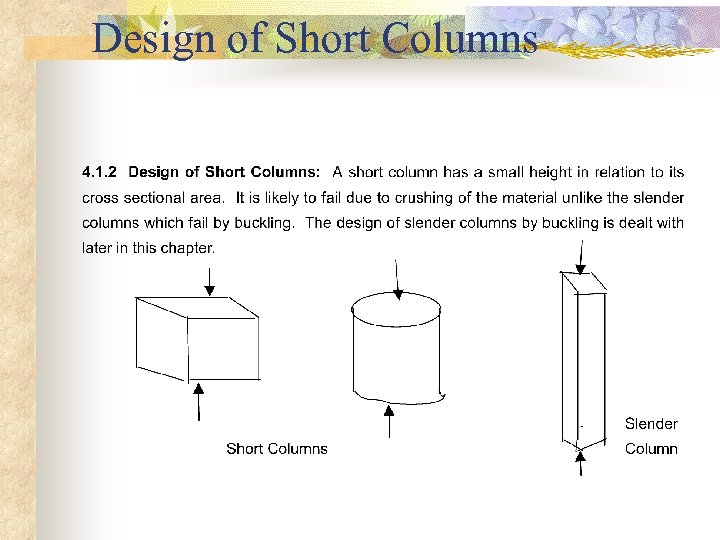
Design of Short Columns

Example n n A square concrete column(pier) which is 0. 5 m high is made of a nominal concrete mix of 1: 2: 4, with a permissible direct stress of 5. 3 N/mm 2. What is the required cross-sectional area if the column is required to carry an axial load of 300 k. N? Solution: Area = Force/stress = 300 x 103 N = 56600 mm 2 5. 3 N/mm 2 n n ie. the column should be minimum 240 mm square.

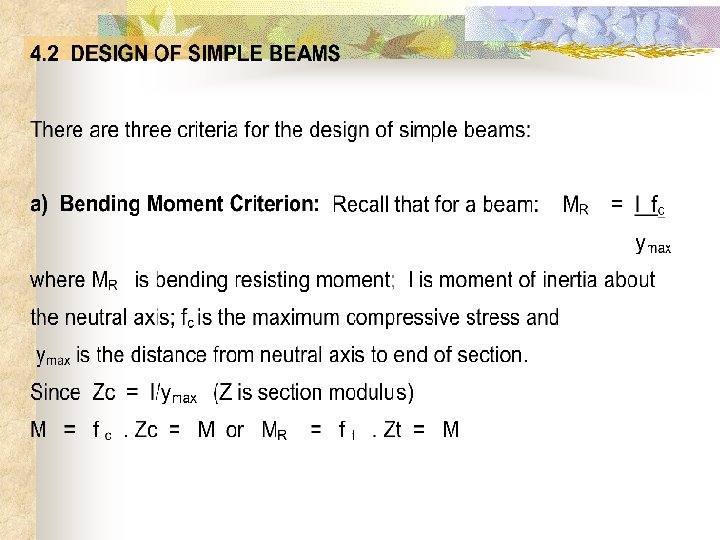
Note n n Maximum compressive stress (fc) occurs in the section where the bending moment is maximum. In the design of simple beams, section modulus (Z) should be selected such that fc does not exceed the allowable value. Allowable working stress values can be found in building codes or Engineering handbooks. For safe bending, fw > f = Mmax/Z where fw is the allowable bending stress; f is the actual stress and Mmax is the maximum bending moment.

b) Deflection of Beams n n n Excessive deflections cause cracking of plaster in ceiling and can lead to jamming of doors and windows. Most building codes limit the amount of allowable deflection as a proportion of the member's length ie. 1/180 , 1/240, or 1/360 of the length.

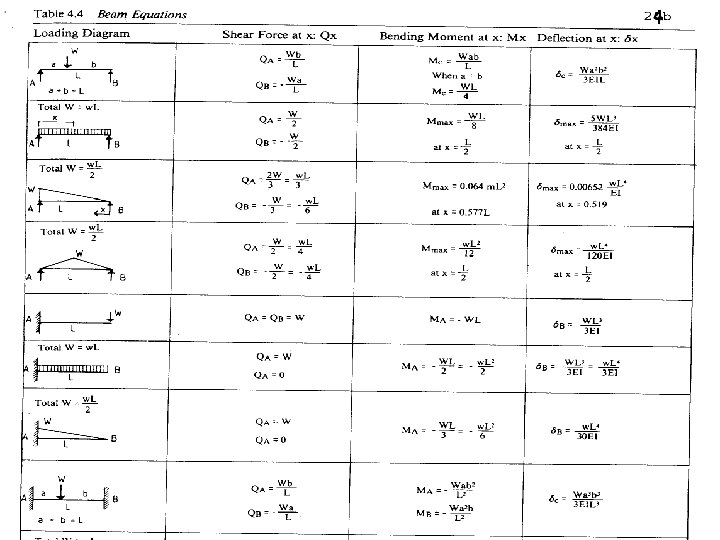
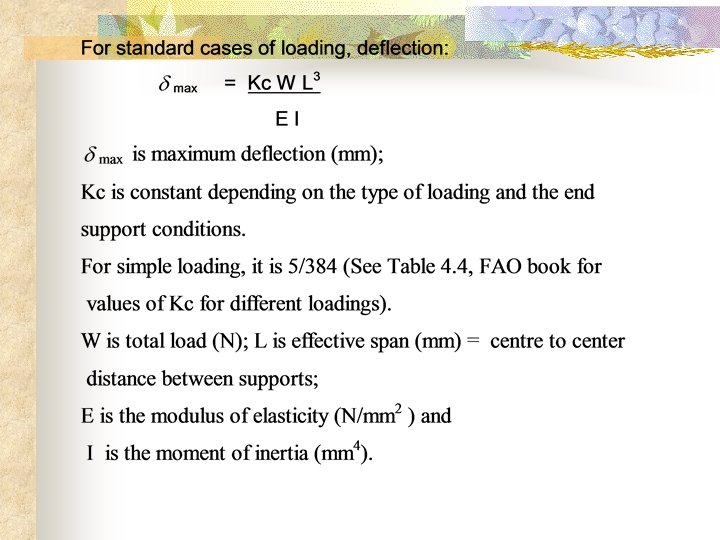
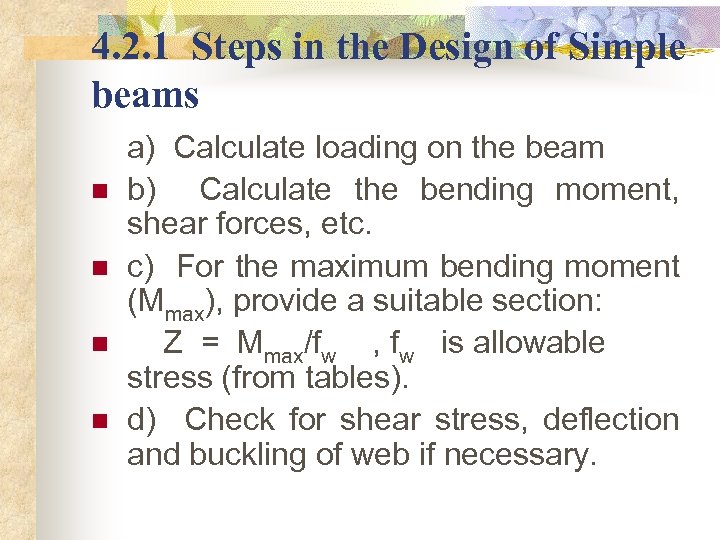
4. 2. 1 Steps in the Design of Simple beams a) Calculate loading on the beam n b) Calculate the bending moment, shear forces, etc. n c) For the maximum bending moment (Mmax), provide a suitable section: n Z = Mmax/fw , fw is allowable stress (from tables). n d) Check for shear stress, deflection and buckling of web if necessary.

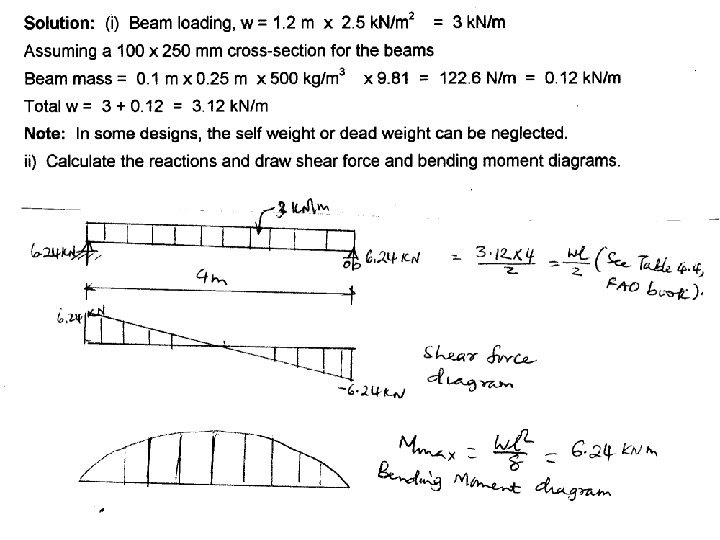
Example n Consider a floor where beams are spaced at 1200 mm and have a span of 4000 mm. The beams are seasoned cypress with the following properties: fw = 8 N/mm 2 , E = 8400 N/mm 2 , density = 500 kg/m 3 . Loading on floor and including floor is 2. 5 k. N/m 2. Allowable deflection is L/240. Design the beam. Allowable shear stress is 0. 7 N/mm 2

4. 2. 2 Bending Moment Caused By Askew Loads n n When the resulting bending moment on a beam is not about one of the axis, the moment need be resolved into components acting about the main axis. The stresses are then calculated separately relative to each axis and the total stress is found by adding the stresses caused by the components of the moment.

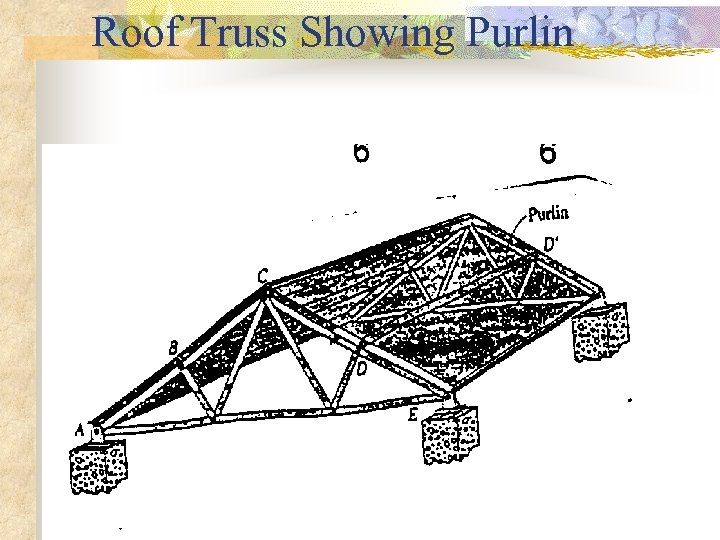
Roof Truss Showing Purlin

Example n Design a timber purlin, which will span rafters 2. 4 m on centre. The angle of the roof slope is 30 ° and the purlin will support a vertical dead load of 250 N/m and a wind load of 200 N/m acting normal to the roof. The allowable bending stress(fw ) for the timber used is 8 N/mm 2. The timber density is 600 kg/m 3

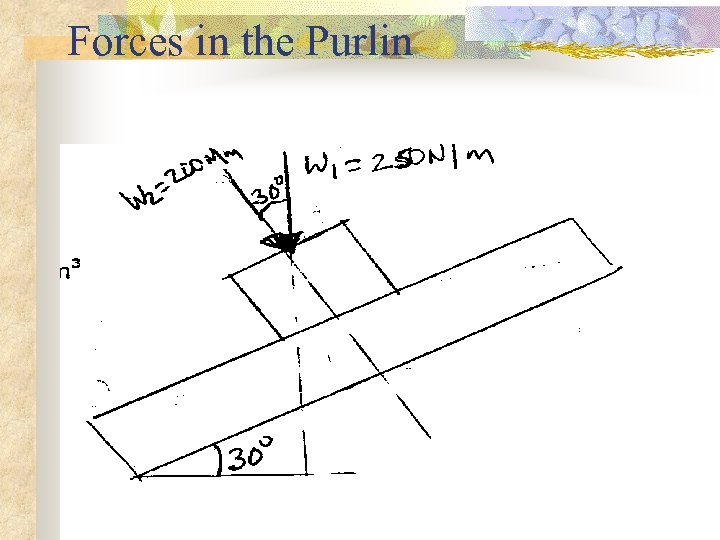
Forces in the Purlin

Solution n n n Assume a purlin cross sectional size of 50 x 125 mm i) Find an estimated self load w = 0. 05 m x 0. 125 m x 600 kg/m 3 x 9. 81 = 37 N/m Total dead load = 250 + 37 = 287 N/m ii) Find the components of the loads relative to the main axes wx = 200 N/m + 287 N/m cos 30 ° = 448. 5 N/m wy = 287 N/m sin 30 ° = 143. 5 N/m

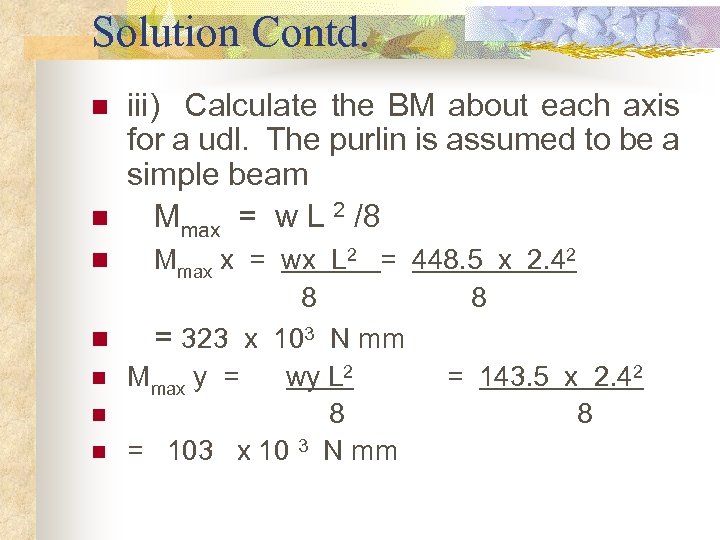
Solution Contd. n n n iii) Calculate the BM about each axis for a udl. The purlin is assumed to be a simple beam Mmax = w L 2 /8 Mmax x = wx L 2 = 448. 5 x 2. 42 8 n = 323 x 103 N mm n Mmax y = wy L 2 = 143. 5 x 2. 42 n 8 n = 103 x 10 3 N mm

Solution Contd. iv) The actual stress in the timber must be less than the allowable stress. n f = Mmax x + Mmax y < fw Zx Zy n v) Try the assumed purlin size of 50 x 125 mm n Zx = b d 2 = 50 x 1252 = 130 x 10 3 mm 3 n 6

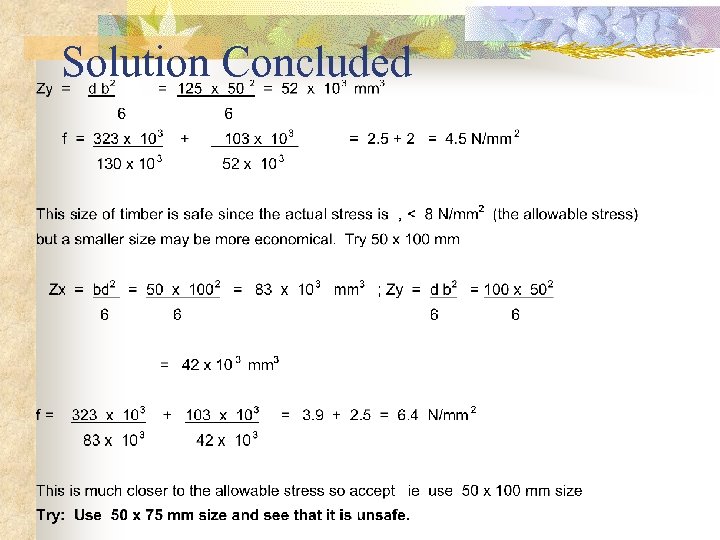
Solution Concluded

4. 2. 3 Design Using the Universal Steel Beams

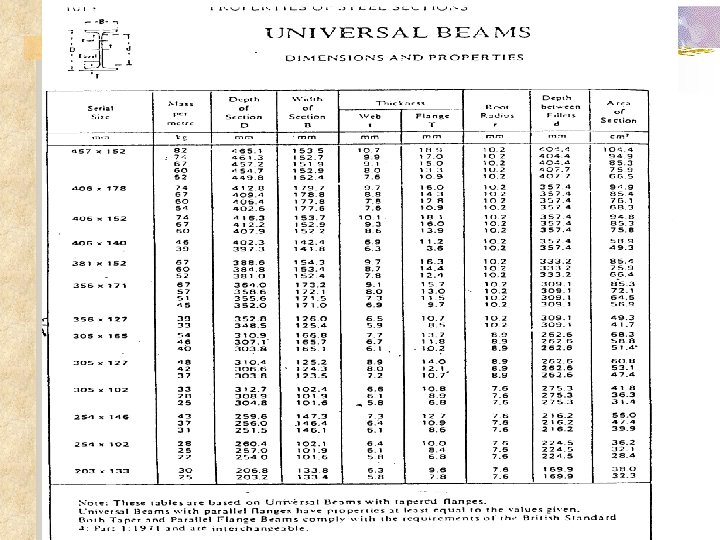
Example n A steel beam used as a lintel over a door opening is required to span 4. 5 m between centres of simple supports. The beam will carry a 220 mm thick and 3. 2 m high brick wall, weighing 20 k. N/m 3 . Allowable bending stress is 165 N/mm 2. Assume allowable shear stress of 100 N/mm 2, E is 2 x 105 N/mm 2. Assume self weight of the beam as 1. 5 k. N.

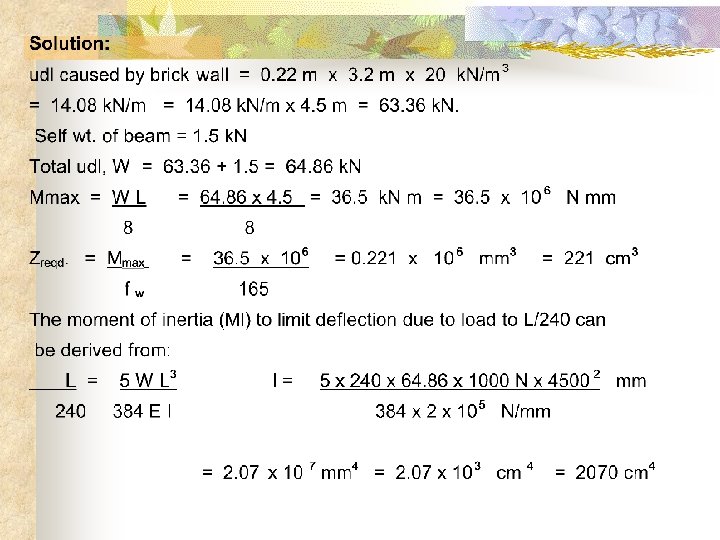
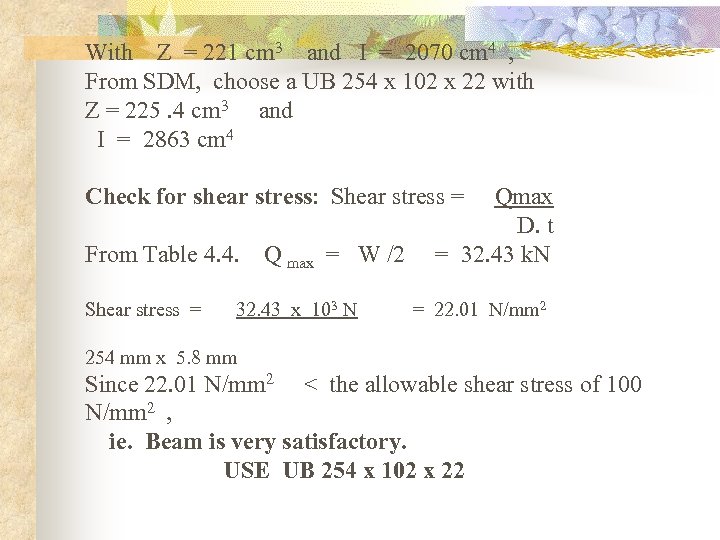
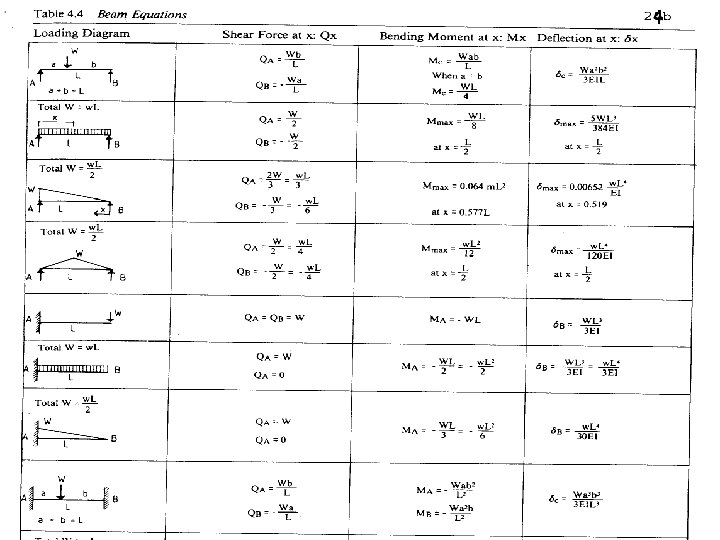
With Z = 221 cm 3 and I = 2070 cm 4 , From SDM, choose a UB 254 x 102 x 22 with Z = 225. 4 cm 3 and I = 2863 cm 4 Check for shear stress: Shear stress = From Table 4. 4. Shear stress = Q max = W /2 32. 43 x 103 N Qmax D. t = 32. 43 k. N = 22. 01 N/mm 2 254 mm x 5. 8 mm Since 22. 01 N/mm 2 < the allowable shear stress of 100 N/mm 2 , ie. Beam is very satisfactory. USE UB 254 x 102 x 22

Composite Beams

4. 3 DESIGN OF COLUMNS n n n Columns are compression members but the manner in which they tend to fail and the amount of load which causes failure depend on: i) The material of which the column is made eg. a steel column carry a greater load than timber column of similar cross-sectional size. ii) The shape of the cross-section of the column. A column having high c/s area compared to the height is likely to fail by crushing rather than by buckling.

Columns Contd. n n n iii) The end conditions of the column. To account for buckling of slender columns, the allowable compressive strength is reduced by a factor k , which depends on the slenderness ratio and the material used. Pbw = k . cw . A where Pbw is the allowable load wrt buckling; k is the reduction factor which depends on the slenderness ratio and A is the cross-sectional area of the column.

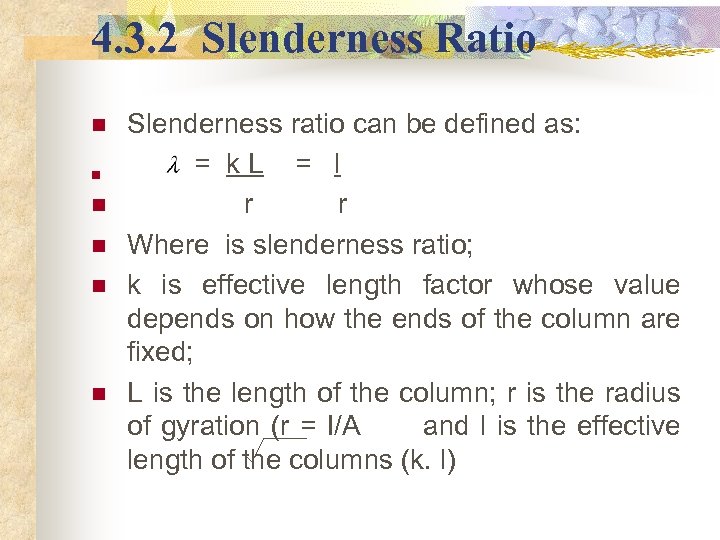
4. 3. 2 Slenderness Ratio n n n Slenderness ratio can be defined as: = k L = l r r Where is slenderness ratio; k is effective length factor whose value depends on how the ends of the column are fixed; L is the length of the column; r is the radius of gyration (r = I/A and l is the effective length of the columns (k. l)

4. 3. 3 Types of End Conditions of a Column: n n n Columns can either be (a) fixed in position nor direction (the weakest condition); (b) fixed in position but not in direction (pinned); (c) fixed in direction but not in position (d) fixed in position and in direction

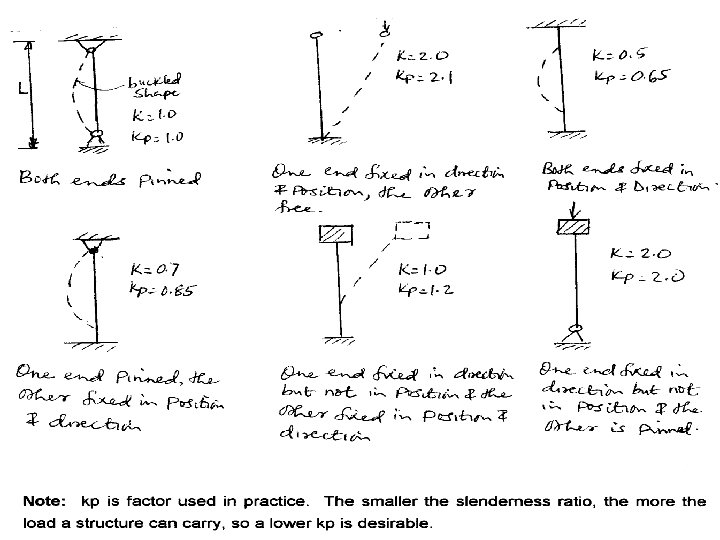
4. 3. 4 Design of Axially Loaded Timber Columns Timber columns are designed with the following formulae: = k L and Pbw = k . cw. A n r n NB: In some building codes, a value of slenderness ratio in case of sawn timber is taken as l/b instead of l/r n

Example n Design a timber column which is 3 m long with a compressive load of 15 k. N. Allowable compressive stress ( cw) for the timber is 5. 2 N/mm 2, kp is 1. 00

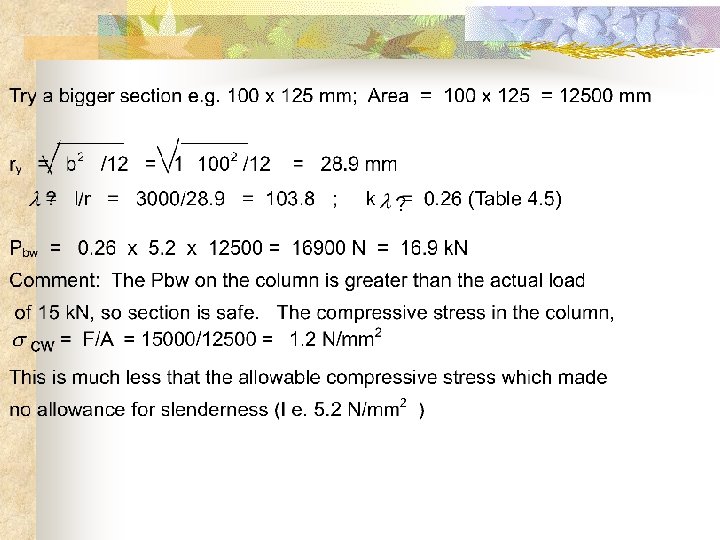
Note n n Actual load/allowable load = 15 k. N/16. 9 k. N = 0. 89 This ratio is all right and shows that the section is economical. A ratio of 0. 85 to 1. 00 is acceptable.

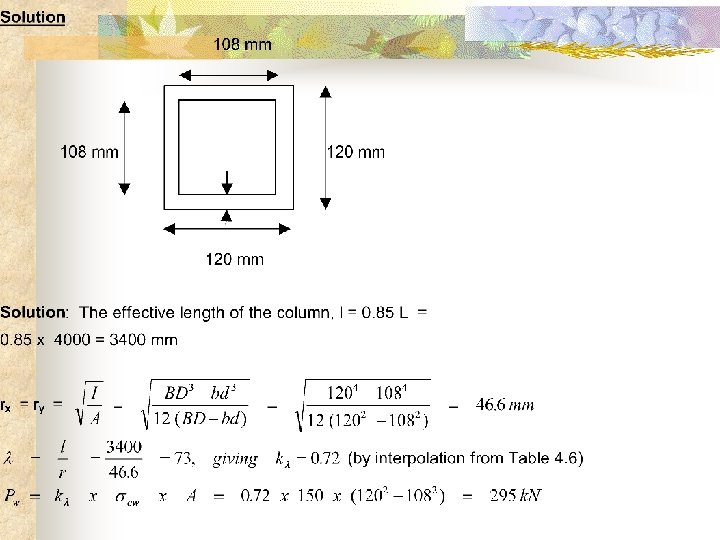
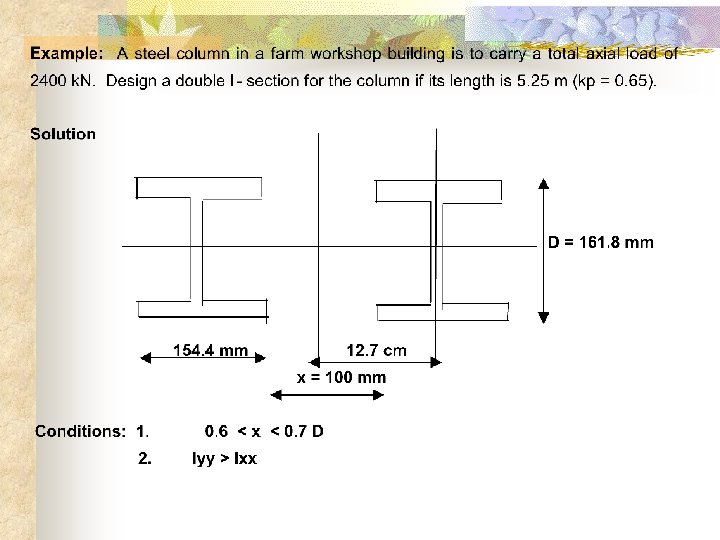
4. 3. 5 Design of Axially Loaded Steel Columns n n The allowable loads for steel with respect to buckling can be calculated in the same manner as for timber. The relationship between the slenderness ratio and the reduction factor ( k ) is slightly different (see Table 4. 6).

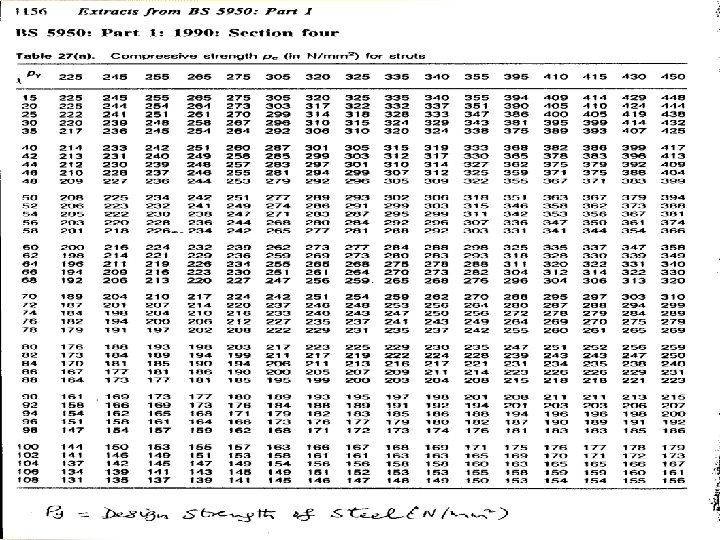
Solution Concluded

4. 3. 7 Axially Loaded Concrete Columns concrete (no reinforcement) only in short columns ie. where the l/r ratio is < 15. For l/r between 10 and 15, the allowable compressive strength must be reduced. The tables of figures relating to l/b in place of a true slenderness ratio are only approximate, since radii of gyration depend on both b and d values in the cross section and must be used with caution.

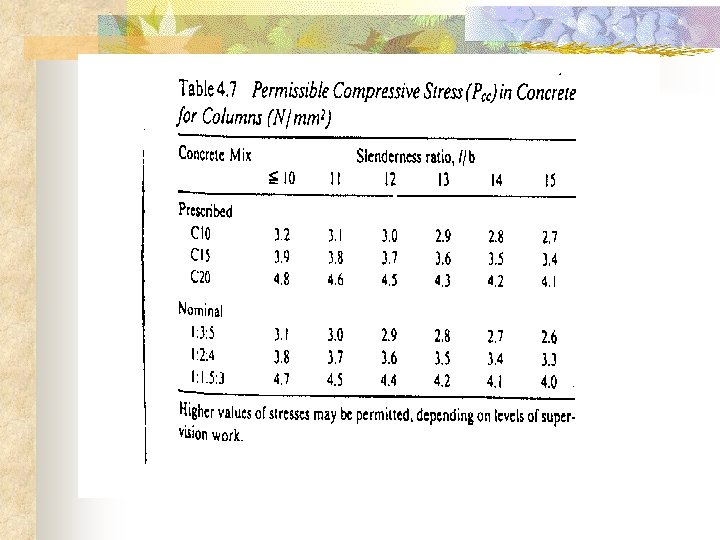
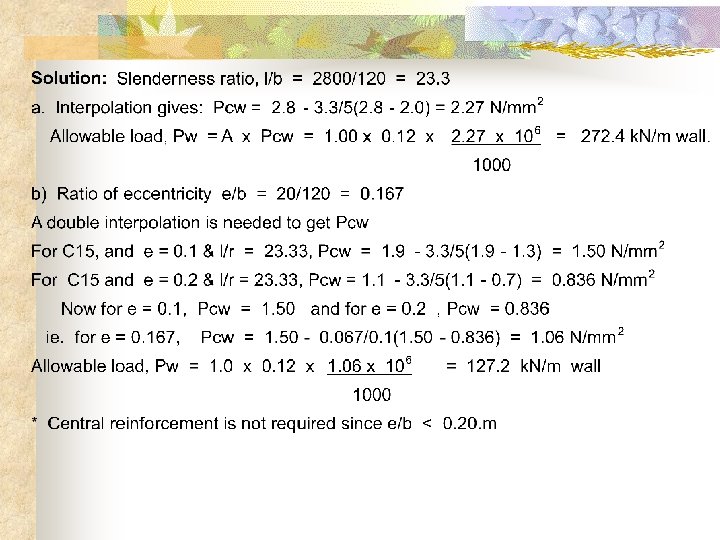
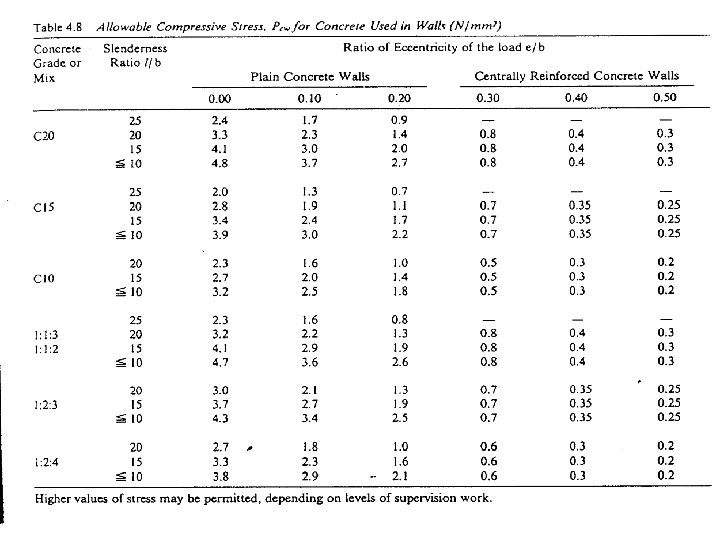
Example n n n A concrete column, with an effective length of 4 m has a cross section of 300 x 400 mm. Calculate the allowable axial load, if a nominal concrete mix is 1: 2: 4 is to be used. Solution: Slenderness ratio, l/b = 4000/300 = 13. 3 Hence Table 4. 7, gives Pcc = 3. 47 N/mm 2 by interpolation. Pw = Pcc. A = 3. 47 x 300 x 400 = 416. 4 k. N

4. 3. 8 Plain & Centrally Reinforced Concrete Walls n n n Walls are designed in the same manner as columns, but there a few differences. A wall is different from a column by having a length which is more than five times its thickness. Plain concrete walls should have a minimum thickness of 100 mm.

Plain and Reinforced Concrete Walls Contd. n n Where the load is eccentric, the wall must have centrally placed reinforcement of at least 0. 2 % of the cross section if the eccentricity > 0. 20. This reinforcement may not be included in the load carrying capacity of the wall.

Example n Determine the maximum allowable load per metre of a 120 mm thick wall, with an effective height of 2. 8 m and made from concrete grade C 15: (a) when the load is central and (b) when the load is eccentric by 20 mm.

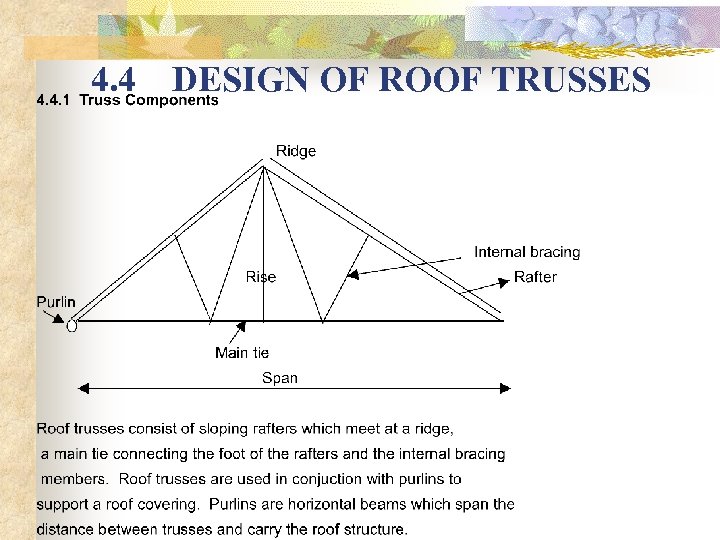
4. 4 DESIGN OF ROOF TRUSSES

Roof Trusses Contd. n n n Steel and timber trusses are usually designed assuming pin-jointed members. In practice, timber trusses are assembled with bolts, nails or special connectors and steel trusses are bolted, riveted or welded. These rigid joint impose secondary stresses which are negligible and therefore not used in design.

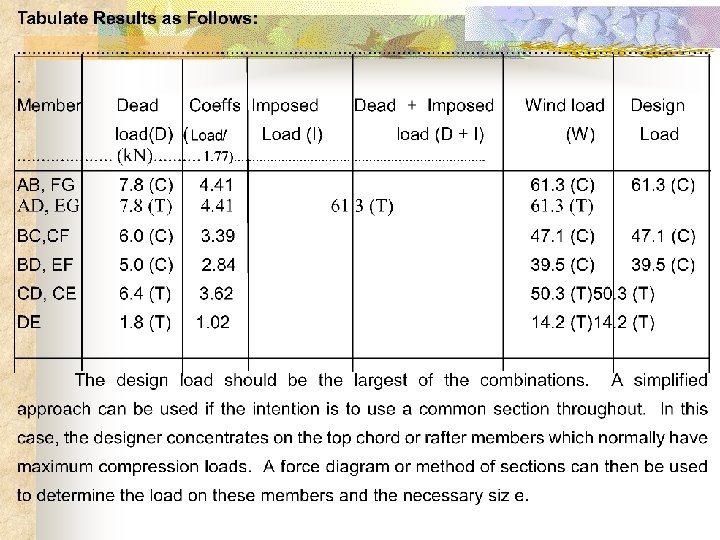
4. 4. 2 Steps in Designing a Truss n n n a) Select general layout of truss members and truss spacing b) Estimate external loads to be applied including self weight of truss, purlins and roof covering, together with wind loads. c) Determine the critical (worst combinations) loading. It is usual to consider dead loads alone, and then dead and imposed load combined.

Steps in Designing a Truss Contd. n d) Analyse framework to find forces in members. n e) Select material and section to provide in each member a stress value which does not exceed the permissible value.

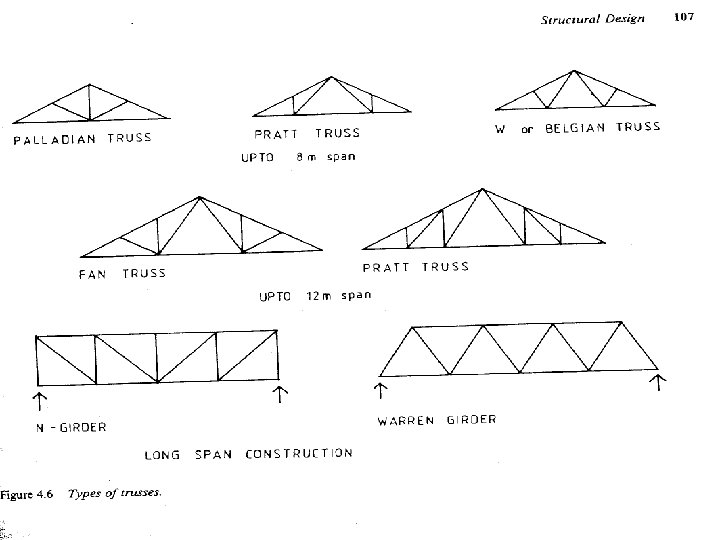
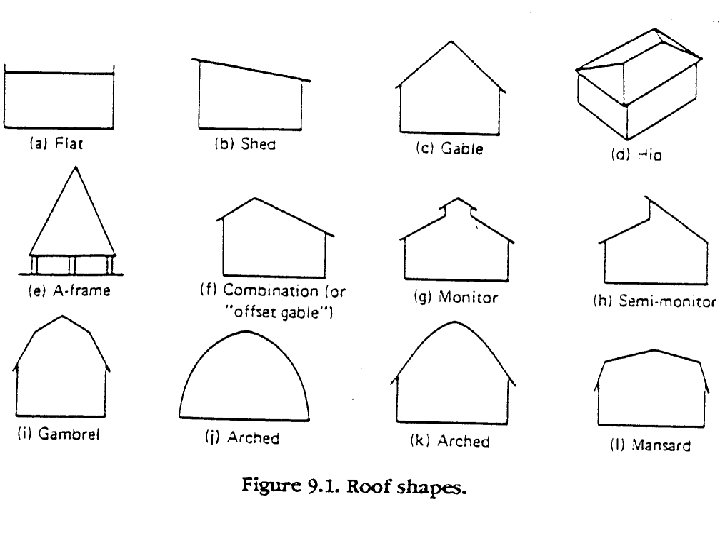
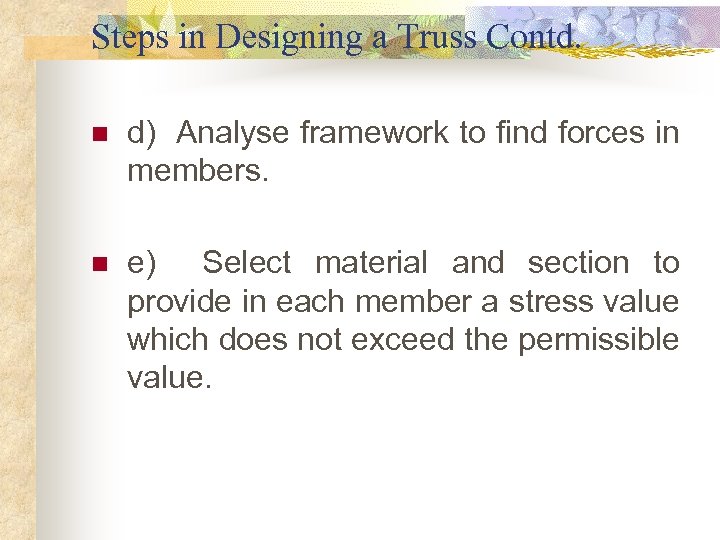
4. 4. 2. 1 Spacing and Layout n Roof trusses should as far as possible be spaced to achieve a minimum of weight and economy of materials used in the total roof structure. n For spans up to 20 m, the spacing of steel trusses is likely to be about 4 m, and in case of timber, 2 m.

Spacing and Layout Contd. n n Short spans up to 8 m should have pitched timber rafters or light weight trusses either pitched or flat. Medium spans of 7 to 15 m or 16 m require truss frames designed of timber or steel. Long spans of over 16 m should if possible be broken into small units or the roofs should be designed by specialists.

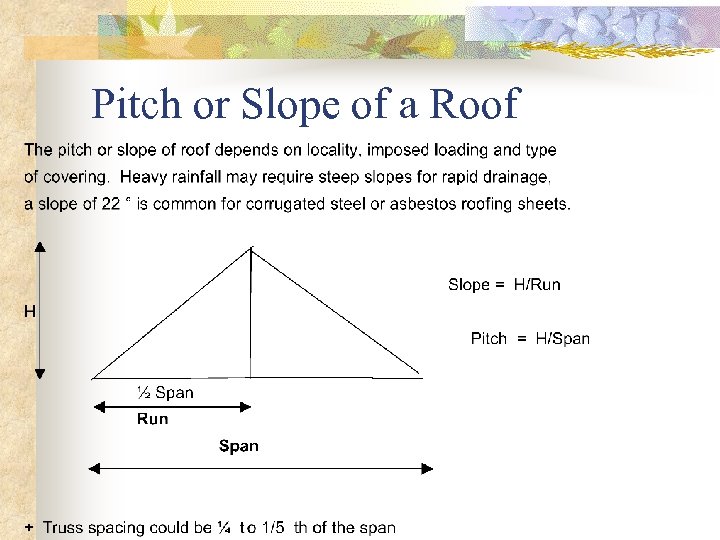
Pitch or Slope of a Roof

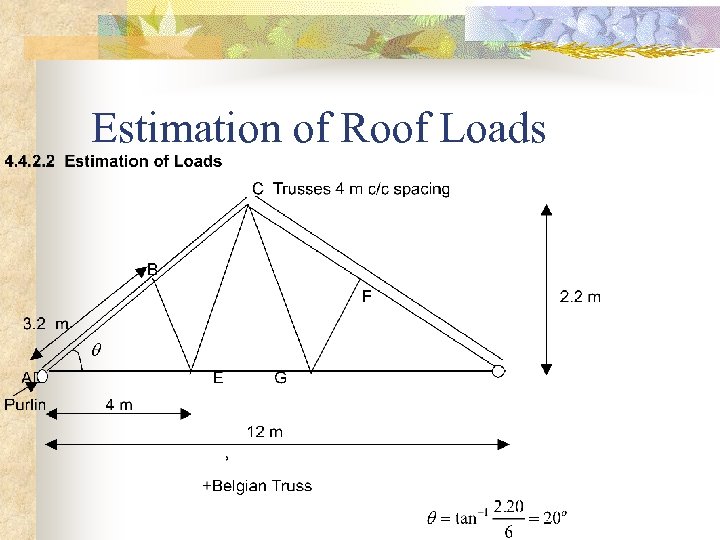
Estimation of Roof Loads

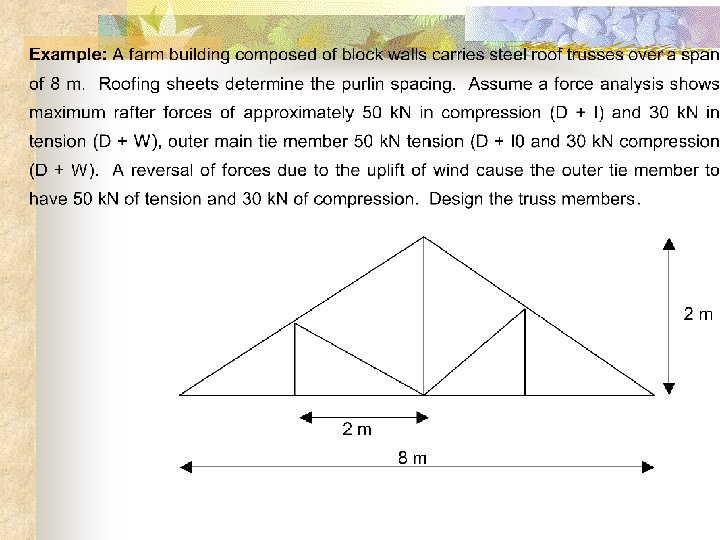
Example n Determine the critical forces for design for each of the members in the left hand of the truss shown above. Assume the following: Trusses are spaced 4 m on centres. The roof deck is of galvanised sheet weighing 6 kg/m 2 . All purlins weigh 22 kg/m. Openings constitute 20% of the wall surface. Calculate the panel loads.

Solution n n n (a) Dead load i) Determine the panel area supported by one purlin = Distance between the purlins x spacing of trusses = 3. 2 m x 4 m = 12. 8 m 2 ii) Calculate the roof deck load supported by one purlin = 12. 8 m 2 x 6 kg/m 2 x 9. 81 = 753. 4 N = 0. 75 k. N. iii) Weight of each purlin = 22 kg/m x 4 m x 9. 81 = 863. 28 N = 0. 86 k. N

Solution Contd. n n Total dead load to be carried by truss = 0. 75 + 0. 86 k. N = 1. 61 k. N iv)Estimate total truss weight per panel as 10 % of the total load to be carried = 0. 16 k. N v) Total dead load per panel point , P = 1. 61 + 0. 16 = 1. 77 k. N

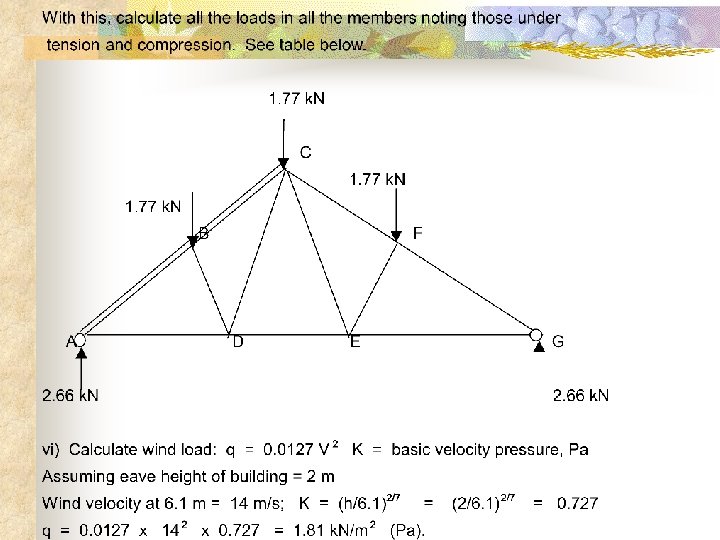
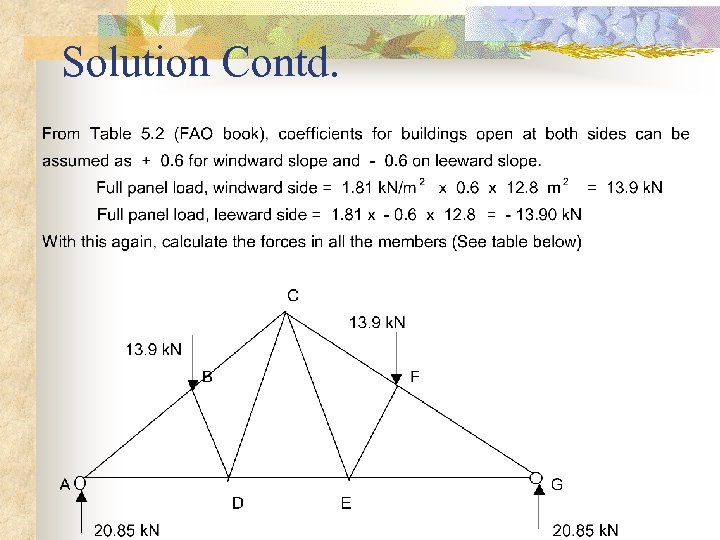
Solution Contd.

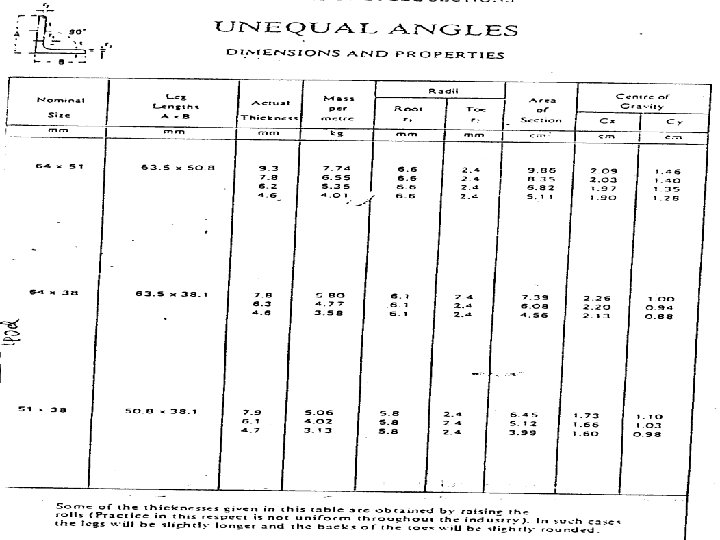
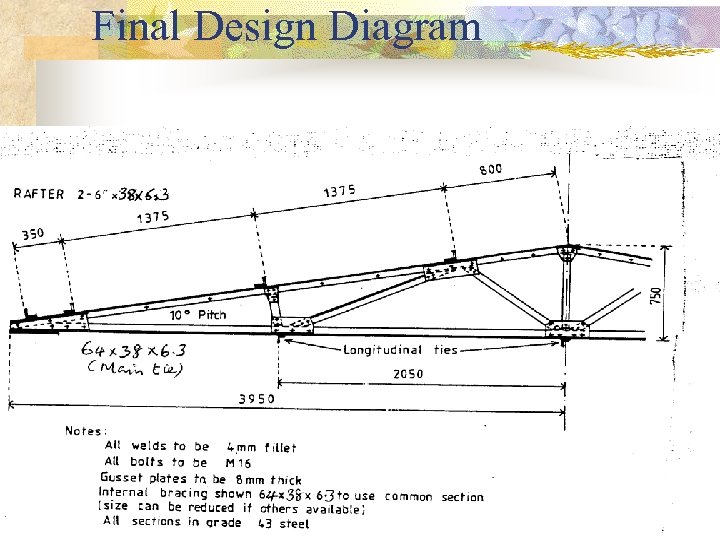
Final Design Diagram

4. 5 FOUNDATIONS AND FOOTINGS DESIGN n n n 4. 5. 1 Introduction: Foundation is the part of the structure through which the load of the structure is transmitted to the ground. A combination of footing and foundation distributes the load on the bearing surface (the soil) and keeps the building level and plumb and reduces settling to a minimum. Footing and foundation are normally made of concrete, no matter the construction material.

Footing and Foundation Contd. n Before design of footing and foundation can be made, the total load from the building as well as soil bearing characteristics should be determined.

Soil Bearing Capacity n n Strength of the soil required to resist the loads resting in it. This is after the top soil has been removed (See Table 5. 6, FAO book for values of soil bearing capacities). Detailed investigations of the soil is not usually required for small scale buildings

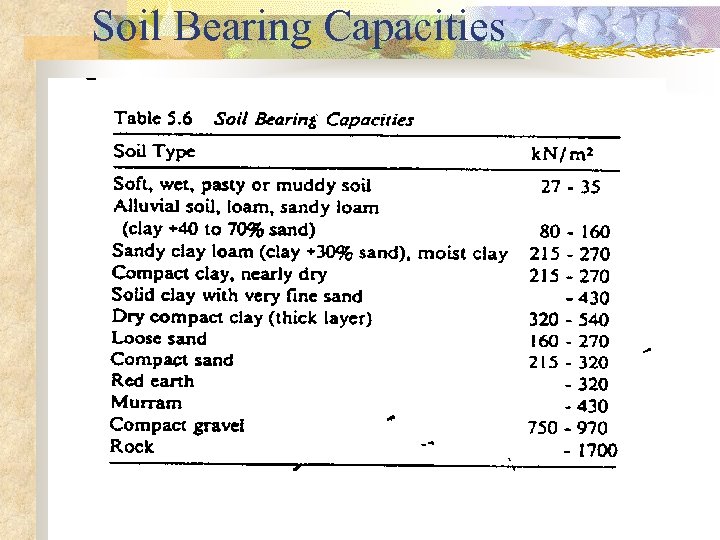
Soil Bearing Capacities

4. 5. 3 Foundation Footings 4. 5. 3. 1 Description: A footing is an enlarged base for a foundation designed to distribute the building load over a larger area of soil and to provide a firm, level surface for constructing the foundation wall. Foundation wall Footing

Footings and Foundation Contd. n n The footing is wider than the foundation wall because the soil's bearing stress is less than that of the material (concrete) of the wall e. g. concrete has a strength of about 1000 k. N/m 2 . A 1 : 3 : 5 ratio of cement, dry sand, and gravel is suggested for footings with 31 litres of water per 50 kg sack of cement.

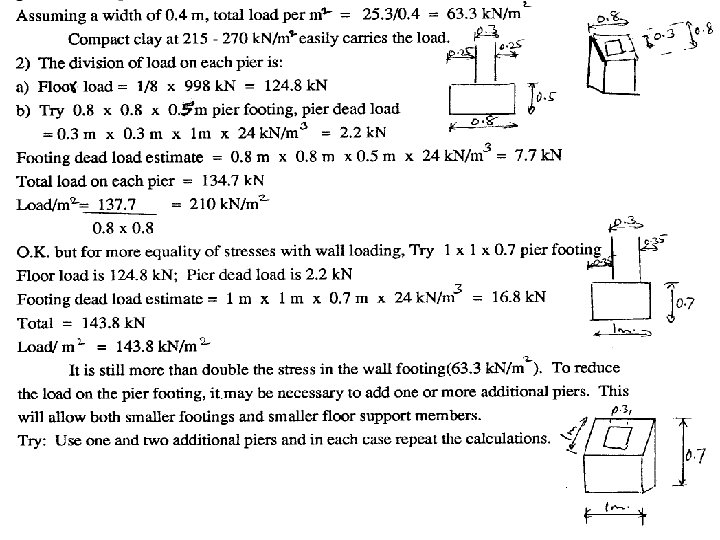
Note n n Unlike the continuous wall footings, the pier and column footings are heavily loaded. It is very important to correctly estimate the proportion of building load to be carried by each pier or column. If wall footings are very lightly loaded, it is advisable to design any pier or column footings required for the building with approximately the same load per unit area. If any settling occurs, it should be uniform throughout.

Example n For the building below, the loads are as follows: n a) The roof framing plus the expected wind load = 130 k. N b) The wall above the foundation is 0. 9 k. N/m c) The floor will be used for grain storage and will support as much as 7. 3 k. N/m 2 . The floor structure is an additional 0. 5 k. N/m 2 The foundation walls and piers are each 1 m high above the footing. The wall is 200 mm thick and the piers 300 mm square. The soil on the site is compacted clay in a well drained area. Find the size of the foundation and pier footing that will safely support the loads. Assume that the weight of the mass 1 kg approximately equals 10 N. The mass of concrete is 24 k. N/m 3. n n n

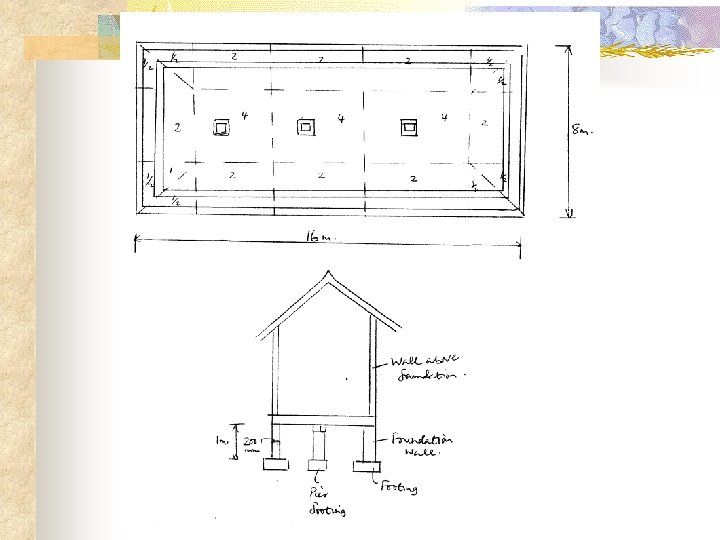
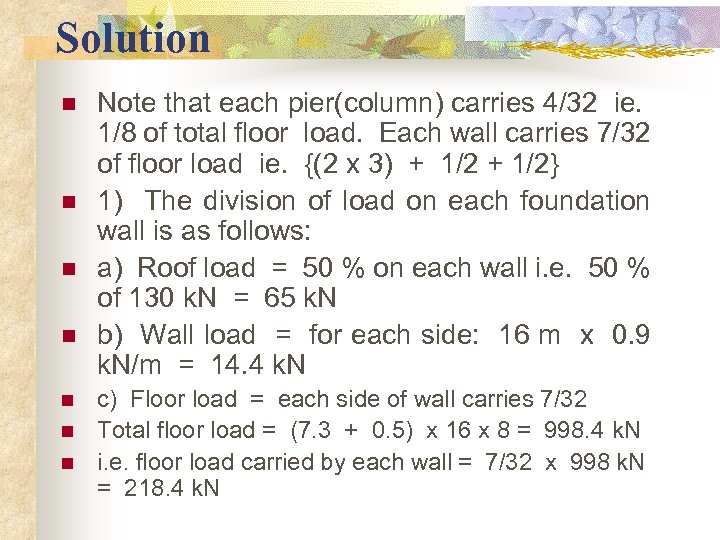
Solution n n n Note that each pier(column) carries 4/32 ie. 1/8 of total floor load. Each wall carries 7/32 of floor load ie. {(2 x 3) + 1/2} 1) The division of load on each foundation wall is as follows: a) Roof load = 50 % on each wall i. e. 50 % of 130 k. N = 65 k. N b) Wall load = for each side: 16 m x 0. 9 k. N/m = 14. 4 k. N c) Floor load = each side of wall carries 7/32 Total floor load = (7. 3 + 0. 5) x 16 x 8 = 998. 4 k. N i. e. floor load carried by each wall = 7/32 x 998 k. N = 218. 4 k. N

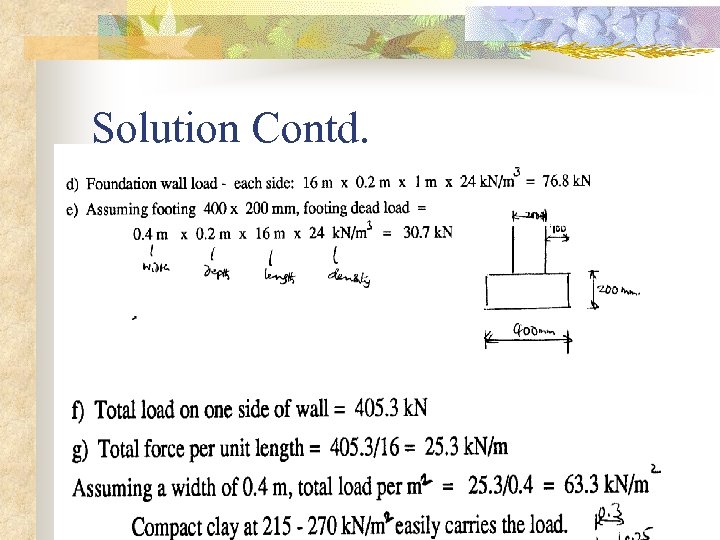
Solution Contd.