Max-Planck Institut für Plasmaphysik Introduction to Nuclear Fusion

Max-Planck Institut für Plasmaphysik Introduction to Nuclear Fusion H. -S. Bosch Max-Planck-Institut für Plasmaphysik, IPP-Euratom Association, D-85748 Garching Fusion in the stars Fusion research on earth - Inertial Confinement Fusion - Magnetic Confinement Fusion (MCF) Prospects for a fusion reactor IPP Summer University on Plasma Physics, Garching, Germany, 17 -21 Septemb HSB, SU 2001, 17. 9. 01

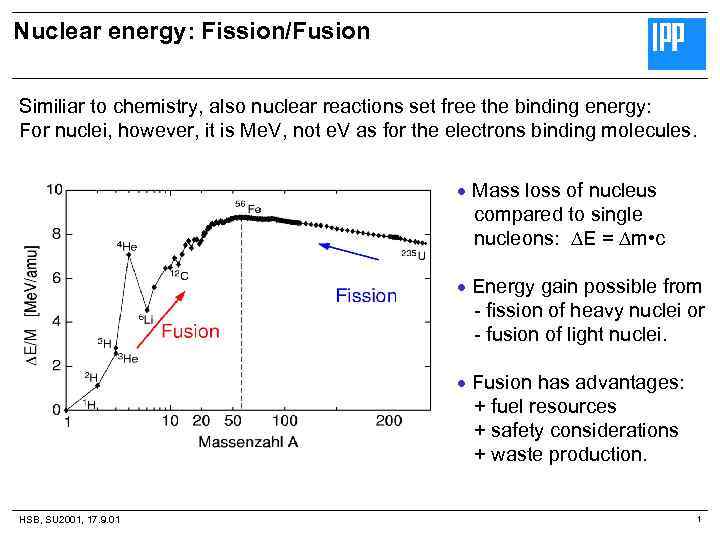Nuclear energy: Fission/Fusion Similiar to chemistry, also nuclear reactions set free the binding energy: For nuclei, however, it is Me. V, not e. V as for the electrons binding molecules. Mass loss of nucleus compared to single nucleons: E = m • c Energy gain possible from - fission of heavy nuclei or - fusion of light nuclei. Fusion has advantages: + fuel resources + safety considerations + waste production. HSB, SU 2001, 17. 9. 01 1

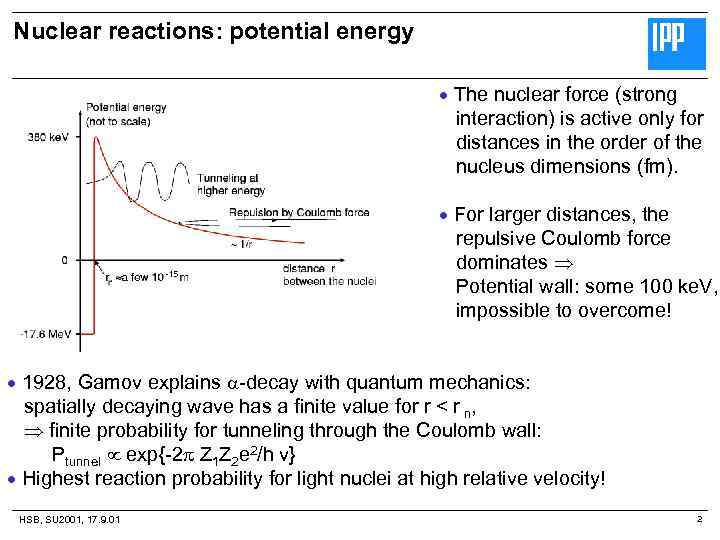Nuclear reactions: potential energy The nuclear force (strong interaction) is active only for distances in the order of the nucleus dimensions (fm). For larger distances, the repulsive Coulomb force dominates Potential wall: some 100 ke. V, impossible to overcome! 1928, Gamov explains -decay with quantum mechanics: spatially decaying wave has a finite value for r < r n, finite probability for tunneling through the Coulomb wall: Ptunnel exp{-2 Z 1 Z 2 e 2/h v} Highest reaction probability for light nuclei at high relative velocity! HSB, SU 2001, 17. 9. 01 2

Fusion is the energy source of the stars The sun produces continously energy, with a total power of 3. 6 • 1017 GW. In doing so, it converts per second 600 Mio. tons of hydrogen into 596 Mio. tons of helium. The power flux arriving on earth is 1. 4 k. W/m 2 (above the atmosphere, without absoprtion). NASA, Skylab space station December 19, 1973, solar flare reaching 588 000 km off solar surface HSB, SU 2001, 17. 9. 01 3

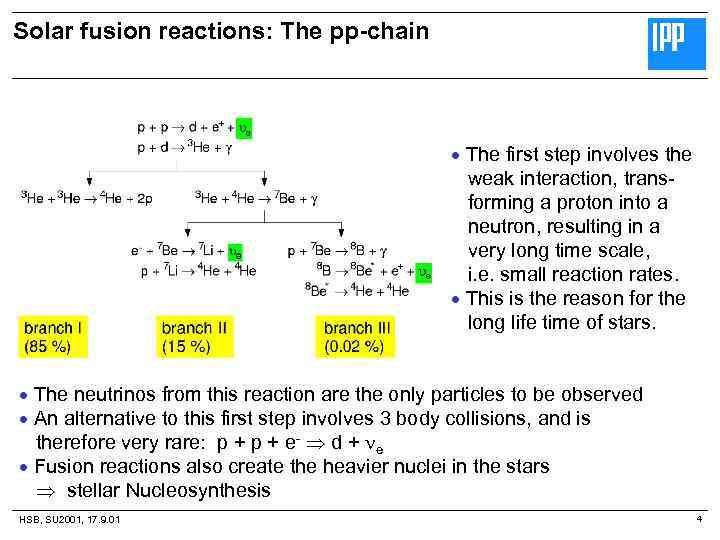Solar fusion reactions: The pp-chain The first step involves the weak interaction, transforming a proton into a neutron, resulting in a very long time scale, i. e. small reaction rates. This is the reason for the long life time of stars. The neutrinos from this reaction are the only particles to be observed An alternative to this first step involves 3 body collisions, and is therefore very rare: p + e- d + e Fusion reactions also create the heavier nuclei in the stars stellar Nucleosynthesis HSB, SU 2001, 17. 9. 01 4

Solar fusion reactions: The pp-chain (II) Early observations of neutrinos in a chlorine detector (Davis, Homestake gold mine, South Dakota, 1964) found only ~ 30% of the expected neutrino flux. Sensitive only to neutrinos above 814 ke. V: 37 Cl+ e 37 Ar + e Measurements with a Cerenkov detector (700 tons H 2 O, Kamiokande, 1989) saw ~ 44% of the expected neutrinos. Only in 1992 (GALLEX, Gran Sasso and SAGE, Caucasus), the low energy neutrinos could be observed: 37 Ga + e 37 Ge + e These experiments found 50% and 58%, respectively, of the expected flux. Solar modell? or Neutrino oscillations? First indications of oscillations in Super-Kamiokande, 1998. HSB, SU 2001, 17. 9. 01 5

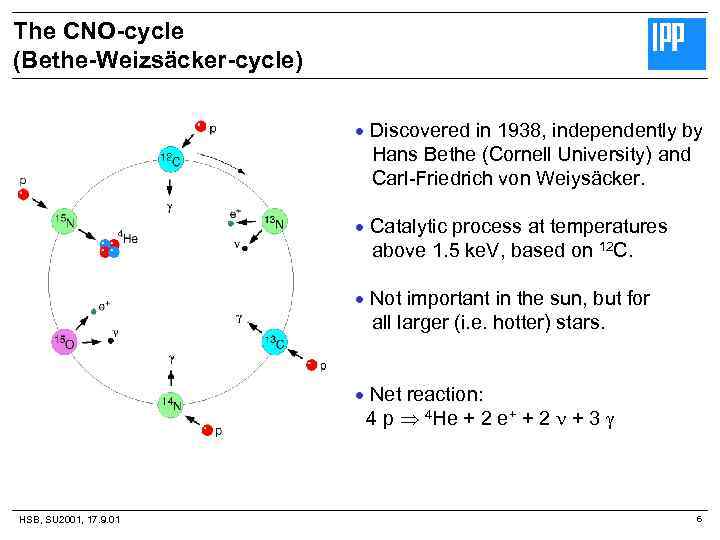The CNO-cycle (Bethe-Weizsäcker-cycle) Discovered in 1938, independently by Hans Bethe (Cornell University) and Carl-Friedrich von Weiysäcker. Catalytic process at temperatures above 1. 5 ke. V, based on 12 C. Not important in the sun, but for all larger (i. e. hotter) stars. Net reaction: 4 p 4 He + 2 e+ + 2 + 3 HSB, SU 2001, 17. 9. 01 6

For a terrestial energy source we need different fusion reactions! The weak interaction makes the pp-chain a rather slow reaction. => long lifetime of stars. The huge mass of the sun makes up for that easily, still resulting in a large power production. However, for power production on earth, the weak interaction has to be avoided. For the small volume we can afford, we need faster fusion reactions. HSB, SU 2001, 17. 9. 01 7

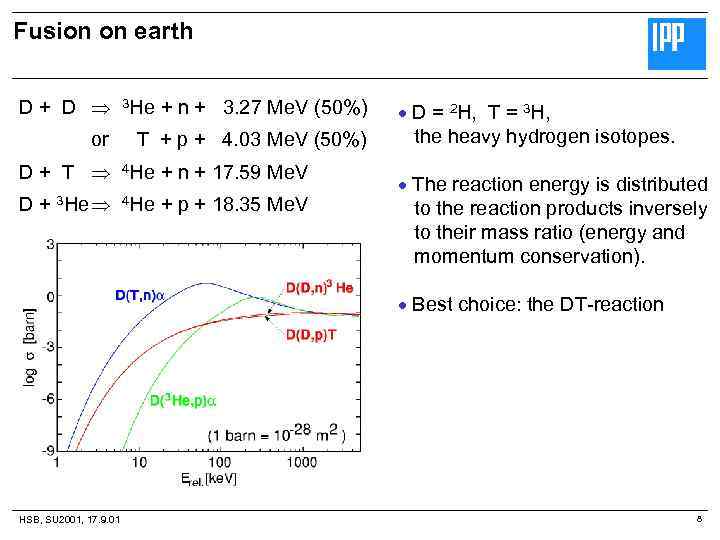Fusion on earth D + D 3 He + n + 3. 27 Me. V (50%) or T + p + 4. 03 Me. V (50%) D + T 4 He + n + 17. 59 Me. V D + 3 He 4 He + p + 18. 35 Me. V D = 2 H, T = 3 H, the heavy hydrogen isotopes. The reaction energy is distributed to the reaction products inversely to their mass ratio (energy and momentum conservation). Best choice: the DT-reaction HSB, SU 2001, 17. 9. 01 8

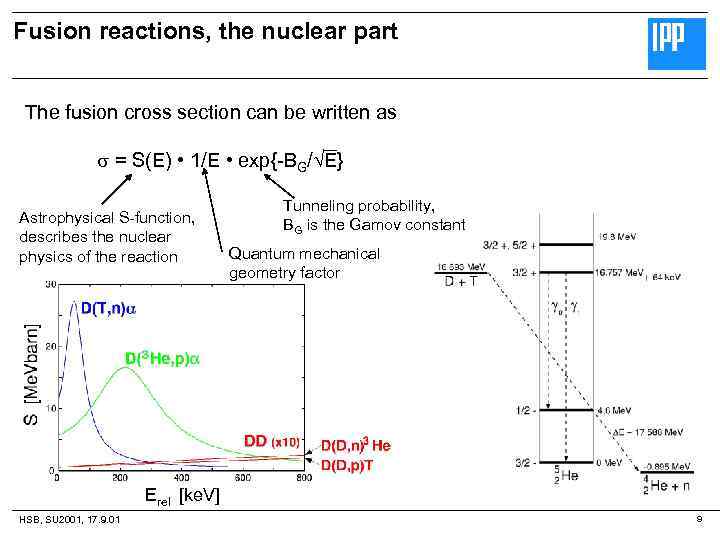Fusion reactions, the nuclear part The fusion cross section can be written as = S(E) • 1/E • exp{-BG/ E} Astrophysical S-function, describes the nuclear physics of the reaction Tunneling probability, BG is the Gamov constant Quantum mechanical geometry factor Erel [ke. V] HSB, SU 2001, 17. 9. 01 9

Fusion fuels Deuterium exists with a weight fraction of 3. 3 • 10 -5 in water static range of billions of years. Tritium is a radioactive isotope and decays with a half life of 12. 33 years: T He + e- + e no natural tritium available, but production with fusion produced neutrons is possible: n + 6 Li 4 He + T + 4. 8 Me. V n + 7 Li 4 He + T + n‘ - 2. 5 Me. V The latter reaction allows self-sufficient tritium breeding. Lithium is very abundant and widespread (in the earth‘s crust and in the ocean water), sufficient for at least 30 0000 years. HSB, SU 2001, 17. 9. 01 10

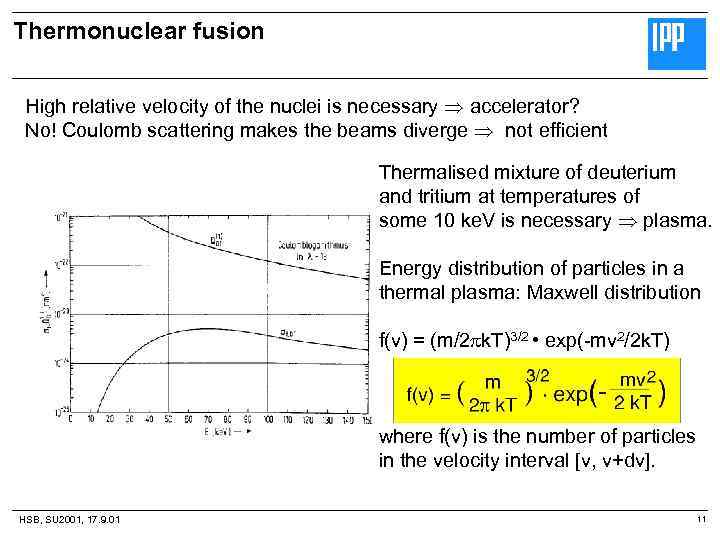Thermonuclear fusion High relative velocity of the nuclei is necessary accelerator? No! Coulomb scattering makes the beams diverge not efficient Thermalised mixture of deuterium and tritium at temperatures of some 10 ke. V is necessary plasma. Energy distribution of particles in a thermal plasma: Maxwell distribution f(v) = (m/2 k. T)3/2 • exp(-mv 2/2 k. T) where f(v) is the number of particles in the velocity interval [v, v+dv]. HSB, SU 2001, 17. 9. 01 11

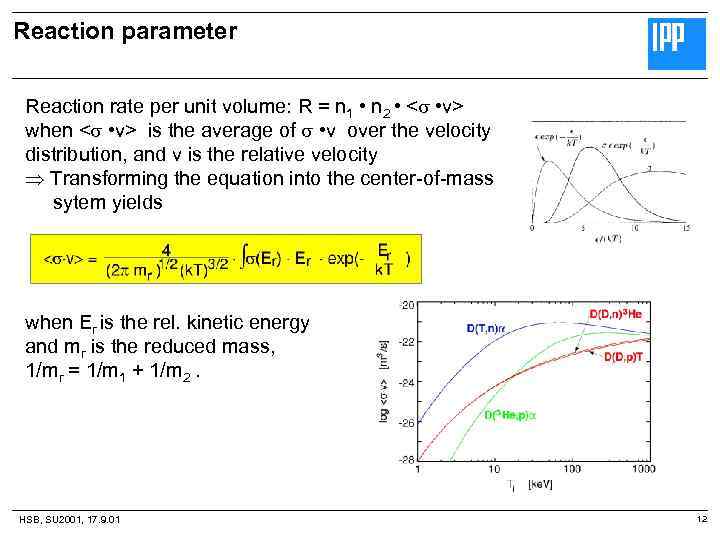Reaction parameter Reaction rate per unit volume: R = n 1 • n 2 • < • v> when < • v> is the average of • v over the velocity distribution, and v is the relative velocity Transforming the equation into the center-of-mass sytem yields < • v> (Er) • Er • exp(-Er/k. T) when Er is the rel. kinetic energy and mr is the reduced mass, 1/mr = 1/m 1 + 1/m 2. HSB, SU 2001, 17. 9. 01 12

Lawson Criterion In 1957 Lawson introduced power balances: Break-even: The fusion power equals the loss by radiation, (when c 1 = 5. 4 • 10 -37 Wm 3 ke. V-1/2, and Zeff = ni. Zi 2/n is the effective plasma charge), and by transport (diffusion, convection, Charge-Exchange): With n. D= n. T= n/2, Ti =Te =T we find a condition for the fusion product n T: Ignition: The neutrons leave the plasma, the -particles are confined and heat it. Only their energy should enter the balance! Efus E HSB, SU 2001, 17. 9. 01 13

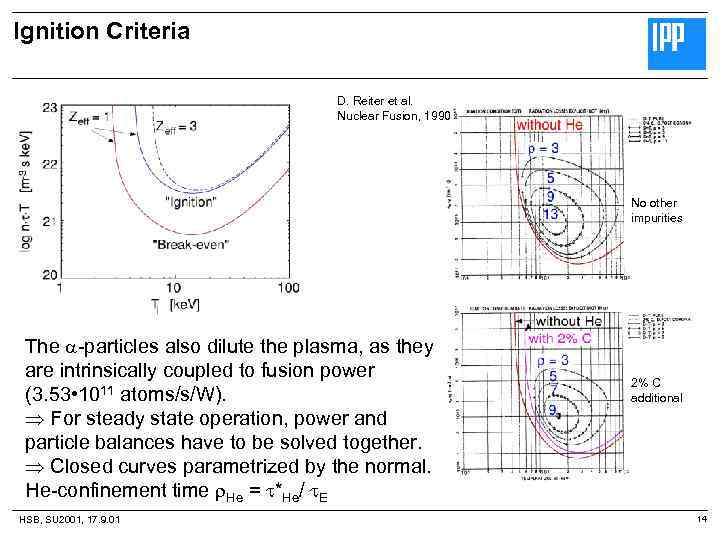Ignition Criteria D. Reiter et al. Nuclear Fusion, 1990 No other impurities The -particles also dilute the plasma, as they are intrinsically coupled to fusion power (3. 53 • 1011 atoms/s/W). For steady state operation, power and particle balances have to be solved together. Closed curves parametrized by the normal. He-confinement time He = *He/ E HSB, SU 2001, 17. 9. 01 2% C additional 14

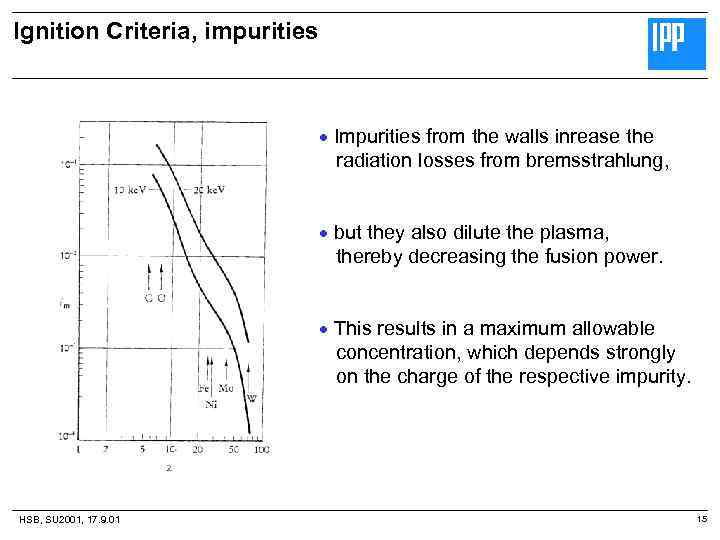Ignition Criteria, impurities Impurities from the walls inrease the radiation losses from bremsstrahlung, but they also dilute the plasma, thereby decreasing the fusion power. This results in a maximum allowable concentration, which depends strongly on the charge of the respective impurity. HSB, SU 2001, 17. 9. 01 15

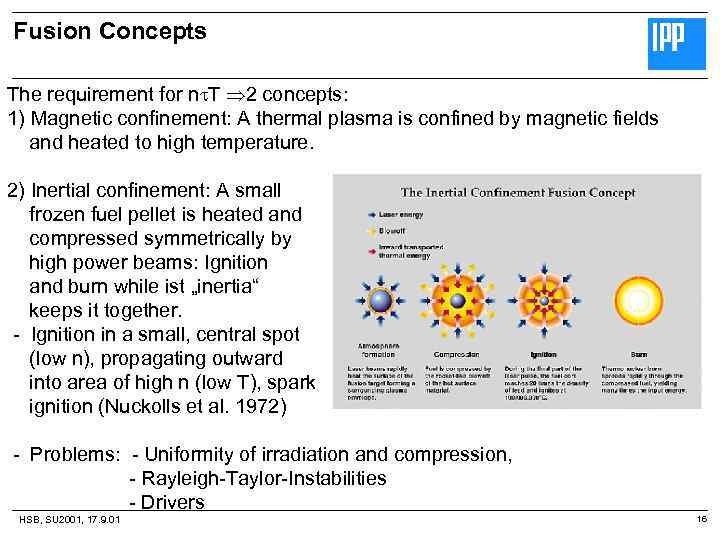Fusion Concepts The requirement for n T 2 concepts: 1) Magnetic confinement: A thermal plasma is confined by magnetic fields and heated to high temperature. 2) Inertial confinement: A small frozen fuel pellet is heated and compressed symmetrically by high power beams: Ignition and burn while ist „inertia“ keeps it together. - Ignition in a small, central spot (low n), propagating outward into area of high n (low T), spark ignition (Nuckolls et al. 1972) - Problems: - Uniformity of irradiation and compression, - Rayleigh-Taylor-Instabilities - Drivers HSB, SU 2001, 17. 9. 01 16

RT-Instabilities, Homogeneity Uniformity of the ablation front is essential to prevent growth of Rayleigh-Taylor instabilities. 1) High requirement for surface finish of fuel capsules, r/r < 0. 08 m/1. 11 mm = 7 • 10 -8 (NIF-Design). 2) With direct drive, irradiation with many beams and high spatial homogeneity of the beam profile is neccessary. A lot of techniques have been developed, and absorption inhomogeneities of about 3% rms have been achieved (GEKKO, 1996). 3) Alternatively: Hohlraum HSB, SU 2001, 17. 9. 01 18

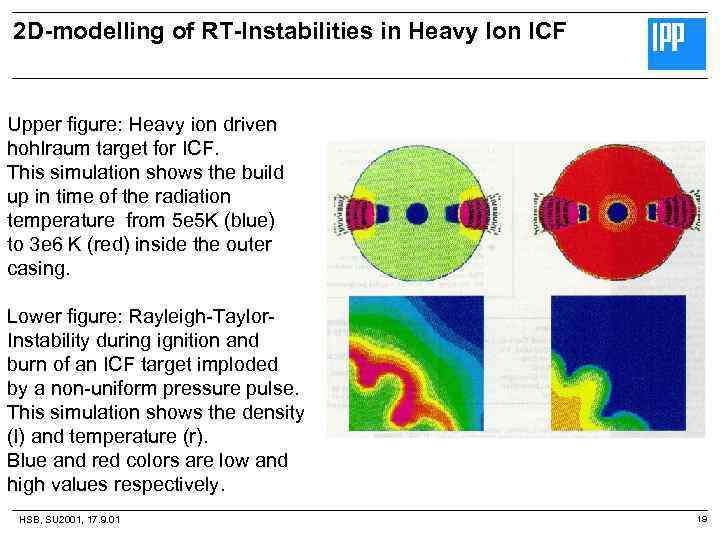2 D-modelling of RT-Instabilities in Heavy Ion ICF Upper figure: Heavy ion driven hohlraum target for ICF. This simulation shows the build up in time of the radiation temperature from 5 e 5 K (blue) to 3 e 6 K (red) inside the outer casing. Lower figure: Rayleigh-Taylor. Instability during ignition and burn of an ICF target imploded by a non-uniform pressure pulse. This simulation shows the density (l) and temperature (r). Blue and red colors are low and high values respectively. HSB, SU 2001, 17. 9. 01 19

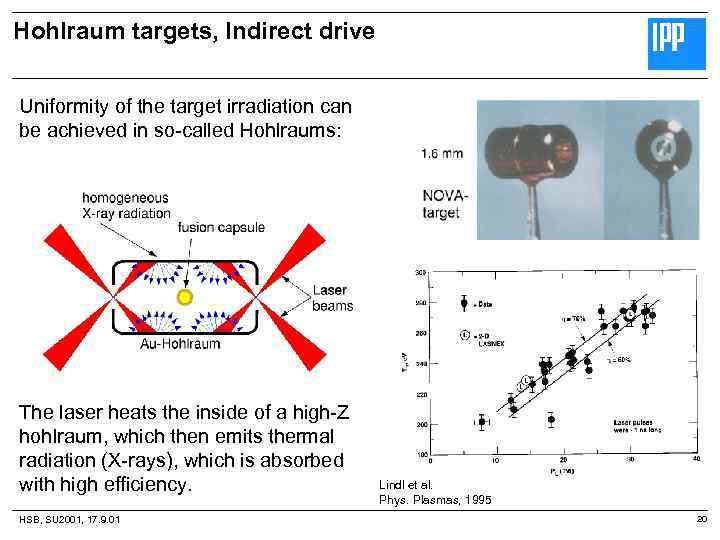Hohlraum targets, Indirect drive Uniformity of the target irradiation can be achieved in so-called Hohlraums: The laser heats the inside of a high-Z hohlraum, which then emits thermal radiation (X-rays), which is absorbed with high efficiency. HSB, SU 2001, 17. 9. 01 Lindl et al. Phys. Plasmas, 1995 20

Drivers I (Lasers) General requirements: Pulse energy: 2 -10 MJ Pulse duration: 10 ns Repetition rate: 1 -10 Hz Energy gain should be larger than 1000 LASER: 1) Neodym glass laser: at = 1. 06 m, pellet too small. Improvement by frequency conversion to 530 nm (70%) or 350 nm (50%). driver < 1%, repetition rate about 1 pulse/2 hrs. GEKKO XII, Osaka 25 k. J in 1 ns, 12 beams NOVA, Livermore 125 k. J in 1 ns, 10 beams Pumping presently by flashlamps (white light) Solid State Diode Pumped Lasers (Yb: S-FAP crystals) with efficiencies up to 20% under development (LLNL, 1998: 1 J). 2) Kr. F gas laser: = 248 nm driver ~ 1%, potenial for development, AURORA, Los Alamos: 10 k. J in 500 ns. HSB, SU 2001, 17. 9. 01 22

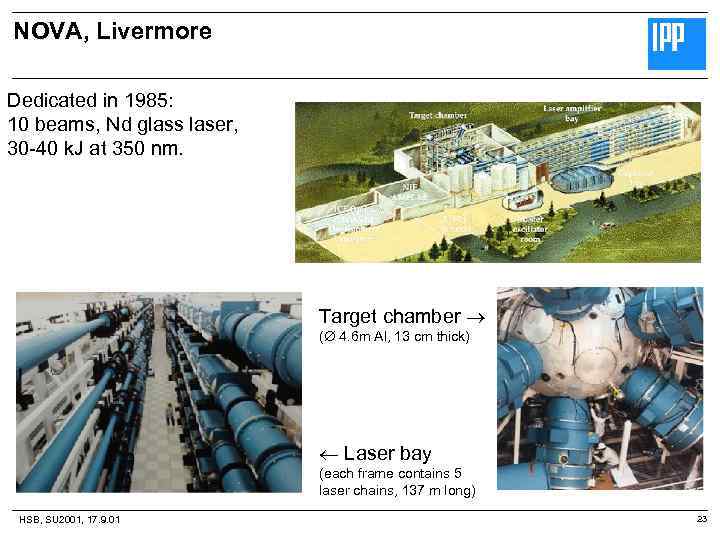NOVA, Livermore Dedicated in 1985: 10 beams, Nd glass laser, 30 -40 k. J at 350 nm. Target chamber ( 4. 6 m Al, 13 cm thick) Laser bay (each frame contains 5 laser chains, 137 m long) HSB, SU 2001, 17. 9. 01 23

Drivers II (Particle Beams) PARTICLE BEAMS: particle energy limited by absorption: light ions: 10 Me. V, requiring ~ 10 MA, heavy ions: 10 Ge. V ~ 10 k. A (Pb) A) Light ions: high currents produced in Pulsed Power Diodes, PBFAII, Sandia: 2 MJ in 20 ns, high efficiency, problems with - arcing - focussing and beam transport - energy spread, - repetition rate. B) Heavy ions: Induction Linacs or RF Linacs with storage rings - high efficiency (25% has been achieved) - high repetition rate (3 Hz, GSI Darmstadt) - good beam transport - and focussing (using plasma lenses) GSI Darmstadt: 500 J in 50 ns, I 20+ 300 Me. V/amu. HSB, SU 2001, 17. 9. 01 24

Heavy Ion Driven Fusion, Reactor Study HIBALL From: R. Bock Phys. Bl. 37 (1981) 214 HSB, SU 2001, 17. 9. 01 25

Drivers III (X-Rays from Z-Pinches) Generally, Z-Pinches are unstable (sausage-instability): However, - they generate strong X-Rays during the collapse, - mult-wire arrays are more stable, generate even more X-Rays! HSB, SU 2001, 17. 9. 01 26

National Ignition Facility, NIF Lindl et al. , Phys. Plasmas, 1995 Being built at Livermore, - 192 lasers (in two stages), - frequency-tripled Nd glass laser at 350 nm, - with an output of 500 TW, - and 1. 8 MJ energy on the target, for defense applications and inertial fusion ignition (explore ignition with both indirect-drive and direct-drive targets). HSB, SU 2001, 17. 9. 01 27

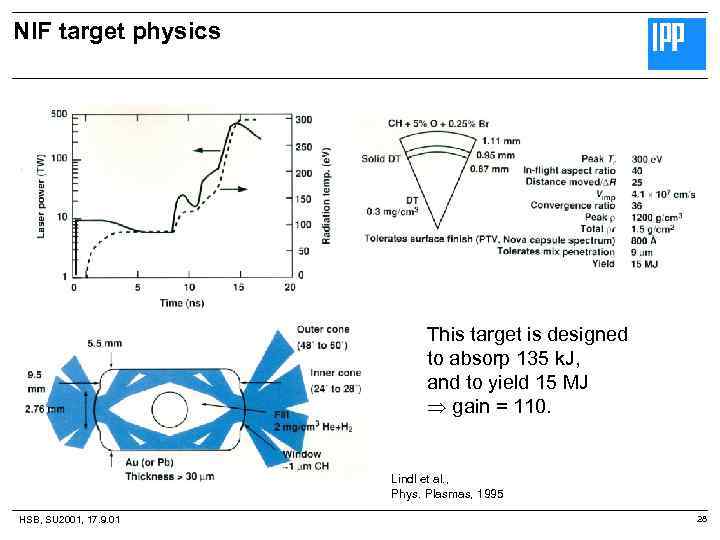NIF target physics This target is designed to absorp 135 k. J, and to yield 15 MJ gain = 110. Lindl et al. , Phys. Plasmas, 1995 HSB, SU 2001, 17. 9. 01 28

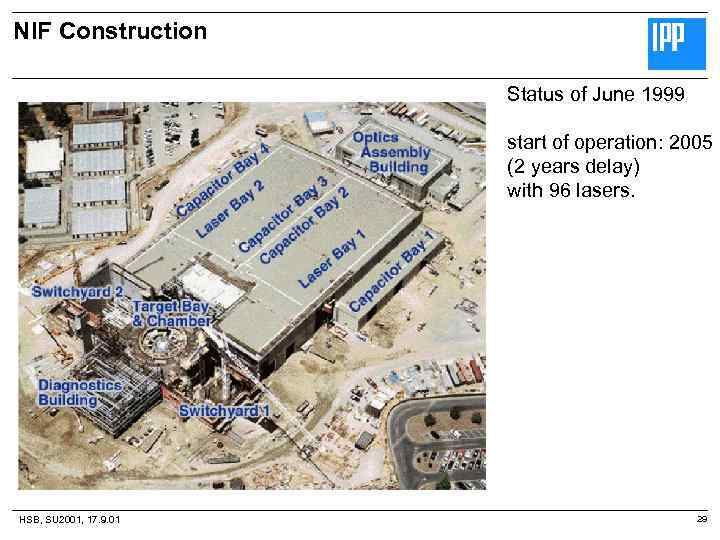NIF Construction Status of June 1999 start of operation: 2005 (2 years delay) with 96 lasers. HSB, SU 2001, 17. 9. 01 29

NIF Construction II, target chamber Installation of the target chamber HSB, SU 2001, 17. 9. 01 30

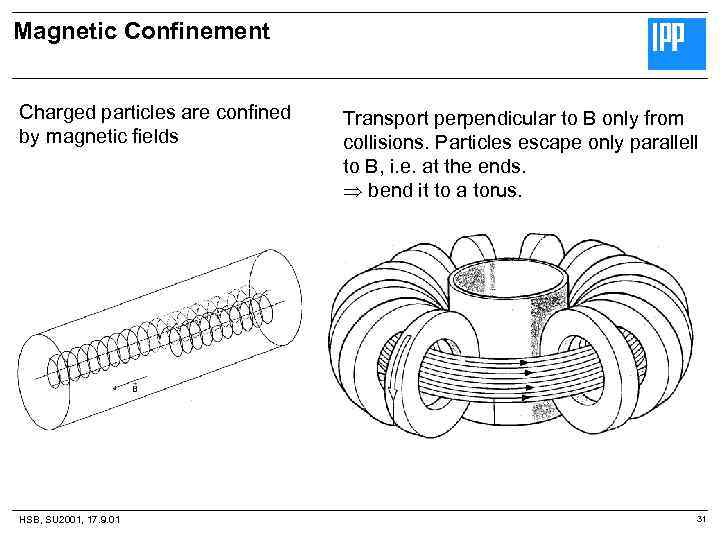Magnetic Confinement Charged particles are confined by magnetic fields HSB, SU 2001, 17. 9. 01 Transport perpendicular to B only from collisions. Particles escape only parallell to B, i. e. at the ends. bend it to a torus. 31

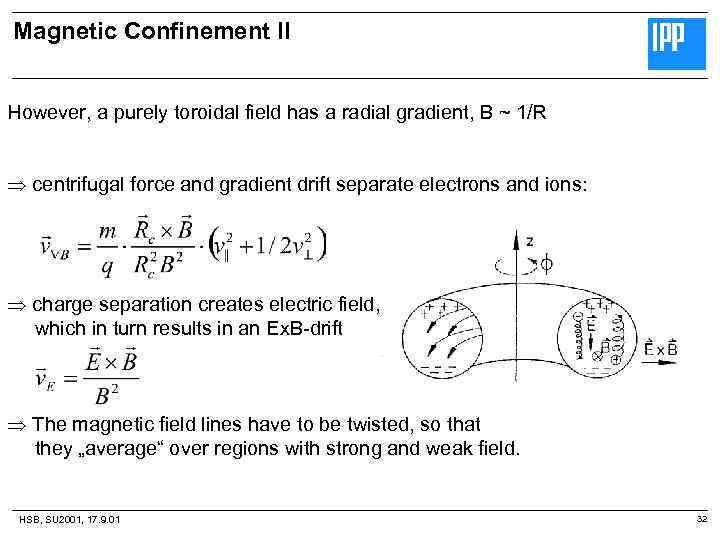Magnetic Confinement II However, a purely toroidal field has a radial gradient, B ~ 1/R centrifugal force and gradient drift separate electrons and ions: charge separation creates electric field, which in turn results in an Ex. B-drift The magnetic field lines have to be twisted, so that they „average“ over regions with strong and weak field. HSB, SU 2001, 17. 9. 01 32

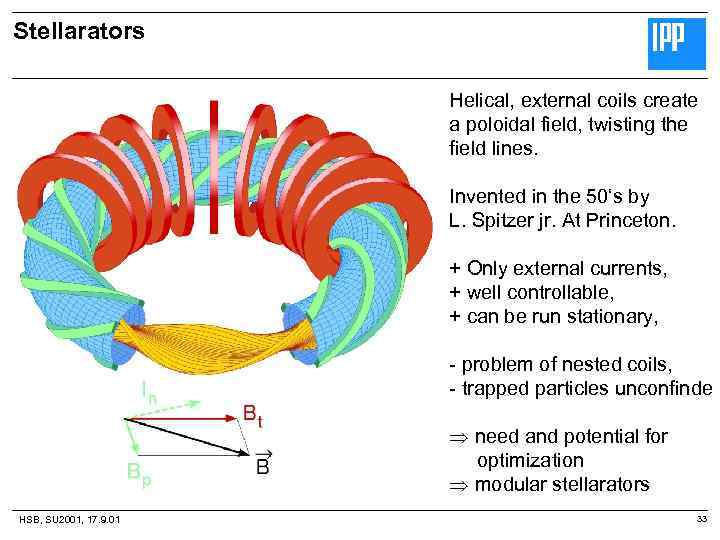Stellarators Helical, external coils create a poloidal field, twisting the field lines. Invented in the 50‘s by L. Spitzer jr. At Princeton. + Only external currents, + well controllable, + can be run stationary, - problem of nested coils, - trapped particles unconfinde need and potential for optimization modular stellarators HSB, SU 2001, 17. 9. 01 33

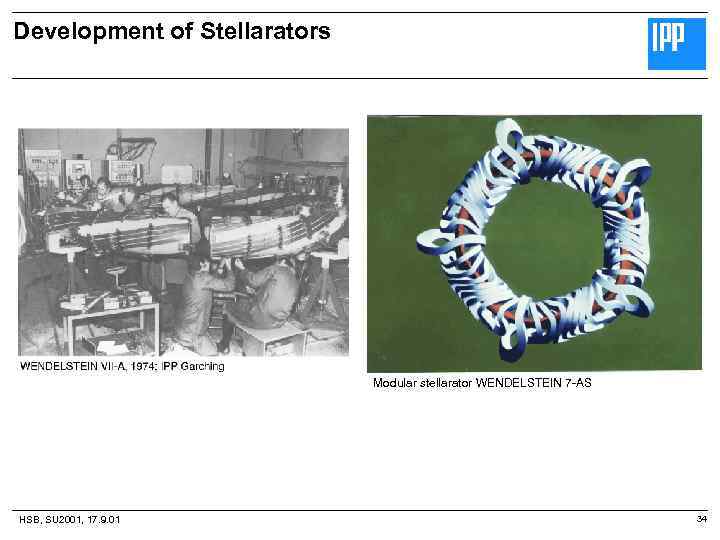Development of Stellarators Modular stellarator WENDELSTEIN 7 -AS HSB, SU 2001, 17. 9. 01 34

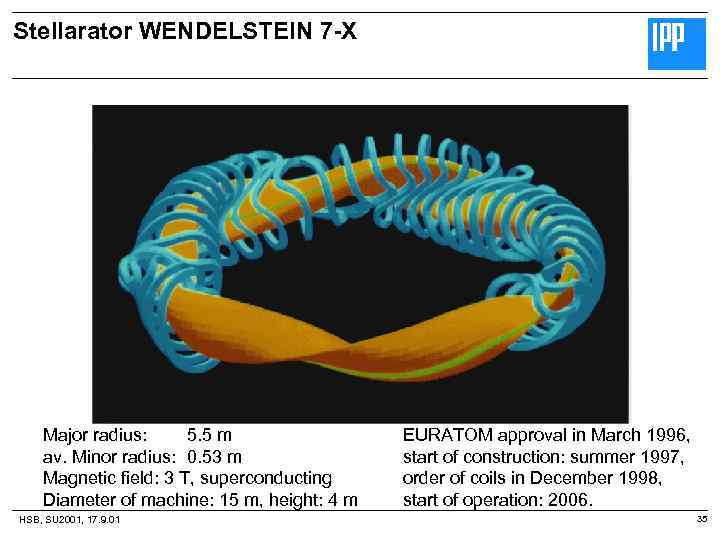Stellarator WENDELSTEIN 7 -X Major radius: 5. 5 m av. Minor radius: 0. 53 m Magnetic field: 3 T, superconducting Diameter of machine: 15 m, height: 4 m HSB, SU 2001, 17. 9. 01 EURATOM approval in March 1996, start of construction: summer 1997, order of coils in December 1998, start of operation: 2006. 35

IPP branch in Greifswald HSB, SU 2001, 17. 9. 01 36

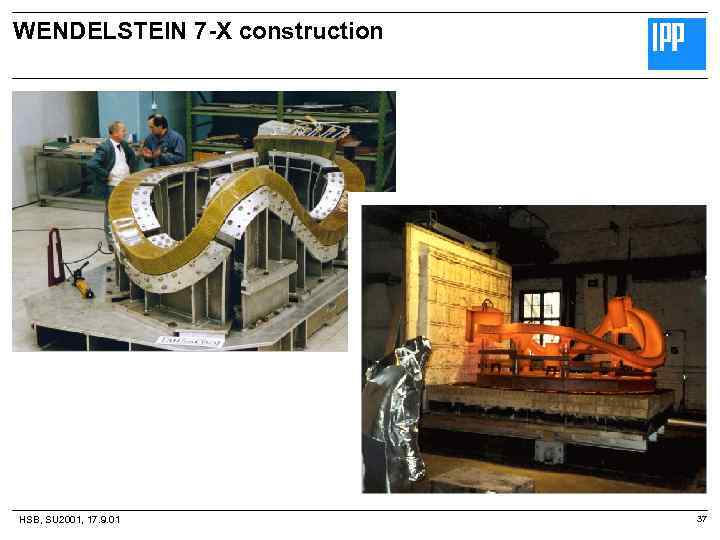WENDELSTEIN 7 -X construction HSB, SU 2001, 17. 9. 01 37

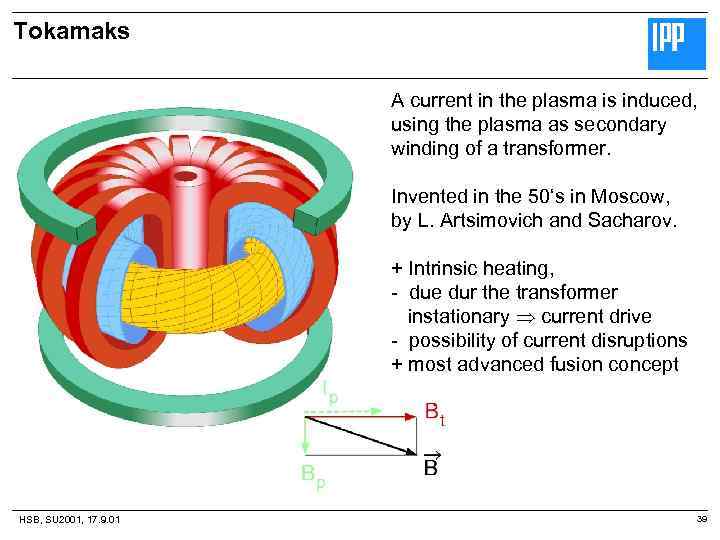Tokamaks A current in the plasma is induced, using the plasma as secondary winding of a transformer. Invented in the 50‘s in Moscow, by L. Artsimovich and Sacharov. + Intrinsic heating, - due dur the transformer instationary current drive - possibility of current disruptions + most advanced fusion concept HSB, SU 2001, 17. 9. 01 39

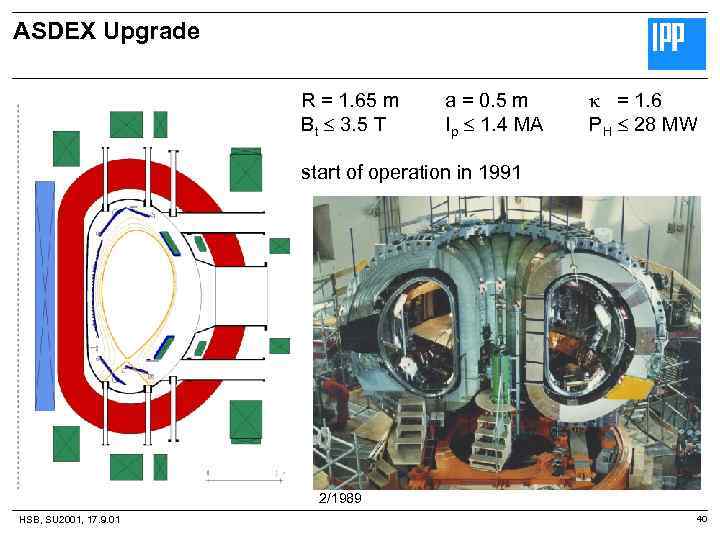ASDEX Upgrade R = 1. 65 m Bt 3. 5 T a = 0. 5 m Ip 1. 4 MA = 1. 6 PH 28 MW start of operation in 1991 2/1989 HSB, SU 2001, 17. 9. 01 40

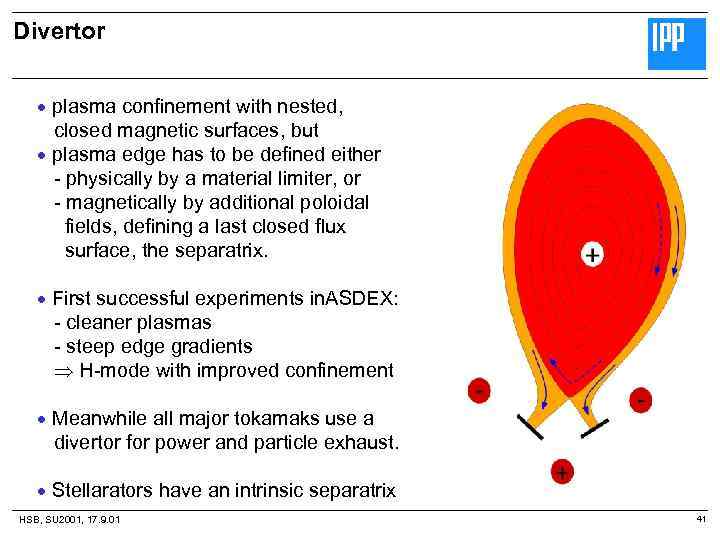Divertor plasma confinement with nested, closed magnetic surfaces, but plasma edge has to be defined either - physically by a material limiter, or - magnetically by additional poloidal fields, defining a last closed flux surface, the separatrix. First successful experiments in. ASDEX: - cleaner plasmas - steep edge gradients H-mode with improved confinement Meanwhile all major tokamaks use a divertor for power and particle exhaust. Stellarators have an intrinsic separatrix HSB, SU 2001, 17. 9. 01 41

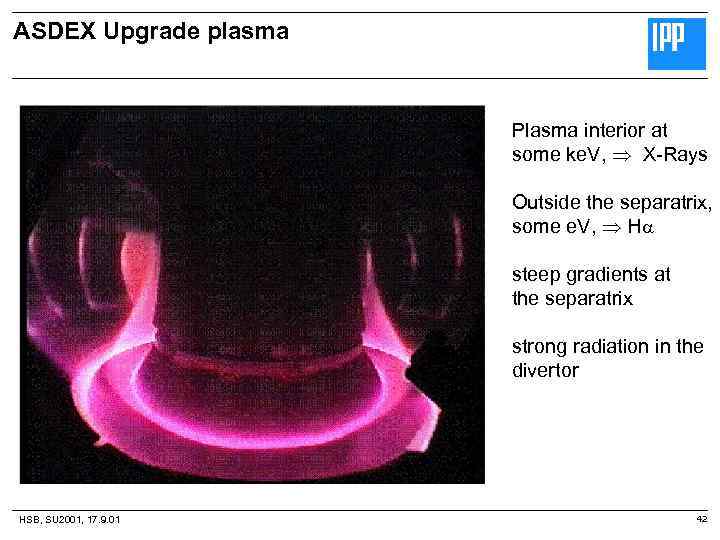ASDEX Upgrade plasma Plasma interior at some ke. V, X-Rays Outside the separatrix, some e. V, H steep gradients at the separatrix strong radiation in the divertor HSB, SU 2001, 17. 9. 01 42

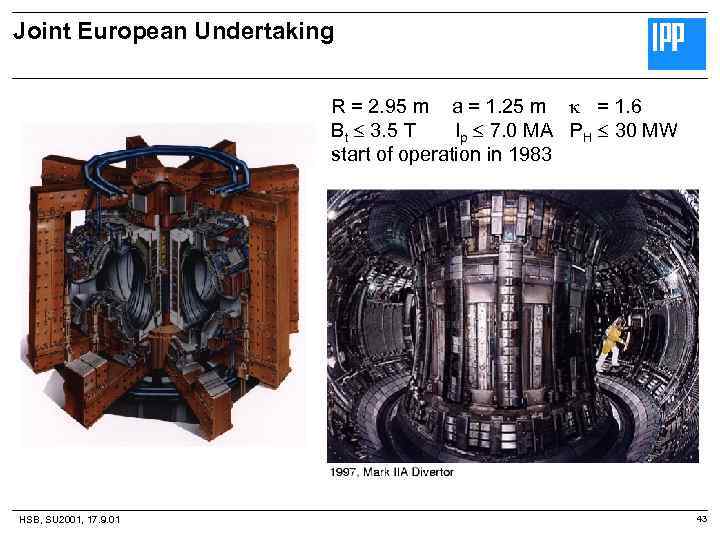Joint European Undertaking R = 2. 95 m a = 1. 25 m = 1. 6 Bt 3. 5 T Ip 7. 0 MA PH 30 MW start of operation in 1983 HSB, SU 2001, 17. 9. 01 43

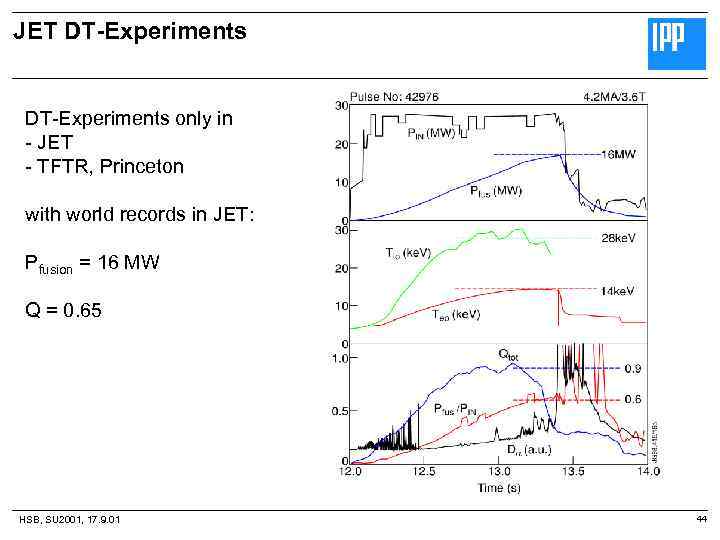JET DT-Experiments only in - JET - TFTR, Princeton with world records in JET: Pfusion = 16 MW Q = 0. 65 HSB, SU 2001, 17. 9. 01 44

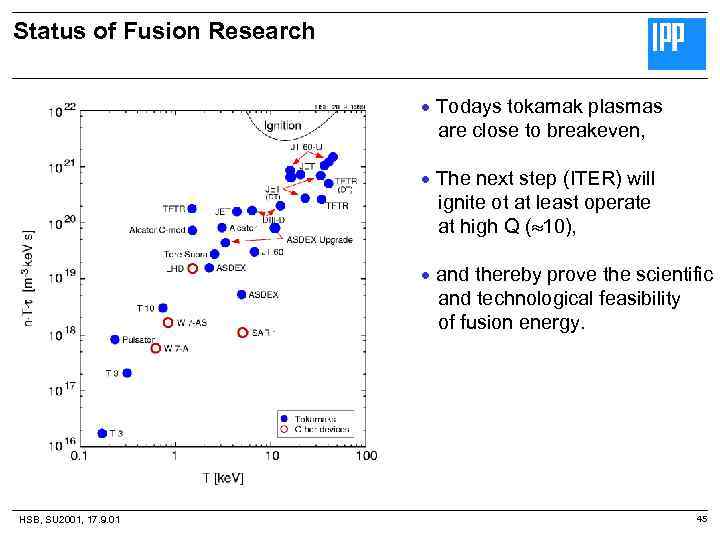Status of Fusion Research Todays tokamak plasmas are close to breakeven, The next step (ITER) will ignite ot at least operate at high Q ( 10), and thereby prove the scientific and technological feasibility of fusion energy. HSB, SU 2001, 17. 9. 01 45

International Thermonuclear Experimental Reactor International project: Europe, Japan, Russia, and the USA (before 1998). Outline Design in 1999, Final Report due July 2001. 12 m HSB, SU 2001, 17. 9. 01 R [m] a [m] k d Ip [MA] B [T] Tpuls [s] Pfusion [MW] 6. 2 2. 0 1. 7 0. 35 15. 1 5. 3 400 46

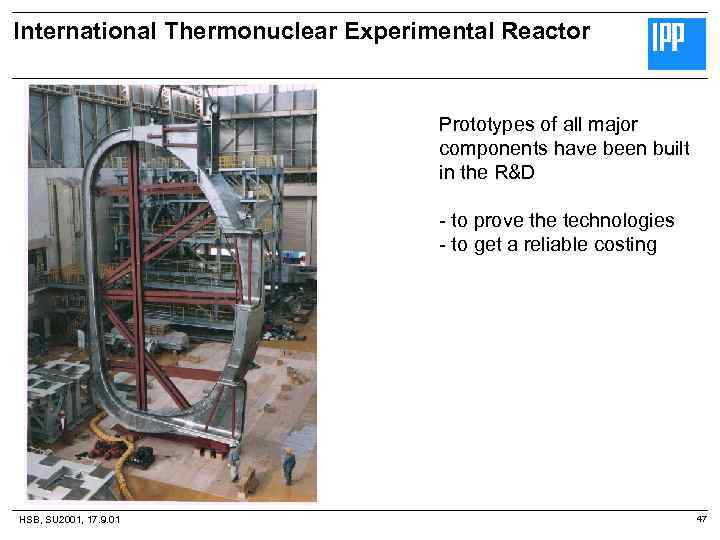International Thermonuclear Experimental Reactor Prototypes of all major components have been built in the R&D - to prove the technologies - to get a reliable costing HSB, SU 2001, 17. 9. 01 47

International Thermonuclear Experimental Reactor First site proposal by ITER CANADA HSB, SU 2001, 17. 9. 01 48

HSB, SU 2001, 17. 9. 01