Magnetic and charge order phase transition in YBa

Magnetic and charge order phase transition in YBa. Fe 2 O 5 (Verwey transition) Peter Blaha, Ch. Spiel, K. Schwarz Institute of Materials Chemistry TU Wien Thanks to P. Karen (Univ. Oslo, Norway)

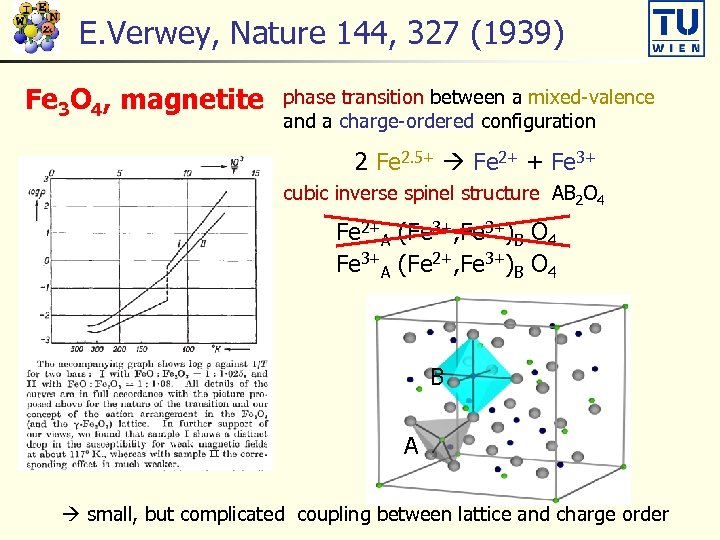
E. Verwey, Nature 144, 327 (1939) Fe 3 O 4, magnetite phase transition between a mixed-valence and a charge-ordered configuration 2 Fe 2. 5+ Fe 2+ + Fe 3+ cubic inverse spinel structure AB 2 O 4 Fe 2+A (Fe 3+, Fe 3+)B O 4 Fe 3+A (Fe 2+, Fe 3+)B O 4 B A small, but complicated coupling between lattice and charge order

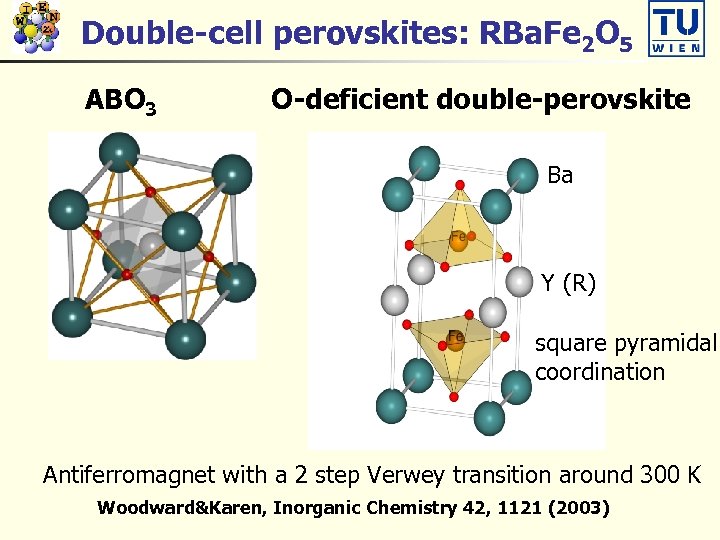
Double-cell perovskites: RBa. Fe 2 O 5 ABO 3 O-deficient double-perovskite Ba Y (R) square pyramidal coordination Antiferromagnet with a 2 step Verwey transition around 300 K Woodward&Karen, Inorganic Chemistry 42, 1121 (2003)

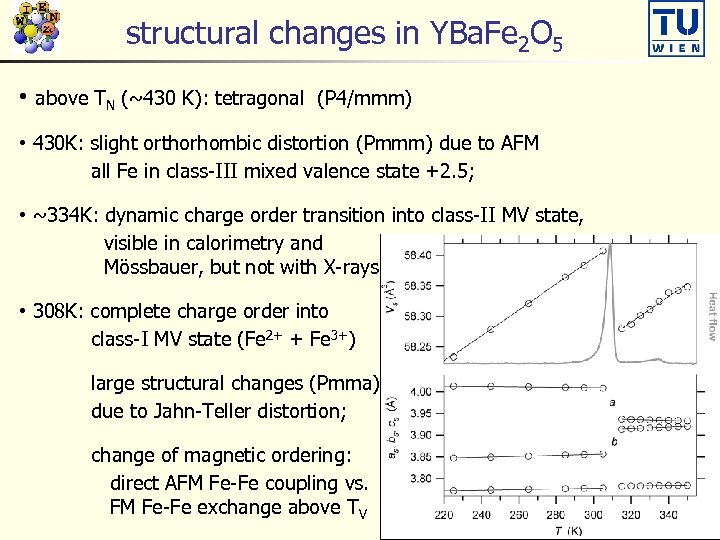
structural changes in YBa. Fe 2 O 5 • above TN (~430 K): tetragonal (P 4/mmm) • 430 K: slight orthorhombic distortion (Pmmm) due to AFM all Fe in class-III mixed valence state +2. 5; • ~334 K: dynamic charge order transition into class-II MV state, visible in calorimetry and Mössbauer, but not with X-rays • 308 K: complete charge order into class-I MV state (Fe 2+ + Fe 3+) large structural changes (Pmma) due to Jahn-Teller distortion; change of magnetic ordering: direct AFM Fe-Fe coupling vs. FM Fe-Fe exchange above TV

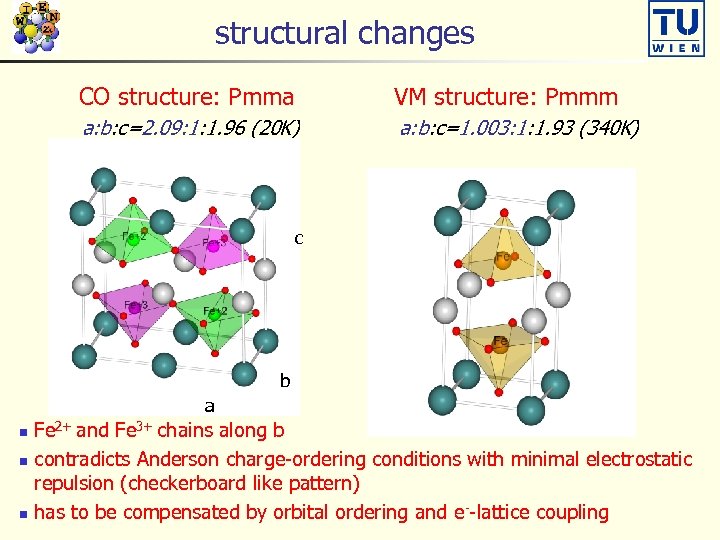
structural changes CO structure: Pmma VM structure: Pmmm a: b: c=2. 09: 1: 1. 96 (20 K) a: b: c=1. 003: 1: 1. 93 (340 K) c b a Fe 2+ and Fe 3+ chains along b n contradicts Anderson charge-ordering conditions with minimal electrostatic repulsion (checkerboard like pattern) n has to be compensated by orbital ordering and e --lattice coupling n

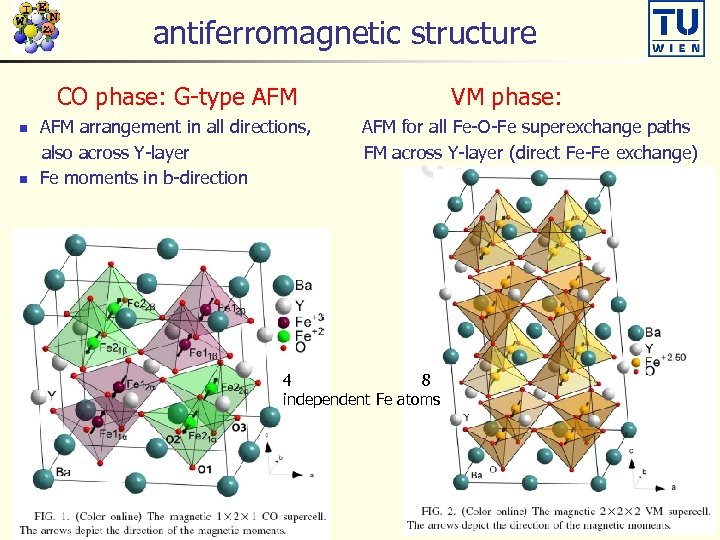
antiferromagnetic structure CO phase: G-type AFM n n AFM arrangement in all directions, also across Y-layer Fe moments in b-direction VM phase: AFM for all Fe-O-Fe superexchange paths FM across Y-layer (direct Fe-Fe exchange) 4 8 independent Fe atoms

Theoretical methods: n WIEN 2 k (APW+lo) calculations Rkmax=7, 100 k-points n spin-polarized, various spin-structures n + spin-orbit coupling n based on density functional theory: n n LSDA or GGA (PBE) n Exc ≡ Exc(ρ, ∇ρ) WIEN 2 K An Augmented Plane Wave Plus Local Orbital Program for Calculating Crystal Properties Peter Blaha Karlheinz Schwarz Georg Madsen Dieter Kvasnicka Joachim Luitz http: //www. wien 2 k. at description of “highly correlated electrons” using “non-local” (orbital dep. ) functionals n n n LDA+U, GGA+U hybrid-DFT (only for correlated electrons) n mixing exact exchange (HF) + GGA 1400 registered groups 2000 mailinglist users

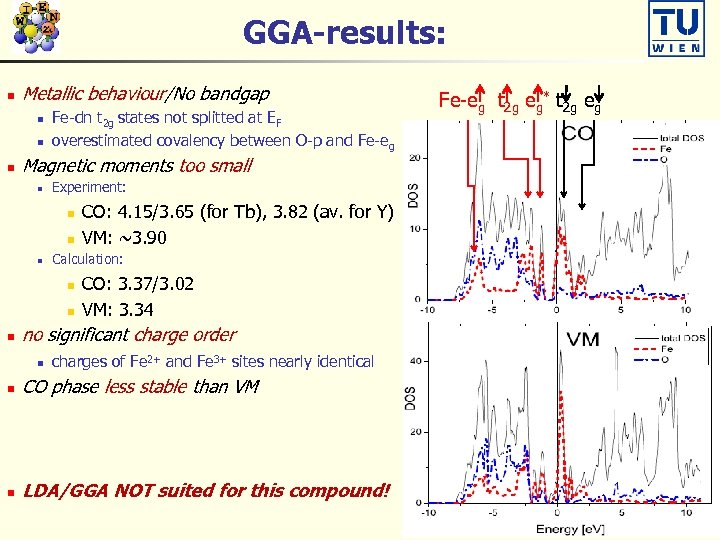
GGA-results: n Metallic behaviour/No bandgap n n n Fe-dn t 2 g states not splitted at EF overestimated covalency between O-p and Fe-eg Magnetic moments too small n Experiment: n n n Calculation: n n n CO: 4. 15/3. 65 (for Tb), 3. 82 (av. for Y) VM: ~3. 90 CO: 3. 37/3. 02 VM: 3. 34 no significant charge order n charges of Fe 2+ and Fe 3+ sites nearly identical n CO phase less stable than VM n LDA/GGA NOT suited for this compound! Fe-eg t 2 g eg* t 2 g eg

“Localized electrons”: GGA+U n Hybrid-DFT n n Exc. PBE 0 [r] = Exc. PBE [r] + a (Ex. HF[Fsel] – Ex. PBE[rsel]) LDA+U, GGA+U n ELDA+U(r, n) = ELDA(r) + Eorb(n) – EDCC(r) n n n separate electrons into “itinerant” (LDA) and localized e- (TM-3 d, RE 4 f e-) treat them with “approximate screened Hartree-Fock” correct for “double counting” n Hubbard-U describes coulomb energy for 2 e- at the same site n orbital dependent potential

Determination of U Take Ueff as “empirical” parameter (fit to experiment) n Estimate Ueff from constraint LDA calculations n constrain the occupation of certain states (add/subtract e -) n switch off any hybridization of these states (“core”-states) n calculate the resulting Etot n n we used Ueff=7 e. V for all calculations

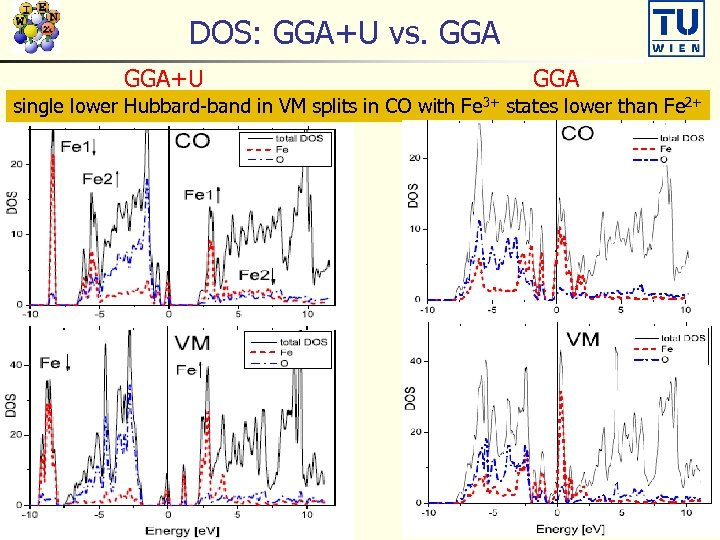
DOS: GGA+U vs. GGA+U GGA singleinsulator, t 2 g band splits VM splits in CO with Fe 3+ states lower than Fe 2+ lower Hubbard-band in metallic

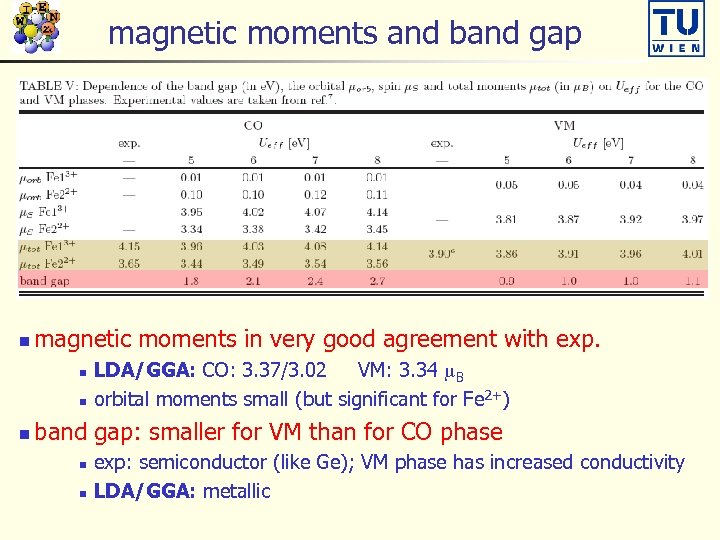
magnetic moments and band gap n magnetic moments in very good agreement with exp. n n n LDA/GGA: CO: 3. 37/3. 02 VM: 3. 34 m. B orbital moments small (but significant for Fe 2+) band gap: smaller for VM than for CO phase n n exp: semiconductor (like Ge); VM phase has increased conductivity LDA/GGA: metallic

Charge transfer n Charges according to Baders “Atoms in Molecules” theory Define an “atom” as region within a zero flux surface n Integrate charge inside this region n

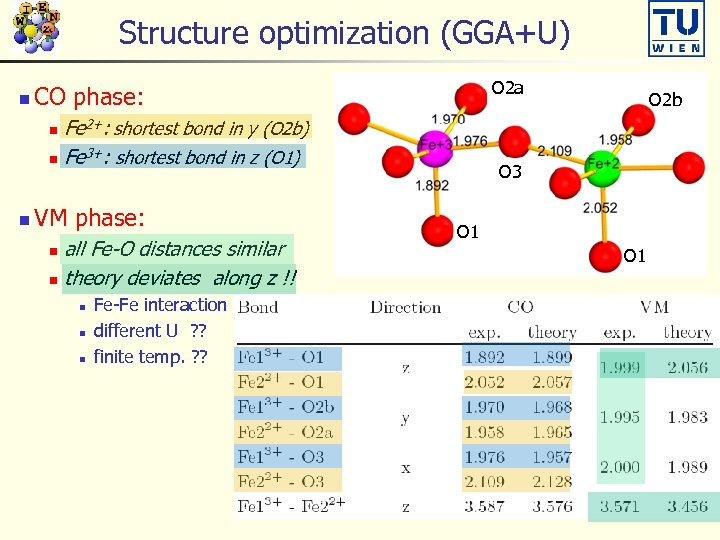
Structure optimization (GGA+U) n O 2 a CO phase: Fe 2+: shortest bond in y (O 2 b) 3+ n Fe : shortest bond in z (O 1) O 2 b n n VM phase: all Fe-O distances similar n theory deviates along z !! n n Fe-Fe interaction different U ? ? finite temp. ? ? O 3 O 1

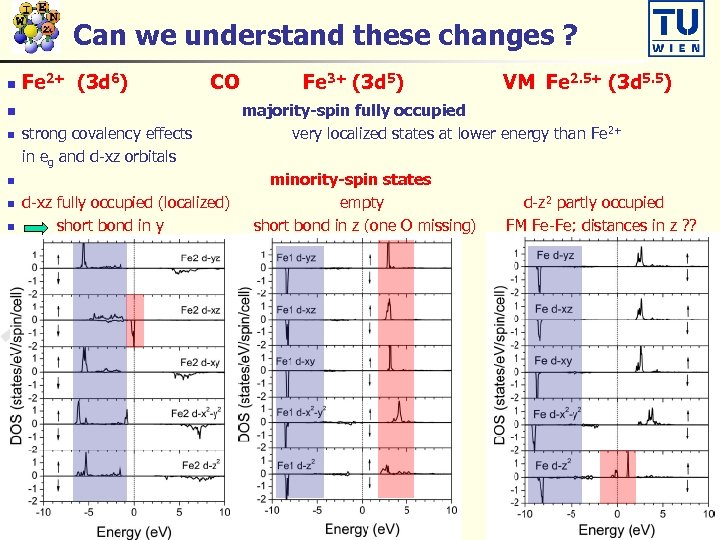
Can we understand these changes ? n Fe 2+ (3 d 6) CO n n strong covalency effects in eg and d-xz orbitals n n n d-xz fully occupied (localized) short bond in y Fe 3+ (3 d 5) VM Fe 2. 5+ (3 d 5. 5) majority-spin fully occupied very localized states at lower energy than Fe 2+ minority-spin states empty short bond in z (one O missing) d-z 2 partly occupied FM Fe-Fe; distances in z ? ?

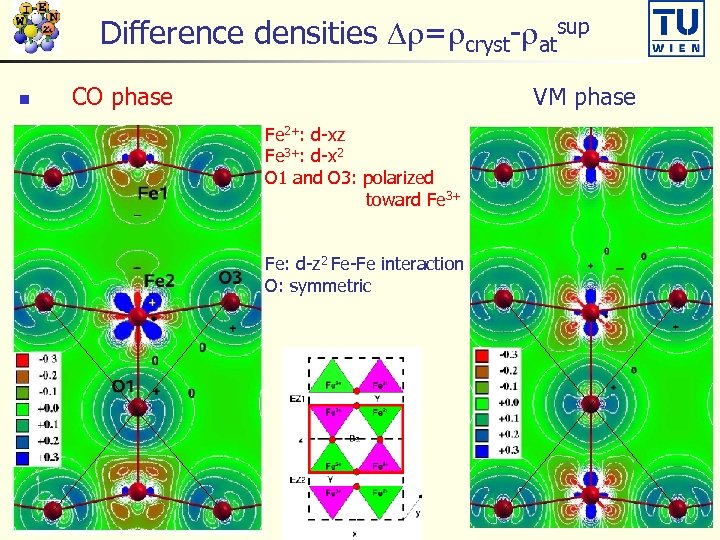
Difference densities Dr=rcryst-ratsup n CO phase VM phase Fe 2+: d-xz Fe 3+: d-x 2 O 1 and O 3: polarized toward Fe 3+ Fe: d-z 2 Fe-Fe interaction O: symmetric

dxz spin density (rup-rdn) of CO phase n n Fe 3+: no contribution Fe 2+: dxz weak p-bond with O tilting of O 3 p-orbital

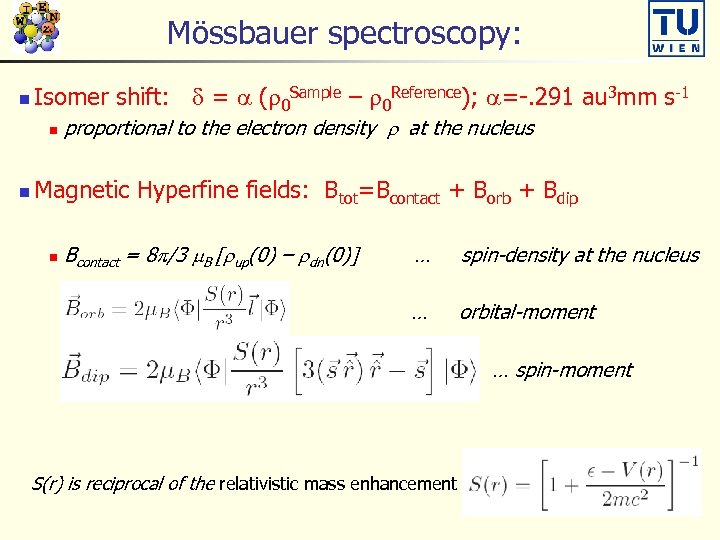
Mössbauer spectroscopy: n Isomer shift: d = a (r 0 Sample – r 0 Reference); a=-. 291 au 3 mm s-1 n n proportional to the electron density r at the nucleus Magnetic Hyperfine fields: Btot=Bcontact + Borb + Bdip Bcontact = 8 p/3 m. B [rup(0) – rdn(0)] … spin-density at the nucleus … n orbital-moment … spin-moment S(r) is reciprocal of the relativistic mass enhancement

Electric field gradients (EFG) Nuclei with a nuclear quantum number I≥ 1 have an electrical quadrupole moment Q n Nuclear quadrupole interaction (NQI) between “non-spherical” nuclear charge Q times the electric field gradient F n Experiments EFG traceless tensor n NMR n NQR n Mössbauer n PAC n with traceless |Vzz| |Vyy| EFG Vzz |Vxx| principal component asymmetry parameter

theoretical EFG calculations: EFG is tensor of second derivatives of VC at the nucleus: Cartesian LM-repr. EFG is proportial to differences of orbital occupations

Hyperfine fields: Fe 2+ has large Borb and Bdip Mössbauer anisotropy in LDA/GGA EFG: Fe 2+ has too small spectroscopy Isomer shift: charge transfer too small in LDA/GGA CO VM

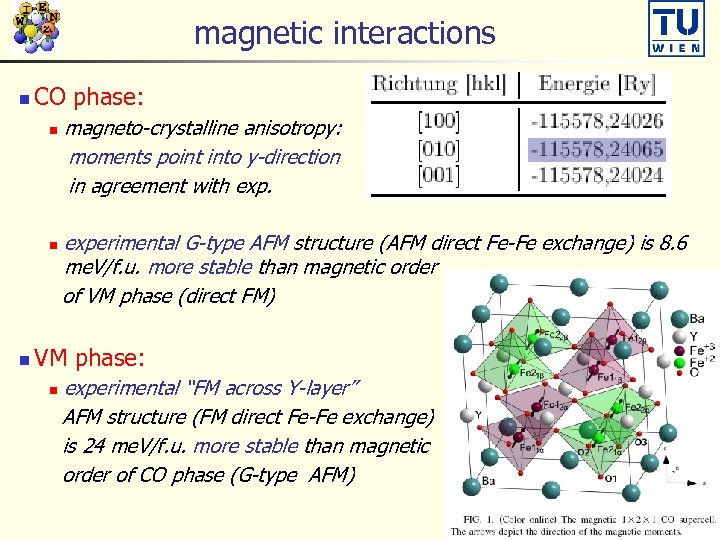
magnetic interactions n CO phase: n n n magneto-crystalline anisotropy: moments point into y-direction in agreement with experimental G-type AFM structure (AFM direct Fe-Fe exchange) is 8. 6 me. V/f. u. more stable than magnetic order of VM phase (direct FM) VM phase: n experimental “FM across Y-layer” AFM structure (FM direct Fe-Fe exchange) is 24 me. V/f. u. more stable than magnetic order of CO phase (G-type AFM)

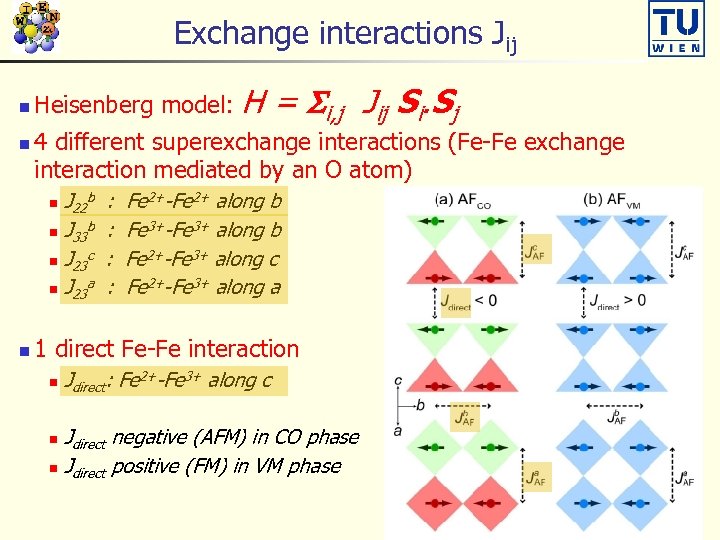
Exchange interactions Jij n n Heisenberg model: 4 different superexchange interactions (Fe-Fe exchange interaction mediated by an O atom) J 22 b b n J 33 c n J 23 a n J 23 n n H = Si, j Jij Si. Sj : : Fe 2+-Fe 2+ along b Fe 3+-Fe 3+ along b Fe 2+-Fe 3+ along c Fe 2+-Fe 3+ along a 1 direct Fe-Fe interaction n Jdirect: Fe 2+-Fe 3+ along c Jdirect negative (AFM) in CO phase n Jdirect positive (FM) in VM phase n

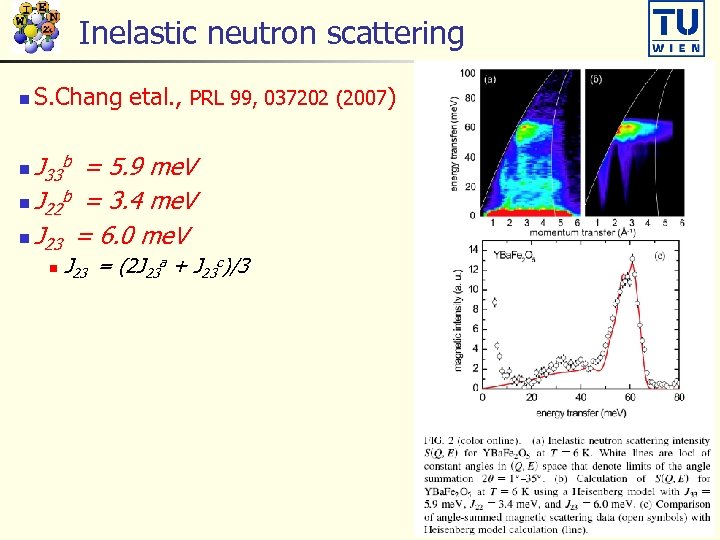
Inelastic neutron scattering n S. Chang etal. , PRL 99, 037202 (2007) J = 5. 9 me. V n J 22 b = 3. 4 me. V n J 23 = 6. 0 me. V n 33 b n J 23 = (2 J 23 a + J 23 c)/3

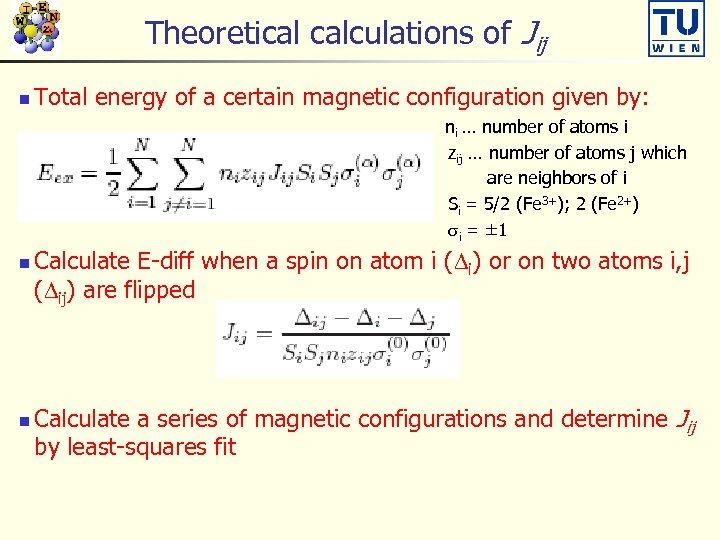
Theoretical calculations of Jij n Total energy of a certain magnetic configuration given by: ni … number of atoms i zij … number of atoms j which are neighbors of i Si = 5/2 (Fe 3+); 2 (Fe 2+) si = ± 1 n n Calculate E-diff when a spin on atom i (Di) or on two atoms i, j (Dij) are flipped Calculate a series of magnetic configurations and determine Jij by least-squares fit

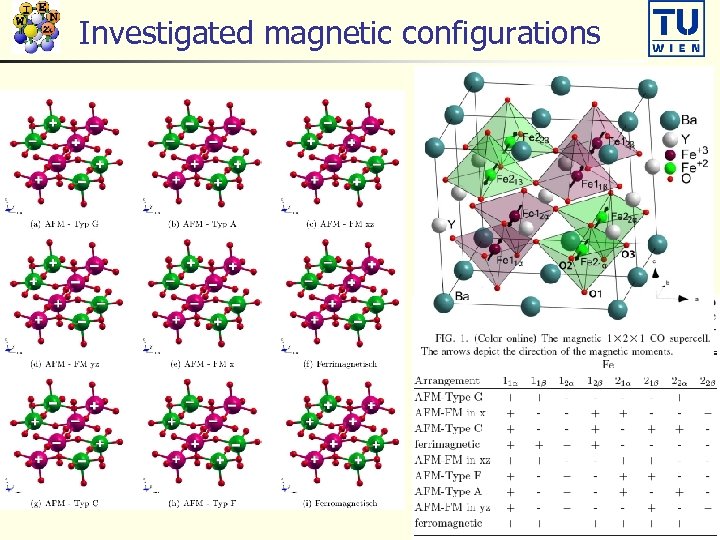
Investigated magnetic configurations

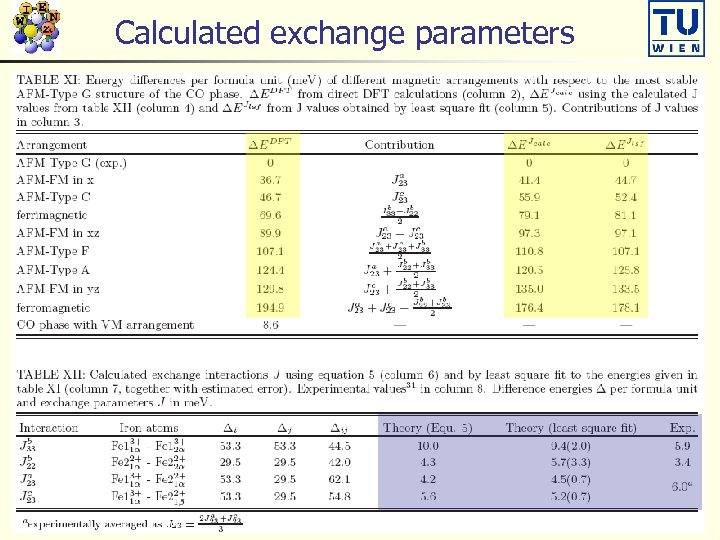
Calculated exchange parameters

Summary n Standard LDA/GGA methods cannot explain YBa. Fe 2 O 5 n metallic, no charge order (Fe 2+-Fe 3+), too small moments Needs proper description of the Fe 3 d electrons (GGA+U, …) n CO-phase: Fe 2+: high-spin d 6, occupation of a single spin-dn orbital ( dxz) n n n Fe 2+/Fe 3+ ordered in chains along b, cooperative Jahn-Teller distortion and strong e--lattice coupling which dominates simple Coulomb arguments (checkerboard structure of Fe 2+/Fe 3+) VM phase: small orthorhombic distortion (AFM order, moments along b) n Fe d-z 2 spin-dn orbital partly occupied (top of the valence bands) leads to direct Fe-Fe exchange across Y-layer and thus to ferromagnetic order (AFM in CO phase). Quantitative interpretation of the Mössbauer data n Calculated exchange parameters Jij in reasonable agreement with exp. n

Thank you for your attention !

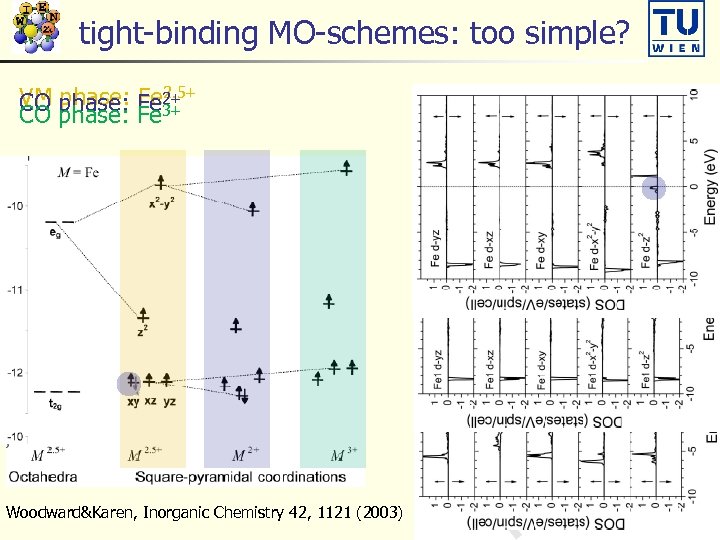
tight-binding MO-schemes: too simple? VM phase: Fe 2+ phase: Fe 2. 5+ CO phase: Fe 3+ CO Woodward&Karen, Inorganic Chemistry 42, 1121 (2003)

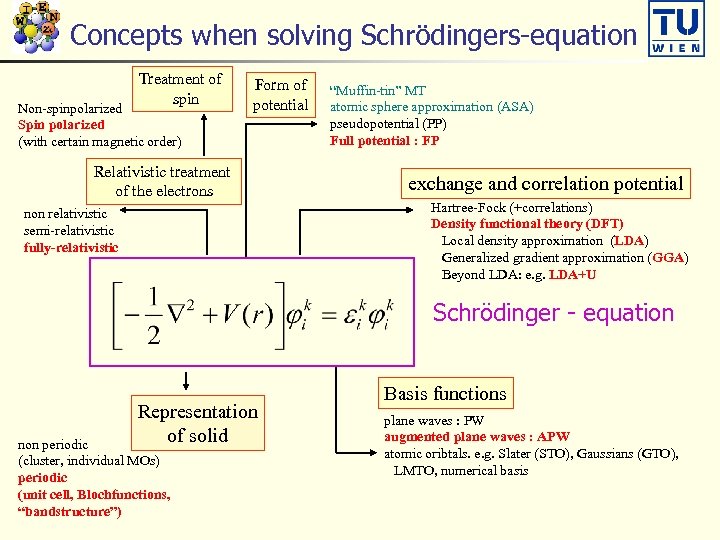
Concepts when solving Schrödingers-equation Treatment of spin Non-spinpolarized Spin polarized (with certain magnetic order) Form of potential Relativistic treatment of the electrons non relativistic semi-relativistic fully-relativistic “Muffin-tin” MT atomic sphere approximation (ASA) pseudopotential (PP) Full potential : FP exchange and correlation potential Hartree-Fock (+correlations) Density functional theory (DFT) Local density approximation (LDA) Generalized gradient approximation (GGA) Beyond LDA: e. g. LDA+U Schrödinger - equation Representation of solid non periodic (cluster, individual MOs) periodic (unit cell, Blochfunctions, “bandstructure”) Basis functions plane waves : PW augmented plane waves : APW atomic oribtals. e. g. Slater (STO), Gaussians (GTO), LMTO, numerical basis

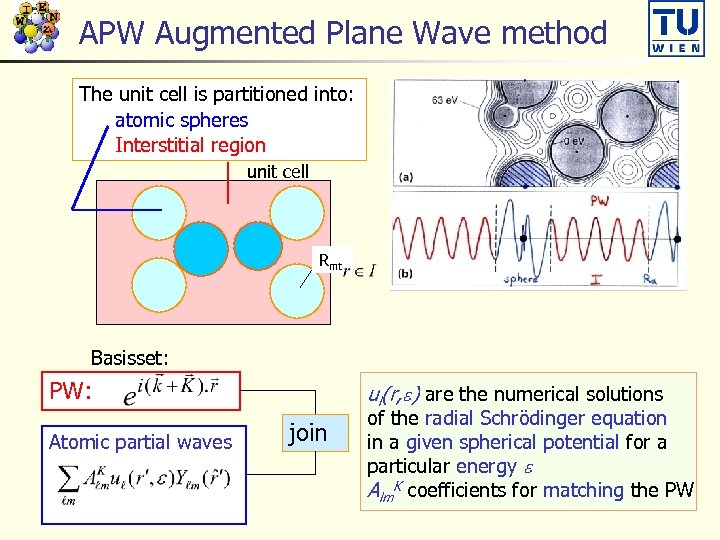
APW Augmented Plane Wave method The unit cell is partitioned into: atomic spheres Interstitial region unit cell Rmt Basisset: PW: Atomic partial waves ul(r, e) are the numerical solutions join of the radial Schrödinger equation in a given spherical potential for a particular energy e Alm. K coefficients for matching the PW

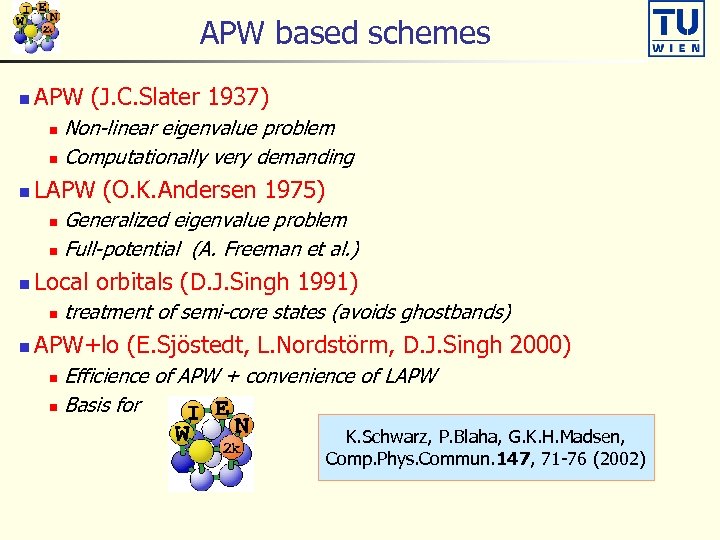
APW based schemes n APW (J. C. Slater 1937) Non-linear eigenvalue problem n Computationally very demanding n n LAPW (O. K. Andersen 1975) Generalized eigenvalue problem n Full-potential (A. Freeman et al. ) n n Local orbitals (D. J. Singh 1991) n n treatment of semi-core states (avoids ghostbands) APW+lo (E. Sjöstedt, L. Nordstörm, D. J. Singh 2000) Efficience of APW + convenience of LAPW n Basis for n K. Schwarz, P. Blaha, G. K. H. Madsen, Comp. Phys. Commun. 147, 71 -76 (2002)

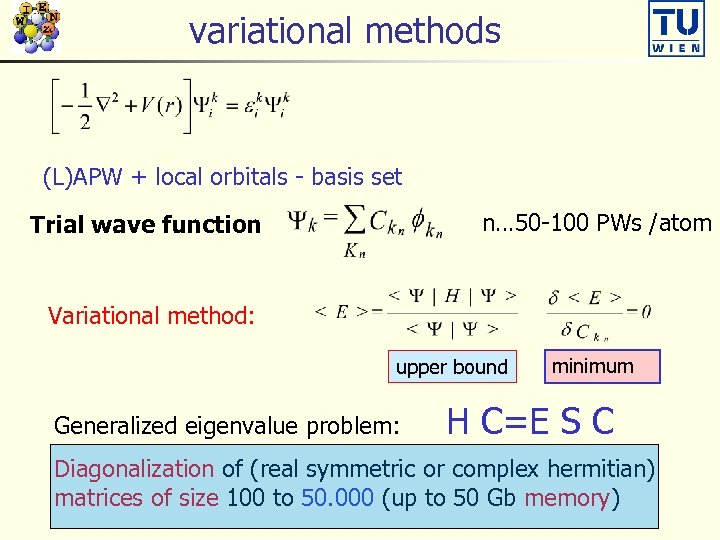
variational methods (L)APW + local orbitals - basis set n… 50 -100 PWs /atom Trial wave function Variational method: upper bound Generalized eigenvalue problem: minimum H C=E S C Diagonalization of (real symmetric or complex hermitian) matrices of size 100 to 50. 000 (up to 50 Gb memory)

Quantum mechanics at work

WIEN 2 k software package An Augmented Plane Wave Plus Local Orbital Program for Calculating Crystal Properties Peter Blaha Karlheinz Schwarz Georg Madsen Dieter Kvasnicka Joachim Luitz WIEN 97: ~500 users WIEN 2 k: ~1150 users mailinglist: 1800 users November 2001 Vienna, AUSTRIA Vienna University of Technology http: //www. wien 2 k. at

Development of WIEN 2 k n Authors of WIEN 2 k P. Blaha, K. Schwarz, D. Kvasnicka, G. Madsen and J. Luitz Other contributions to WIEN 2 k n C. Ambrosch-Draxl (Univ. Graz, Austria), optics n T. Charpin (Paris), elastic constants n R. Laskowski (Vienna), non-collinear magnetism, parallelization n L. Marks (Northwestern, US) , various optimizations, new mixer n P. Novák and J. Kunes (Prague), LDA+U, SO n B. Olejnik (Vienna), non-linear optics, n C. Persson (Uppsala), irreducible representations n M. Scheffler (Fritz Haber Inst. , Berlin), forces n D. J. Singh (NRL, Washington D. C. ), local oribtals (LO), APW+lo n E. Sjöstedt and L Nordström (Uppsala, Sweden), APW+lo n J. Sofo and J. Fuhr (Barriloche), Bader analysis n B. Yanchitsky and A. Timoshevskii (Kiev), spacegroup n and many others …. n

International co-operations n More than 1000 user groups worldwide Industries (Canon, Eastman, Exxon, Fuji, A. D. Little, Mitsubishi, Motorola, NEC, Norsk Hydro, Osram, Panasonic, Samsung, Sony). n Europe: (EHT Zürich, MPI Stuttgart, Dresden, FHI Berlin, DESY, TH Aachen, ESRF, Prague, Paris, Chalmers, Cambridge, Oxford) n America: ARG, BZ, CDN, MX, USA (MIT, NIST, Berkeley, Princeton, Harvard, Argonne NL, Los Alamos Nat. Lab. , Penn State, Georgia Tech, Lehigh, Chicago, SUNY, UC St. Barbara, Toronto) n far east: AUS, China, India, JPN, Korea, Pakistan, Singapore, Taiwan (Beijing, Tokyo, Osaka, Sendai, Tsukuba, Hong Kong) n n Registration at www. wien 2 k. at 400/4000 Euro for Universites/Industries n code download via www (with password), updates, bug fixes, news n usersguide, faq-page, mailing-list with help-requests n

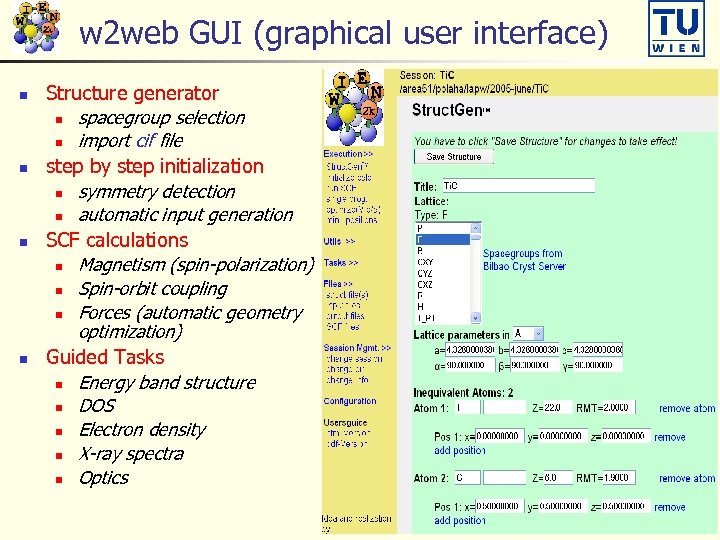
w 2 web GUI (graphical user interface) n n Structure generator n spacegroup selection n import cif file step by step initialization n symmetry detection n automatic input generation SCF calculations n Magnetism (spin-polarization) n Spin-orbit coupling n Forces (automatic geometry optimization) Guided Tasks n Energy band structure n DOS n Electron density n X-ray spectra n Optics

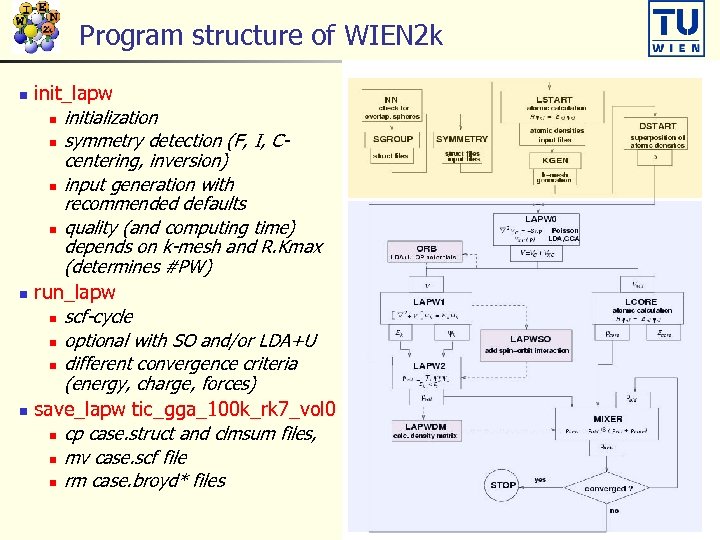
Program structure of WIEN 2 k init_lapw n initialization n symmetry detection (F, I, Ccentering, inversion) n input generation with recommended defaults n quality (and computing time) depends on k-mesh and R. Kmax (determines #PW) n run_lapw n scf-cycle n optional with SO and/or LDA+U n different convergence criteria (energy, charge, forces) n save_lapw tic_gga_100 k_rk 7_vol 0 n cp case. struct and clmsum files, n mv case. scf file n rm case. broyd* files n

Advantage/disadvantage of WIEN 2 k + + + ? robust all-electron full-potential method (new effective mixer) unbiased basisset, one convergence parameter (LDA-limit) all elements of periodic table (equal expensive), metals LDA, GGA, meta-GGA, LDA+U, spin-orbit many properties and tools (supercells, symmetry) w 2 web (for novice users) speed + memory requirements + very efficient basis for large spheres (2 bohr) (Fe: 12 Ry, O: 9 Ry) - less efficient for small spheres (1 bohr) (O: 25 Ry) - large cells, many atoms (n 3, but new iterative diagonalization) - full H, S matrix stored large memory required + effective dual parallelization (k-points, mpi-fine-grain) + many k-points do not require more memory - no stress tensor - no linear response

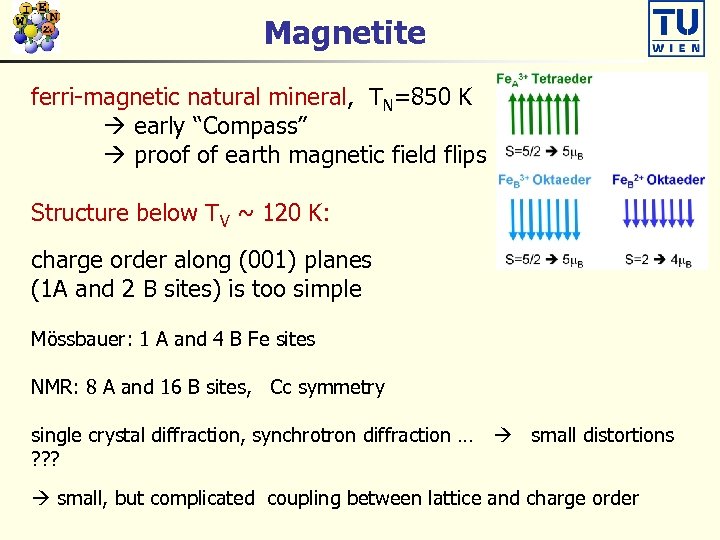
Magnetite ferri-magnetic natural mineral, TN=850 K early “Compass” proof of earth magnetic field flips Structure below TV ~ 120 K: charge order along (001) planes (1 A and 2 B sites) is too simple Mössbauer: 1 A and 4 B Fe sites NMR: 8 A and 16 B sites, Cc symmetry single crystal diffraction, synchrotron diffraction … small distortions ? ? ? small, but complicated coupling between lattice and charge order