MAE 5360: Hypersonic Airbreathing Engines Rayleigh Flow and Fanno Flow Overview Mechanical and Aerospace Engineering Department Florida Institute of Technology D. R. Kirk
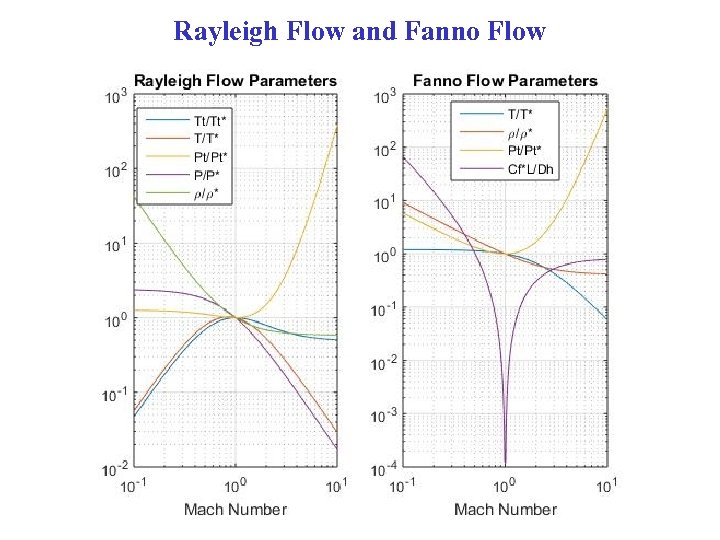
Rayleigh Flow and Fanno Flow
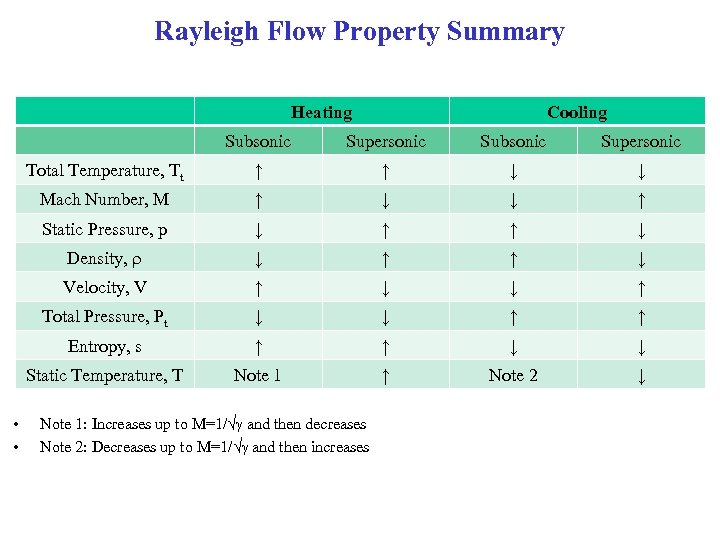
Rayleigh Flow Property Summary Heating Cooling Subsonic Supersonic Total Temperature, Tt ↑ ↑ ↓ ↓ Mach Number, M ↑ ↓ ↓ ↑ Static Pressure, p ↓ ↑ ↑ ↓ Density, r ↓ ↑ ↑ ↓ Velocity, V ↑ ↓ ↓ ↑ Total Pressure, Pt ↓ ↓ ↑ ↑ Entropy, s ↑ ↑ ↓ ↓ Static Temperature, T • • Supersonic Note 1 ↑ Note 2 ↓ Note 1: Increases up to M=1/√g and then decreases Note 2: Decreases up to M=1/√g and then increases
Rayleigh Flow Summary • Subsonic Inlet – Heating: • No limit to heat addition • Flow chokes more and more as we add more heat with inlet velocity → 0. – Cooling: • No theoretical limit on amount of cooling allowed • Exit flow becomes slower and slower • Exit T → 0 • Supersonic Inlet – Heating: • Even if M 1 → ∞, Tt 1/Tt* = 0. 4898 • If heat is added without limit to supersonic flow, normal shock wave adjustment required to accommodate property changes – Cooling: • Only finite amount of cooling can be allowed before exit Mach number → ∞ • Tt 2/Tt* = 0. 4898
Example 1 • Fuel-Air mixture enters a combustor duct • Inlet conditions: – V 1=75 m/s – P 1=150 k. Pa – T 1=300 K • There is heat transfer to the fluid at a rate of 900 k. J/kg • Assume that the flow is inviscid • Calculate – All properties at duct exit – Plot Rayleigh line on a T-S diagram – Increase heating to 1400 k. J/kg – Plot Rayleigh line on a T-S diagram
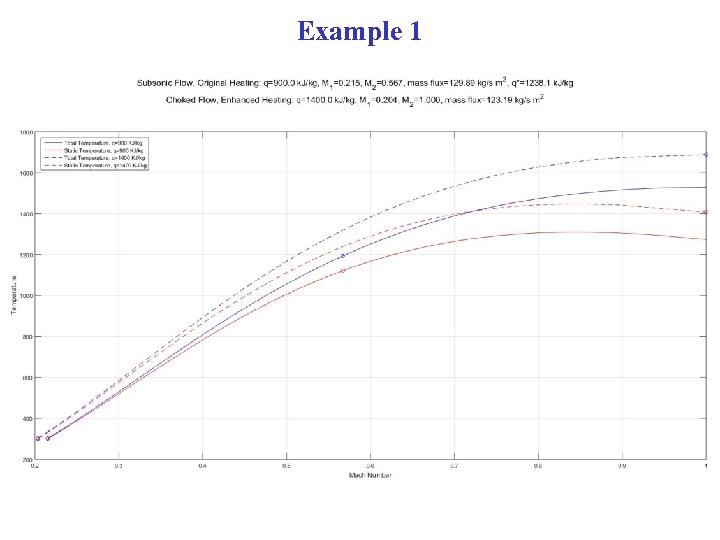
Example 1
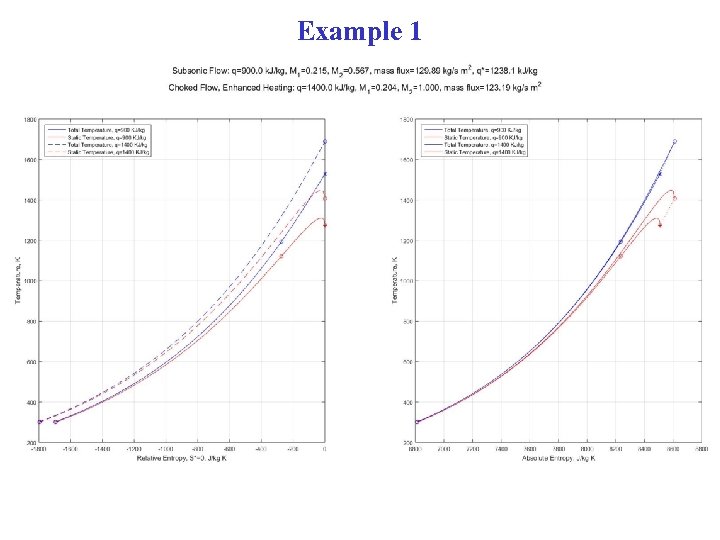
Example 1
Example 2 • Air enters a constant area duct of circular cross-section with diameter D = 20 cm • Inlet conditions: – M 1=0. 2 – Pt 1=100 k. Pa – Tt 1=288 K • There is heat transfer to the fluid at a rate of 100 k. W • Assume that the flow is inviscid • Calculate – Mass flow rate through the duct – The critical heat flux that would choke the duct for given M 1 – The exit Mach number, M 2 – The percentage total pressure loss – Entropy rise, DS – Static pressure drop – Plot Rayleigh line on a T-S diagram
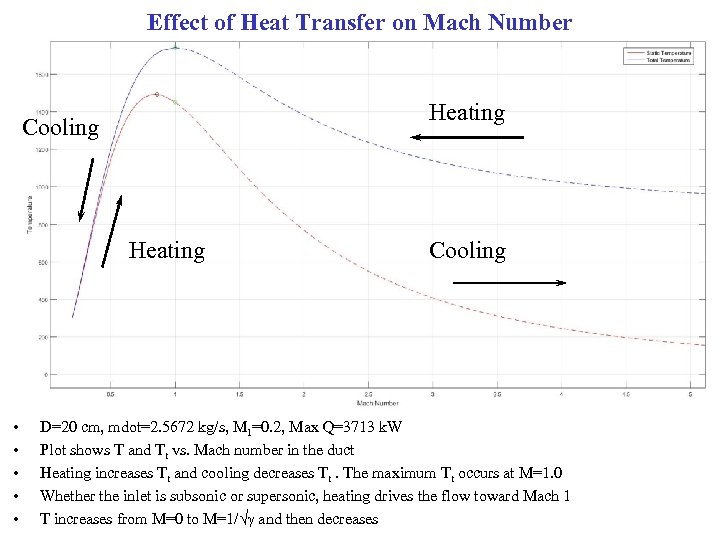
Effect of Heat Transfer on Mach Number Heating Cooling Heating • • • Cooling D=20 cm, mdot=2. 5672 kg/s, M 1=0. 2, Max Q=3713 k. W Plot shows T and Tt vs. Mach number in the duct Heating increases Tt and cooling decreases Tt. The maximum Tt occurs at M=1. 0 Whether the inlet is subsonic or supersonic, heating drives the flow toward Mach 1 T increases from M=0 to M=1/√g and then decreases
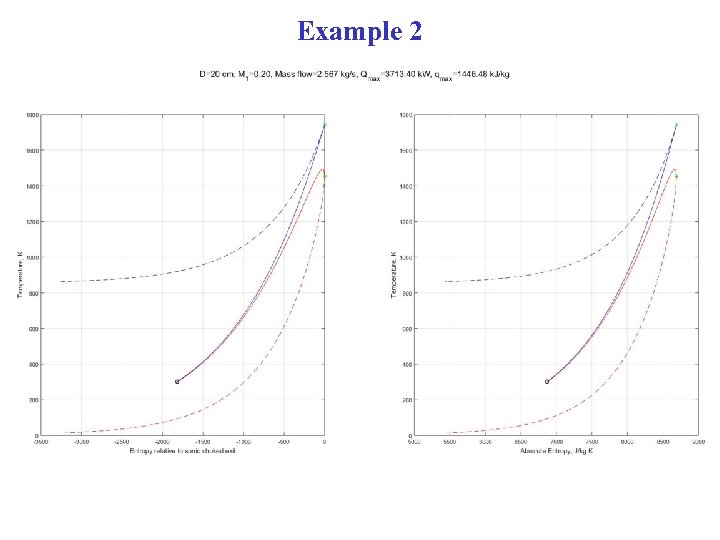
Example 2
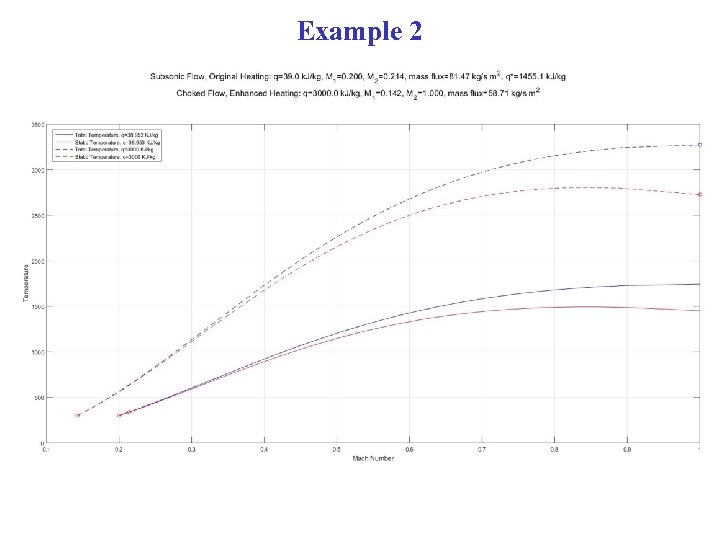
Example 2
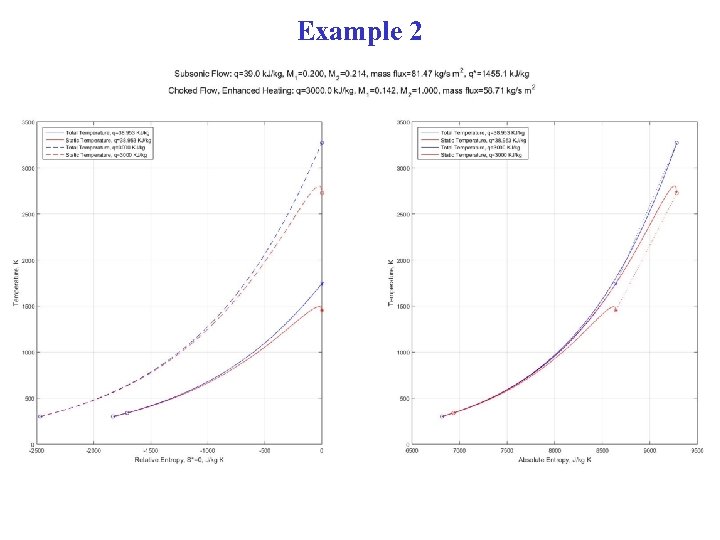
Example 2
Example 3: SCRAMJet Combustor • • • Air enters a constant area combustion chamber Inlet conditions: – M 1=3. 0 – Pt 1=100 k. Pa – Tt 1=1, 800 K Fuels (assume f << 1) – n-Decane (C 10 H 22): QR=48, 000 k. J/kg – Methane (C 10 H 22): QR=55, 500 k. J/kg – Hydrogen (H 2): QR=141, 800 k. J/kg Assume that the flow is inviscid Calculate – Exit total temperature if the exit is choked – Maximum heat release per unit mass of air – Required fuel-to-air ratio to thermally choke the combustor exit – Total pressure loss in the supersonic combustor
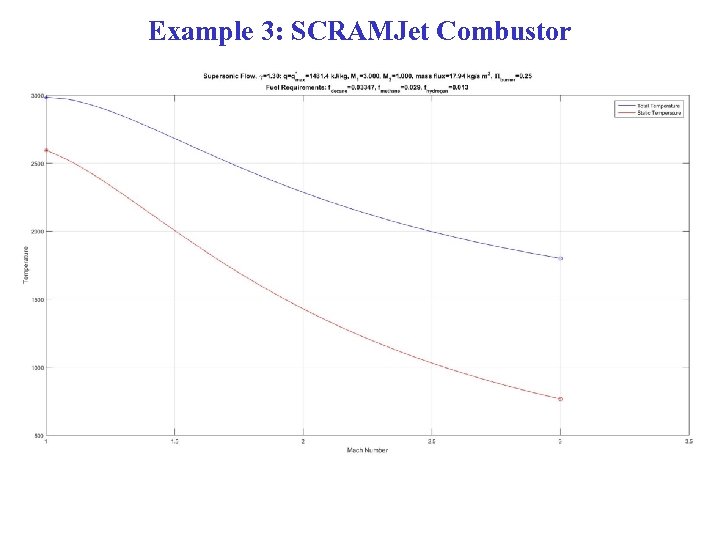
Example 3: SCRAMJet Combustor
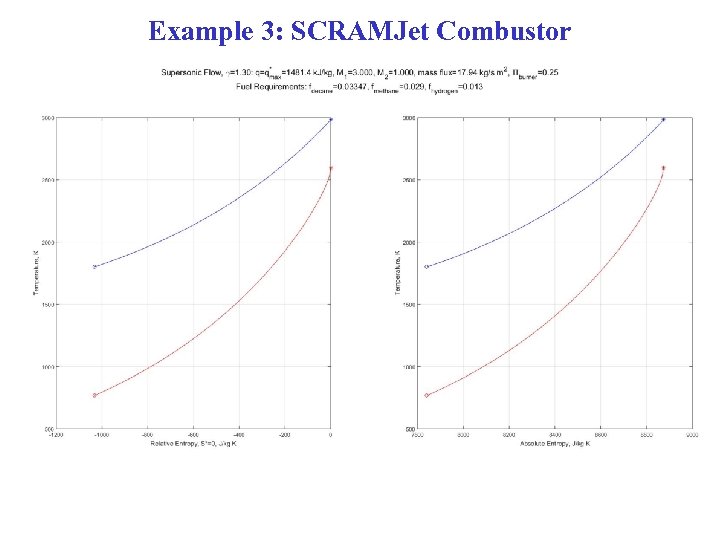
Example 3: SCRAMJet Combustor
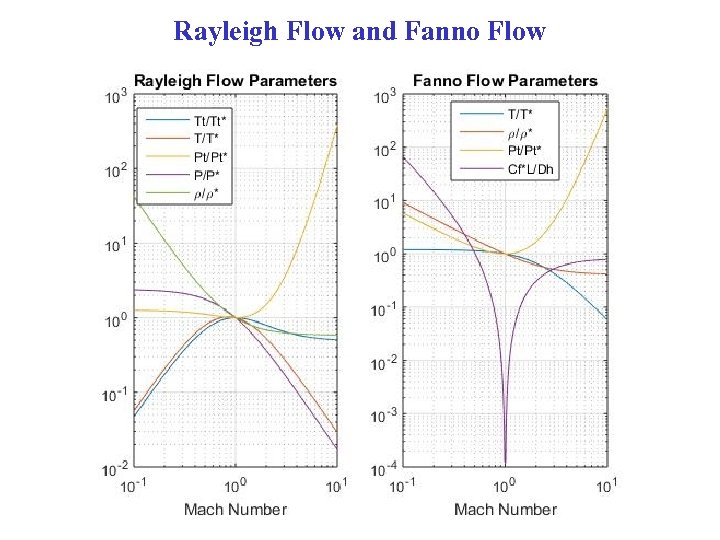
Rayleigh Flow and Fanno Flow
Rayleigh Flow and Fanno Flow: T-s
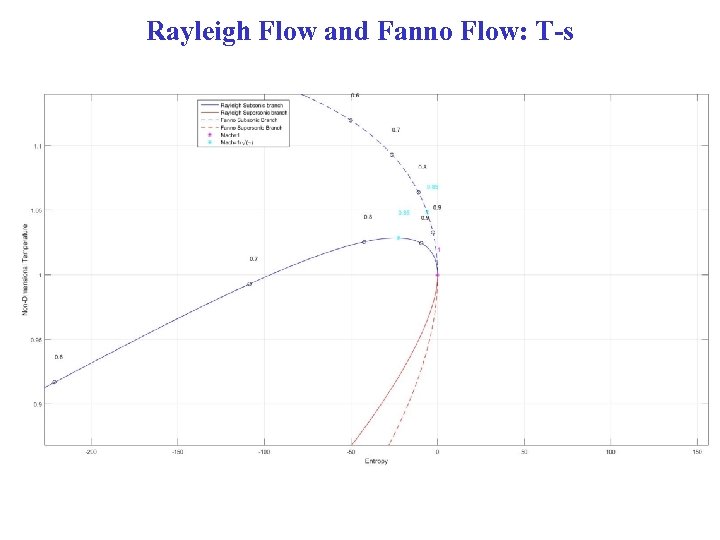
Rayleigh Flow and Fanno Flow: T-s
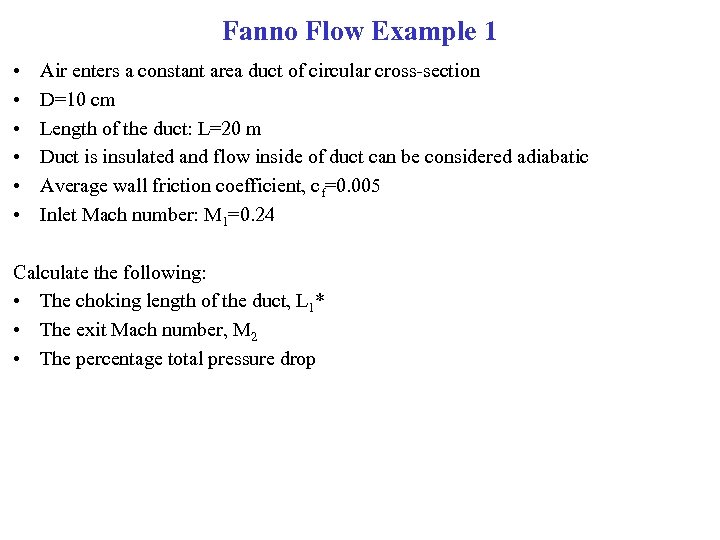
Fanno Flow Example 1 • • • Air enters a constant area duct of circular cross-section D=10 cm Length of the duct: L=20 m Duct is insulated and flow inside of duct can be considered adiabatic Average wall friction coefficient, cf=0. 005 Inlet Mach number: M 1=0. 24 Calculate the following: • The choking length of the duct, L 1* • The exit Mach number, M 2 • The percentage total pressure drop
Fanno Flow Example 1: Local Mach vs. Duct Length
Fanno Flow Example 1: T-s
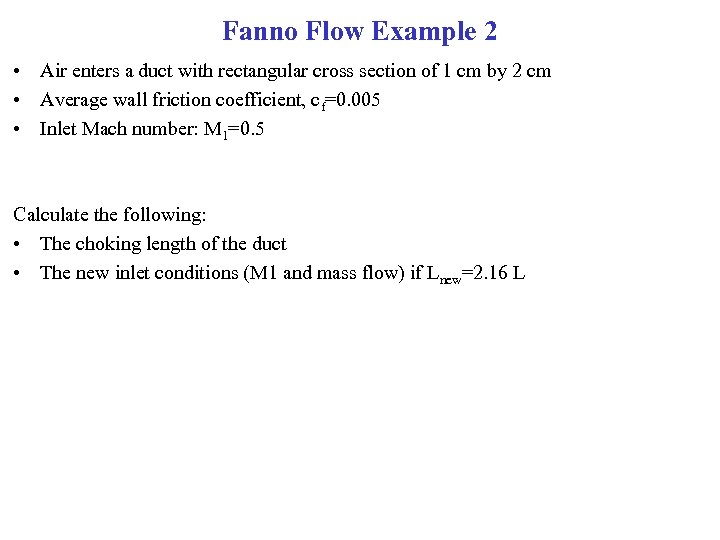
Fanno Flow Example 2 • Air enters a duct with rectangular cross section of 1 cm by 2 cm • Average wall friction coefficient, cf=0. 005 • Inlet Mach number: M 1=0. 5 Calculate the following: • The choking length of the duct • The new inlet conditions (M 1 and mass flow) if Lnew=2. 16 L
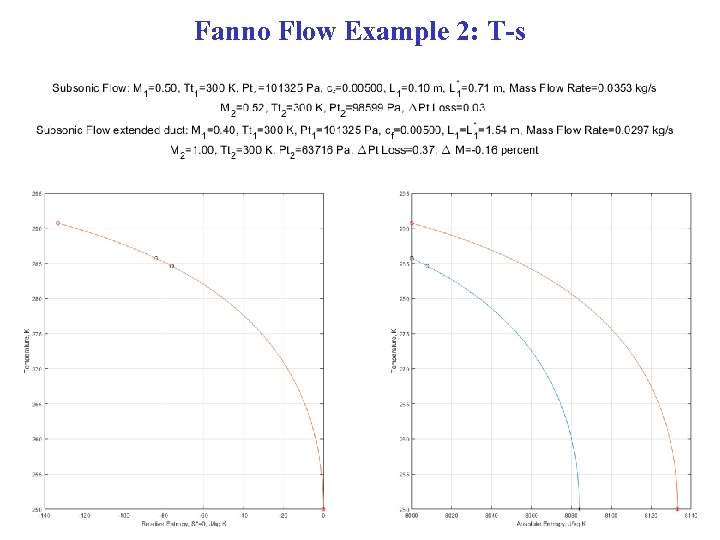
Fanno Flow Example 2: T-s
Example 3: Supersonic Duct Flow • Mach 3 flow enters isolator of hypersonic vehicle • Skin friction coefficient, cf=0. 05 • Consider several cases of isolator length, L – L/D=1 – L/D=4 – L/D=6 – L/D=8 (case does not work – why? )
Fanno Flow Example 3: L/D = 1, (L/D)*=2. 61
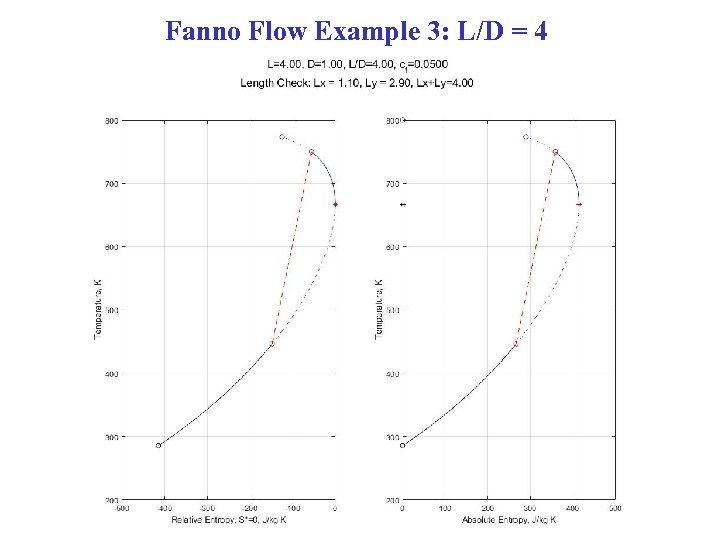
Fanno Flow Example 3: L/D = 4
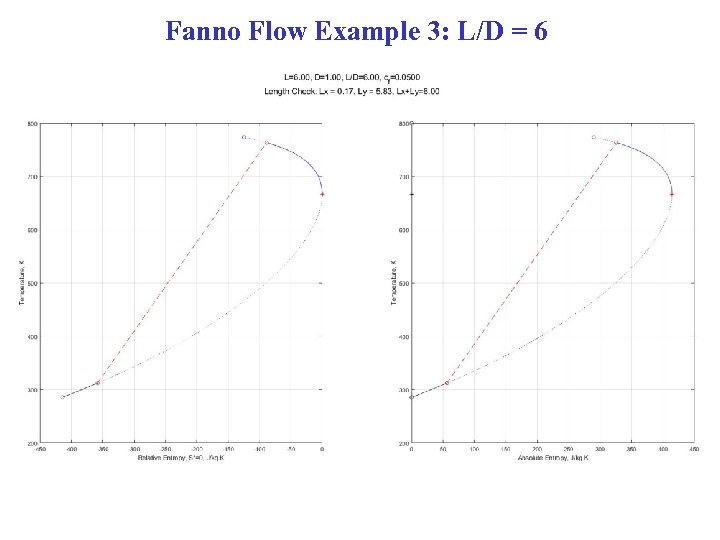
Fanno Flow Example 3: L/D = 6
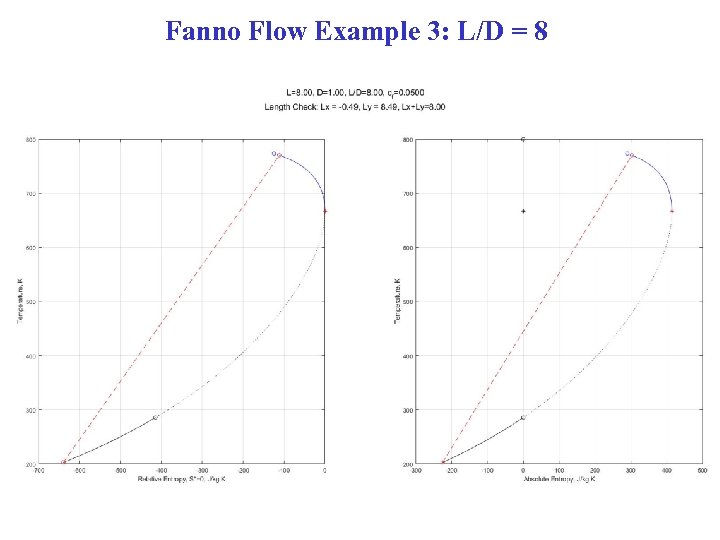
Fanno Flow Example 3: L/D = 8
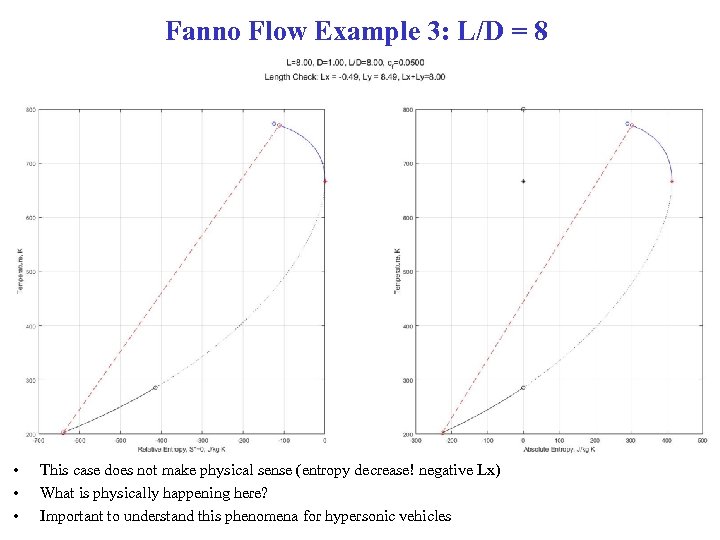
Fanno Flow Example 3: L/D = 8 • • • This case does not make physical sense (entropy decrease! negative Lx) What is physically happening here? Important to understand this phenomena for hypersonic vehicles
Скачать презентацию MAE 5360 Hypersonic Airbreathing Engines Rayleigh Flow and
7b5a5b89c92ac9662874981171c5bb1d.ppt