91e2c234fc0c9b99964342d286bdeeff.ppt
- Количество слайдов: 77

Macroeconomics & The Global Economy -Term III Ace Institute of Management Chapter 10: Keynesian Model and ISLM curves (Based on Macroeconomic Analysis: Edward Shapiro. Chap. 8, 9, 12 and Macroeconomics by N. G. Mankiw) Instructor Sandeep Basnyat Sandeep_basnyat@yahoo. com Mobile: 9841 892281

Classical approach-a reminder § Stable equilibrium in all sectors through market mechanism § The interaction between demand supply forces in a long run. § Even wage rate and prices moves both ways – upward and downward – adjusting with the change in demand supply in an economy. § No economy would suffer from over production, as supply will automatically create its own demand. § No government intervention Theories didn’t work with the Great Depression of 1930 s

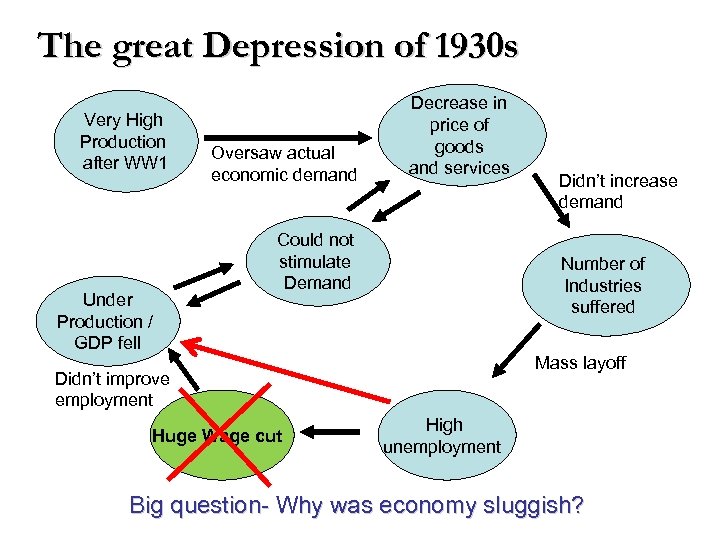
The great Depression of 1930 s Very High Production after WW 1 Oversaw actual economic demand Decrease in price of goods and services Could not stimulate Demand Under Production / GDP fell Number of Industries suffered Mass layoff Didn’t improve employment Huge Wage cut Didn’t increase demand High unemployment Big question- Why was economy sluggish?

Evolution of Keynesian Theory British Economist John Maynard Keynes analyzed economic issues in a different way through his world famous book – A General Theory of Employment, Interest and Money Basic Concepts: Effective demand Aggregate Demand Aggregate Supply

Keynesian Macroeconomics § His arguments were: § Due to the presence of strong labor union wage cut is not possible. Therefore Wage is sticky downwards and flexible upwards § Economy, thus, does not operate at full employment level, but at less than full employment level.
The great Depression of 1930 s Very High Production after WW 1 Under Production / GDP fell Oversaw actual economic demand Decrease in price of goods and services Could not stimulate Demand Number of Industries suffered Mass layoff Didn’t improve employment Huge Wage cut Didn’t increase demand High unemployment Big question- Why was economy sluggish?

Keynesian Macroeconomics § His arguments were: § Due to the presence of strong labor union wage cut is not possible. Therefore Wage is sticky downwards and flexible upwards § Economy, thus, does not operate at full employment level, but at less than full employment level. § Role of government is crucial because its policy decisions can affect level of aggregate demand, which in turn affects economic growth, employment and general price level

J. M. Keynes: Equilibrium in the Economy § For equilibrium, Planned Exp. = Actual Exp. § Planned Exp. Comes from Aggregate Demand (AD) where as Actual Exp. Comes from Aggregate Supply (AS) or National Income (Y) Therefore, AD = Y or, § For Eg: Two sector equilibrium: C+I =Y § The equilibrium is known as Keynesian Cross § Case 1: Y > C + I -> (Actual investment > planned investment) § Case 2: C + I > Y -> (Actual investment < planned investment) Note: Investment is an expenditure on expected demand

Components of Aggregate Demand § There are four types of demands in an economy § Consumption Demand (C) § Investment Demand (I) § Demand for Government Expenditure (G) § Demand for Foreign Trade (X-M)

Consumption Demand (C) § Reminder, . Y = C +S where, C = f (Yd) and S = f (Yd) § Marginal Propensity to Consume (mpc): The portion of any increase in disposable income that goes towards consumer spending. mpc = Change in Consumption Expenditure Change in Disposable Income = ∆ C / ∆Y d § Marginal Propensity to Save (mps): The portion of any increase in disposable income that is saved. Change in Savings = ∆S / ∆Y d mps = Change in Disposable Income If, mpc + mps = 1, then mpc = 1 - mps;
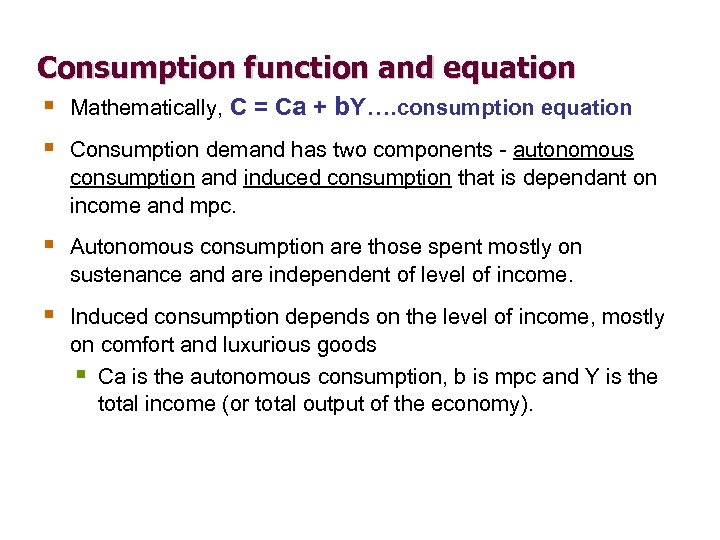
Consumption function and equation § Mathematically, C = Ca + b. Y…. consumption equation § Consumption demand has two components - autonomous consumption and induced consumption that is dependant on income and mpc. § Autonomous consumption are those spent mostly on sustenance and are independent of level of income. § Induced consumption depends on the level of income, mostly on comfort and luxurious goods § Ca is the autonomous consumption, b is mpc and Y is the total income (or total output of the economy).

Consumption Demand (continued …. ) § With the help of consumption equation we can construct hypothetical income-consumption schedule § Let Ca=50, b = 0. 75). Using, C = Ca + b. Y Income (Y) 0 100 200 300 400 Consumption (C) C 50 125 200 275 350 n tio p and dem m nsu o C Y The functional relationship between Income and Consumption demand is the consumption function as drawn before.
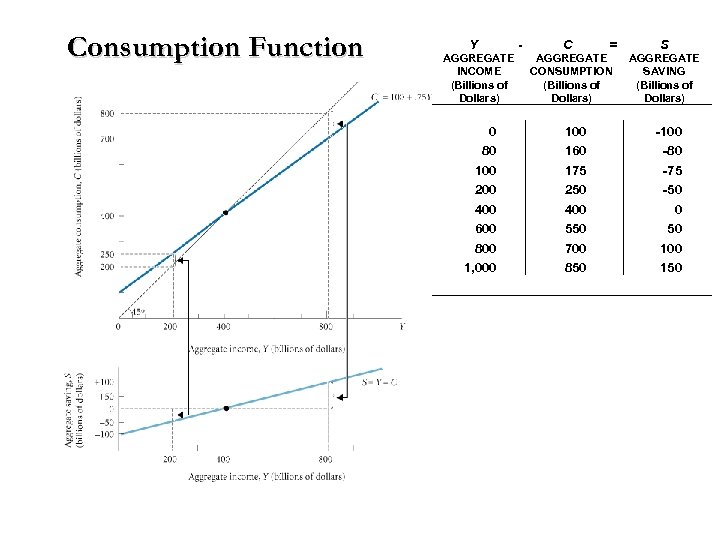
Consumption Function Y AGGREGATE INCOME (Billions of Dollars) 0 80 100 200 400 600 800 1, 000 - C = AGGREGATE CONSUMPTION (Billions of Dollars) 100 160 175 250 400 550 700 850 S AGGREGATE SAVING (Billions of Dollars) -100 -80 -75 -50 0 50 100 150
Investment Demand (I) § Spending by businesses on capital goods—factories, machinery, and other aids to production. § 2 influences on the economy: § Helps determine the economy’s level of total output and total § employment Critical determinant of the economy’s rate of growth as it enlarges the economy’s stock of capital goods and thereby helps increase the economy’s capacity to produce goods and services § There are 3 forms of investment. § Business Fixed Investment § Residential Fixed Investment and § Inventory Investment.
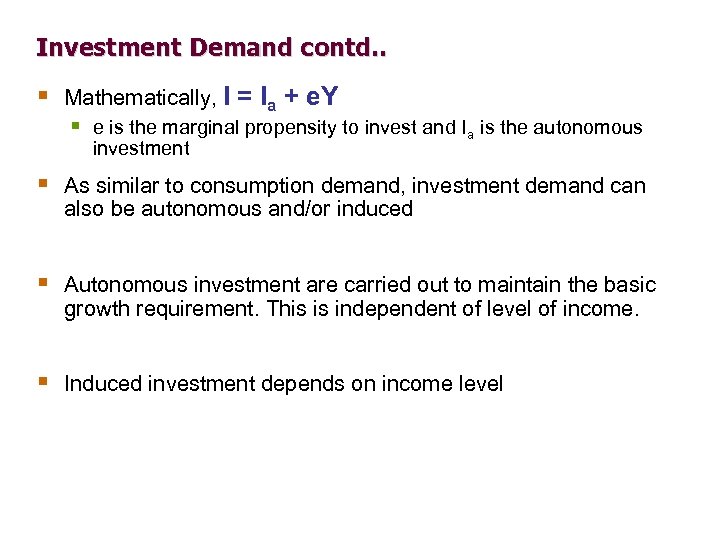
Investment Demand contd. . § Mathematically, I = Ia + e. Y § e is the marginal propensity to invest and Ia is the autonomous investment § As similar to consumption demand, investment demand can also be autonomous and/or induced § Autonomous investment are carried out to maintain the basic growth requirement. This is independent of level of income. § Induced investment depends on income level

Equilibrium Aggregate Output (Income) (All Figures in Billions of Dollars) (1) (2) (3) (4) (5) (6) UNPLANNED D AGGREGATE INVENTORY OUTPUT PLANNED EXPENDITURE (AE) EQUILIBRIUM? CONSUMPTION CHANGE (INCOME) (Y) INVESTMENT (I) C+I (Y = AE? ) (C= 100+. 75 Y) Y - (C + I) 100 175 25 200 - 100 No 200 25 275 - 75 No 400 25 425 - 25 No 500 475 25 500 0 Yes 600 550 25 575 + 25 No 800 700 25 725 + 75 No 1, 000 850 25 875 + 125 No
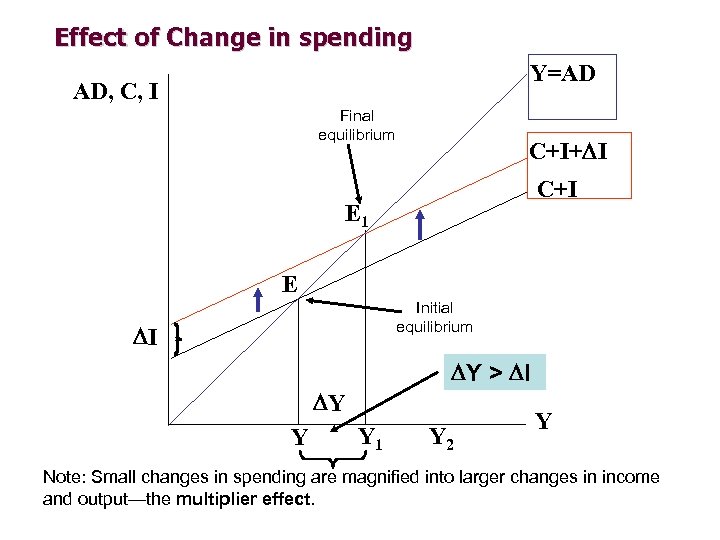
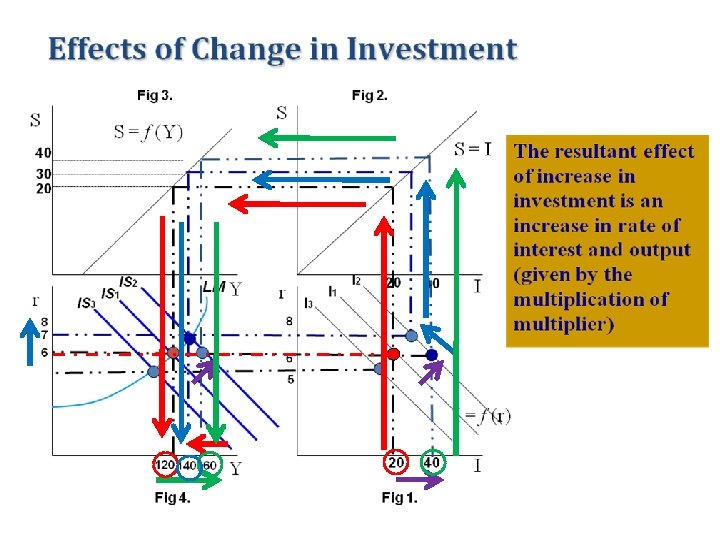
Effect of Change in spending Y=AD AD, C, I Final equilibrium C+I+ I C+I E 1 E Initial equilibrium I Y > I Y Y Y 1 Y 2 Y Note: Small changes in spending are magnified into larger changes in income and output—the multiplier effect.
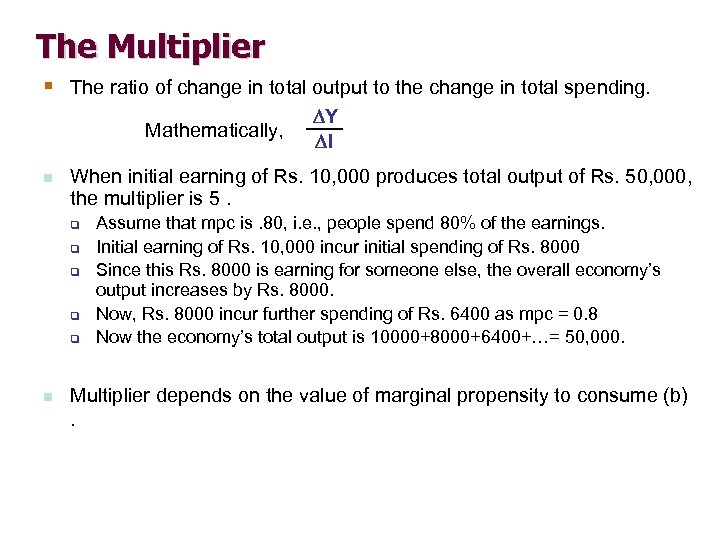
The Multiplier § The ratio of change in total output to the change in total spending. Mathematically, n When initial earning of Rs. 10, 000 produces total output of Rs. 50, 000, the multiplier is 5. q q q n Y I Assume that mpc is. 80, i. e. , people spend 80% of the earnings. Initial earning of Rs. 10, 000 incur initial spending of Rs. 8000 Since this Rs. 8000 is earning for someone else, the overall economy’s output increases by Rs. 8000. Now, Rs. 8000 incur further spending of Rs. 6400 as mpc = 0. 8 Now the economy’s total output is 10000+8000+6400+…= 50, 000. Multiplier depends on the value of marginal propensity to consume (b).

The Multiplier How much is the multiplier here?
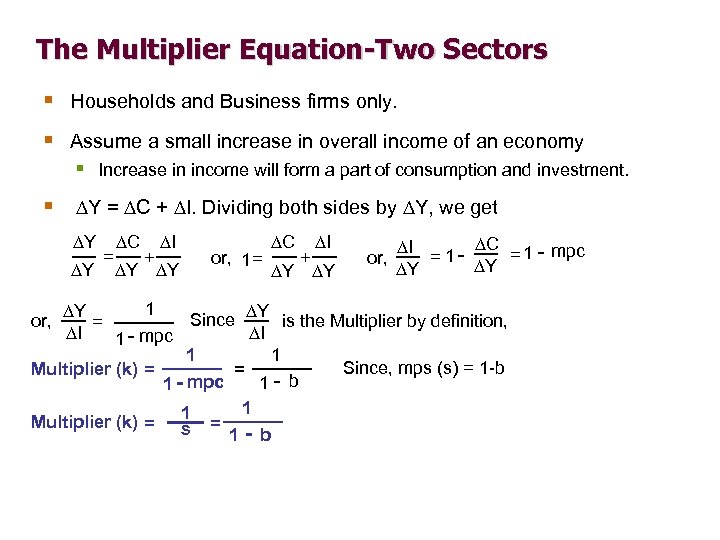
The Multiplier Equation-Two Sectors § Households and Business firms only. § Assume a small increase in overall income of an economy § Increase in income will form a part of consumption and investment. § Y = C + I. Dividing both sides by Y, we get Y Y = C I + Y Y or, 1 = C I + Y Y C I = 1 - Y = 1 - mpc or, Y 1 Y Since Y is the Multiplier by definition, = I I 1 - mpc 1 1 Since, mps (s) = 1 -b Multiplier (k) = = -b 1 1 - mpc 1 Multiplier (k) = 1 = s 1 - b or,

Finding equilibrium in 2 sector economy § For equilibrium, Y = C+I Let us assume that all investment is autonomous, So, I = Ia (Remember I = Ia + e. Y) Then, Y = Ca + b. Y + Ia or Y – b. Y = Ca + Ia or (1 -b)Y = Ca + Ia or Y = [1/(1 -b)](Ca + Ia) Hence, equilibrium output is [1/(1 -b)] (Ca + Ia) As, [1/(1 -b)] is the multiplier and is denoted by k, equilibrium output will be Y = k (Ca + Ia)

Effect of Change in Investment What happens if there is an increase in investment? § Let I be increased by I. This will subsequently increase the output by Y § New investment will be I + I and corresponding output will be Y + Y Hence, Y+ Y = k (Ca + Ia+ I) or Y = k (Ca + Ia) + k I – Y = Y + k I – Y Y = k I This shows that an increase in investment by I will increase the output by k I.

Basic Keynesian Model-3 sectors economy § The aggregate demand of an economy will be total demand created by three sectors namely, household, business and government. § Government spending (G): purchases of goods and services by government § Cases: What happens to equilibrium when government uses its fiscal policies? Note: § Increase in ‘G’ increases total output § Increase in Revenue through tax decreases total output § Works with Multiplier effect

Government In The Economy-Equilibrium
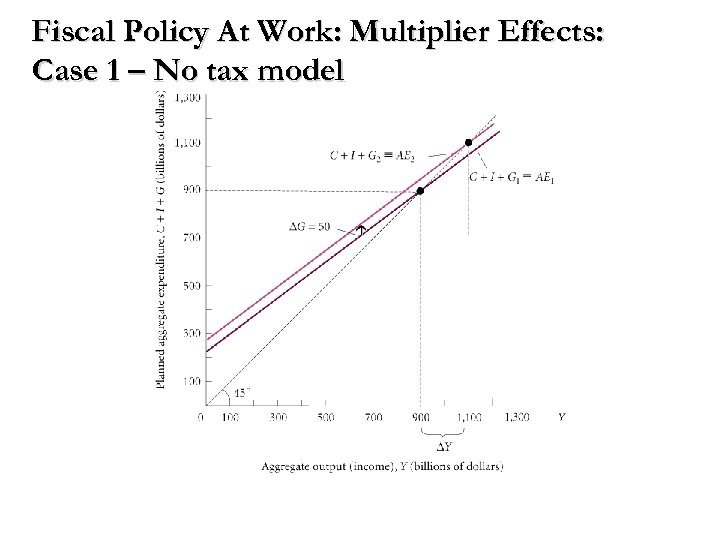
Fiscal Policy At Work: Multiplier Effects: Case 1 – No tax model
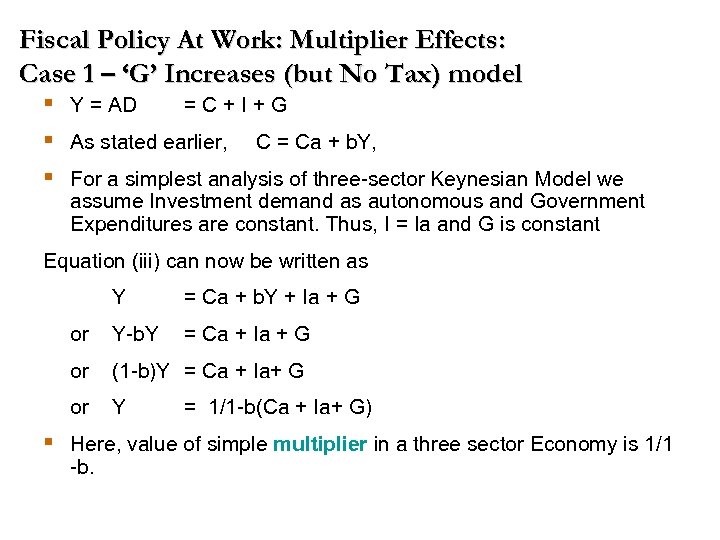
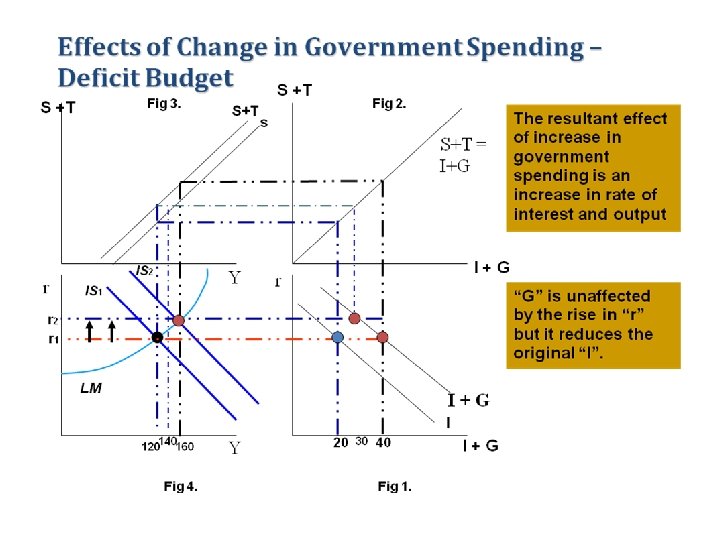
Fiscal Policy At Work: Multiplier Effects: Case 1 – ‘G’ Increases (but No Tax) model § Y = AD =C+I+G § As stated earlier, C = Ca + b. Y, § For a simplest analysis of three-sector Keynesian Model we assume Investment demand as autonomous and Government Expenditures are constant. Thus, I = Ia and G is constant Equation (iii) can now be written as Y = Ca + b. Y + Ia + G or Y-b. Y = Ca + Ia + G or (1 -b)Y = Ca + Ia+ G or Y = 1/1 -b(Ca + Ia+ G) § Here, value of simple multiplier in a three sector Economy is 1/1 -b.

Multiplier Effects: Case 2 – Tax imposed by the govt. § When tax is imposed, the disposable income of a consumer decreases by Y-T. C = Ca + b. Y= Ca + b(Y-T) Assume, I = Ia and G is constant Y = Ca + b(Y-T) + Ia + G or Y – b. Y = Ca + Ia + G – b. T or (1 -b)Y = Ca + Ia + G – b. T or Y = 1/1 -b(Ca + Ia + G – b. T)

Keynesian Economics-Four Sector (Open) Economy n n Export and Import are included in a four sector economy. Exports depend on the income of foreigners and therefore it is exogenously determined in the home country. Import on the other hand is a function of level of income. Import is given by M = Ma + m. Y q Ma = Autonomous Import and m. Y = Induced Import

Four Sector Economy- Case 1: Simple Keynesian model including the external sector § Y = AD = C + I + G + (X-M) Where C = Ca + b. Y and M = Ma + m. Y § I is autonomous and G and X are exogenously determined § Now, putting these values in Y, Y = Ca + b. Y + I + G + X – Ma – m. Y or Y – b. Y + m. Y = Ca + I + G + X – Ma or (1 -b+m)Y = Ca + I + G + X – Ma or Y = (1/1 -b+m)(Ca + I + G + X – Ma) So, Equilibrium Output: Y = (1/1 -b+m)(Ca + I + G + X – Ma) Value of Multiplier: 1/1 -b+m

Keyne’s explanation on-The Recessionary and Inflationary § § § S = National Saving I = National Investment S – I = Saving investment gap If S > I, National Surplus Govt. can utilize the surplus if, G > T. How much, G-T = Expenditure revenue gap § For an economy to be in equilibrium, S – I = G-T
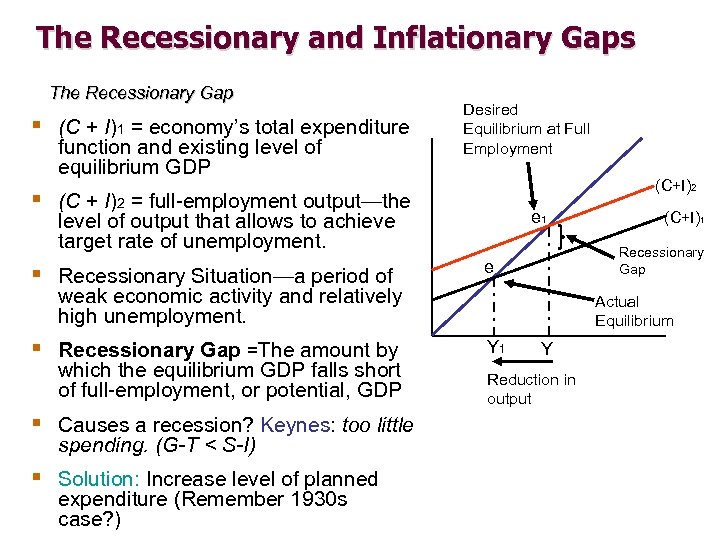
The Recessionary and Inflationary Gaps The Recessionary Gap § (C + I)1 = economy’s total expenditure function and existing level of equilibrium GDP Desired Equilibrium at Full Employment (C+I)2 § (C + I)2 = full-employment output—the e 1 level of output that allows to achieve target rate of unemployment. § Recessionary Situation—a period of Recessionary Gap e weak economic activity and relatively high unemployment. § Recessionary Gap =The amount by which the equilibrium GDP falls short of full-employment, or potential, GDP § Causes a recession? Keynes: too little spending. (G-T < S-I) § Solution: Increase level of planned expenditure (Remember 1930 s case? ) (C+I)1 Actual Equilibrium Y 1 Y Reduction in output

Two Gaps Model-The Recessionary and Inflationary Gaps The Inflationary Gap § (C + I)1 = economy’s planned expenditure function and existing level of equilibrium GDP Desired Equilibrium at Full Employment § (C + I)2 = full-employment output—the level of (C+I)1 output that allows to achieve target rate of unemployment. e § If the economy is operating in full employment level, the economy cannot provide more than Y 1 level of goods and services. Inflationary Gap e 1 § If consumers and investors attempt to purchase more output than the economy is capable of producing, higher prices result as prospective buyers bid against one another. § But Real GDP will not increase. Only money GDP § Inflationary Gap =The amount by which the equilibrium GDP exceeds full-employment, or potential, GDP (G-T > S-I) § Solution: Decrease level of planned expenditure. (C+I)2 Actual Equilibrium Y 1 Y Increase in potential GDP

Paradox of thrift § Where higher savings become disincentive in an economy when it leads to reduction in national output. S 1 S § Shift of savings curve towards left results a shift in equilibrium point § Given an unchanged Investment I curve, the shift in savings curve towards left decreases output subsequently from “Y” to “Y 1”. § Thriftiness (increased desire to save) without support from other economic variables can have undesired consequences Y 1 Y

One Big Question ! How would the market be in equilibrium? • Develop Goods market and money market • Check how goods market and money market interact to determine the level of output and the interest rate.

The Goods Market and the Money Market Goods market: in which goods and services are exchanged n Equilibrium level of price of a product is determined by the demand the supply of the product Money market : The market in which financial instruments are exchanged n Equilibrium level of the interest rate is determined by the supply of money and the demand for money

The Goods Market Equilibrium § In two sector economy, equilibrium level of goods market is given by Y = C + I (Keynes) § Similarly, We know, Y = C+S. § So, C + I = C + S or S = I § Therefore, in two sector economy, goods market is also in equilibrium if S = I (financial market) How will the Goods Market be in equilibrium?

The Goods Market Equilibrium § We know that, § Savings is the positive function of Income S = f(Y) § Investment is the inverse function of rate of interest I = f(r);
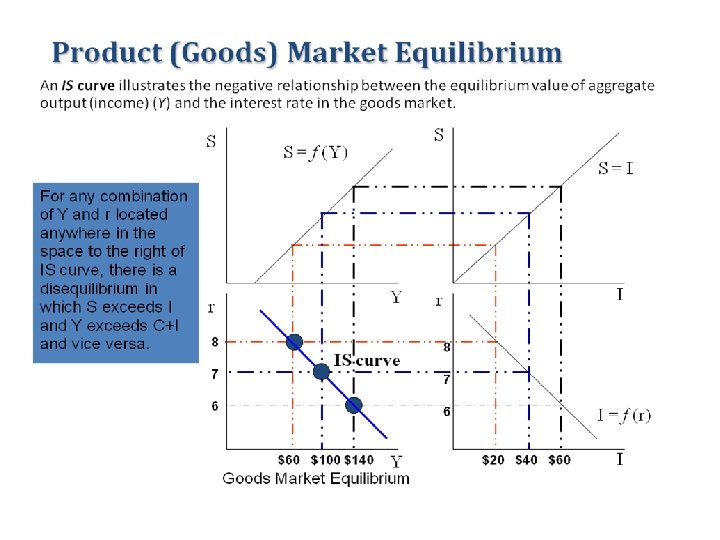

Goods Market Disequilibrium r S>I Y>C+I E 8 7 G S>I Y>C+I . . F Disequilibrium Point I>S C+I>Y IS 60 100 Y

Money Market Equilibrium MD = M S Keyne’s 3 Determinants of Demand for money: § Transaction Demand § Precautionary Demand § Speculative Demand 1. Transaction Demand: The main reason that people hold money—to buy things. § As the income level increases people want to spend more money. § So, Transaction Demand is the direct function of Income. DT = f (Y)

Money Market Equilibrium Precautionary Demand for Money n n People hold money to meet emergencies and unexpected contingencies As in the case of transaction demand, it is some fraction of total income and is positively related with the changes in money income DP = f (Y) Note: In most of cases precautionary demand is combined with transaction demand.
Money Market Equilibrium Speculative Demand for Money n n n Speculative demand for Money refers to demand for holding certain amount of cash in reserve to make speculative gain out of purchase and sale of bonds and securities. The amount people prefer to maintain idle cash balance for speculative purpose depends on the rate of interest in the economy There is an inverse relationship between speculative demand for money and rate of interest DSP = f (r) Now Total Money Demand, MD = DT + DSP

Money Market Equilibrium Supply of Money § Money Supply is exogenously determined – that is from outside this model § The central monetary authority fixes the level of nominal stock of money supply. § For equality in money market, Money Demand must be equal to Money supply MD = M S Since, Total Money Demand, MD = DT + DSP Now Total Money Supply, Ms = DT + DSP
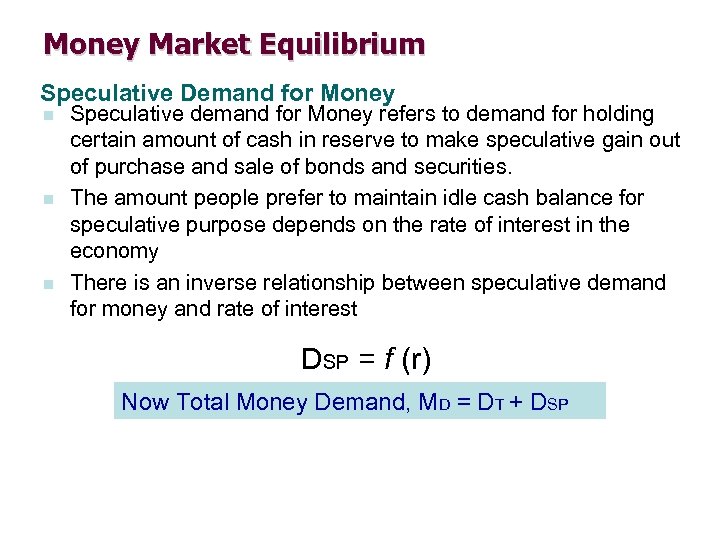
Money Market Equilibrium-Liquidity Preference Theory The money market is in equilibrium when Md = Ms The equilibrium interest rate is the point at which the quantity of money supplied equals the quantity of money demanded. If the interest rate is initially high enough to create an excess supply of money, the interest rate will immediately fall, discouraging people from moving out of money and into bonds. If the interest rate is initially low enough to create an excess demand for money, the interest rate will immediately rise, discouraging people from moving out of bonds and into money.

Money Market Equilibrium An LM curve illustrates the positive relationship between the equilibrium value of the interest rate and aggregate output (income) (Y) in the money market. High level of income calls for relatively large transaction balances, which, with a given supply of money can be drawn out of speculative balances only by pushing up the interest rates. Transaction Demand Money Supply DT DT DT = f (Y) Total Ms =$100 $60 $50 MS = DT + DSP $50 $100 $120 DSP Y r r Assume Total Ms =$100 LM curve 6 6 5 DSP = f (r) 5 $100 $120 Money Market Equilibrium Ms = Md Y $40 $50 Speculative Demand DSP
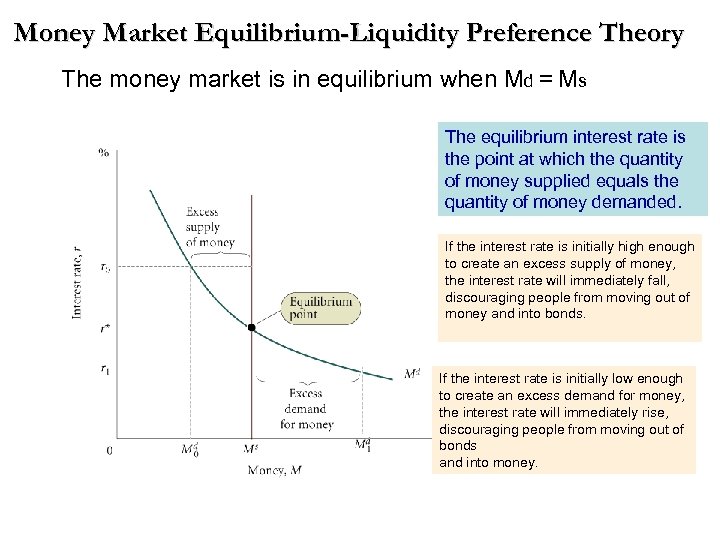
Money Market Disequilibrium r LM Ms > Md Md > Ms For any combination located in the space to the right of the LM curve, there is a disequilibrium in which Md > Ms. 6 5 F E 4 100 120 For any combination located in the space to the left of the LM curve, there is a disequilibrium in which Ms > Md. Y

Two Market Equilibrium-The Goods and Money Markets r LM re IS Ye Y
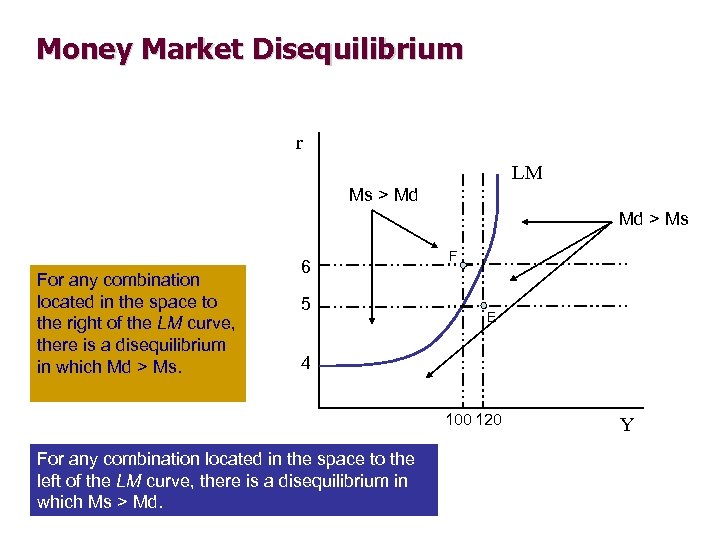
Two Market Equilibrium and Disequilibrium S>I and MD<MS r LM I>S and MD<MS re S>I and MD>MS IS I>S and MD>MS Ye Y
A numerical example. Consider the economy of Nepal. § The consumption function is given by C = 250+0. 75(Y-T). The investment function is I = 200 -25 r, where r is the interest rate. Government purchases and taxes are both 100. For this economy, find the IS equation. § The money demand function in Nepal is (M/P)d = Y -100 r. The money supply Ms is 1, 000 and the price level is 2. For this economy, find the LM equation. § Find the equilibrium interest rate and the equilibrium level of income. Assume that r =1 is equal to r =1%. § Suppose that the government purchases are raised from 100 to 150. How much does the IS curve shift? What are the new equilibrium interest rate and level of income? § With the initial values of monetary and fiscal policies, What are the new equilibrium interest rate and level of income when: § § a) money supply doubles in the economy b) price level rises from 2 to 4. What happens?

A numerical example The consumption function is given by C = 250+0. 75(Y-T). The investment function is I = 200 -25 r, where r is the interest rate. Government purchases and taxes are both 100. For this economy, find the IS equation. Solution: Consumption: C = 250 + 0. 75(Y − T) Investment: I = 200 − 25 r Balanced budget: T = G = 100 IS equation? We know in three sector economy, the IS equation is Y = C + I + G.
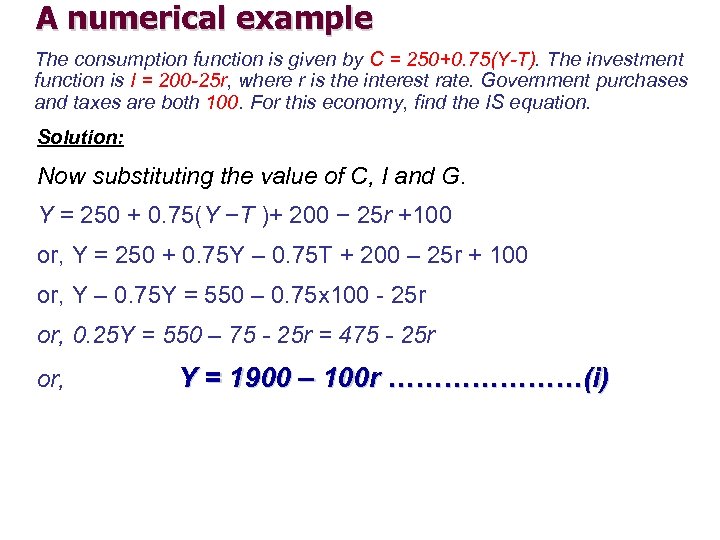
A numerical example The consumption function is given by C = 250+0. 75(Y-T). The investment function is I = 200 -25 r, where r is the interest rate. Government purchases and taxes are both 100. For this economy, find the IS equation. Solution: Now substituting the value of C, I and G. Y = 250 + 0. 75(Y −T )+ 200 − 25 r +100 or, Y = 250 + 0. 75 Y – 0. 75 T + 200 – 25 r + 100 or, Y – 0. 75 Y = 550 – 0. 75 x 100 - 25 r or, 0. 25 Y = 550 – 75 - 25 r = 475 - 25 r or, Y = 1900 – 100 r …………………(i)
A numerical example. Consider the economy of Nepal. § The consumption function is given by C = 250+0. 75(Y-T). The investment function is I = 200 -25 r, where r is the interest rate. Government purchases and taxes are both 100. For this economy, find the IS equation. § The money demand function in Nepal is (M/P)d = Y -100 r. The money supply Ms is 1, 000 and the price level is 2. For this economy, find the LM equation. § Find the equilibrium interest rate and the equilibrium level of income. Assume that r =1 is equal to r =1%. § Suppose that the government purchases are raised from 100 to 150. How much does the IS curve shift? What are the new equilibrium interest rate and level of income? § With the initial values of monetary and fiscal policies, What are the new equilibrium interest rate and level of income when: § § a) money supply doubles in the economy b) price level rises from 2 to 4. What happens?

A numerical example b) Solution: § The money demand function (M/P)d = Y -100 r. § Money Supply (Ms) = 1000 and § Price Level (P) = 2 LM equation? Money market equilibrium, Ms = Md Substituting the value of Ms and Md, 1000 = Y − 100 r Or, Y = 1000 + 100 r or …………. . (2) or, r = - 10 + 0. 01 Y
A numerical example. Consider the economy of Nepal. § The consumption function is given by C = 250+0. 75(Y-T). The investment function is I = 200 -25 r, where r is the interest rate. Government purchases and taxes are both 100. For this economy, find the IS equation. § The money demand function in Nepal is (M/P)d = Y -100 r. The money supply Ms is 1, 000 and the price level is 2. For this economy, find the LM equation. § Find the equilibrium interest rate and the equilibrium level of income. Assume that r =1 is equal to r =1%. § Suppose that the government purchases are raised from 100 to 150. How much does the IS curve shift? What are the new equilibrium interest rate and level of income? § With the initial values of monetary and fiscal policies, What are the new equilibrium interest rate and level of income when: § § a) money supply doubles in the economy b) price level rises from 2 to 4. What happens?

A numerical example Here, IS Equation: Y = 1900 – 100 r and LM Equation: Y = 1000 + 100 r Solving IS-LM Equation, Therefore, Y = 1450 Substituting the value of Y in r r = - 10 + 0. 01 Y = -10 + 0. 01 x 1450 = -10 + 14. 5 = 4. 5 Therefore, r = 4. 5%

A numerical example. Consider the economy of Nepal. § The consumption function is given by C = 250+0. 75(Y-T). The investment function is I = 200 -25 r, where r is the interest rate. Government purchases and taxes are both 100. For this economy, find the IS equation. § The money demand function in Nepal is (M/P)d = Y -100 r. The money supply Ms is 1, 000 and the price level is 2. For this economy, find the LM equation. § Find the equilibrium interest rate and the equilibrium level of income. Assume that r =1 is equal to r =1%. § Suppose that the government purchases are raised from 100 to 150. How much does the IS curve shift? What are the new equilibrium interest rate and level of income? § With the initial values of monetary and fiscal policies, What are the new equilibrium interest rate and level of income when: § § a) money supply doubles in the economy b) price level rises from 2 to 4. What happens?
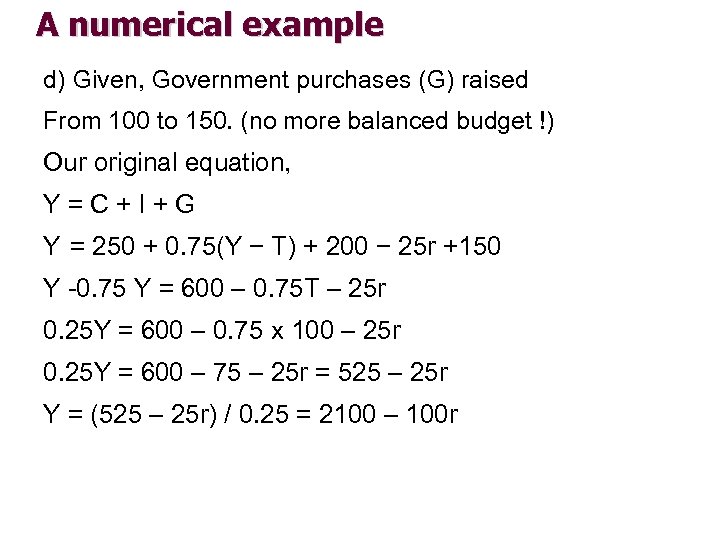
A numerical example d) Given, Government purchases (G) raised From 100 to 150. (no more balanced budget !) Our original equation, Y=C+I+G Y = 250 + 0. 75(Y − T) + 200 − 25 r +150 Y -0. 75 Y = 600 – 0. 75 T – 25 r 0. 25 Y = 600 – 0. 75 x 100 – 25 r 0. 25 Y = 600 – 75 – 25 r = 525 – 25 r Y = (525 – 25 r) / 0. 25 = 2100 – 100 r
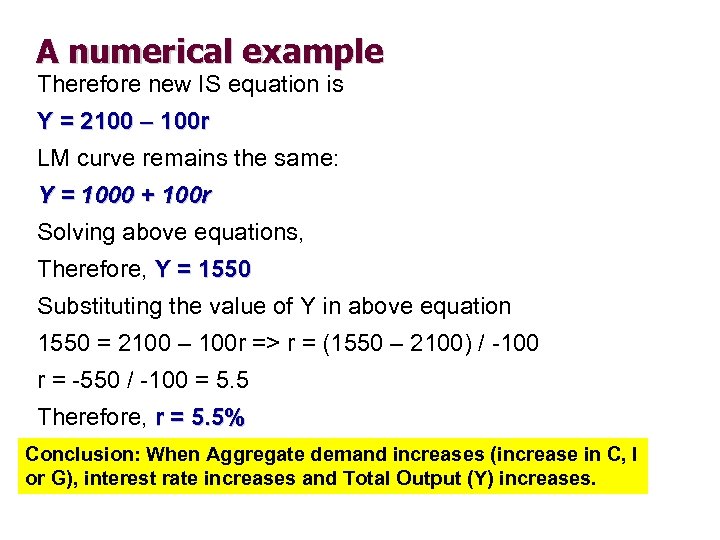
A numerical example Therefore new IS equation is Y = 2100 – 100 r LM curve remains the same: Y = 1000 + 100 r Solving above equations, Therefore, Y = 1550 Substituting the value of Y in above equation 1550 = 2100 – 100 r => r = (1550 – 2100) / -100 r = -550 / -100 = 5. 5 Therefore, r = 5. 5% Conclusion: When Aggregate demand increases (increase in C, I or G), interest rate increases and Total Output (Y) increases.

A numerical example. Consider the economy of Nepal. § The consumption function is given by C = 250+0. 75(Y-T). The investment function is I = 200 -25 r, where r is the interest rate. Government purchases and taxes are both 100. For this economy, find the IS equation. § The money demand function in Nepal is (M/P)d = Y -100 r. The money supply Ms is 1, 000 and the price level is 2. For this economy, find the LM equation. § Find the equilibrium interest rate and the equilibrium level of income. Assume that r =1 is equal to r =1%. § Suppose that the government purchases are raised from 100 to 150. How much does the IS curve shift? What are the new equilibrium interest rate and level of income? § With the initial values of monetary and fiscal policies, What are the new equilibrium interest rate and level of income when: § § a) money supply doubles in the economy b) price level rises from 2 to 4. What happens?

A numerical example-Ms doubles b) Given, The money demand function (M/P)d = Y -100 r. § Since Ms doubles Ms = 2000 § Now New LM curve is, 2000 = Y -100 r. New LM Equation is, Y = 2000 + 100 r Solving new LM and old IS curve r = 0. 5% Y = 2050 Conclusion: When Money Supply increases, interest rate decreases and Total Output (Y) increases.
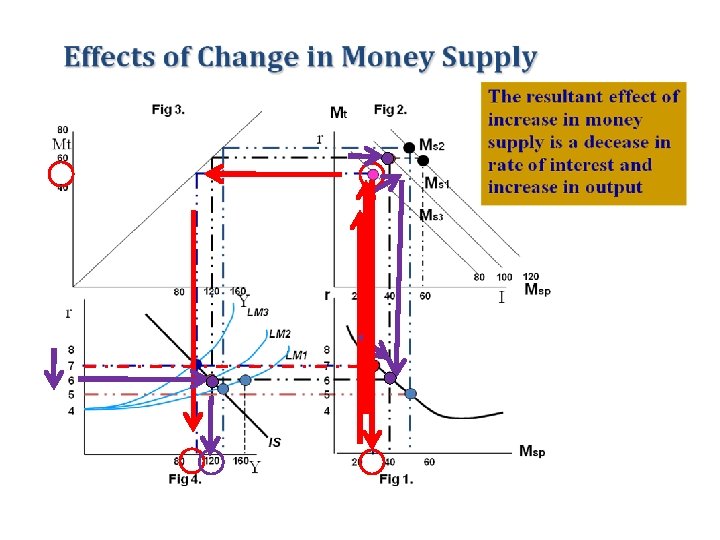

A numerical example-P increases from 2 to 4 b) Given, The money demand function (M/P)d = Y -100 r. § Since (M/P) = Ms, Any increase in “M” increases Ms and any increase in “P” decreases Ms. § When P = 2, Ms = 1000 So, when, P = 4, Ms = 500 Now, original equation becomes, 500 = Y -100 r => New LM Equation is Y = 500 + 100 r or, r = -5 + 0. 01 Y
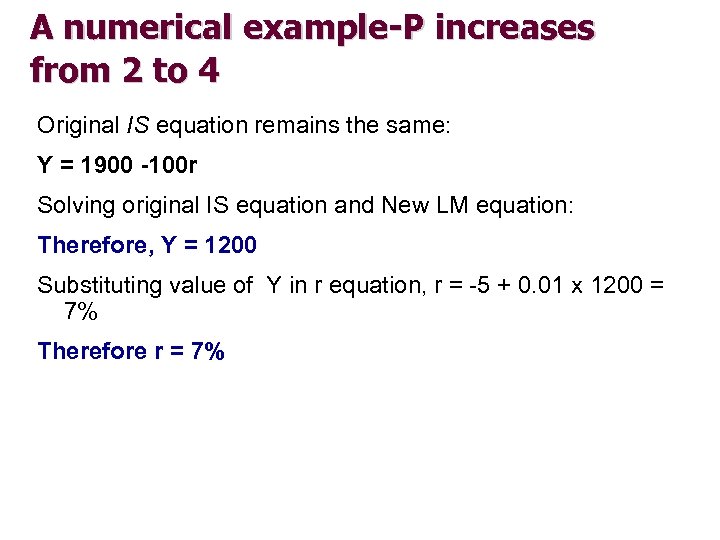
A numerical example-P increases from 2 to 4 Original IS equation remains the same: Y = 1900 -100 r Solving original IS equation and New LM equation: Therefore, Y = 1200 Substituting value of Y in r equation, r = -5 + 0. 01 x 1200 = 7% Therefore r = 7%

A numerical example. Price Level changes-graphical presentation LM 1 r LM 7% 4. 5% IS 1200 1450 Y

How do government policies affect the market? § Monetary policy § Fiscal Policy

IS and LM and Monetary-Fiscal Policies r r 5 r 4 LM Curve Classical Range r 3 r 2 r 1 Keynesian Range Intermediate Range Y Different policies have different effects on different ranges

Use of Fiscal and Monetary Policies r Assume Very Low. Close to Zero LM 1 IS 2 LM 2 Liquidity Trap- Central Bank’s liquidity can not increase further output r 1 Y 2 Y Keynesian Range- as observed by Keynes

Keynesian Range-Liquidity Trap Situation § Liquidity Trap- a situation when the nominal § interest rate is close or equal to zero and the monetary authority is unable to stimulate the economy with traditional monetary policy tools (failure of monetary policy). People do not expect high returns on physical or financial investments, so they keep assets in cash bank accounts.
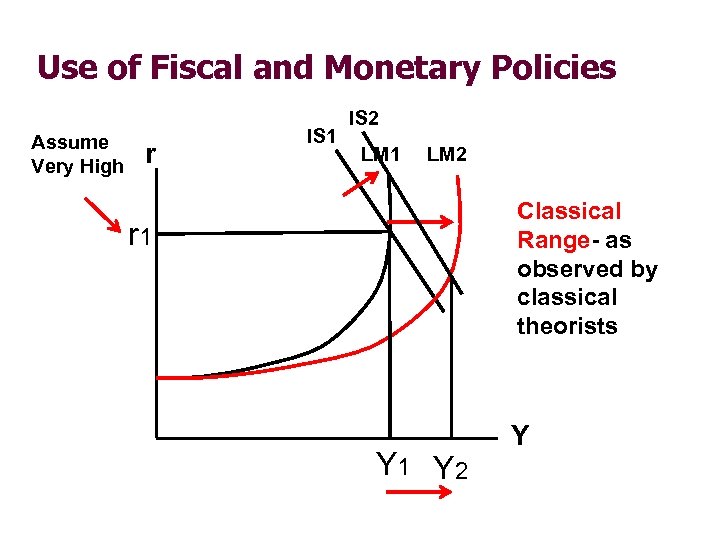
Use of Fiscal and Monetary Policies Assume Very High r IS 1 IS 2 LM 1 LM 2 Classical Range- as observed by classical theorists r 1 Y 2 Y

Monetary and Fiscal Policy-The Intermediate Range-Shift in IS curve LM r IS’ 2 r 3 IS 2 r 1 Y 2 Y 3 The more closer is the equilibrium towards Keynesian range, more effective the fiscal policy is.
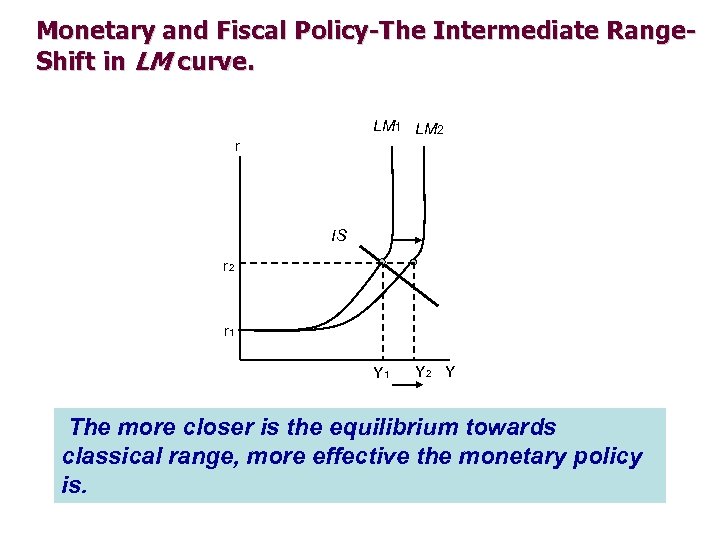
Monetary and Fiscal Policy-The Intermediate Range. Shift in LM curve. LM 1 LM 2 r IS r 2 r 1 Y 2 Y The more closer is the equilibrium towards classical range, more effective the monetary policy is.

Effectiveness of Monetary and Fiscal Policies-General Conclusion Range Keynesian Range Intermediate Range Classical Range Most Effective Ineffective Effective Most Effective Policy Fiscal Policy Monetary Policy Ineffective

Try it ! § Money Demand: Md = Y (0. 2 -i) where i is the rate of interest and Y is the income. Nominal Income Y = 2000 and Money Supply (Ms) = 300 § Find Md for i = 10 % (or 0. 1) and i = 5 % (or 0. 05) § What is the relation between i and Y § Graph Ms and Md and Calculate the equilibrium i. § Central bank increases the Money Supply by 50. What happens to money market equilibrium? (solve and graph)

Try it ! § Find the simultaneous equilibrium for the goods and money market when C = 100 + 0. 80 Yd; I = 150 – 6 i; T = 0. 25 Y; G = 100; Md = 0. 2 Y-2 i and Ms = 150. § Calculate equilibrium rate of interest and output if C = 100 + 0. 8 Y, I = 150 - 6 i, M = 150 and L = 0. 20 Y – 4 i

Thank You 77
91e2c234fc0c9b99964342d286bdeeff.ppt