M Elin The Galilee Research Center for Applied

M. Elin The Galilee Research Center for Applied Mathematics of ORT Braude College Karmiel, Israel 1

Continuous Semigroups

Spirallike and starlike mappings 3

Spirallike and starlike mappings In the one-dimensional settings - the well-known criteria of Nevanlinna, Study and Špaček In multi-dimensional situations – Suffridge, Gurganus, Pfaltzgraff, Gong, … In multi-dimensional situations – not all of the analogues hold, proofs are very complicated, examples are rather hard to construct. 4

Extension Operators Since the work of Roper and Suffridge in 1995, there has been considerable interest in constructing holomorphic mappings of the unit ball in a Banach space with various geometric properties by using mappings with similar properties acting in a subspace. Such properties include convexity, starlikeness, spirallikeness, and so on. It is also of interest to extend subordination chains, semigroups and semigroup generators. 5

Roper-Suffridge extension operator

Modifications of R-S extension operator Pfaltzgraff, Suffridge, 1997 I. Graham, G. Kohr, M. Kohr, 2000 I. Graham, G. Kohr, 2000 7

Modifications of R-S extension operator - the chain rule is invertible 8

Some notations 9

Main notation and notion 10

Extension operators for semigroups 11

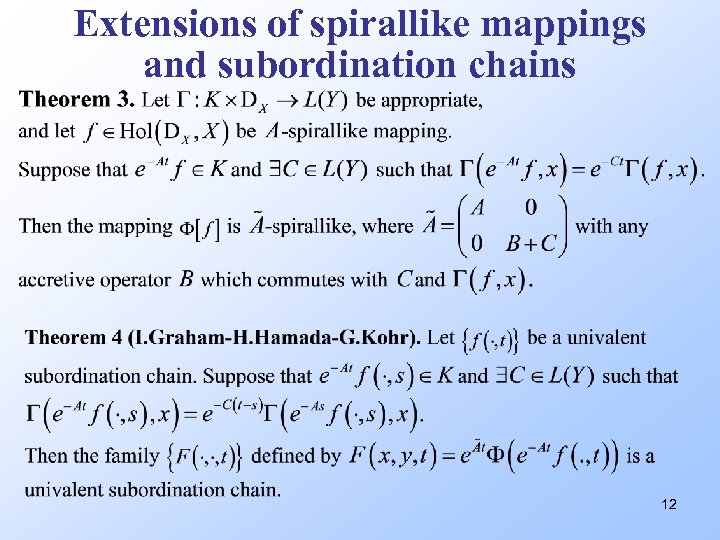Extensions of spirallike mappings and subordination chains 12

Extensions of spirallike mappings 13

Extensions of spirallike mappings 14

Extensions of spirallike mappings 15

Extreme Points, Support Points Further question Extension operator: 16

Muir’s extension operator

Geometric explanation

Geometric explanation

Covering results

Roper-Suffridge type operator

Spiralikeness for Muir’s type operator

Thank you for your attention! 23