Lemma of force translation to a parallel position

Lemma of force translation to a parallel position Задача: осуществить параллельный перенос силы F в точку О, не изменяя состояние тела Совокупность (Q’, F) образует Уравновешенная система сил пару сил, которая полностью характеризуется моментом может быть приложена к телу без изменения его состояния. Выберем систему Полученная система (Q, M) эквивалентна исходной (Q, Q’) такую, что Q=-Q’=F

Lemma of force translation to a parallel position The force applied at the point O of a rigid body is equivalent to the same force applied at the another point of the body and couple with the moment equal to the moment of the original force with respect to the new center

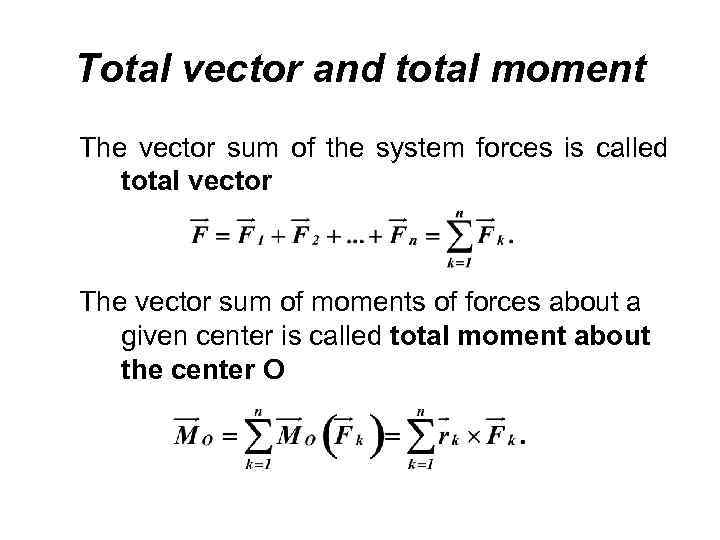Total vector and total moment The vector sum of the system forces is called total vector The vector sum of moments of forces about a given center is called total moment about the center O

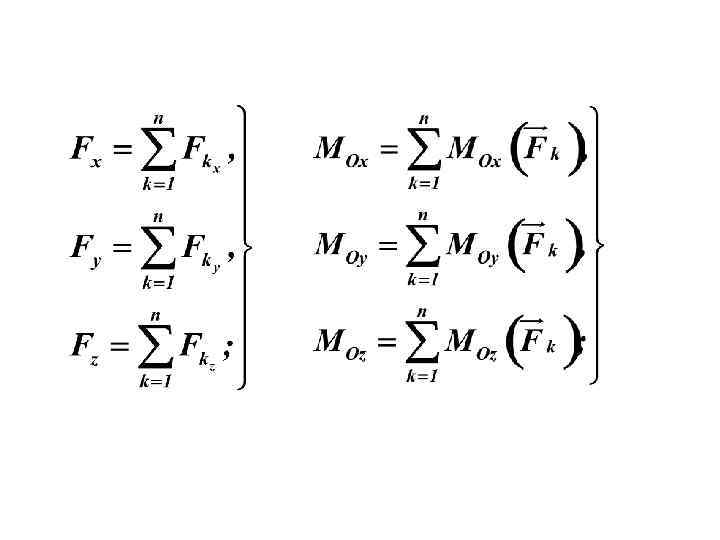
The simplest equivalent of general force system (Основная теорема статики) Theorem. General force system applied to a rigid body is equivalent to a set of two elements: a force and a couple. The force is equal to the total vector and is applied at the arbitrary chosen center. The couple has vector moment equal to the total moment of original force system about chosen center.

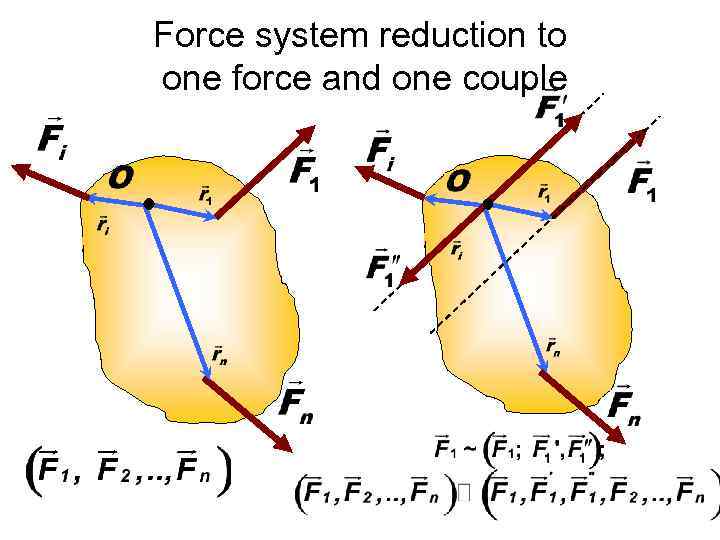Force system reduction to one force and one couple

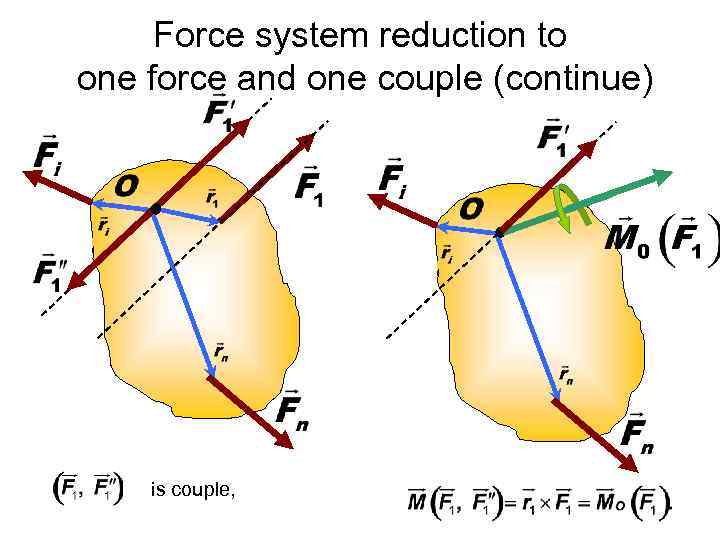Force system reduction to one force and one couple (continue) is couple,

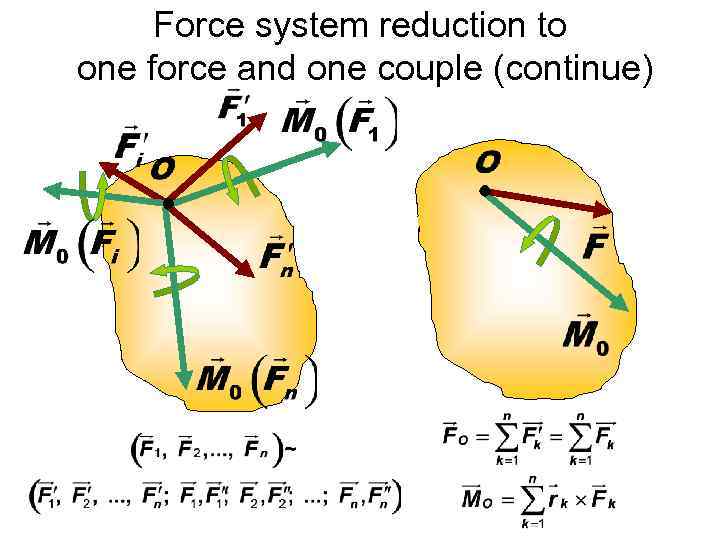Force system reduction to one force and one couple (continue)

Total vector and total moment dependence on the center of reduction position For any chosen reduction center, the total vector is equal to the vector sum of the system forces. So the total vector is independent of the reduction center position, i. e. the total vector is invariant with respect to the center of reduction.

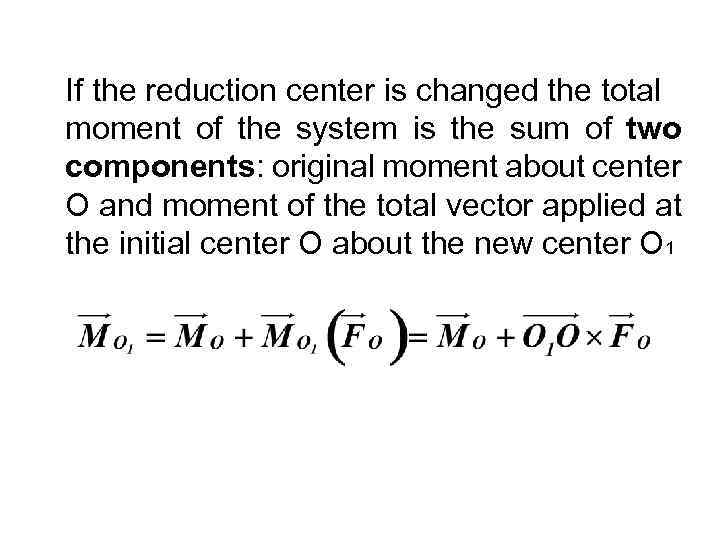If the reduction center is changed the total moment of the system is the sum of two components: original moment about center O and moment of the total vector applied at the initial center O about the new center O 1

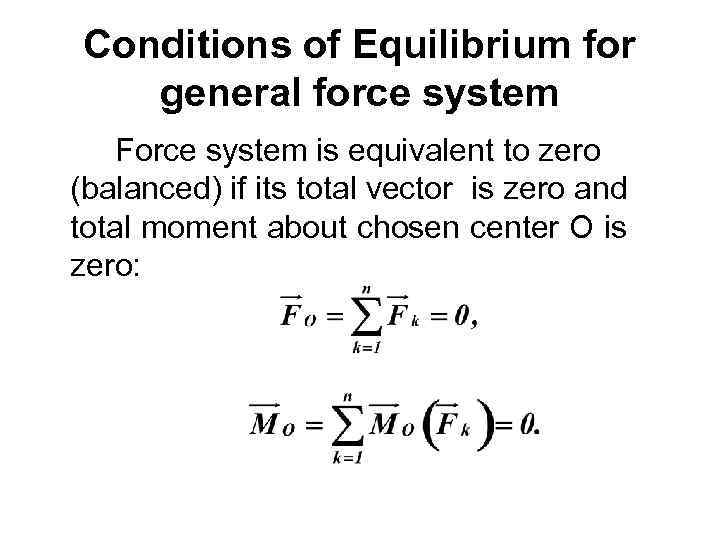Conditions of Equilibrium for general force system Force system is equivalent to zero (balanced) if its total vector is zero and total moment about chosen center O is zero:

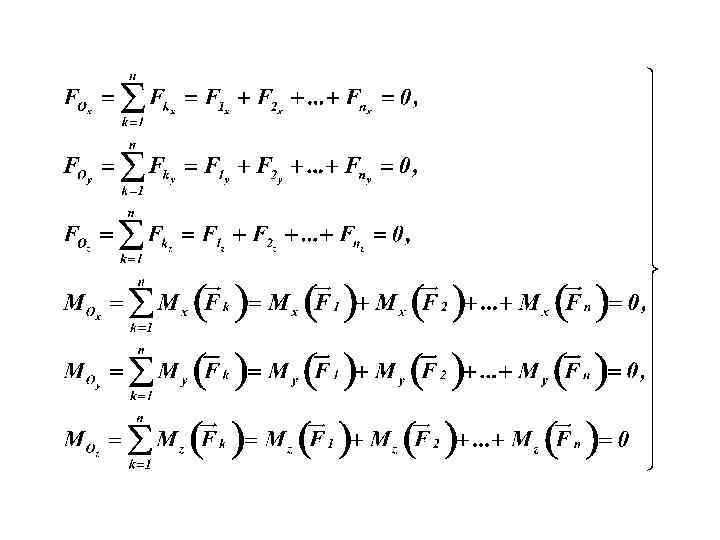
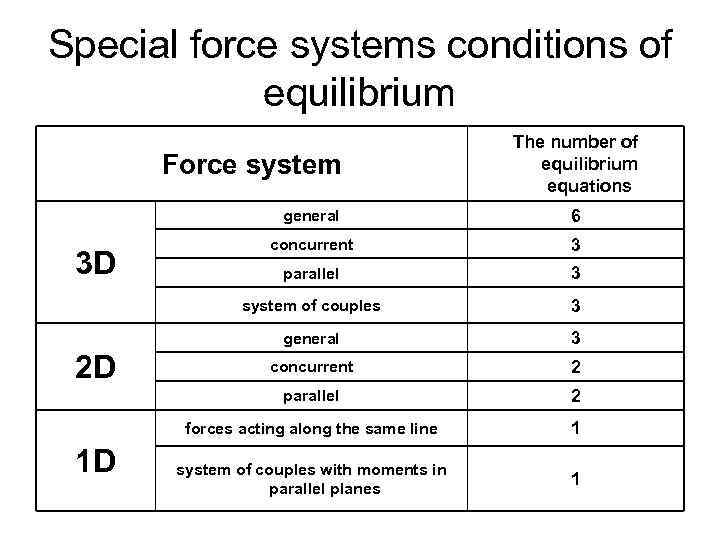Special force systems conditions of equilibrium Force system The number of equilibrium equations general parallel 3 3 3 concurrent 2 parallel 2 forces acting along the same line 1 D 3 general 2 D concurrent system of couples 3 D 6 1 system of couples with moments in parallel planes 1

Bodies system equilibrium Bodies systems (or structures) analyzed in our course are subdivided on: 1. Sets of bodies that simply supported. 2. Frames that are engineering structures designed to support loads, frames are usually stationary fully constrained structures. 3. Machines that are engineering structures designed to transmit and modify forces and are structures containing moving parts.

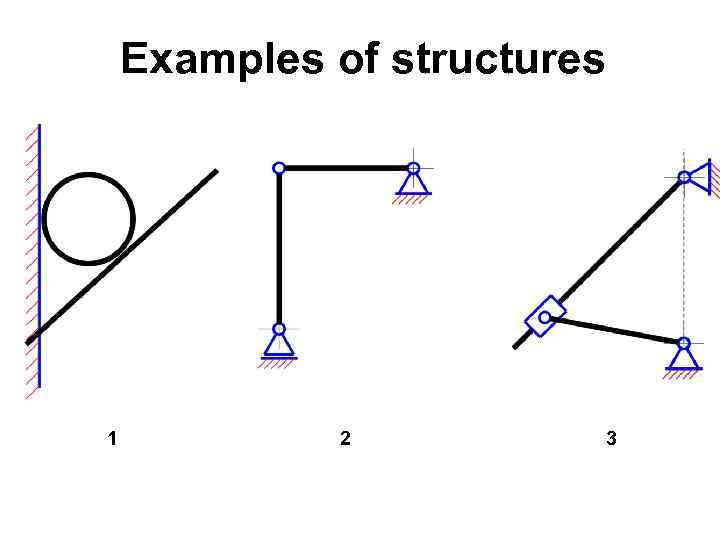Examples of structures 1 2 3

Method of section 1. Divide the system of bodies onto the parts by destroying (sectioning) the internal constraints between the bodies of the system. 2. Form FBD for each part of the system. For each adjacent part at the section apply the reactions corresponded to the type of the destroyed constraint. The direction you choose for each of the internal force components exerted on the first part is arbitrary, but you must apply equal and opposite force components of the same name to the other parts. 3. For each part write the equilibrium conditions taking into consideration all forces acting on the part with including reactions of destroyed internal constraints. 4. Analyze the statically determinacy of the problem. For the structure consisting of n members under the action of general coplanar force system it is possible to form 3 n independent equations of equilibrium. If the total number of unknowns is no more than the number of independent equations (3 n) the problem is statically determinate and can be solved by the methods of statics. 5. Solve these 3 n equations as a system; check the accuracy of your solution using the equilibrium conditions for the structure as a whole.

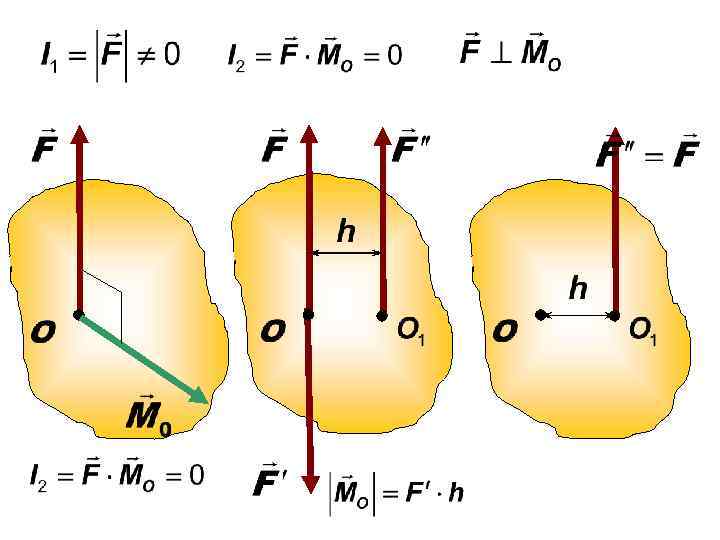
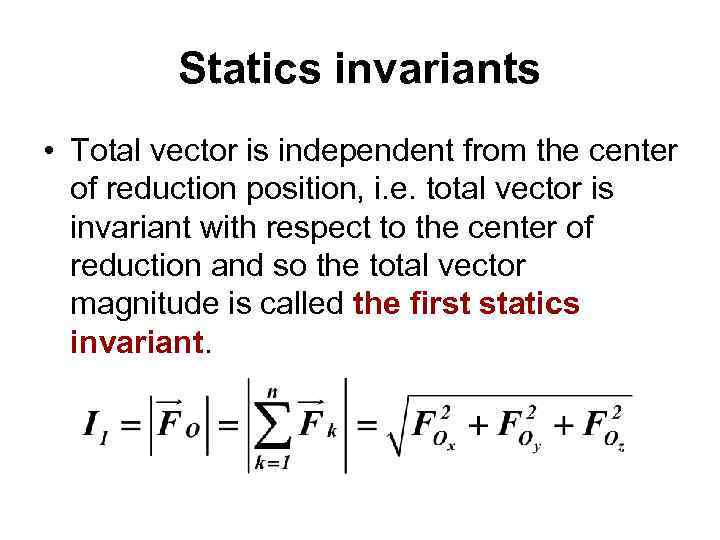Statics invariants • Total vector is independent from the center of reduction position, i. e. total vector is invariant with respect to the center of reduction and so the total vector magnitude is called the first statics invariant.

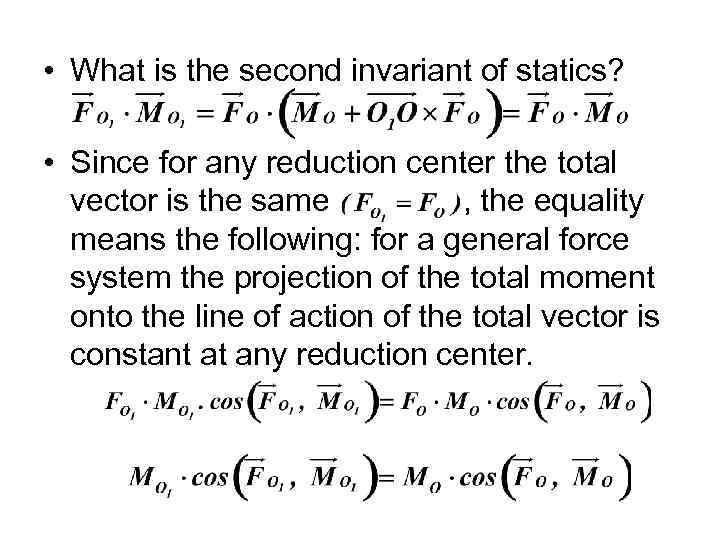• What is the second invariant of statics? • Since for any reduction center the total vector is the same , the equality means the following: for a general force system the projection of the total moment onto the line of action of the total vector is constant at any reduction center.

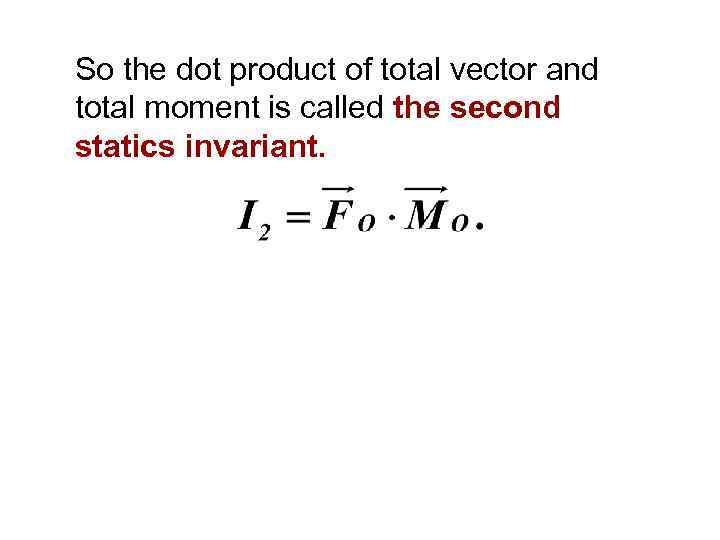So the dot product of total vector and total moment is called the second statics invariant.

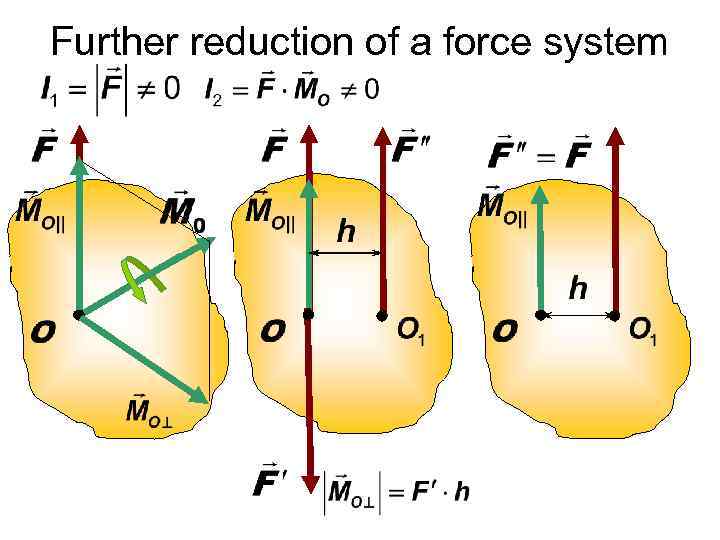Further reduction of a force system

WRENCH The set of a force and a couple with the couple moment parallel to the force is called wrench.

WRENCHES right and left

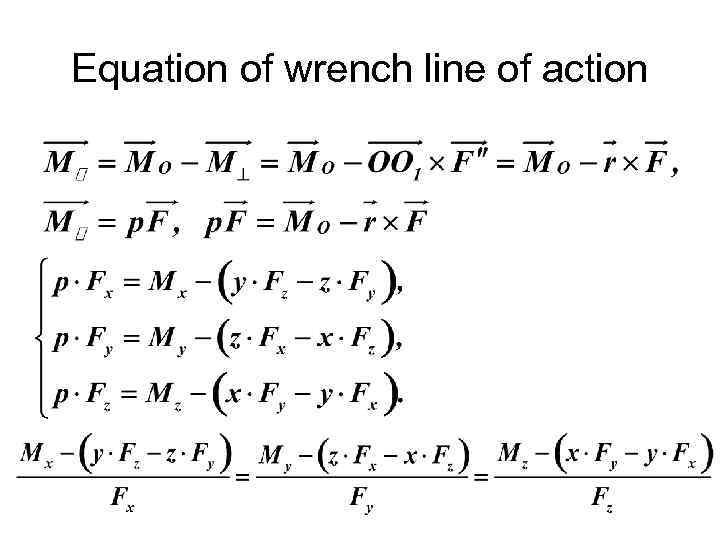Equation of wrench line of action

Equation of resultant line of action

Further reduction of a force system Case of force system reduction 1 2 0 0 0 =0 wrench 3 0 =0 =0 resultant at original center of reduction 4 5 =0 =0 =0 =0 couple resultant at new center of reduction balanced force system