e5b19c56fcfacc27da0b54e566a067a0.ppt
- Количество слайдов: 30

Lecture 6: beam optics in Linacs LINAC overview Acceleration Focussing Compression R. Bartolini, John Adams Institute, 3 May 2013 1/29

LINAC overview A LINAC is an accelerator consisting of several subsystems Gun (particle source) Accelerating section (and RF sources) Magnetic system (focussing and steering) Diagnostics – Vacuum – etc Depending on the application a LINAC might have bunch compression system (radiation sources, FELs, colliders) beam delivery systems (medical linacs, colliders) R. Bartolini, John Adams Institute, 3 May 2013 2/29

A 100 Me. V LINAC (at Diamond Light Source) R. Bartolini, John Adams Institute, 3 May 2013 3/29

Acceleration is achieved with RF cavities, using e. m. modes with the electric field pointing in the longitudinal direction (direction of motion of the charged particle) The RF electric field can be provided by travelling wave structure or standing wave structure Ez Ez c c c z z Travelling wave: the bunch sees a constant electric field Standing wave: the bunch sees a varying electric field Ez=E 0 cos( ) Ez=E 0 cos( t+ )sin(kz) R. Bartolini, John Adams Institute, 3 May 2013 4/29
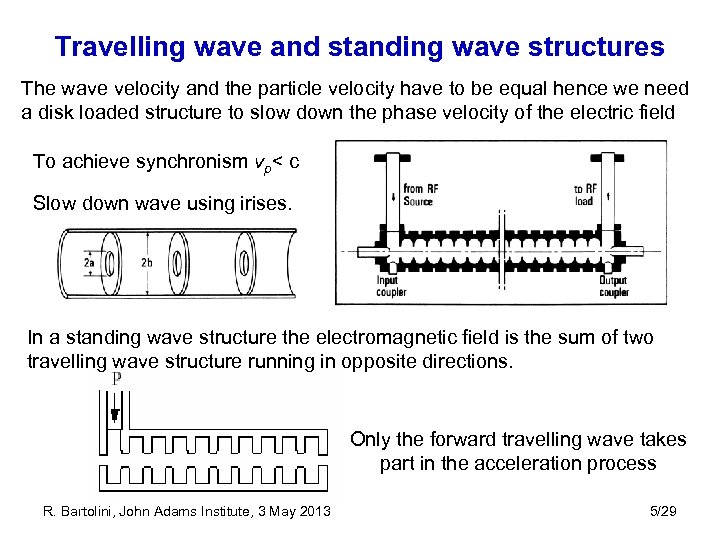
Travelling wave and standing wave structures The wave velocity and the particle velocity have to be equal hence we need a disk loaded structure to slow down the phase velocity of the electric field To achieve synchronism vp< c Slow down wave using irises. In a standing wave structure the electromagnetic field is the sum of two travelling wave structure running in opposite directions. Only the forward travelling wave takes part in the acceleration process R. Bartolini, John Adams Institute, 3 May 2013 5/29
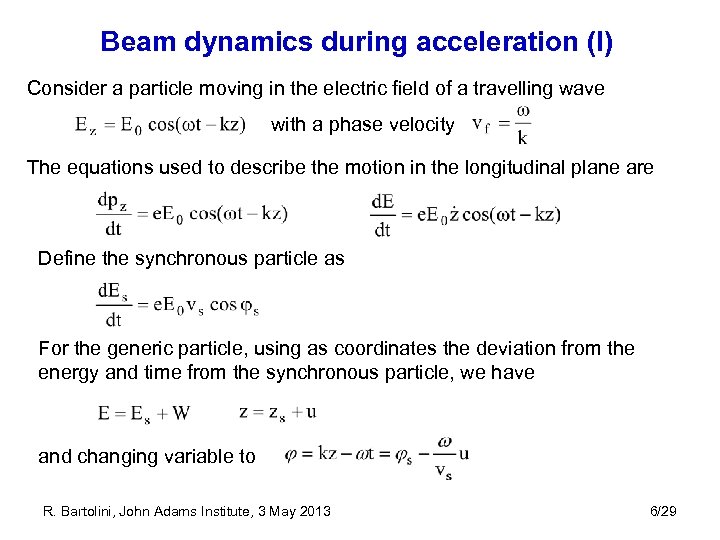
Beam dynamics during acceleration (I) Consider a particle moving in the electric field of a travelling wave with a phase velocity The equations used to describe the motion in the longitudinal plane are Define the synchronous particle as For the generic particle, using as coordinates the deviation from the energy and time from the synchronous particle, we have and changing variable to R. Bartolini, John Adams Institute, 3 May 2013 6/29
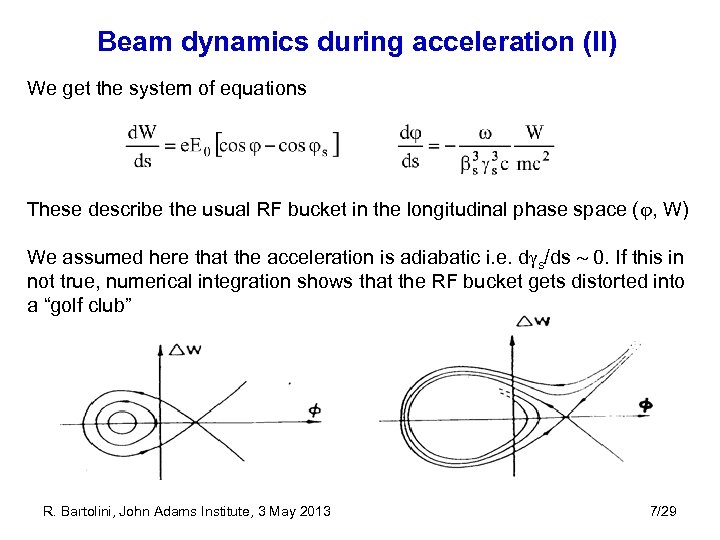
Beam dynamics during acceleration (II) We get the system of equations These describe the usual RF bucket in the longitudinal phase space ( , W) We assumed here that the acceleration is adiabatic i. e. d s/ds 0. If this in not true, numerical integration shows that the RF bucket gets distorted into a “golf club” R. Bartolini, John Adams Institute, 3 May 2013 7/29
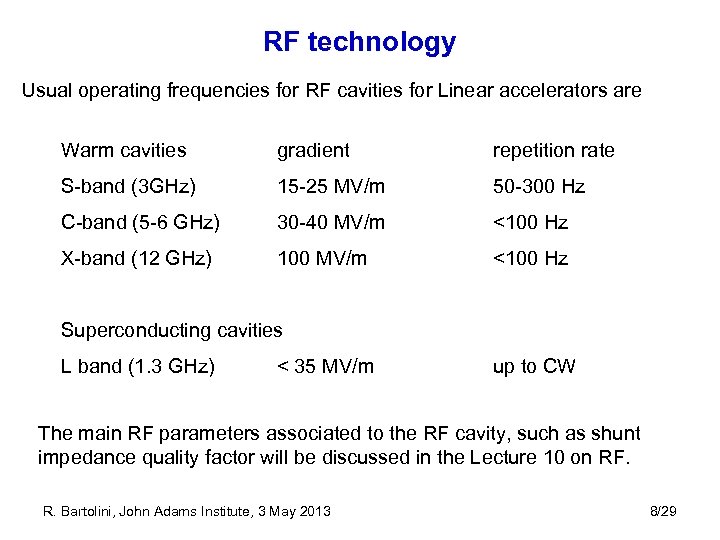
RF technology Usual operating frequencies for RF cavities for Linear accelerators are Warm cavities gradient repetition rate S-band (3 GHz) 15 -25 MV/m 50 -300 Hz C-band (5 -6 GHz) 30 -40 MV/m <100 Hz X-band (12 GHz) 100 MV/m <100 Hz Superconducting cavities L band (1. 3 GHz) < 35 MV/m up to CW The main RF parameters associated to the RF cavity, such as shunt impedance quality factor will be discussed in the Lecture 10 on RF. R. Bartolini, John Adams Institute, 3 May 2013 8/29

Particle sources and Gun Electrons Thermionic gun Photocathode guns Protons and Hplasma discharge Penning ion sources R. Bartolini, John Adams Institute, 3 May 2013 9/29

Thermionic gun (I) Electrons are generated by thermionic emission from the cathode and accelerated across a high voltage gap to the anode. A grid between anode and cathode can be pulsed to generate a train of pulses suitable for RF acceleration cathode assembly Ba. O/Ce. O-impregnated tungsten disc is heated and electrons are emitted R. Bartolini, John Adams Institute, 3 May 2013 10/29
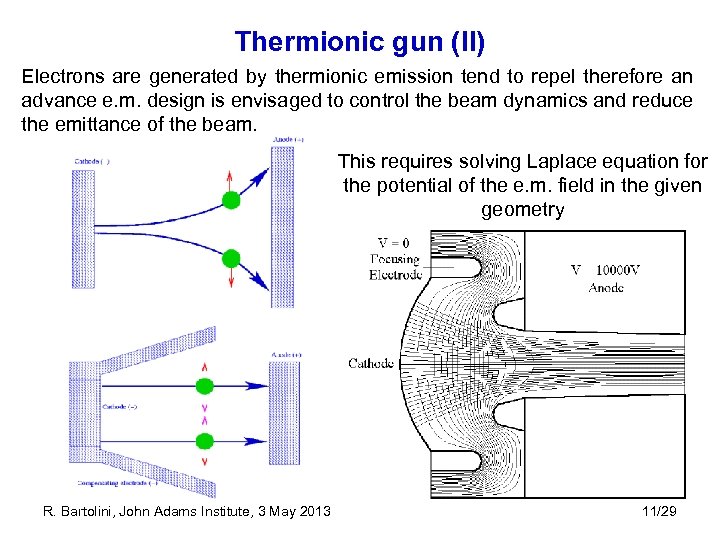
Thermionic gun (II) Electrons are generated by thermionic emission tend to repel therefore an advance e. m. design is envisaged to control the beam dynamics and reduce the emittance of the beam. This requires solving Laplace equation for the potential of the e. m. field in the given geometry R. Bartolini, John Adams Institute, 3 May 2013 11/29
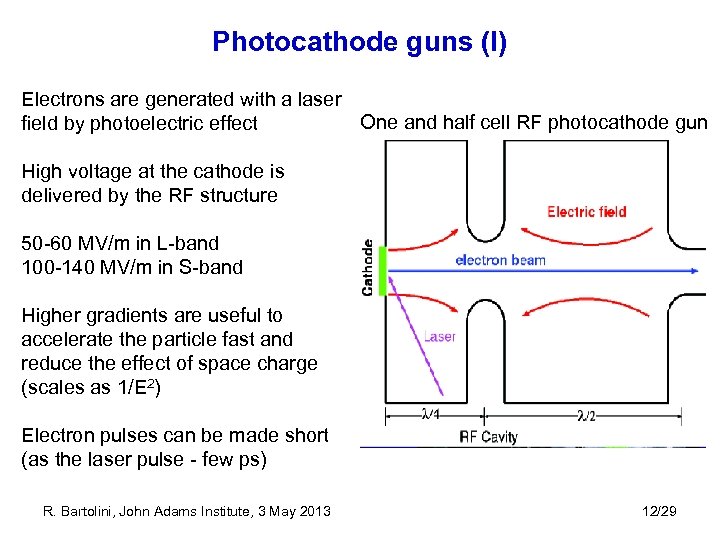
Photocathode guns (I) Electrons are generated with a laser One and half cell RF photocathode gun field by photoelectric effect High voltage at the cathode is delivered by the RF structure 50 -60 MV/m in L-band 100 -140 MV/m in S-band Higher gradients are useful to accelerate the particle fast and reduce the effect of space charge (scales as 1/E 2) Electron pulses can be made short (as the laser pulse - few ps) R. Bartolini, John Adams Institute, 3 May 2013 12/29
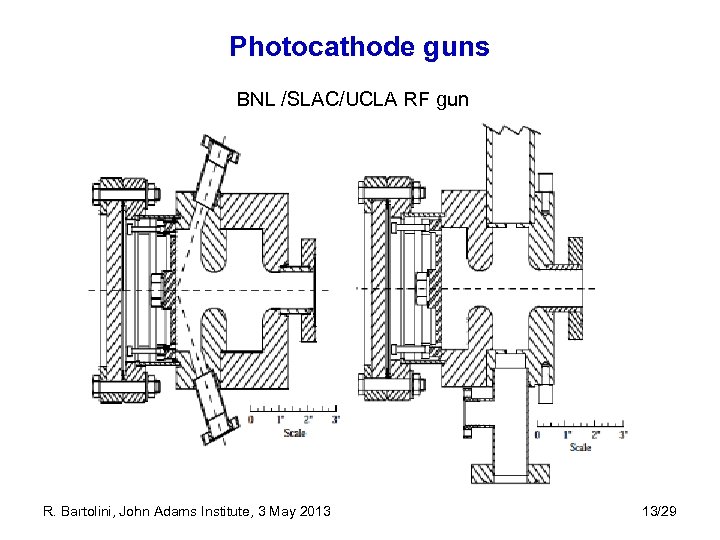
Photocathode guns BNL /SLAC/UCLA RF gun R. Bartolini, John Adams Institute, 3 May 2013 13/29
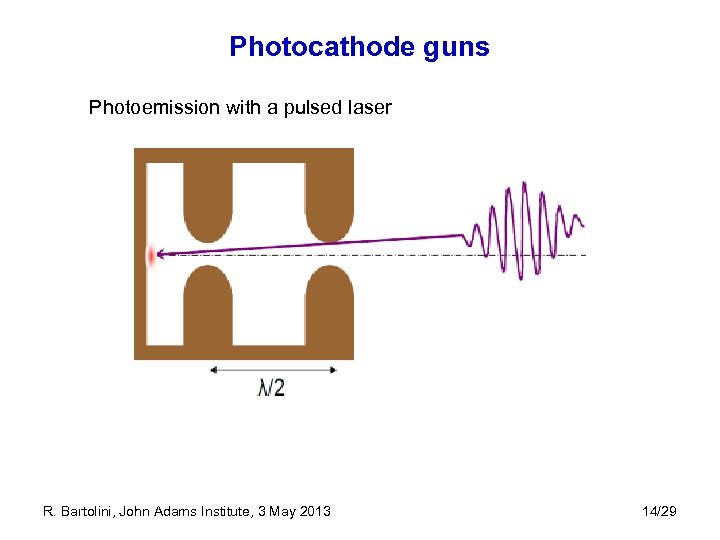
Photocathode guns Photoemission with a pulsed laser R. Bartolini, John Adams Institute, 3 May 2013 14/29
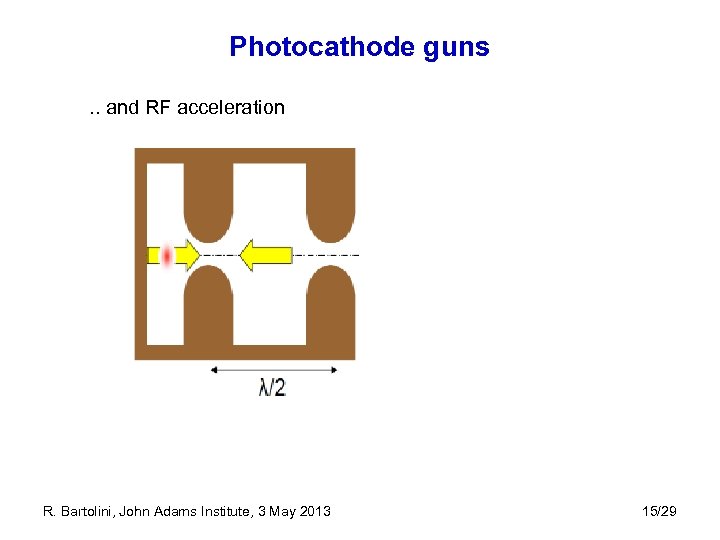
Photocathode guns. . and RF acceleration R. Bartolini, John Adams Institute, 3 May 2013 15/29

Photocathode guns. . and RF acceleration R. Bartolini, John Adams Institute, 3 May 2013 16/29

Photocathode guns. . and RF acceleration The emittance and the energy spread are determined by the laser parameters and the properties of the cathode material. The emittance can be tens of times better than in a thermionic guns (< 1 m) R. Bartolini, John Adams Institute, 3 May 2013 17/29
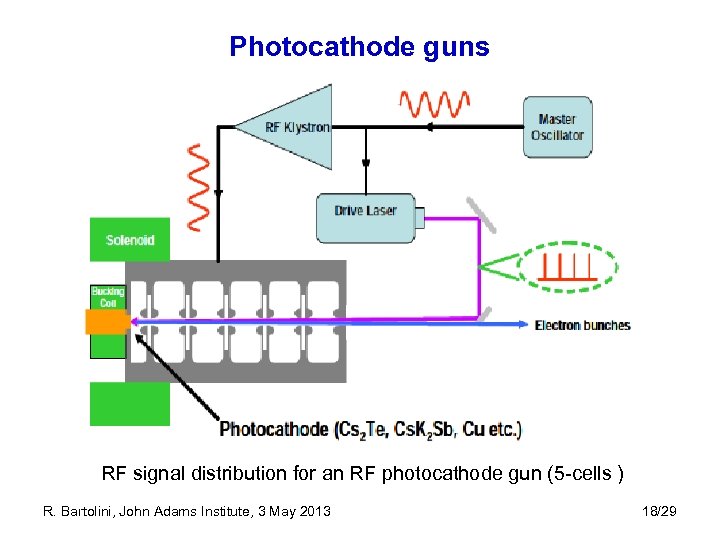
Photocathode guns RF signal distribution for an RF photocathode gun (5 -cells ) R. Bartolini, John Adams Institute, 3 May 2013 18/29
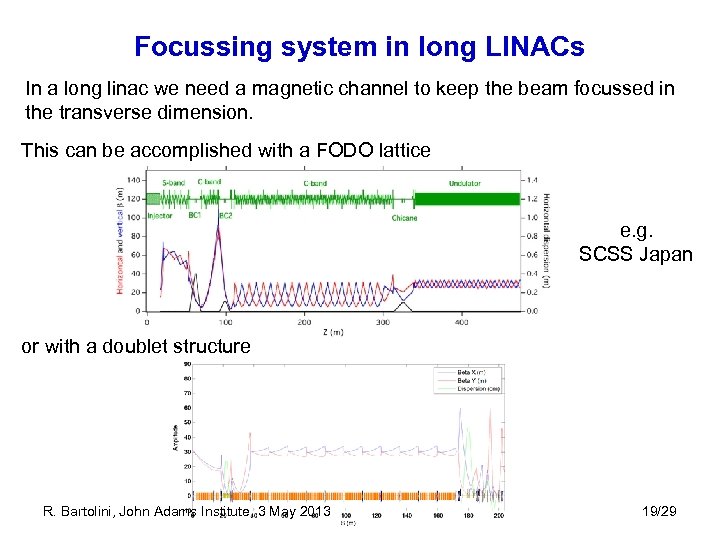
Focussing system in long LINACs In a long linac we need a magnetic channel to keep the beam focussed in the transverse dimension. This can be accomplished with a FODO lattice e. g. SCSS Japan or with a doublet structure R. Bartolini, John Adams Institute, 3 May 2013 19/29
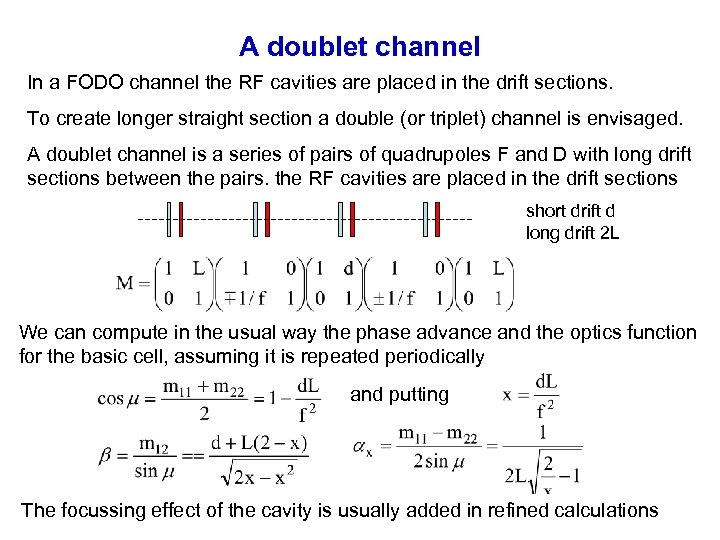
A doublet channel In a FODO channel the RF cavities are placed in the drift sections. To create longer straight section a double (or triplet) channel is envisaged. A doublet channel is a series of pairs of quadrupoles F and D with long drift sections between the pairs. the RF cavities are placed in the drift sections short drift d long drift 2 L We can compute in the usual way the phase advance and the optics function for the basic cell, assuming it is repeated periodically and putting 20/29 The focussing effect of the cavity is usually added in refined calculations
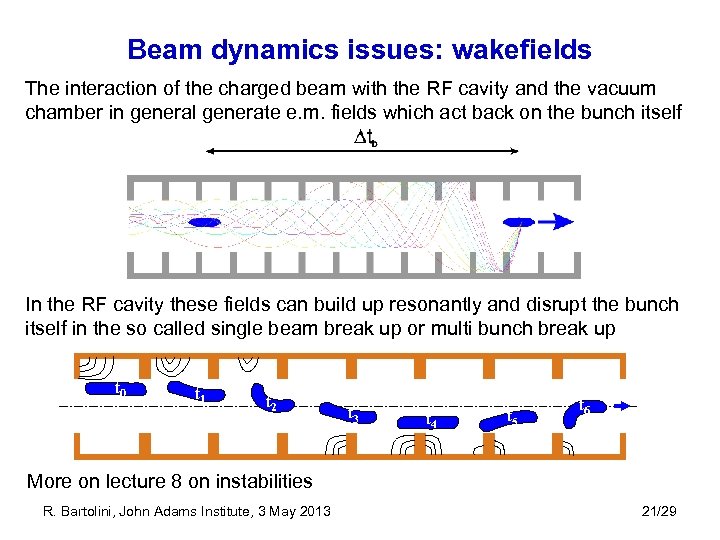
Beam dynamics issues: wakefields The interaction of the charged beam with the RF cavity and the vacuum chamber in general generate e. m. fields which act back on the bunch itself In the RF cavity these fields can build up resonantly and disrupt the bunch itself in the so called single beam break up or multi bunch break up t 0 t 1 t 2 t 3 t 4 t 5 t 6 More on lecture 8 on instabilities R. Bartolini, John Adams Institute, 3 May 2013 21/29
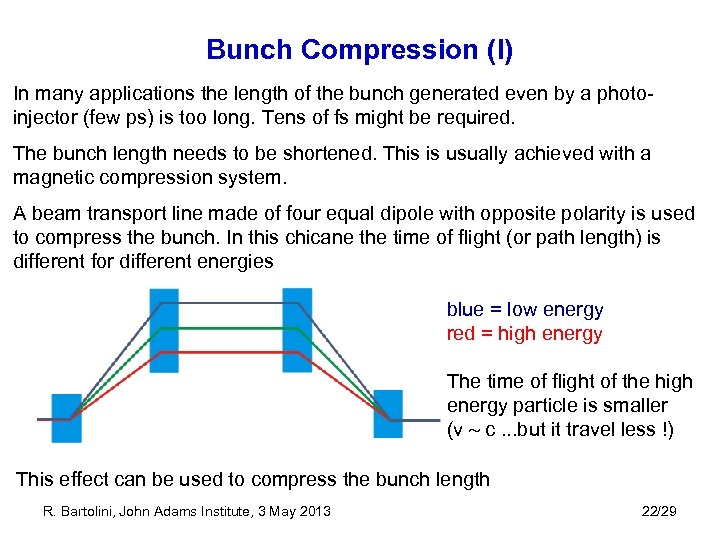
Bunch Compression (I) In many applications the length of the bunch generated even by a photoinjector (few ps) is too long. Tens of fs might be required. The bunch length needs to be shortened. This is usually achieved with a magnetic compression system. A beam transport line made of four equal dipole with opposite polarity is used to compress the bunch. In this chicane the time of flight (or path length) is different for different energies blue = low energy red = high energy The time of flight of the high energy particle is smaller (v c. . . but it travel less !) This effect can be used to compress the bunch length R. Bartolini, John Adams Institute, 3 May 2013 22/29
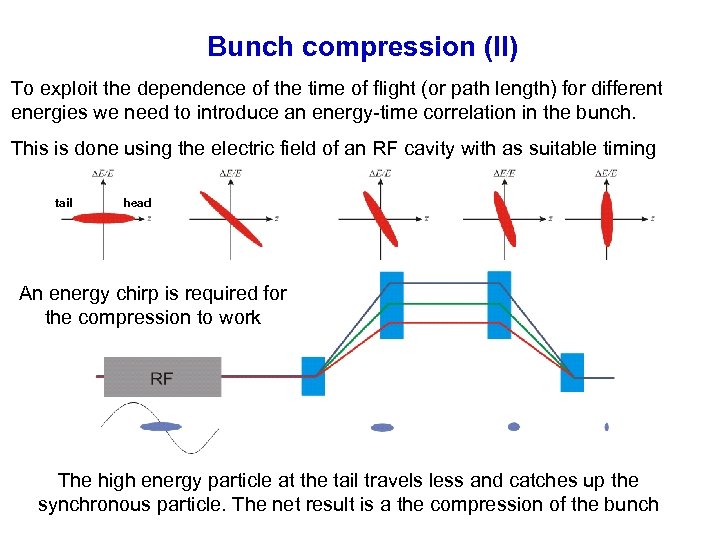
Bunch compression (II) To exploit the dependence of the time of flight (or path length) for different energies we need to introduce an energy-time correlation in the bunch. This is done using the electric field of an RF cavity with as suitable timing tail head An energy chirp is required for the compression to work The high energy particle at the tail travels less and catches up the 23/28 synchronous Adams Institute, 13 net result is a the compression of the bunch R. Bartolini, John particle. The May 2011 23/29
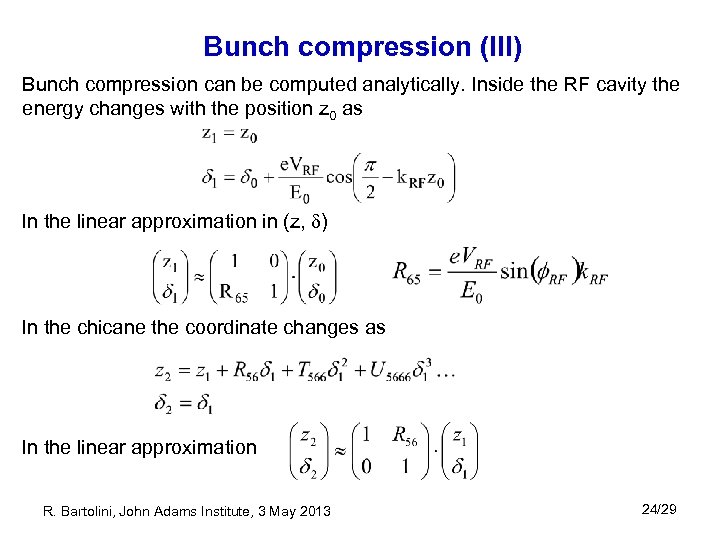
Bunch compression (III) Bunch compression can be computed analytically. Inside the RF cavity the energy changes with the position z 0 as In the linear approximation in (z, ) In the chicane the coordinate changes as In the linear approximation R. Bartolini, John Adams Institute, 3 May 2013 24/29

Bunch compression (IV) The full transformation is, as usual, the composition of the matrices of each, element and reads For a given value of R 65 (energy chirp induced), the best compression that can be achieved is C is the compression factor. It can be a large number! Since the transformation is symplectic (i. e. area preserving Liouville theorem) the longitudinal emittance is conserved The minimum reachable bunch length is limited to the product of the energy spread times R 56 R. Bartolini, John Adams Institute, 3 May 2013 25/29
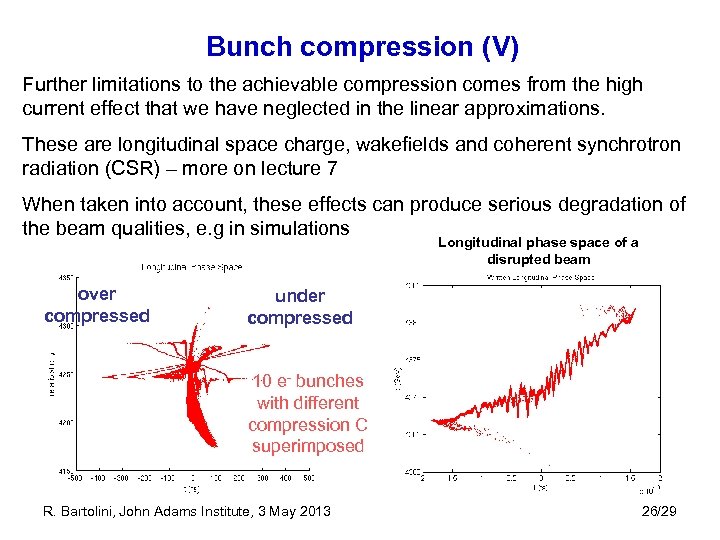
Bunch compression (V) Further limitations to the achievable compression comes from the high current effect that we have neglected in the linear approximations. These are longitudinal space charge, wakefields and coherent synchrotron radiation (CSR) – more on lecture 7 When taken into account, these effects can produce serious degradation of the beam qualities, e. g in simulations Longitudinal phase space of a disrupted beam over compressed under compressed 10 e- bunches with different compression C superimposed R. Bartolini, John Adams Institute, 3 May 2013 26/29
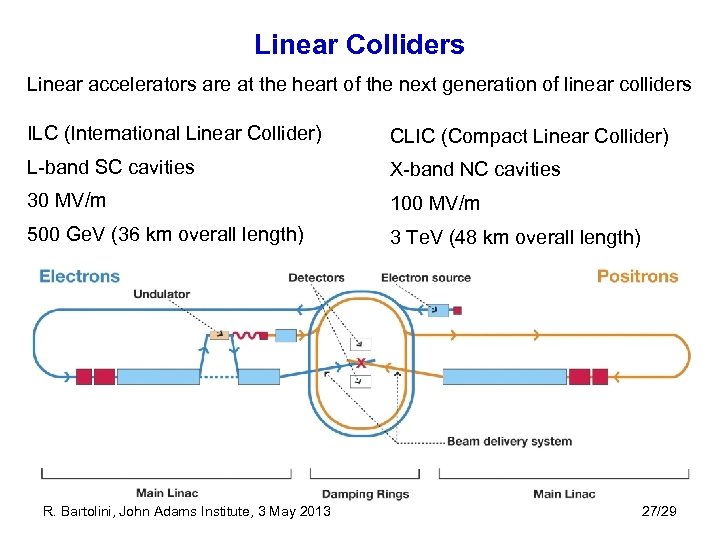
Linear Colliders Linear accelerators are at the heart of the next generation of linear colliders ILC (International Linear Collider) CLIC (Compact Linear Collider) L-band SC cavities X-band NC cavities 30 MV/m 100 MV/m 500 Ge. V (36 km overall length) 3 Te. V (48 km overall length) R. Bartolini, John Adams Institute, 3 May 2013 27/29
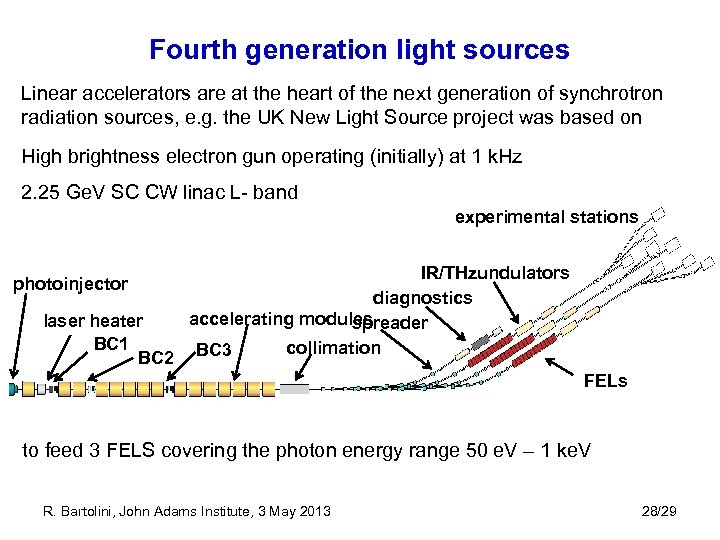
Fourth generation light sources Linear accelerators are at the heart of the next generation of synchrotron radiation sources, e. g. the UK New Light Source project was based on High brightness electron gun operating (initially) at 1 k. Hz 2. 25 Ge. V SC CW linac L- band experimental stations photoinjector laser heater BC 1 BC 2 IR/THzundulators diagnostics accelerating modules spreader collimation BC 3 FELs to feed 3 FELS covering the photon energy range 50 e. V – 1 ke. V R. Bartolini, John Adams Institute, 3 May 2013 28/29

Bibliography M. Conte, W. W. Mac. Kay, The physics of particle accelerators, World Scientific (1991) P. Lapostolle Theorie des Accelerateurs Lineaires, CERN 87 -10, (1987) J. Le Duff Dynamics and Acceleration in linear structures, CERN 85 -19, (1985) T. P. Wangler RF Linear Accelerators, Wiley, (2008) R. Bartolini, John Adams Institute, 3 May 2013 29/29

Syllabus and slides • • • • Lecture 1: Overview and history of Particle accelerators (EW) Lecture 2: Beam optics I (transverse) (EW) Lecture 3: Beam optics II (longitudinal) (EW) Lecture 4: Liouville's theorem and Emittance (RB) Lecture 5: Beam Optics and Imperfections (RB) Lecture 6: Beam Optics in linac (Compression) (RB) Lecture 7: Synchrotron radiation (RB) Lecture 8: Beam instabilities (RB) Lecture 9: Space charge (RB) Lecture 10: RF (ET) Lecture 11: Beam diagnostics (ET) Lecture 12: Accelerator Applications (Particle Physics) (ET) Visit of Diamond Light Source/ ISIS / (some hospital if possible) The slides of the lectures are available at http: //www. adams-institute. ac. uk/training Dr. Riccardo Bartolini (DWB room 622) r. bartolini 1@physics. ox. ac. uk
e5b19c56fcfacc27da0b54e566a067a0.ppt