LECTURE 23 24 Special Relativity At

LECTURE 23 & 24 : Special Relativity At the end of these lectures you should: • Understand “frame of reference” and “inertial frame of reference”. • Know that the velocity of light is invariant. • State Einstein’s postulates on which Special Relativity is based. • Understand “time dilation” and be able to perform simple calculations. • Understand “length contraction” and be able to perform simple calculations. • Understand Einstein’s equation E = mc 2 and be able to perform calculations using this equation. • Understand use relativistic equations for total energy and kinetic energy.

Space contraction Zoe measures the distance between the clocks d=v. TP According to Zoe, Adam and his two clocks are moving to our left at speed v Adam measures the distance between the clocks d. P=v. T

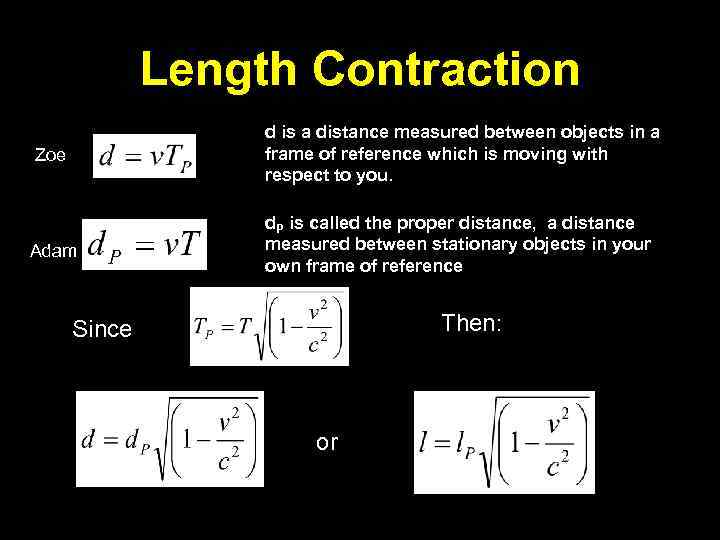Length Contraction Zoe d is a distance measured between objects in a frame of reference which is moving with respect to you. Adam d. P is called the proper distance, a distance measured between stationary objects in your own frame of reference Then: Since or

• If an observer measures a length on a moving object (with the length parallel to the direction of movement) then the length will be shorter than if the object is stationary with respect to the observer. Thus in our example Zoe measures a shorter length than Adam.

There is no unique inertial frame of reference • Zoe thinks that Adam is moving to our left, and Adam thinks that Zoe is moving to our right. • We have seen that Zoe will observe lengths (parallel to the motion) in Adam’s frame of reference to be reduced compared to Adam’s measurement. • Conversely Adam will observe lengths in Zoe’s frame of reference to be reduced compared with Zoe’s measurement.

Example 3 A spaceship of proper length 100 m passes close to a space station. According to an observer on the station the speed of the spaceship is 2. 5 x 108 ms 1. What is the length of the spaceship according to i) the pilot of the spaceship? ii) the observer on the space station?

Example 3 continued The observer on the space station continues to check the motion of the spaceship and finds that it travels at a constant velocity for 3 years and then turns round and returns at the same speed taking another 3 years to its original position, i. e. , the total journey time according to the space station observer is 6 years. According to the travellers on the spaceship, is the journey time greater than, equal to, or less than 6 years? Explain your answer.

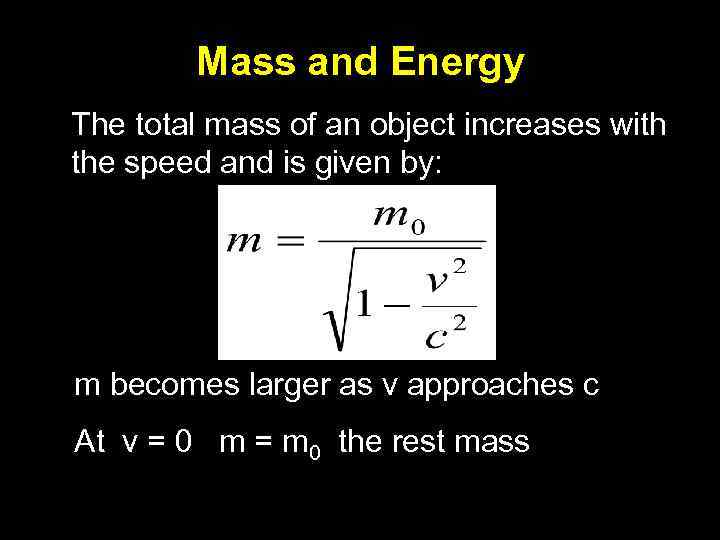Mass and Energy The total mass of an object increases with the speed and is given by: m becomes larger as v approaches c At v = 0 m = m 0 the rest mass

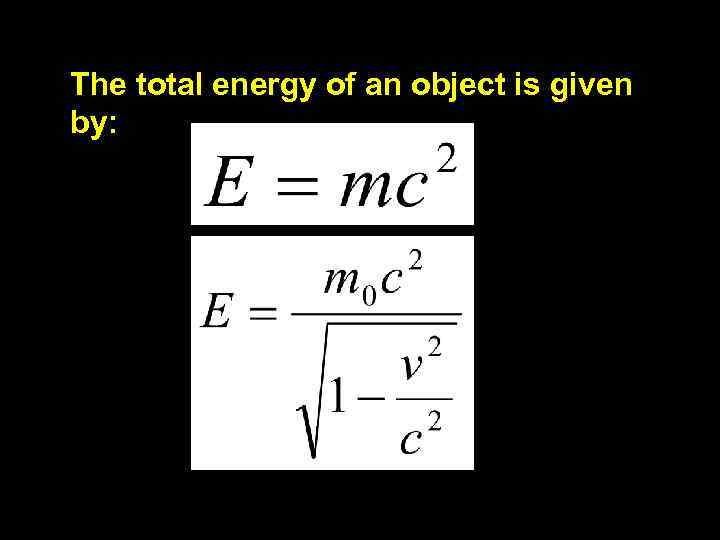The total energy of an object is given by:

The kinetic energy is the difference between the total energy and the rest mass energy. Check that when v is much less than c, this gives:

Example 4 The total energy of an electron is 2. 5 Me. V. Find its kinetic energy and its velocity. (The rest mass energy for an electron is 0. 511 Me. V)

Mass and energy are equivalent • Increase in kinetic energy means an increase in mass • Objects possess energy even when stationary - we can sometimes release this energy as kinetic energy – eg nuclear power, chemical explosions etc (How much energy is “stored” in a glass of water? )

LECTURE : CHECK LIST READING Adams and Allday: 8. 23, 3. 19, 8. 24, 8. 25 At the end of these lectures you should • Understand the concepts of frame of reference and inertial frame of reference. • Know that the velocity of light is invariant. • State Einstein’s postulates on which Special Relativity is based • Understand what is meant by time dilation and be able to perform simple calculations. • Understand what is meant by length contraction and be able to perform simple calculations. • Understand Einstein’s equation E = mc 2 and be able to perform calculations using this equation. • Understand use relativistic equations for total energy and kinetic energy.