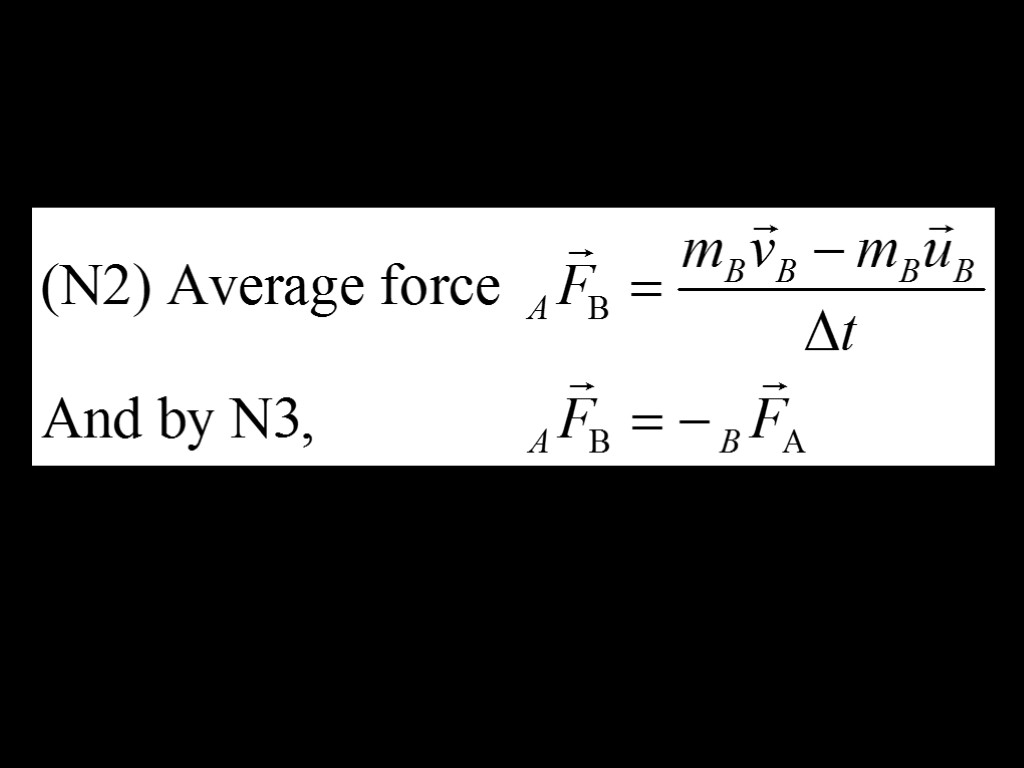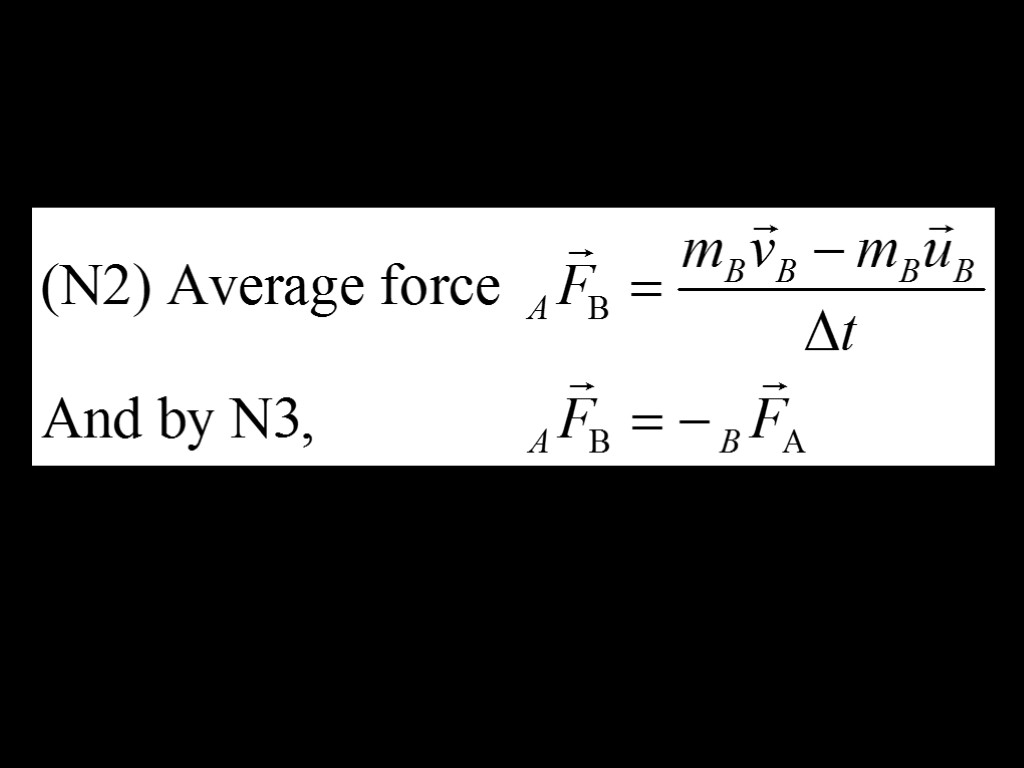Items of Note Test tomorrow on L 1

Items of Note Test tomorrow on L1-4, PSC 1-4, CW 1-2 Be there at 1.55 pm; you should know where! Please RESPECT the LECTURE time Students late for lectures are asked not to attend. Please remember Project groups meet this week. You should know when and where!

Forces and Linear Momentum At the end of this lecture you should: Be able to prove the conservation of linear momentum from Newton's Laws Perform calculations using the conservation law Know the difference elastic and inelastic collisions Understand what impulse is Be able to do calculations involving 'impulse equals the area under a force-time graph'

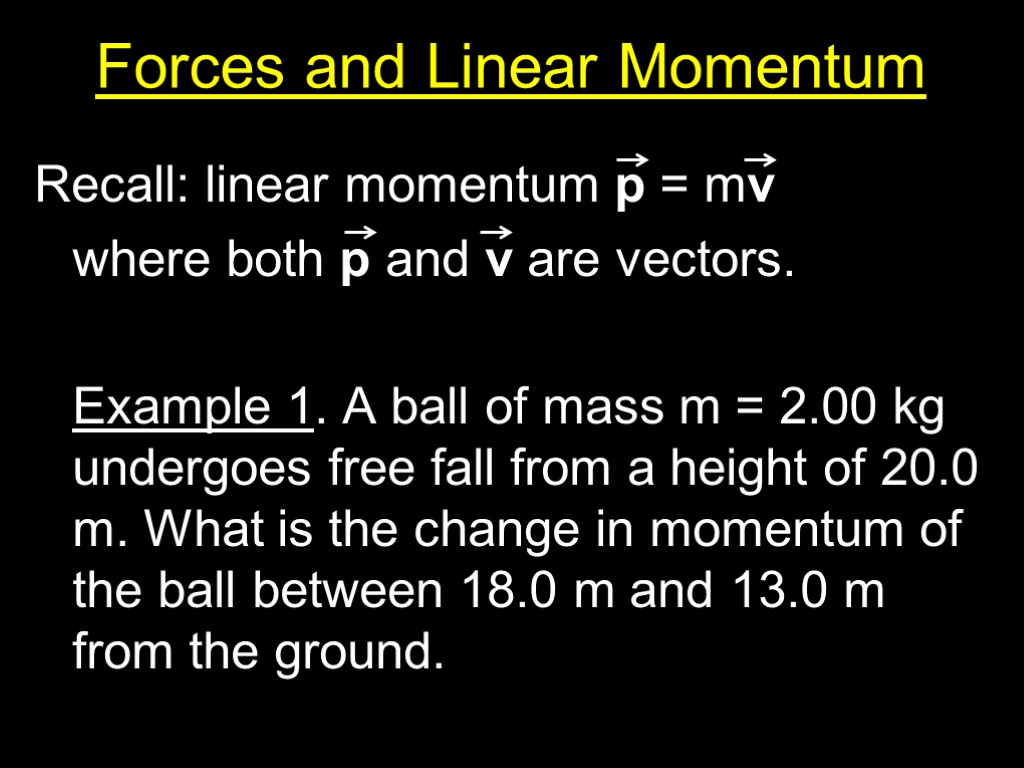Forces and Linear Momentum Recall: linear momentum p = mv where both p and v are vectors. Example 1. A ball of mass m = 2.00 kg undergoes free fall from a height of 20.0 m. What is the change in momentum of the ball between 18.0 m and 13.0 m from the ground.

Example 2: Using Newton’s 2nd and 3rd laws show that if no external forces act on a system of two interacting bodies, linear momentum is always conserved. Hint: Consider initial and final velocities.

Example 3: A truck of 2,000 kg travelling at 20 ms-1 smashes into the back of a car of 500 kg travelling at 10 ms-1 on an icy road. After the collision, they stick together and move with the same velocity, v. Find v. In solving this problem, why can we use our equation for conservation of momentum?

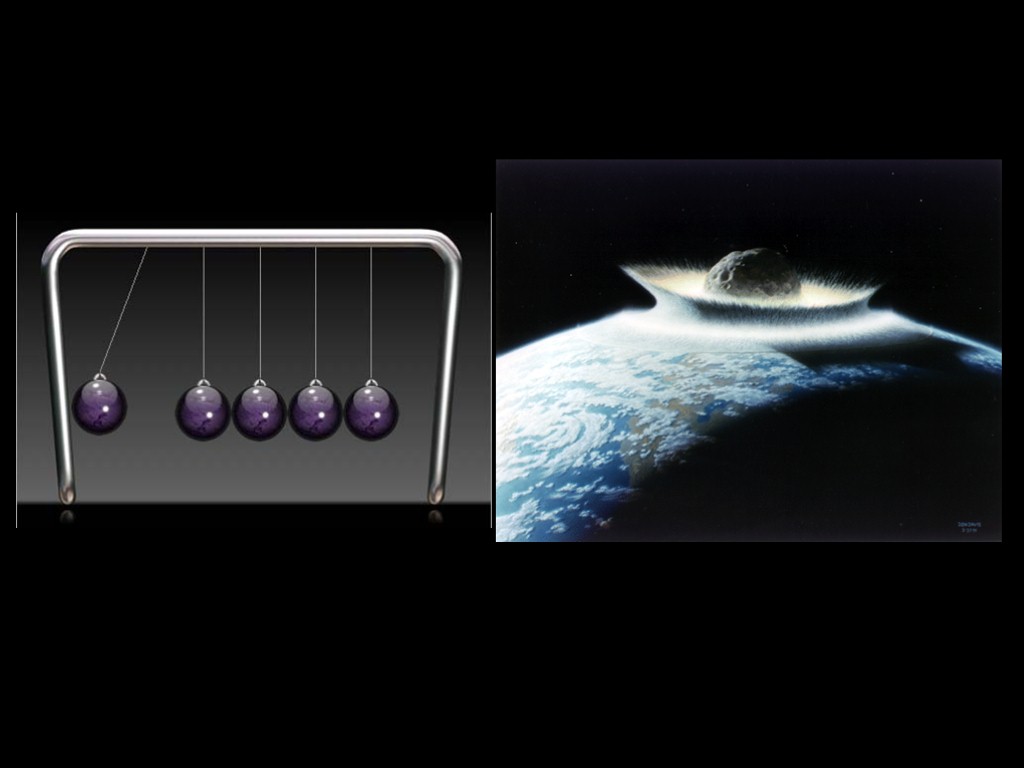
Collision types If the kinetic energy (KE) in a collision is conserved, the collision is elastic. If some KE is lost, the collision is inelastic. Where does the lost KE go?

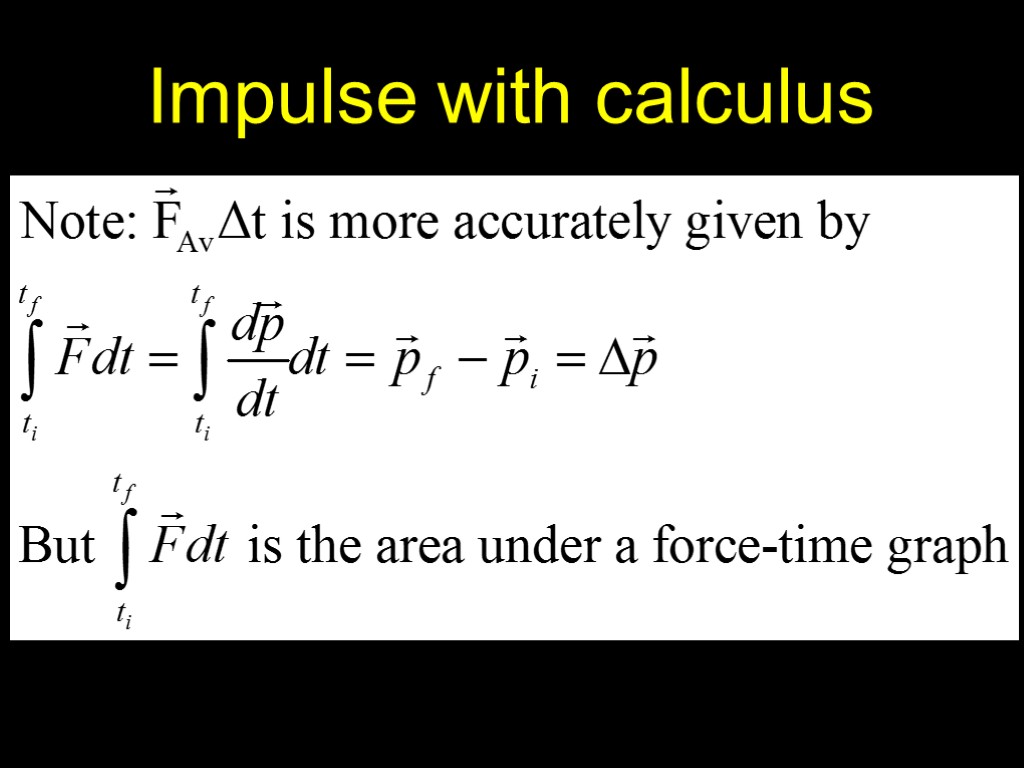Impulse with calculus

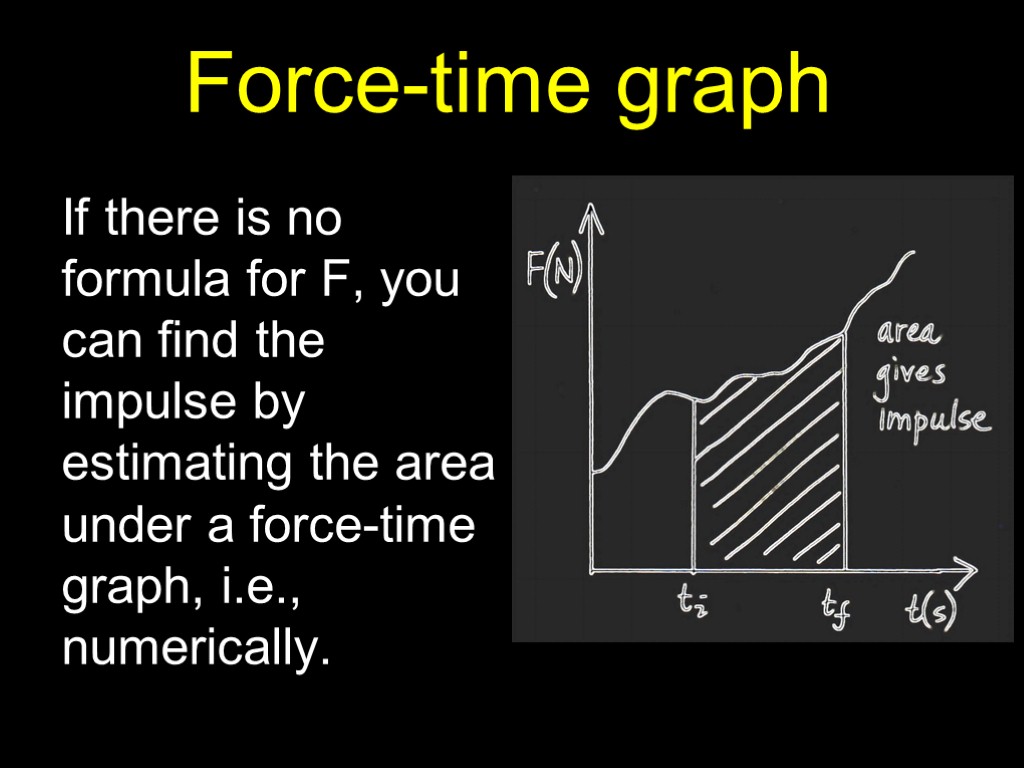Force-time graph If there is no formula for F, you can find the impulse by estimating the area under a force-time graph, i.e., numerically.

Example 4: A 0.15-kg rubber ball's velocity just before impact with a floor is 6.5 m/s down, and just after is 3.5 m/s straight up. If the ball is in contact with the floor for 0.025 s, what is the magnitude of the average force applied by the floor on the ball?

Example 5: A metal ball, of mass 20 g, traveling at 350 m/s, strikes a steel plate at an angle of 30 degrees with a plane of the plate. It ricochets off at the same angle, at a speed of 320 m/s. What is the magnitude of the impulse that the steel plate gives to the ball? (Ignore gravity)

CHECK LIST READING Adams and Allday: 3.24, 3.26 At the end of this lecture you should Be able to prove the conservation of linear momentum from Newton's Laws Perform calculations demonstrating your understanding of this conservation law Know the difference between an elastic and an inelastic collision Understand what is meant by impulse Be able to do calculations to show your understanding, including demonstration of the fact that 'impulse equals the area under a force-time graph'