Integration by parts • This is the





- Размер: 311.5 Кб
- Количество слайдов: 15
Описание презентации Integration by parts • This is the по слайдам
 Integration by parts • This is the name given to the process of integration which uses the differentiation product rule backwards
Integration by parts • This is the name given to the process of integration which uses the differentiation product rule backwards
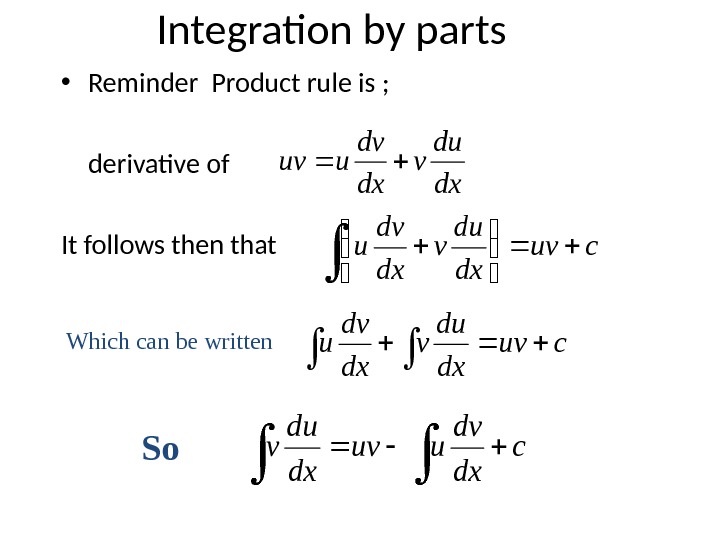
 Integration by parts • Reminder Product rule is ; derivative of It follows then thatdx du v dx dv uuv cuv dx du v dx dv u c dx dv uuv dx du v So cuv dx du v dx dv u Which can be written
Integration by parts • Reminder Product rule is ; derivative of It follows then thatdx du v dx dv uuv cuv dx du v dx dv u c dx dv uuv dx du v So cuv dx du v dx dv u Which can be written
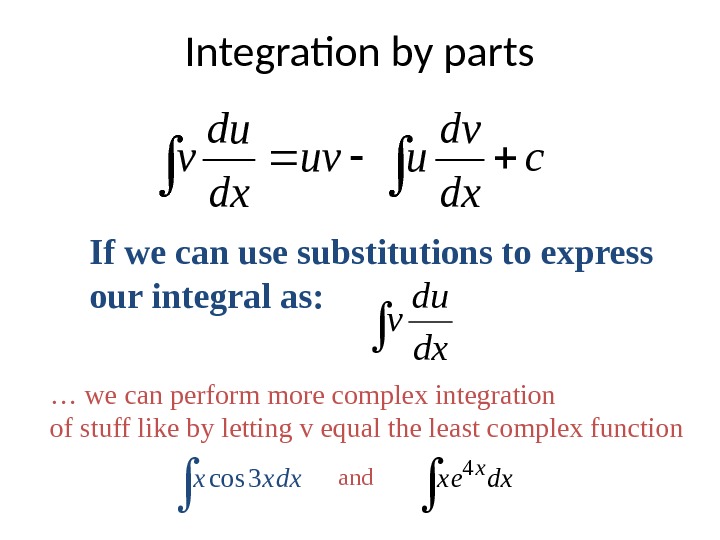
 If we can use substitutions to express our integral as: dx du v Integration by parts x dxx 3 cosdxx e x 4 c dx dv uuv dx du v … we can perform more complex integration of stuff like by letting v equal the least complex function and
If we can use substitutions to express our integral as: dx du v Integration by parts x dxx 3 cosdxx e x 4 c dx dv uuv dx du v … we can perform more complex integration of stuff like by letting v equal the least complex function and
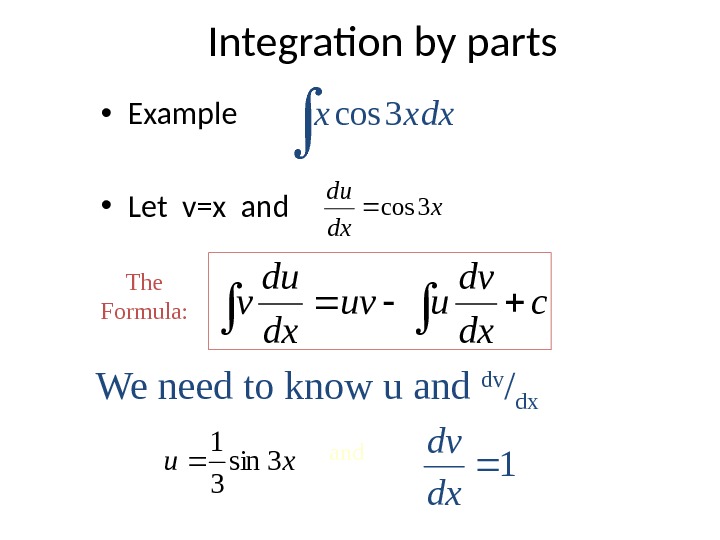
 Integration by parts • Example • Let v=x and x dxx 3 cos x dx du 3 cos xu 3 sin 3 1 c dx dv uuv dx du v We need to know u and dv / dx. The Formula: and 1 dx dv
Integration by parts • Example • Let v=x and x dxx 3 cos x dx du 3 cos xu 3 sin 3 1 c dx dv uuv dx du v We need to know u and dv / dx. The Formula: and 1 dx dv
 Integration by partsc dx dv uuv dx du v x dxxxxx 3 sin 3 1 3 cos cxxxxx 3 cos 9 1 3 sin 3 1 3 cos Substitute in Leads to 1 dx dv x dx du 3 cosxu 3 sin 3 1 v = x
Integration by partsc dx dv uuv dx du v x dxxxxx 3 sin 3 1 3 cos cxxxxx 3 cos 9 1 3 sin 3 1 3 cos Substitute in Leads to 1 dx dv x dx du 3 cosxu 3 sin 3 1 v = x
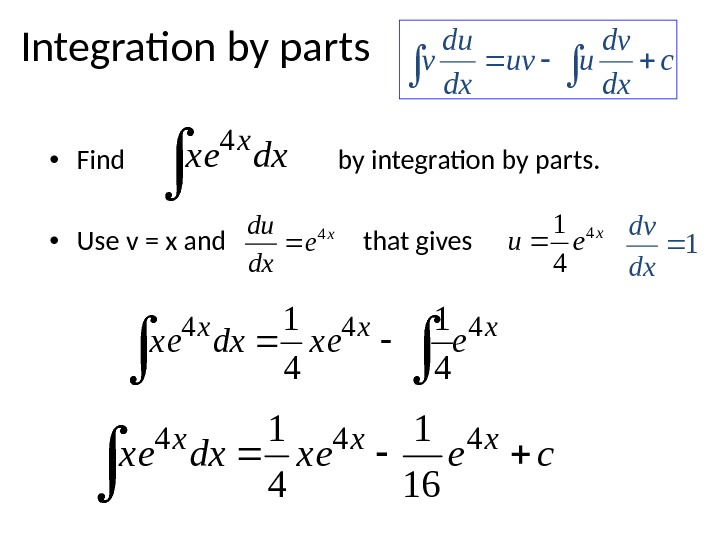
 Integration by parts • Find by integration by parts. • Use v = x and that gives dxx e x 4 x e dx du 4 x eu 4 4 1 xxx ex edxx e 444 4 1 cex edxx e xxx 444 16 1 4 1 1 dx dv c dx dv uuv dx du v
Integration by parts • Find by integration by parts. • Use v = x and that gives dxx e x 4 x e dx du 4 x eu 4 4 1 xxx ex edxx e 444 4 1 cex edxx e xxx 444 16 1 4 1 1 dx dv c dx dv uuv dx du v
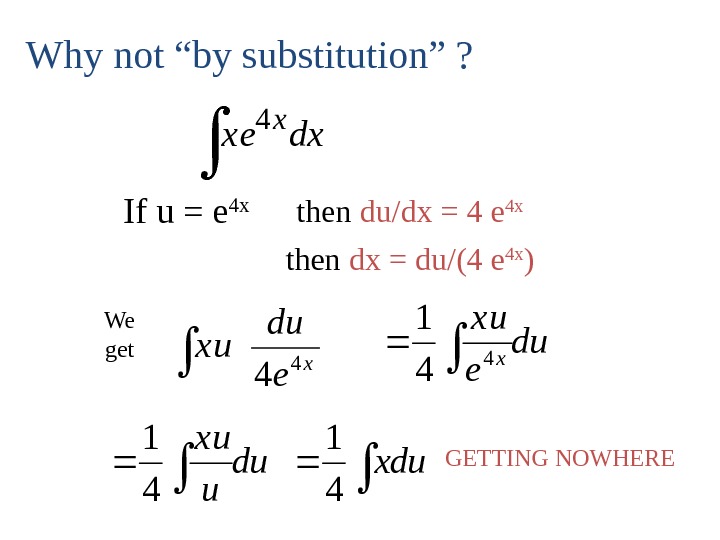
 dxx e x 4 Why not “by substitution” ? If u = e 4 x then du/dx = 4 e 4 x then dx = du/(4 e 4 x ) x e du x u 4 4 We get du e x u x 4 4 1 du u x u 4 1 dux 4 1 GETTING NOWHER
dxx e x 4 Why not “by substitution” ? If u = e 4 x then du/dx = 4 e 4 x then dx = du/(4 e 4 x ) x e du x u 4 4 We get du e x u x 4 4 1 du u x u 4 1 dux 4 1 GETTING NOWHER
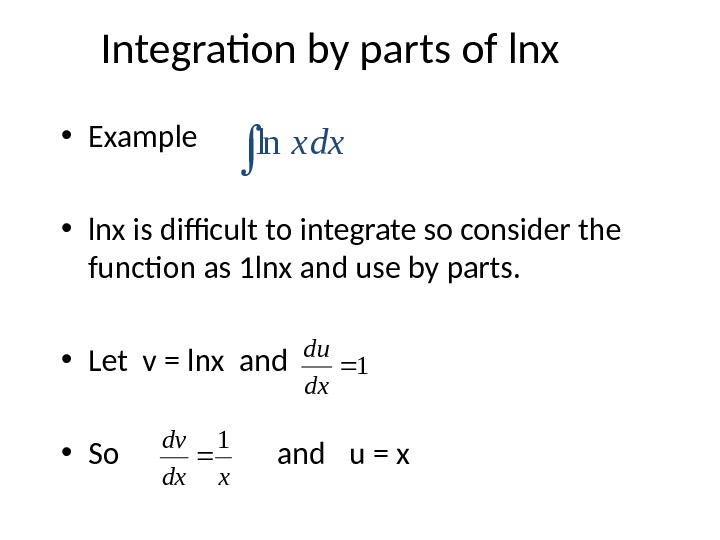
 Integration by parts of lnx • Example • lnx is difficult to integrate so consider the function as 1 lnx and use by parts. • Let v = lnx and • So and u = x x dxln 1 dx du xdx dv
Integration by parts of lnx • Example • lnx is difficult to integrate so consider the function as 1 lnx and use by parts. • Let v = lnx and • So and u = x x dxln 1 dx du xdx dv
 Integration by parts of lnxc dx dv uuv dx du v dx x xxxx 1 lnln cxxxx lnln Substitute in Leads to 1 dx du v = lnx xdx dv 1 u = x
Integration by parts of lnxc dx dv uuv dx du v dx x xxxx 1 lnln cxxxx lnln Substitute in Leads to 1 dx du v = lnx xdx dv 1 u = x
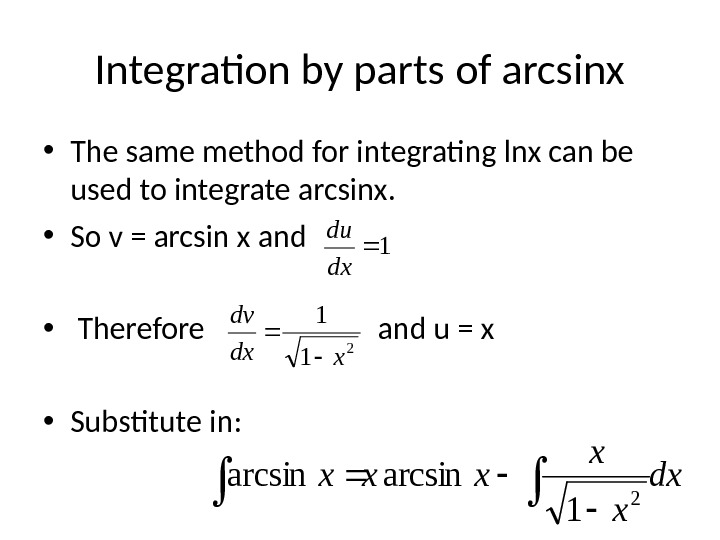
 • The same method for integrating lnx can be used to integrate arcsinx. • So v = arcsin x and • Therefore and u = x • Substitute in: Integration by parts of arcsinx 1 dx du 2 1 1 xdx dv dx x x xxx 2 1 arcsin
• The same method for integrating lnx can be used to integrate arcsinx. • So v = arcsin x and • Therefore and u = x • Substitute in: Integration by parts of arcsinx 1 dx du 2 1 1 xdx dv dx x x xxx 2 1 arcsin
 Integration by parts of arcsinx • Now use substitution to integrate • Let m = 1 – x 2 so dm = -2 xdx • So dx x x 2 1 dm mx dm m x dx x x 1 21 2 cxcm
Integration by parts of arcsinx • Now use substitution to integrate • Let m = 1 – x 2 so dm = -2 xdx • So dx x x 2 1 dm mx dm m x dx x x 1 21 2 cxcm
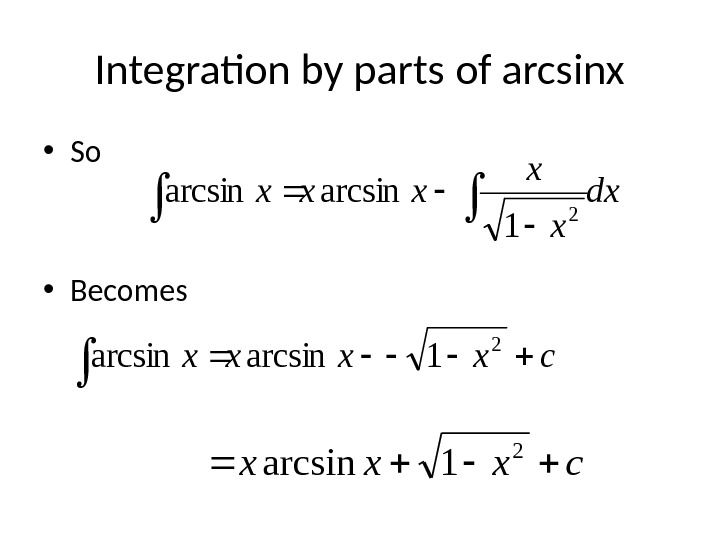
 Integration by parts of arcsinx • So • Becomes cxxx 2 1 arcsindx x x xxx 2 1 arcsin cxxxx 2 1 arcsin
Integration by parts of arcsinx • So • Becomes cxxx 2 1 arcsindx x x xxx 2 1 arcsin cxxxx 2 1 arcsin
 • This involves some algebraic manipulation since the second integral does not resolve into an easily integratable function. • Let v = ex and • Therefore and u = sinx • So: Integration by parts of e x cosx x dx du cos x e dx dv dxxexex dxe xxx sinsincos [1]
• This involves some algebraic manipulation since the second integral does not resolve into an easily integratable function. • Let v = ex and • Therefore and u = sinx • So: Integration by parts of e x cosx x dx du cos x e dx dv dxxexex dxe xxx sinsincos [1]
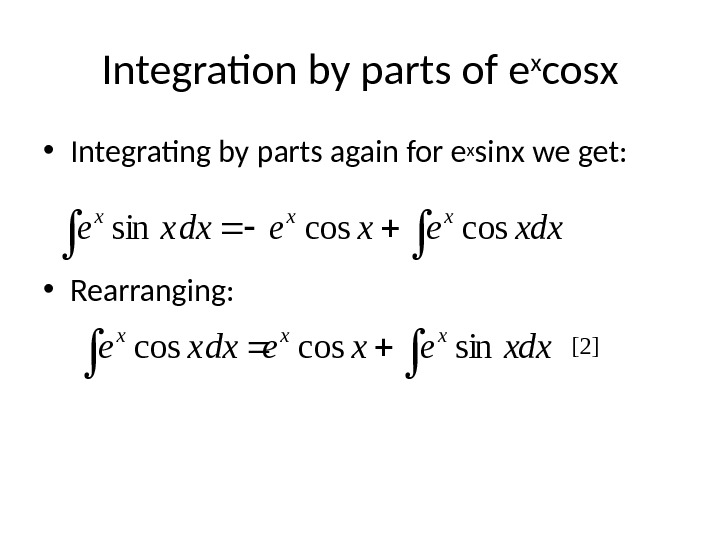
 • Integrating by parts again for ex sinx we get: • Rearranging: Integration by parts of e x cosx dxxexex dxe xxx coscossin dxxexex dxe xxx sincoscos [2]
• Integrating by parts again for ex sinx we get: • Rearranging: Integration by parts of e x cosx dxxexex dxe xxx coscossin dxxexex dxe xxx sincoscos [2]
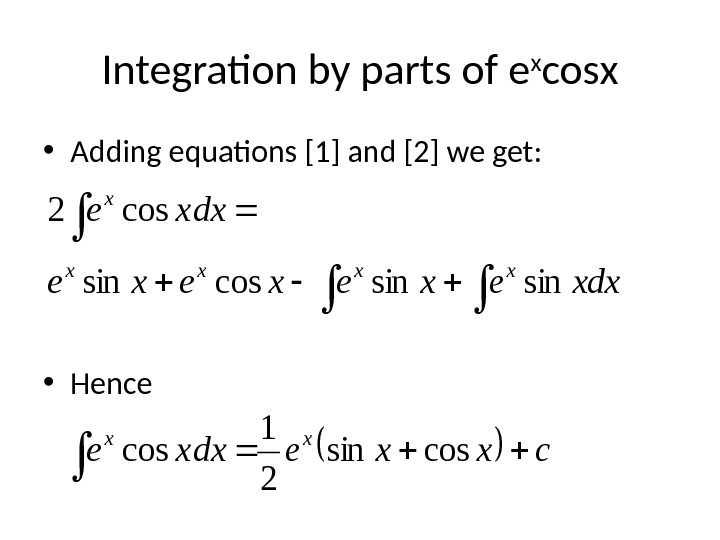
![• Adding equations [1] and [2] we get: • Hence Integration by parts of • Adding equations [1] and [2] we get: • Hence Integration by parts of](/docs//1_integration_by_parts_images/1_integration_by_parts_14.jpg) • Adding equations [1] and [2] we get: • Hence Integration by parts of e x cosxdxxexe x dxe xxxx x sinsincossin cos 2 cxxex dxe xx cossin 2 1 cos
• Adding equations [1] and [2] we get: • Hence Integration by parts of e x cosxdxxexe x dxe xxxx x sinsincossin cos 2 cxxex dxe xx cossin 2 1 cos

