Implied Dividend Growth Rate When we feel that

Implied Dividend Growth Rate When we feel that P 0 = V 0 Because the dividend growth rate affects the estimated value of a stock using the Gordon growth model, differences between estimated values of a stock and its actual market value might be explained by different growth rate assumptions. An analyst can then judge whether the implied dividend growth rate is reasonable, high, or low, based on what he or she knows about the company. In effect, the calculation of the implied dividend growth rate provides an alternative perspective on the valuation of the stock (fairly valued, overvalued, or undervalued).

Implied Dividend Growth Rate

Estimating the expected rate of return with the GGM When we feel that P 0 = V 0 Under the assumption of efficient prices, the Gordon growth model is frequently used to estimate a stock's expected rate of return given the stock's price and expected growth rate. When the Gordon growth model is solved for r, the expected rate of return is

Estimating the expected rate of return with the GGM

Present Value of Growth Opportunities Present value of growth opportunities is the part of a stock’s total value that comes from profitable future growth opportunities, in contrast to the stock’s value associated with assets already in place. • Earnings growth can occur under several scenarios, including when a company retains earnings (increasing its capital base) and earns a constant positive return on equity, even if that return is low. • Increases in shareholder wealth, however, occur only when reinvested earnings are directed to investments that earn more than the opportunity cost of the funds needed to undertake them (positive net present value projects). • Thus, investors actively assess whether and to what degree companies will have the opportunity to invest in profitable projects in the future. • In principle, companies without any positive NPV projects should distribute most or all of earnings to shareholders as dividends so the shareholders can redirect capital to more attractive areas.

Example: Assume that a company is expected to earn $20 mln per year in perpetuity if no investments are made. However, the firm will invest $2 mln next year in a project that will generate additional earnings of $360’ 000 in every subsequent year. The company has 10 mln shares outstanding. The firm’s cost of capital is 10%. What is the value of its stock on a per share basis if it undertakes the project? First, let’s identify the No-growth value of the stock No-Growth Value Now, let’s find what value to the firm is brought by the new project NPV of the project after year 1 NPV of the project after year 0 Or 0, 145 per share So the value of a company assuming that it undertakes this project is V 0 = No-growth value + PVGO = $20 + $0, 145 = $20, 15 per share In our case the return on the project is 18% per year. What would happen to the firm value if the project would have brought $180’ 000 per year instead of the $360’ 000?

In our case the return on the project is 18% per year. What would happen to the firm value if the project would have brought $180’ 000 per year instead of the $360’ 000? NPV of the project after year 1 NPV of the project after year 0 Or -0, 018 per share So the value of a company assuming that it undertakes this project is V 0 = No-growth value + PVGO = $20 - $0, 018 = $19, 982 = $19, 98 per share Why the value of the company has dropped although we have a project that generates cash flows?

In our case the return on the project is 18% per year. What would happen to the firm value if the project would have brought $180’ 000 per year instead of the $360’ 000? NPV of the project after year 1 NPV of the project after year 0 Or -0, 018 per share So the value of a company assuming that it undertakes this project is V 0 = No-growth value + PVGO = $20 - $0, 018 = $19, 982 = $19, 98 per share Why the value of the company has dropped although we have a project that generates cash flows? Because the rate of return on the project is lower than the required rate of return (9% VS 10%) -> thus this project has a negative PV -> it is better for the company not to initiate this project but rather pay the $2’ 000 in dividends in order to maximize the value of its shares.

Present Value of Growth Opportunities • If the stock price equals its IV, growth rate is sustained, the stock should sell at: • If all earnings paid out as dividends, price should be lower (assuming growth opportunities exist)

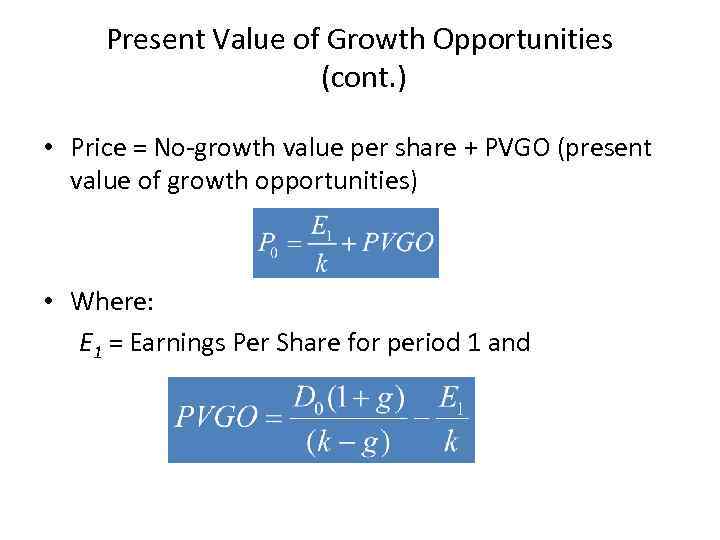Present Value of Growth Opportunities (cont. ) • Price = No-growth value per share + PVGO (present value of growth opportunities) • Where: E 1 = Earnings Per Share for period 1 and

For example, we can assess how much of value the market assign to the no-growth value of the CTWS and how much the market assigns to the possibility of the future growth of the CTWS: Remind that the company has current earnings of $1. 27, required rate of return of 6, 2% and the current price of $30 per share. We have: V 0 = E/r + PVGO => If we assume that Price=Value => 30 = 1, 27/0, 062 + PVGO => PVGO = 30 – 20, 48 = 9, 52 So, we may see that market assigns $20, 48 to the firm’s no-growth value And $9, 52 to the present value of the company’s future growth opportunities. As analysts, we may be interested in this assignment because the value of growth and the value in hand (no-growth value, based on existing assets) may have different risk characteristics. Whenever we calculate a stock’s value, V 0, whether using the Gordon growth or any other valuation model, we can calculate the value of growth, based on the value estimate, using the above equation.

Strengths and Weaknesses of GGM

GORDON GROWTH MODEL AND THE PRICE EARNINGS RATIO Using the GGM , we can develop an expression for P/E in terms of the fundamentals. This expression has two uses: • When used with forecasts of the inputs to the model, the analyst obtains a justified (fundamental) P/E – the P/E that is fair, warranted, or justified on the basis of fundamentals (given that the valuation model is appropriate). The analyst can then state his view of value in terms not of the GGM value but of the justified P/E. Because P/E is so widely recognized, this method can be an effective way to communicate the analysis. • The analyst may also use the expression for P/E to weigh whether the forecasts of earnings growth built into the current stock price are reasonable. What expected earnings growth rate is implied by the actual market P/E? Is that growth rate plausible? We can state the expression for P/E in terms of the current (trailing) P/E (today’s market price per share divided by trailing 12 months’ earnings per share) or in terms of the leading (or forward) P/E (today’s market price per share divided by a forecast of the next 12 months’ earnings per share, or sometimes next fiscal year’s earnings per share).

GORDON GROWTH MODEL AND THE PRICE EARNINGS RATIO Two definitions of P/E and GGM Trailing P/E: Is usually reported in newspapers Leading P/E: Is used when business fundamentals change and current earnings are no longer a good representative of future company performance where: P 0 = current price of a share E 0 = trailing (current-year) earnings E 1 = next year’s earnings b = retention rate, the fraction of earnings reinvested in the company rather than paid out in dividends (1 – b) = Dividend per share / Earnings per share = Dt / Et, is the dividend payout ratio r = required rate of return g = dividend growth rate

Trailing P/E: Leading P/E:

Life Cycles and Multistage Growth Models For many publicly traded companies practitioners assume growth falls into three stages: GROWTH PHASE. A company in its growth phase typically enjoys rapidly expanding markets, high profit margins, and an abnormally high growth rate in earnings per share (supernormal growth). Companies in this phase often have negative free cash flow to equity, because company invests heavily in expanding operations. Given high prospective returns on equity, the dividend payout ratios of growth-phase companies are often low, or even zero. As the company’s markets mature, or as unusual growth opportunities attract competitors, earnings growth rates eventually decline. TRANSITION PHASE. In this phase, which is a transition to maturity, earnings growth slows as competition puts pressure on prices and profit margins, or as sales growth slows because of market saturation. In this phase , earnings growth rates may be above average but declining towards the growth rate for the overall economy. Capital requirements typically decline in this phase, often resulting in positive free cash flow and increasing dividend payout ratios (or the initiation of dividends). MATURE PHASE. In maturity, the company reaches equilibrium in which investment opportunities on average just earn their opportunity cost of capital. Return on equity approaches the cost of equity, and earnings growth, the dividend payout ratio, and the return on equity stabilize at levels that can be sustained long term. We call the dividend and earnings growth rate of this phase the mature growth rate. This phase, in fact, reflects the stage in which a company can properly be valued using the GGM, and that model is one tool for valuing this phase of a currently high-growth company’s future.

Life Cycles and Multistage Growth Models A company may attempt to restart the growth phase by changing its strategic focuses and business mix. Technological advances may alter a company's growth prospects for better or worse with surprising rapidity. Nevertheless, this growth-phase picture of a company is a useful approximation.

Life Cycles and Multistage Growth Models Two-Stage DDM The two-stage DDM is based on the multiple-period model where we use Vn as an estimate of Pn. The two-stage model assumes that the first n dividends grow at an extraordinary short-term rate, gs: After time n, the annual dividend growth rate changes to a normal long-term rate, g. L. The dividend at time n + 1 is Dn+1 = Dn(1+ g. L) = D 0(1+g. S)(1+g. L). and this dividend continues to grow at g. L. Using Dn+1, we can use the Gordon growth model to find Vn: To find the value at t = 0, V 0, we simply find the present value of the first n dividends and the present value of the projected value at time n

Multistage Growth Rate Model: Example D 0 = $2. 00 g 1 = 20% g 2 = 5% k = 15% t = 3 D 1 = 2. 40 D 2 = 2. 88 D 3 = 3. 46 D 4 = 3. 63 V 0 = D 1/(1. 15) + D 2/(1. 15)2 + D 3/(1. 15)3 + D 4 / (1. 15)3(. 15 -. 05) V 0 = 2. 09 + 2. 18 + 2. 27 + 23. 86 = $30. 40 PV of dividends PV of expected price after 3 years

What is the value of the company’s share based on the two-stage GGM?

Table 2 -7 shows the calculations of the first five dividends and their present values discounted at 10. 7 percent. The terminal stock value at t = 5 is:

• In this two-stage model, we are forecasting the five individual dividends during the first stage and then calculating their present values. • We use the Gordon growth model to derive the terminal value (the value of the dividends in the second stage at the beginning of Stage 2). As shown above, the terminal value is V 5 = D 6/(r – g. L). • • The Period 6 dividend is $2, 002 (= D 5 × 1. 08 = $1, 854 × 1. 08). • Recalling our discussion of the sensitivity of the Gordon growth model to changes in the inputs, we might calculate an interval for the intrinsic value of GIS by varying the mature growth rate over the range of plausible values. The present value of the terminal value is $44. 60 = 74. 14/1. 1075. The total estimated value of GIS is $50. 14 using this model. Notice that almost 90 percent of this value, $44. 60, is the present value of V 5, and the balance, $50. 14 - $44. 60 = $5. 54, is the present value of the first five dividends.

Note, Companies that don’t pay dividends. If a company is not paying a dividend but is very profitable, an analyst might be willing to forecast its future dividends. Of course, for non-dividend-paying, unprofitable companies, such a forecast would be very difficult. Furthermore, as discussed in Section 2. 2 (Streams of Expected Cash Flows), it is usually difficult for the analyst to estimate the timing of the initiation of dividends and the dividend policy that will then be established by the company. Thus the analyst may prefer a free cash flow or residual income model for valuing such companies.

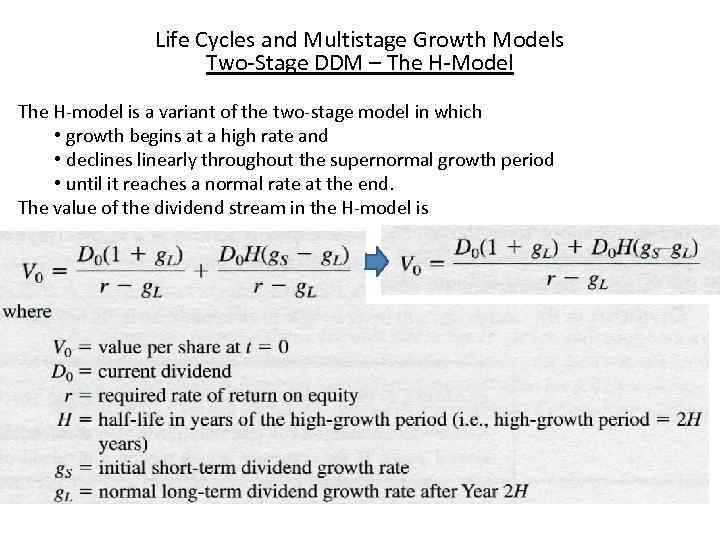Life Cycles and Multistage Growth Models Two-Stage DDM – The H-Model The H-model is a variant of the two-stage model in which • growth begins at a high rate and • declines linearly throughout the supernormal growth period • until it reaches a normal rate at the end. The value of the dividend stream in the H-model is

Find the value of the stock today using the H-model.

The H-model is an approximation model, which estimates the valuation that would result from discounting all of the future dividends individually. In many circumstances, this approximation is very close. • For a long extraordinary growth period (a high H) or • for a large difference in growth rates (the difference between gs and g. L), the analyst might abandon the approximation model for the more exact model. Fortunately, the many tedious calculations of the exact model are made fairly easy using a spreadsheet program.

Life Cycles and Multistage Growth Models Three-Stage DDMs EXAMPLE 2 -21. The Three-Stage D DM with Three Distinct Stages. IBM currently pays a dividend of $0. 55 per year. We estimate the current required rate of return at 12 percent. Assume we believe that dividends will grow at 7. 5 percent for the next two years, 13. 5 percent for the following four years, and 11. 25 percent into perpetuity. What is the current estimated value of IBM using a three-stage approach?

EXAMPLE 2 -22. The Three-Stage DDM with Declining Growth Rates in Stage 2. Elaine Bouvier is evaluating HRL (addressed earlier in Example 2 -2). She wishes to value HRL using the three-stage dividend growth model with a linearly declining dividend growth rate in Stage 2. After considerable study, Bouvier has decided to use the following information in her valuation (as of beginning of 2003): • The current dividend is $0. 39. • Bouvier estimates the required rate of retum on HRL stock at 8. 72 percent. • In Stage 1, the dividend will grow at 11. 3 percent annually for the next five years. • In Stage 2, which will last 10 years, the dividend growth rate will declinearly, starting at the Stage 1 rate and ending at the Stage 3 rate. • The equilibrium long-term dividend growth rate (in Stage 3) will be 5. 7 percent. Bouvier values HRL by computing the five dividends in Stage 1 and finding their present values at 8. 72 percent. The dividends in Stages 2 and 3 can be valued with the H-model, which estimates their value at the beginning of Stage 2. This value is then discounted back to find the dividends' present value at t = 0. Help Elaine to find the value of the stock.

Life Cycles and Multistage Growth Models Spreadsheet Modeling

STRENGTHS AND WEAKNESSES OF MULTISTAGE DDMs Strengths • The multistage DDMs can accommodate a variety of patterns of future streams of expected dividends. • Even though the multistage DDMs may use stylized assumptions about growth, they can provide useful approximations. • In addition to valuing dividend streams with a DDM, the expected rates of return can be imputed by finding the discount rate that equates the present value of the dividend stream to the current stock price. These expected return values can be adjusted to reflect expected market correction of mispricing. • Because of the variety of the DDMs available, the analyst is both enabled and compelled to carefully evaluate assumptions about the stock under examination. The valuation model should fit the assumptions (because the analyst is not forced to accept a set of assumptions that fit a specific model). • Spreadsheets are widely available, allowing the analyst to construct and examine an almost limitless number of models. • Using a model forces the analyst to specify assumptions, rather than simply using subjective assessments. Analysts can thus use common assumptions, understand the reasons for differing valuations when they occur, and react to changing market conditions in a systematic manner.

STRENGTHS AND WEAKNESSES OF MULTISTAGE DDMs Weaknesses • Garbage in, garbage out. If the inputs are not economically meaningful and appropriate for the company being valued, the outputs for the model will not be useful. • Analysts sometimes employ models that they don’t understand fully. For example, the H -model is an approximation model. An analyst may think it is exact and misuse it. • As a sensitivity analysis usually shows, valuations are very sensitive to models’ inputs. Programming and data errors in spreadsheet models are very common. Spreadsheet models should be checked thoroughly.