Implementing CAS into Teaching Learning and Assessment An

Implementing CAS into Teaching, Learning and Assessment: An Australian Experience Peter Flynn The University of Melbourne, Australia flynnpj@unimelb. edu. au

Computer Algebra Systems. Curriculum, Assessment and Teaching Project (CAS-CAT) (2000 -2002) • Explore the effect of CAS on teaching/learning/assessment • Research partners including TI • Presented at many teacher and research conferences and published many papers • http: //extranet. edfac. unimelb. edu. au /DSME/CAS-CAT

Why CAS? • Make students better users of • • mathematics Closer link between ‘real’ and school mathematics Achieve deeper learning by students Promote a less procedural view of mathematics Introduce new topics into the curriculum

Mathematics in Victoria • Victorian Certificate Education (2 years) • State-wide examinations • 3 mathematics subjects • Mathematical Methods (MM) • ‘Middle subject’ in terms of difficulty • Coordinate Geometry, Functions, Calculus, Algebra and Probability

CAS-CAT Project 2000 -2002 • Started with 3 schools (N=78) • School A: TI-89 • School B: CASIO FX 2. 0 • School C: HP 40 G • 2 CAS-permitted examinations • 80% common questions between Mathematical Methods and Mathematical Methods (CAS)

2003 -2005 Extended Pilot • 2003: ~ 13 schools offering Mathematical Methods (CAS) • 2005: ~ 40 schools • 2003 -: Various hand-held or computer-based CAS permitted • 2006 -: Calculator free and calculator permitted examination

CAS-CAT Project Findings • Effect on Teaching • Effect on Student learning • Effect on Examination Assessment

Effect on Teaching • Took longer than expected to learn CAS • Used CAS as an ‘add-on’ initially but with • • experience and confidence, CAS became a greater part of teaching Provided more solution methods for doing mathematics Used more for learning mathematics Promoted greater dialogue between teacher and students Create changes in teaching philosophy

By-Head/By-Hand/By-CAS • Balance is important • One teacher: – simple cases (eg derivative of sin(x)) completed by-head/by-hand – recognise when CAS was required – decisions made on efficiency and accuracy • Some students were worried that CAS would ‘steal’ their skills • Some students became too CAS-dependent

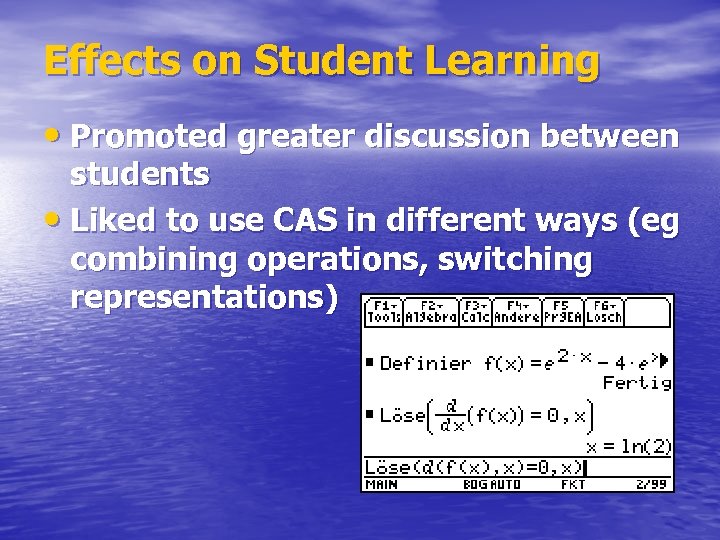Effects on Student Learning • Promoted greater discussion between students • Liked to use CAS in different ways (eg combining operations, switching representations)

Promoting Functional Thought • Students became more comfortable working with functions

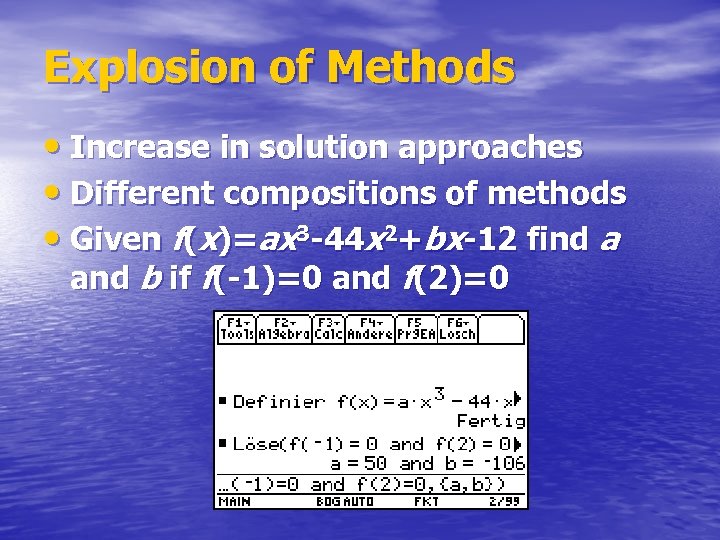Explosion of Methods • Increase in solution approaches • Different compositions of methods • Given f(x)=ax 3 -44 x 2+bx-12 find a and b if f(-1)=0 and f(2)=0

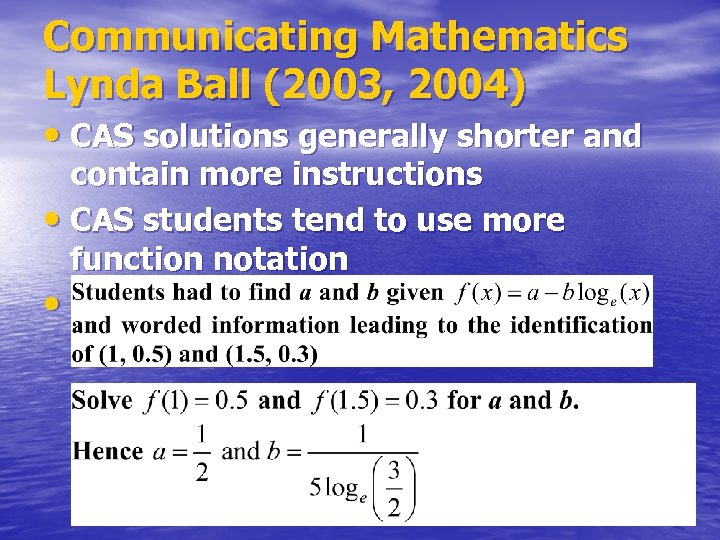Communicating Mathematics Lynda Ball (2003, 2004) • CAS solutions generally shorter and contain more instructions • CAS students tend to use more function notation •

Algebraic Knowledge • Teachers maintained and valued basic by-hand algebra skills • Algebraic knowledge improved but not more than normal • Algebraic knowledge required to use CAS was underestimated initially • Entering expressions correctly and recognising equivalent forms • Given more attention by teachers.

You have learned about factorising quadratics and cubics. What about quartics, polynomials of degree of 4?

We haven’t learned about that but they would have 4 factors.

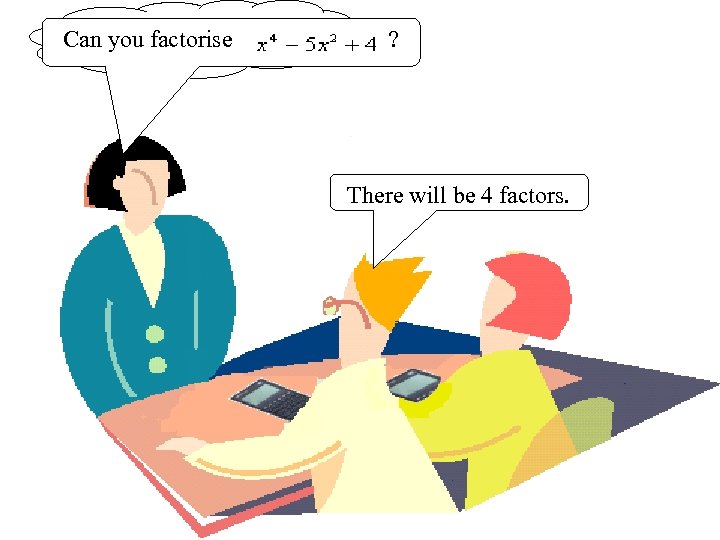Can you factorise ? There will be 4 factors.

That’s what I expected. There are 4 factors.

Oh wow! How come I got that?

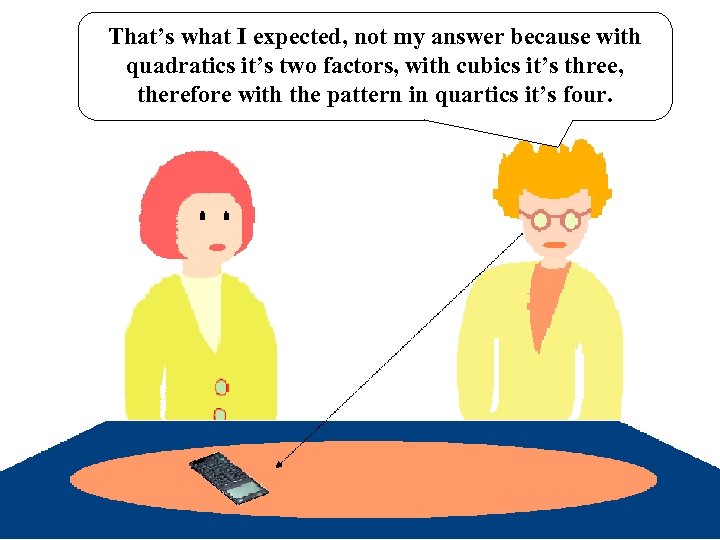That’s what I expected, not my answer because with quadratics it’s two factors, with cubics it’s three, therefore with the pattern in quartics it’s four.

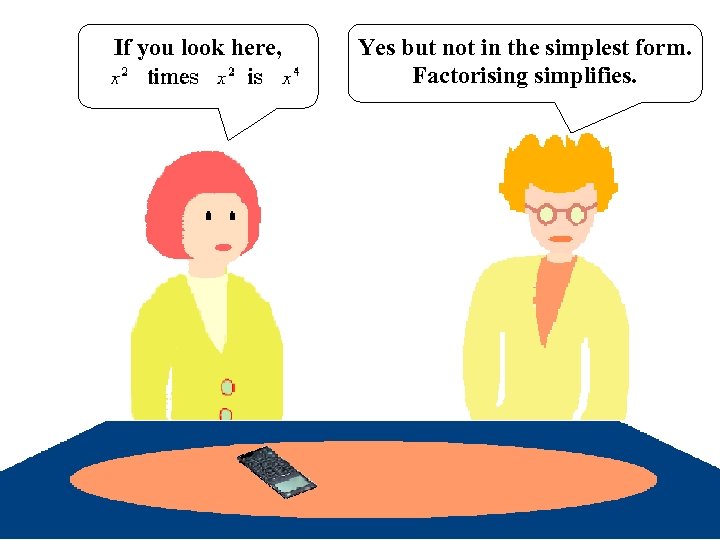If you look here, Yes but not in the simplest form. Factorising simplifies.

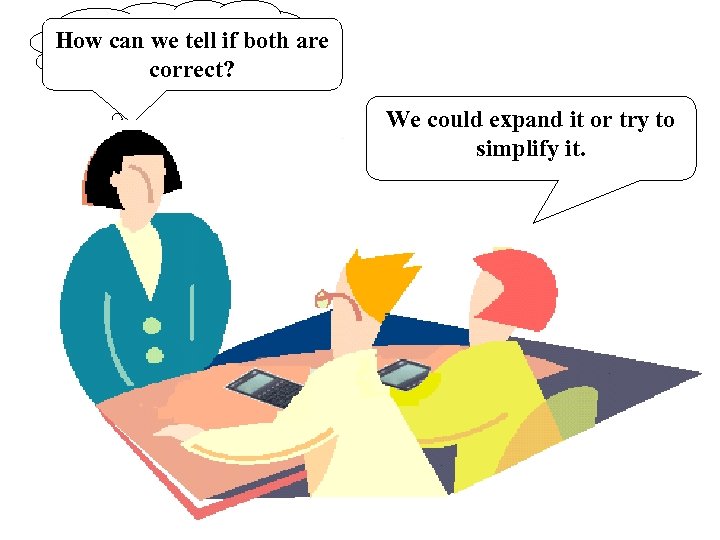Are both answers How can we tell if both are correct? We could expand it or try to simplify it.

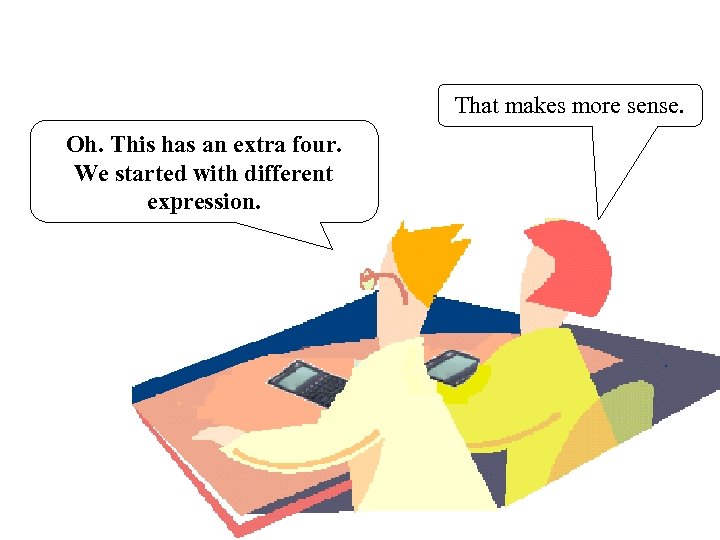That makes more sense. Oh. This has an extra four. We started with different expression.

Equivalence of Form • CAS outputs was a critical issue • Students need algebraic knowledge of a different flavour • Students with a more well-developed by-hand/mental algebra seemed to adapt to CAS more readily

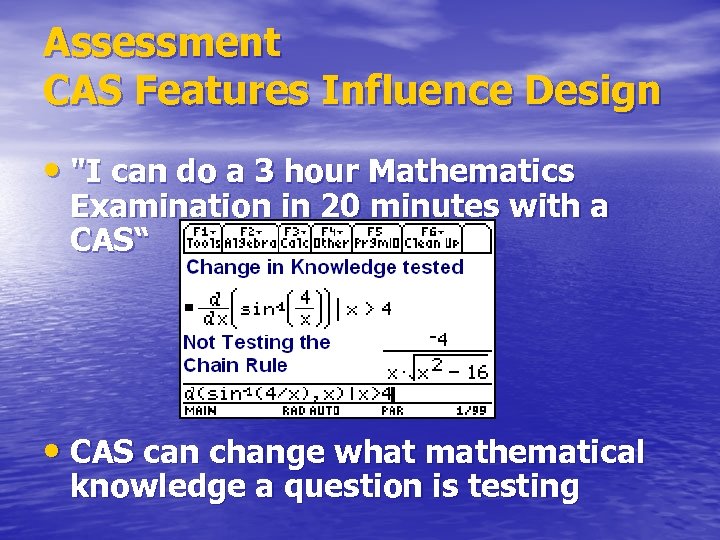Assessment CAS Features Influence Design • "I can do a 3 hour Mathematics Examination in 20 minutes with a CAS“ • CAS can change what mathematical knowledge a question is testing

Examination Assessment • If CAS is permitted in examinations, • • • assessment needs to change Reluctance to construct questions that really require the symbolic power of CAS Examiners tended to set questions that only require numerical/graphical A common response was to set questions with parameters

Student Performance in CAS Examinations • Questions thought as 'trivialised' may not be so. 69% of CAS students correctly identified the linear factors of x 4+x 3 -3 x 2 -3 x

Some General Conclusions • Increasing number of schools • General positive attitude towards CAS • Time required to learn effective CAS use • Students can be puzzled by CAS outputs • Students (and teachers) require good • • • algebraic knowledge to work with CAS effectively Assessment will take time to evolve CAS can cause major change to teaching methods and beliefs Teachers must be well-supported

The University of Melbourne’s CAS-CAT Research Project website http: //extranet. edfac. unimelb. edu. au /DSME/CAS-CAT Danke Schön