GCE Mathematics Support Event Tuesday 22 nd November

GCE Mathematics Support Event Tuesday 22 nd November 2016 Corr’s Corner Belfast Tuesday November 29 th 2016 Glenavon Hotel Cookstown

AGENDA 0900 – 0915 Registration 0915 – 0940 Welcome 0940 – 1000 Introduction from Subject Officer 1000 – 1015 Break – tea/coffee 1015 – 1100 Reports on C 1, C 2, M 1, S 1 1110 – 1200 Reports on C 3, C 4, (M 2) 1200 – 1215 Plenary/Questions 1215 – 1300 Lunch 1300 – 1315 – 1500 – 1530 Introduction to GCE Further Mathematics Reports on F 1, M 3, M 4, F 2, F 3, S 4 Plenary/Questions

CCEA website

GCE Mathematics page

Mathematics microsite

GCE Mathematics • Current specification will be assessed for the last time in 2018 • The current AS will still be available for teaching in 2017 for one year (resits unavailable for AS after 2018) • GCE A 2 in 2017, 2018, resits 2019

GCE Mathematics • Examiner/student support Events • Topic Tracker • Past Papers/mark schemes/tips on web Centre support • •

GCE Mathematics • On line marking from summer 2016 C 1, C 2, C 3, C 4, S 1 & M 1 • Statistical analysis available • Not for Further Maths New question/ Answer booklet for 2017 • •

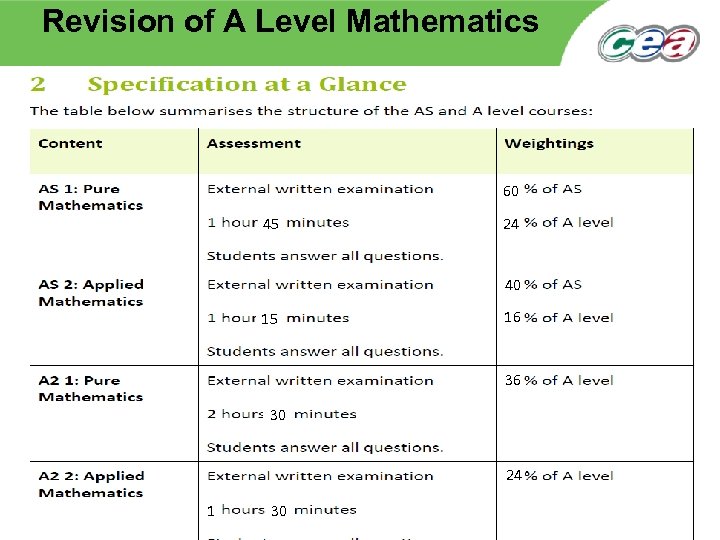
Revision of A Level Mathematics 60 45 24 40 15 16 36 30 24 1 30

Revision of A Level Mathematics • Specification written to meet the recommendations of the A Level Content Advisory Board (ALCAB) • Content based on current proposals for English Boards • Modular structure retained • Portability and comparability assured • Retention of AS/A 2 structure • AS award contributes to 40% of the overall A level • AS 180 guided learning hours (GLH) A 2 360 GLH total • No option choices • One resit opportunity per unit allowed • Exams in the summer series only

Revision of A Level Mathematics • 60% Pure Maths and 40% Applied Maths weighting across both AS and A 2 levels • Applied Maths weightings 50% Statistics, 50% Mechanics • AS papers Pure 100 marks 1 hr 45 min Applied 70 marks 1 hr 15 min • A 2 papers Pure 150 marks 2 hr 30 min Applied 100 marks 1 hr 30 min • Candidates answer all questions

Revision of Further Mathematics • Further Mathematics content has been revised in line with the A level Mathematics content • 50% of Pure Mathematics is prescribed – ensuring consistency but retaining flexibility for students to specialise • Modular structure retained • Portability and comparability assured • Retention of AS/A 2 structure • AS award contributes to 50% of the overall A level • AS 180 guided learning hours (GLH) A 2 360 GLH total • 4 option choices in Applied Mathematics

Revision of Further Mathematics • 50% Pure Maths and 50% Applied Maths weighting across both AS and A 2 levels • Applied Maths 4 options (a) Mechanics 1 (b) Mechanics 2 (c) Statistics (d) Discrete & Decision Mathematics • Candidates answer all questions from 2 options • AS papers Pure 100 marks 1 hr 30 min Applied 100 marks 1 hr 30 min • A 2 papers Pure 150 marks 2 hr 15 min Applied 150 marks 2 hr 15 min • One resit opportunity per unit allowed • Exams in the summer series only

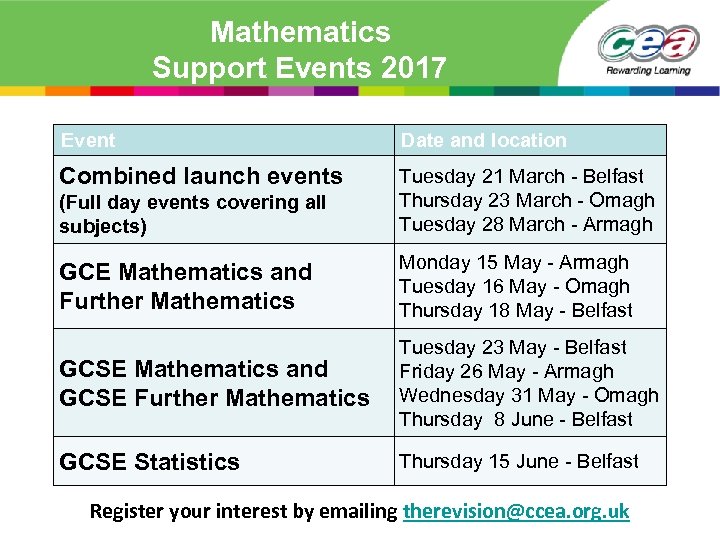
Mathematics Support Events 2017 Event Date and location Combined launch events (Full day events covering all subjects) Tuesday 21 March - Belfast Thursday 23 March - Omagh Tuesday 28 March - Armagh GCE Mathematics and Further Mathematics Monday 15 May - Armagh Tuesday 16 May - Omagh Thursday 18 May - Belfast GCSE Mathematics and GCSE Further Mathematics Tuesday 23 May - Belfast Friday 26 May - Armagh Wednesday 31 May - Omagh Thursday 8 June - Belfast GCSE Statistics Thursday 15 June - Belfast Register your interest by emailing therevision@ccea. org. uk

Join The Re. Vision mailing list to keep up to date on the latest news, subject specification updates and notifications of events. Sign up at www. ccea. org. uk/therevision

Information Day Summer Series 2016 Eleanor Smylie Chair of Examiners

General Comments C 1 & C 2

Common Points • Q 1 – 4 generally well done • Q 5 – 8 differentiated well at various grades • Need to read questions carefully • Generally clear layout when a familiar question type • Difficulty in finding strategy when unfamiliar question type • Weak algebra • Weak numeracy • Weak prior knowledge

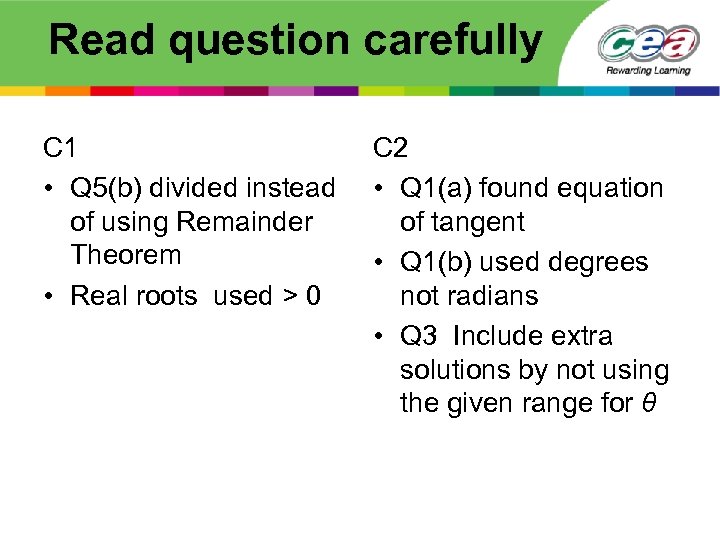
Read question carefully C 1 • Q 5(b) divided instead of using Remainder Theorem • Real roots used > 0 C 2 • Q 1(a) found equation of tangent • Q 1(b) used degrees not radians • Q 3 Include extra solutions by not using the given range for θ

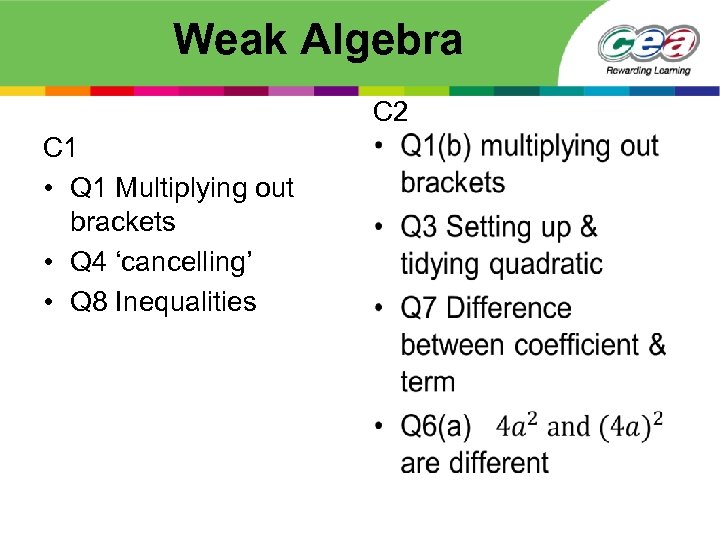
Weak Algebra C 1 • Q 1 Multiplying out brackets • Q 4 ‘cancelling’ • Q 8 Inequalities C 2 •

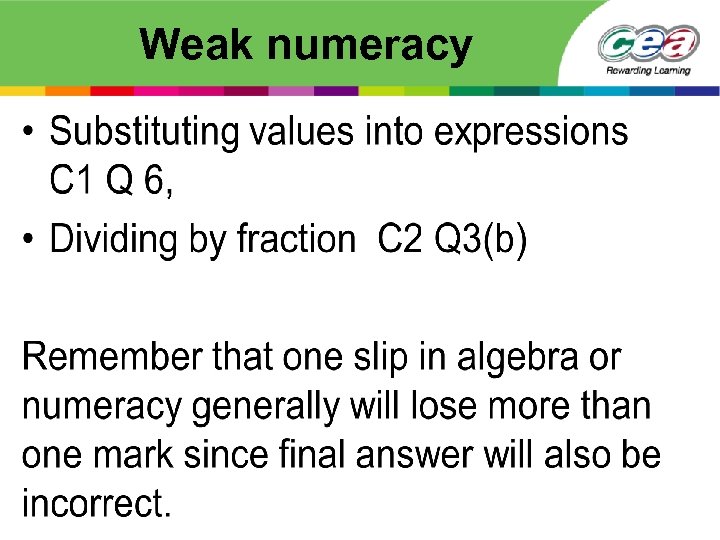
Weak numeracy •

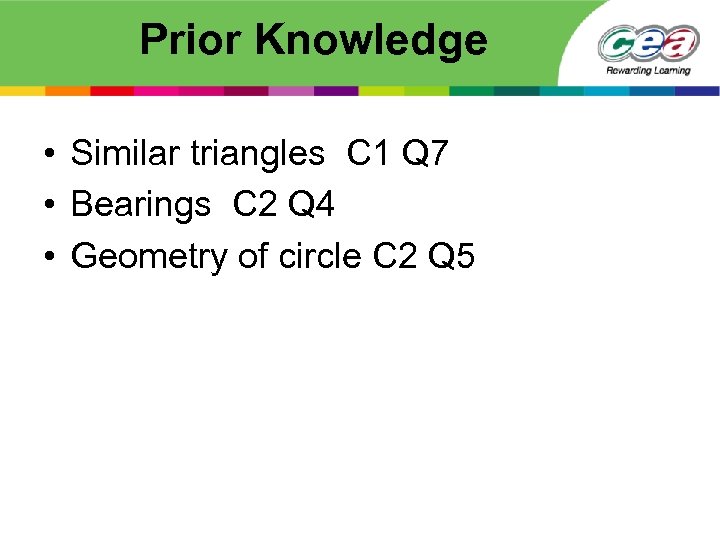
Prior Knowledge • Similar triangles C 1 Q 7 • Bearings C 2 Q 4 • Geometry of circle C 2 Q 5

C 1 Summer Series 2016

Q 2 Sketching Transformations • In (i), many still had sketch going through the origin • From 2017 onwards, candidates will answer on question paper so often axes will be already drawn.

Q 3 Indices •

Q 5 Algebraic Division and Remainder Theorem Marks lost here by not reading question carefully • Solving - not writing as linear factors • Using long division instead of Remainder Theorem

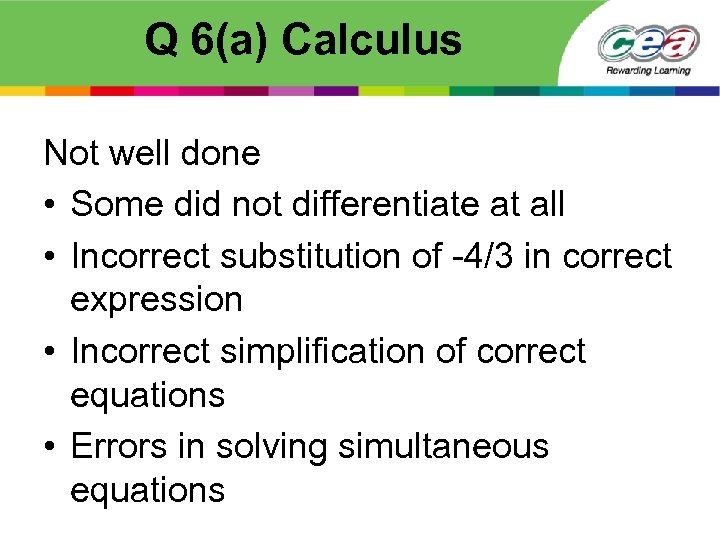
Q 6(a) Calculus Not well done • Some did not differentiate at all • Incorrect substitution of -4/3 in correct expression • Incorrect simplification of correct equations • Errors in solving simultaneous equations

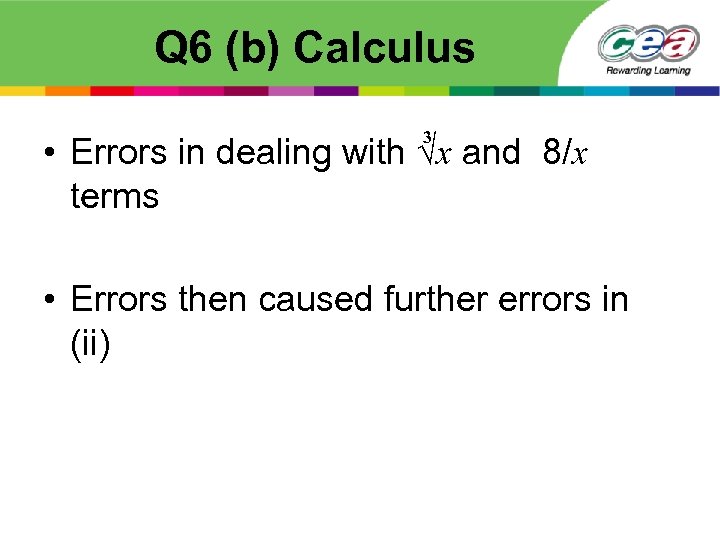
Q 6 (b) Calculus 3 • Errors in dealing with √x and 8/x terms • Errors then caused further errors in (ii)

Q 7(i) Max/ min Problem • • Not usual setup so many could not see how to start Very few used similar triangles Most used areas but often not correct ones Even if correct areas they were not always correctly combined • Poor layout in many cases • In (ii) poor notation often dy/dx rather than d. A/dw • Had expression for A not been given answers would not have been good

Q 8 (a) Quadratic roots Common errors • Using only > 0 • Incorrect substituting • Incorrect tidying up to quadratic • Not dealing correctly with negative squared term

Question 8(b) • Full marks only for the best candidates • Several different approaches tried • Unfamiliar setup so often poor layout of work • Many candidates had only 2 marks here for a correct first step in valid method

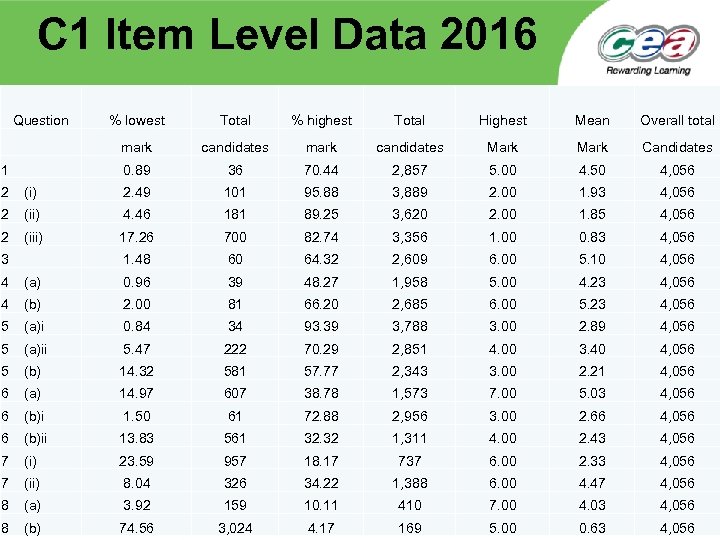
C 1 Item Level Data 2016 Question % lowest Total % highest Total Highest Mean Overall total mark candidates Mark Candidates 1 0. 89 36 70. 44 2, 857 5. 00 4. 50 4, 056 2 (i) 2. 49 101 95. 88 3, 889 2. 00 1. 93 4, 056 2 (ii) 4. 46 181 89. 25 3, 620 2. 00 1. 85 4, 056 2 (iii) 17. 26 700 82. 74 3, 356 1. 00 0. 83 4, 056 3 1. 48 60 64. 32 2, 609 6. 00 5. 10 4, 056 4 (a) 0. 96 39 48. 27 1, 958 5. 00 4. 23 4, 056 4 (b) 2. 00 81 66. 20 2, 685 6. 00 5. 23 4, 056 5 (a)i 0. 84 34 93. 39 3, 788 3. 00 2. 89 4, 056 5 (a)ii 5. 47 222 70. 29 2, 851 4. 00 3. 40 4, 056 5 (b) 14. 32 581 57. 77 2, 343 3. 00 2. 21 4, 056 6 (a) 14. 97 607 38. 78 1, 573 7. 00 5. 03 4, 056 6 (b)i 1. 50 61 72. 88 2, 956 3. 00 2. 66 4, 056 6 (b)ii 13. 83 561 32. 32 1, 311 4. 00 2. 43 4, 056 7 (i) 23. 59 957 18. 17 737 6. 00 2. 33 4, 056 7 (ii) 8. 04 326 34. 22 1, 388 6. 00 4. 47 4, 056 8 (a) 3. 92 159 10. 11 410 7. 00 4. 03 4, 056 3, 024 4. 17 169 5. 00 0. 63 4, 056 8 (b) 74. 56

C 2 Summer Series 2016

Question 1 • Some did not read question carefully • (a) Some found the equation of the tangent. • (b) Used degrees instead of radians • Correct formula needs to tried in order to gain marks

Question 2 • First part well done • Many did not explain what happened to fish population saying sequence diverged • (ii) many did not relate back to context saying • Negative number of fish • Population decreasing • (iii) Not well done. Not the usual setup

Question 4 • Poor knowledge of bearings caused many to lose marks • Many had wrong starting setup • Many did not know perpendicular distance was the shortest distance • Some good solutions by use of Pythagoras Theorem

Question 5 • Many were unhappy using radians. Often saw angle of 360 - 8π/9 • Many did not see logo was sector and 2 triangles • Many missed ABO was right angled then used Sine Rule incorrectly • Often lots of calculations which were not correctly put together • Layout of answers here not good since many did not have a definite method in mind at start

Question 6 •

Question 7 • Few scored well here • Some did not know difference between ‘term’ and ‘coefficient’ • There was difficulty dealing with the k in x/k in terms after the 2 nd • Simplifying a correct equation correctly was problem for many • (ii)The 4 was often on the wrong side • Basic equation was incorrectly simplified • This resulted in incorrect equation • (iii) Very few correct answers here since depended on correct (i)

Question 8 • • • (i) was not always correct Careless algebra cost many marks here. Various methods were possible The algebra defeated many. Errors were made in substitution and often squares were omitted on one or more terms • Many had more than one attempt at this

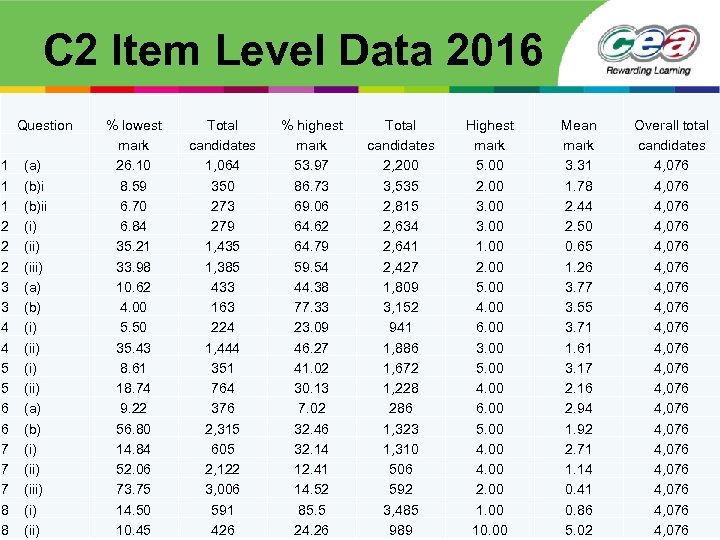
C 2 Item Level Data 2016 Question % lowest mark 1 (a) 26. 10 1 (b)i 8. 59 1 (b)ii 6. 70 2 (i) 6. 84 2 (ii) 35. 21 2 (iii) 33. 98 3 (a) 10. 62 3 (b) 4. 00 4 (i) 5. 50 4 (ii) 35. 43 5 (i) 8. 61 5 (ii) 18. 74 6 (a) 9. 22 6 (b) 56. 80 7 (i) 14. 84 7 (ii) 52. 06 7 (iii) 73. 75 8 (i) 14. 50 8 (ii) 10. 45 Total candidates 1, 064 350 273 279 1, 435 1, 385 433 163 224 1, 444 351 764 376 2, 315 605 2, 122 3, 006 591 426 % highest mark 53. 97 86. 73 69. 06 64. 62 64. 79 59. 54 44. 38 77. 33 23. 09 46. 27 41. 02 30. 13 7. 02 32. 46 32. 14 12. 41 14. 52 85. 5 24. 26 Total candidates 2, 200 3, 535 2, 815 2, 634 2, 641 2, 427 1, 809 3, 152 941 1, 886 1, 672 1, 228 286 1, 323 1, 310 506 592 3, 485 989 Highest mark 5. 00 2. 00 3. 00 1. 00 2. 00 5. 00 4. 00 6. 00 3. 00 5. 00 4. 00 6. 00 5. 00 4. 00 2. 00 10. 00 Mean mark 3. 31 1. 78 2. 44 2. 50 0. 65 1. 26 3. 77 3. 55 3. 71 1. 61 3. 17 2. 16 2. 94 1. 92 2. 71 1. 14 0. 41 0. 86 5. 02 Overall total candidates 4, 076 4, 076 4, 076 4, 076 4, 076

Information Day Summer Series 2016 Sara Neill Chief Examiner

General • For the first time 6 of the modules were marked online this summer • It is now even more important that candidates use only black ink • From next summer the format of the question papers is changing for C 1 - 4, M 1 and S 1 • Candidates will be expected to write their answers in the spaces provided on the question paper as in GCSE

General • Sufficient space will be made available in which candidates can write their answers. Some candidates repeat attempts at questions or try an alternate method if their first one is not working or like to go back to check their answers or …… extra paper available (referred to as additional objects) • So candidates need to be reassured that they do not need to fill all of the available space if they do not need to.

General comments on 2016 summer papers • As mentioned in the Chief Examiner’s report many excellent scripts were seen and pupils and teaching staff deserve much credit for this. • Algebra continues to be the greatest ‘bug bearer’. This is said every year but candidates do continue to lose significant marks. • Centres should not be distributing graph paper to candidates as only sketches are required at ‘A’ level. Those who use it waste time and often do not plot the points that show the information required to answer the question.

• Some candidates, on several papers, did not show the full development of their answers and so lost marks. • A number of candidates did answers side by side on the page and if parts were not clearly labelled this made the marker’s job rather difficult. • Candidates should be encouraged to work down the page.

• For the benefit of those teaching ‘A’ level for the first time, notes have been added before the power point which cover all of the general advice that candidates may need.

C 3 Summer Series 2016

C 3 • The paper was structured so that all candidates had success in the first few questions but only the more able were successful in answering the latter parts of the last questions. • The paper was of an appropriate length. • Markers commented that candidates’ work was sometimes untidy and poorly set out. • A number of candidates did not answer the question set but perhaps the one that they would have been preferred to have been answering.

Question 1 For those who used the appropriate trigonometric equation, this question was generally completed successfully. Any candidate who changed to sin/cos was generally left with a double angle formula that they could not move on from. Very few used an incorrect identity or did not have the required number of solutions.

Question 2 Part (ii) caused real problems as most did not know what they were being asked for. In (i), not putting the 2 x in brackets meant that answers were incorrect.

Question 3 On the new format we are hoping to include axes on which to sketch these graphs. Asymptotes being missing led to marks being lost in (a) (i) and (ii). A place where, if graph paper was used, sketches were often incomplete. It was disappointing that candidates did not always notice that the power on the top was greater than the power on the bottom or that there was a repeated term in (b). The idea of Partial Fractions was well known.

Question 4 If candidates had taken time to think about the set up this should have been an easy question. All they needed to do was integrate sin x between 0 and π and multiply by 4. Too many split it up into bits and sometimes forgot that the area below the x-axis is negative. Some got an answer of 0 and just left this: others had the sense to go back and rethink their work.

Question 5 (i) Was well answered. (ii) As happened before in questions of the type seen in (ii) and (iii), candidates did not read the question carefully and think about the function to use. The Newton. Raphson method was well known but was too often applied to the ‘y’ function. Too many applied N-R twice when they were only asked to apply it once.

Question 6 As this question was unstructured it was the least well done question on the paper. Some simply did not know where to start. The question asked for an answer in years which a number failed to do.

Question 7 Q 7 It was disappointing the number who had forgotten how to answer (i). C 3 is a synoptic paper and a question like this can be asked as a help in answering (ii). The differentiation in (ii) was very well done but candidates’ explanations of why dy/dx < 0 was poor. (i), as a connected part, was supposed to help but only the most able were able to pull it together properly.

Question 8 Part (a) was reasonably well done but errors were seen in differentiating the logarithm and in differentiating the top of the quotient. Only the most able were able to answer (b) – difficulties in being able to think what could be applied from their knowledge of C 1 – 3

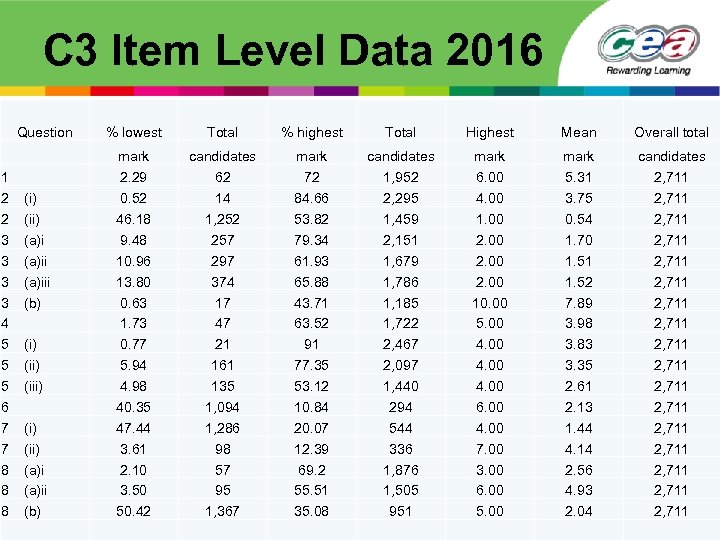
C 3 Item Level Data 2016 Question % lowest Total % highest Total Highest Mean Overall total mark 2. 29 0. 52 46. 18 9. 48 10. 96 13. 80 0. 63 1. 73 0. 77 5. 94 4. 98 40. 35 47. 44 3. 61 2. 10 3. 50 50. 42 candidates 62 14 1, 252 257 297 374 17 47 21 161 135 1, 094 1, 286 98 57 95 1, 367 mark 72 84. 66 53. 82 79. 34 61. 93 65. 88 43. 71 63. 52 91 77. 35 53. 12 10. 84 20. 07 12. 39 69. 2 55. 51 35. 08 candidates 1, 952 2, 295 1, 459 2, 151 1, 679 1, 786 1, 185 1, 722 2, 467 2, 097 1, 440 294 544 336 1, 876 1, 505 951 mark 6. 00 4. 00 1. 00 2. 00 10. 00 5. 00 4. 00 6. 00 4. 00 7. 00 3. 00 6. 00 5. 00 mark 5. 31 3. 75 0. 54 1. 70 1. 51 1. 52 7. 89 3. 98 3. 83 3. 35 2. 61 2. 13 1. 44 4. 14 2. 56 4. 93 2. 04 candidates 2, 711 2, 711 2, 711 2, 711 2, 711 1 2 (i) 2 (ii) 3 (a)iii 3 (b) 4 5 (i) 5 (iii) 6 7 (i) 7 (ii) 8 (a)ii 8 (b)

C 4 Summer Series 2016

C 4 • Again the paper allowed for differentiation between candidates of all abilities. • In this paper the poor use of trigonometry as well as of algebra led to a loss of marks. • A number of candidates scored out work without doing it again and a number left different solutions for the marker to choose the correct one. • Some of even the very best candidates had problems with the function question.

Question 1 A small number of candidates did not recognise that they had to use Partial Fractions. A minor error was to leave out the negative sign in the logarithmic term after integrating.

Question 2 In Q 2, (i) a number failed to find the magnitude of the vector. A number in (ii) failed to give an equation – they omitted the r = …. . In (iii), some forgot to compare all components. Generally candidates answered this question well.

Question 3 The usual mistakes were seen in the answering of this question. Candidates divided through by cosine and so lost solutions or forgot that the square root can be both positive and negative – again losing solutions. A small number did not give answers in the required range.

Question 4 Parts (i) and (ii) of Q 4 were generally well done but in (iii) too many did not read the question carefully enough and sketched the curve in the 1 st and 2 nd quadrants when the sketch should only have been in the 1 st quadrant. Part (iv) was very poorly answered. A different sort of question that required some thought and understanding of functions.

Question 5 Candidates knew how to do implicit differentiation but as the answer was on the paper, sign errors, made at the start, suddenly disappeared in (i). Poor algebraic manipulation in (ii) led to errors when some candidates tried to simplify the 2 nd derivative. It was unnecessary to do the simplification as x and y could have been substituted earlier. Candidates need to find the 2 nd derivative before they comment on its sign. (see mark scheme)

Question 6 This question was slightly different from those set previously and so many struggled to produce a coherent mathematical argument. Candidates should have started with the ‘given that’ and worked to the tan x. Candidates should not start with what they are asked to prove. (see mark scheme)

Question 7 In Q 7, most candidates knew how to start and set up the integration correctly. However, many did not realise that the double angle formula was required before the integration could be completed. (see mark scheme)

Question 8 The term ‘gradient function’ is a term that all candidates should know but a number sitting this paper did not appear to know that the expression that they had been given was dy/dx. So they did not realise that what they were being asked to do was solve a differential equation. The most common error was to separate the variables incorrectly. (see mark scheme) Some candidates tried to do ‘parts’ on a function involving both x and y. However, able candidates did well on this question.

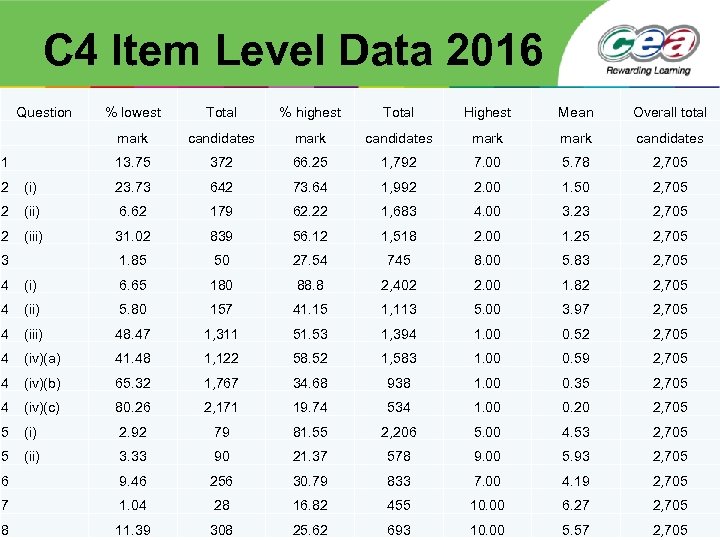
C 4 Item Level Data 2016 Question % lowest Total % highest Total Highest Mean Overall total mark candidates 1 13. 75 372 66. 25 1, 792 7. 00 5. 78 2, 705 2 (i) 23. 73 642 73. 64 1, 992 2. 00 1. 50 2, 705 2 (ii) 6. 62 179 62. 22 1, 683 4. 00 3. 23 2, 705 2 (iii) 31. 02 839 56. 12 1, 518 2. 00 1. 25 2, 705 3 1. 85 50 27. 54 745 8. 00 5. 83 2, 705 4 (i) 6. 65 180 88. 8 2, 402 2. 00 1. 82 2, 705 4 (ii) 5. 80 157 41. 15 1, 113 5. 00 3. 97 2, 705 4 (iii) 48. 47 1, 311 51. 53 1, 394 1. 00 0. 52 2, 705 4 (iv)(a) 41. 48 1, 122 58. 52 1, 583 1. 00 0. 59 2, 705 4 (iv)(b) 65. 32 1, 767 34. 68 938 1. 00 0. 35 2, 705 4 (iv)(c) 80. 26 2, 171 19. 74 534 1. 00 0. 20 2, 705 5 (i) 2. 92 79 81. 55 2, 206 5. 00 4. 53 2, 705 5 (ii) 3. 33 90 21. 37 578 9. 00 5. 93 2, 705 6 9. 46 256 30. 79 833 7. 00 4. 19 2, 705 7 1. 04 28 16. 82 455 10. 00 6. 27 2, 705 8 11. 39 308 25. 62 693 10. 00 5. 57 2, 705

M 1 Summer Series 2016

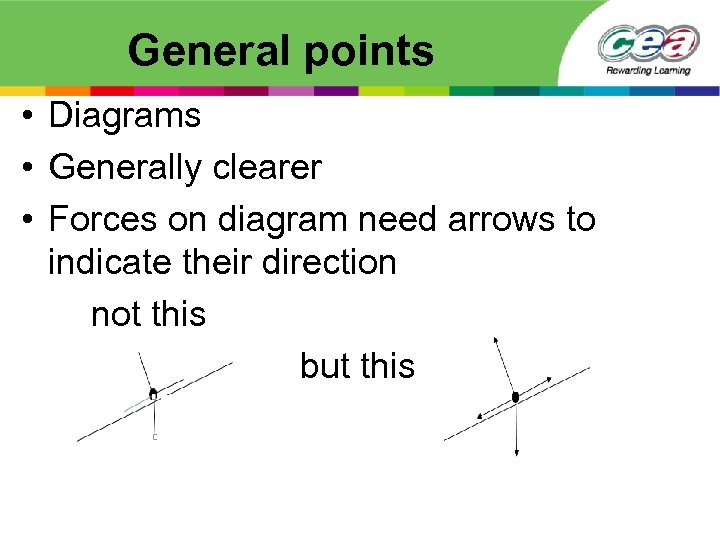
General points • Diagrams • Generally clearer • Forces on diagram need arrows to indicate their direction not this but this

General points • Algebraic errors led to wrong answers form correct mechanics • Trigonometric errors led to wrong answers from correct mechanics • In Q 2 many treated graph as v-t graph rather than s-t graph

Q 1 Equations of motion •

Q 2 s – t graph • Some took graph as v - t graph. Lost marks • Some did not know difference between displacement and distance • Some did not know how to find average speed

Q 4 Acceleration as a function of time • When asked to show k = -7. 2 means it should not be assumed first • Finding + c for displacement better this time • Combining the various displacements to find the distance covered not well done

Q 5 Impulse and momentum • As vector quantities direction is important • Indicating which direction is positive at start of question would help • Many lost mark in (ii) by losing the negative sign

Q 6 F = ma and Friction etc • Needed good diagram with arrows on forces • Needed clearly developed solution • Read question carefully for X moving UP plane, Y on SMOOTH plane • Best solutions found • Finding R for X • Finding Friction for X • F = ma along plane for X • All combined • F = ma along plane for Y • Solve the simultaneous equations

Q 7 Moments • Better attempts this year • Fewer had extra forces on diagram • More knew to use perpendicular distance in moment • Still many errors in finding the perpendicular distances • Still many errors in solving the correct equation • Some did not see taking moments about A was easiest

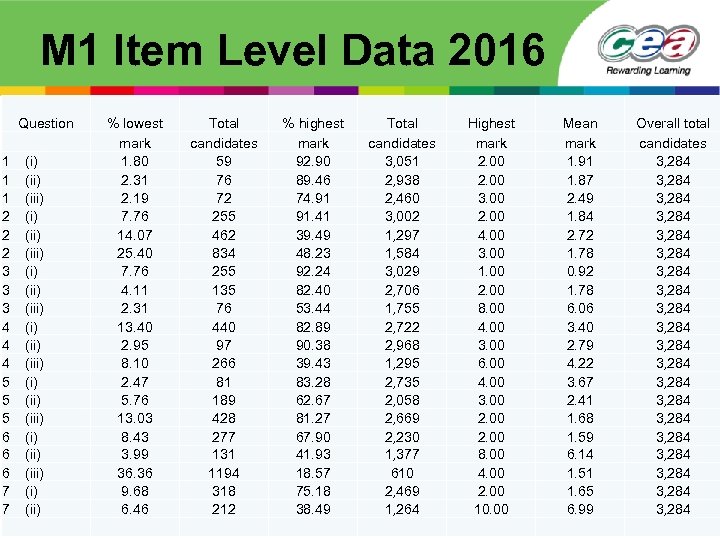
M 1 Item Level Data 2016 Question % lowest mark 1 (i) 1. 80 1 (ii) 2. 31 1 (iii) 2. 19 2 (i) 7. 76 2 (ii) 14. 07 2 (iii) 25. 40 3 (i) 7. 76 3 (ii) 4. 11 3 (iii) 2. 31 4 (i) 13. 40 4 (ii) 2. 95 4 (iii) 8. 10 5 (i) 2. 47 5 (ii) 5. 76 5 (iii) 13. 03 6 (i) 8. 43 6 (ii) 3. 99 6 (iii) 36. 36 7 (i) 9. 68 7 (ii) 6. 46 Total candidates 59 76 72 255 462 834 255 135 76 440 97 266 81 189 428 277 131 1194 318 212 % highest mark 92. 90 89. 46 74. 91 91. 41 39. 49 48. 23 92. 24 82. 40 53. 44 82. 89 90. 38 39. 43 83. 28 62. 67 81. 27 67. 90 41. 93 18. 57 75. 18 38. 49 Total candidates 3, 051 2, 938 2, 460 3, 002 1, 297 1, 584 3, 029 2, 706 1, 755 2, 722 2, 968 1, 295 2, 735 2, 058 2, 669 2, 230 1, 377 610 2, 469 1, 264 Highest mark 2. 00 3. 00 2. 00 4. 00 3. 00 1. 00 2. 00 8. 00 4. 00 3. 00 6. 00 4. 00 3. 00 2. 00 8. 00 4. 00 2. 00 10. 00 Mean mark 1. 91 1. 87 2. 49 1. 84 2. 72 1. 78 0. 92 1. 78 6. 06 3. 40 2. 79 4. 22 3. 67 2. 41 1. 68 1. 59 6. 14 1. 51 1. 65 6. 99 Overall total candidates 3, 284 3, 284 3, 284 3, 284 3, 284

S 1 Summer Series 2016

S 1 • Again the paper allowed candidates to show their depth of knowledge and understanding. • Q 1, 2 and 3 were well done by most candidates. From Q 4 onwards, some candidates started to lose marks. • Candidates should be reminded that they should use the tables provided.

Question 1 This question was answered well by most. In (ii) a few candidates forgot to change lambda.

Question 2 (i) Was extremely well done. (ii) A small number of candidates used a discrete variable approach or had incorrect limits. Otherwise this part was well done. (iii) Last year too many candidates had an incorrect formula for Var(X). Few candidates used an incorrect formula this year.

Question 3 Again candidates coped well with this question although a number mixed up ‘at most’ with ‘at least’. In (iii) some candidates calculated the answers from basics when they could simply have used the formulae given in the formulae booklet.

Question 4 Some candidates did not use the tables provided to set up the equation to find the mean. Those who had an incorrect mean from (i) often had difficulty when answering (ii) and (iii) as their z-values were outside the range of the tables provided. Part (ii) was the point at which the less able candidate started to have difficulty. These candidates may have used the tables ‘the wrong way round’ or been unable to find the correct method for the combination of the probabilities. It was surprising that a large number of candidates failed to give their answer as a percentage.

Question 4 Q 4 (iii) only the most able correctly answered this part. Most did not recognise conditional probability. A number who knew that they had to use conditional probability only found P(X ≥ 117) for the numerator but because probability <1 then turned the fraction upside down. This normal distribution question is, for many, easier to answer if they draw diagrams. Especially in (ii) it enables them to see the area that they need to find. They then can work out a way of finding that area.

Question 5 In Q 5(i) most candidates calculated their answers using a calculator. Candidates need to show some of the interim steps otherwise if their answer is wrong, a marker does not know if their method was correct. (ii) In statistics questions’ solutions to parts like this need to be related to the context of the question. Too many candidates simply compared the data values.

Question 6 Part (a) was testing basic theory and most candidates did well in this part. However, some knew that ‘exhaustive’ meant that the total probability was 1 but then used P(A)+P(B) = 1 (b) Was the least well done question on the paper and was difficult to mark. It was not always clear what candidates were trying to do. Candidates were asked to find x but some candidates on getting to the equation in x stopped. Others only gave 1 of the 2 possible answers.

Question 7 Candidates needed to realise in Q 7 that if sweets were eaten that this was a trial without replacement question. To obtain full marks in (ii) candidates needed to have all of the probabilities correct. Many had only one correct probability, usually for 1, and scored 2 out of 6 (see mark scheme). Some candidates tried to use a uniform distribution to find the probabilities. Again obtaining mark in (iii) depended on the probabilities in (ii) being correct.

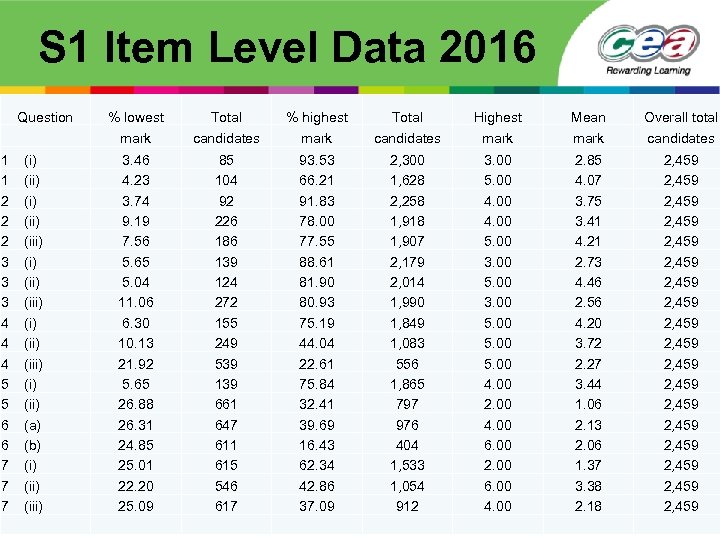
S 1 Item Level Data 2016 Question Total % highest Total Highest Mean Overall total mark 1 (i) 1 (ii) 2 (iii) 3 (i) 3 (iii) 4 (i) 4 (iii) 5 (i) 5 (ii) 6 (a) 6 (b) 7 (ii) 7 (iii) % lowest candidates mark candidates 3. 46 4. 23 3. 74 9. 19 7. 56 5. 65 5. 04 11. 06 6. 30 10. 13 21. 92 5. 65 26. 88 26. 31 24. 85 25. 01 22. 20 25. 09 85 104 92 226 186 139 124 272 155 249 539 139 661 647 611 615 546 617 93. 53 66. 21 91. 83 78. 00 77. 55 88. 61 81. 90 80. 93 75. 19 44. 04 22. 61 75. 84 32. 41 39. 69 16. 43 62. 34 42. 86 37. 09 2, 300 1, 628 2, 258 1, 918 1, 907 2, 179 2, 014 1, 990 1, 849 1, 083 556 1, 865 797 976 404 1, 533 1, 054 912 3. 00 5. 00 4. 00 5. 00 3. 00 5. 00 4. 00 2. 00 4. 00 6. 00 2. 00 6. 00 4. 00 2. 85 4. 07 3. 75 3. 41 4. 21 2. 73 4. 46 2. 56 4. 20 3. 72 2. 27 3. 44 1. 06 2. 13 2. 06 1. 37 3. 38 2. 18 2, 459 2, 459 2, 459 2, 459 2, 459

M 2 Summer Series 2016

M 2 • This paper is still taken by a large number of candidates as part of their ‘A’ Level. • Candidates taking this paper are usually very good at Mechanics. Very few candidates obtained fewer than 40 marks. • Q 2 – 5 were successfully attempted by the vast majority of candidates.

Question 1 The force in this question is not dependent on ‘t’ so can be answered using the constant acceleration formulae. Some candidates chose to use integration in (i) and (ii) but forgot to consider the constants of integration. A number did not seem to know what a unit vector was when answering (iii). A disappointing response to an easy question.

Question 2 This question was well done although simple errors, perhaps on not reading the question carefully enough, were seen: using s = 3 m rather than 2. 1 in (i), forgetting to add on the 0. 9 in (ii) or suggesting inappropriate refinements in (iii). (see mark scheme)

Question 3 Some candidates did not check that t = 5 worked for both components nor made a statement to this effect. Otherwise candidates knew what to do and did it well.

Question 4 A small number of candidates, again perhaps because they did not read the question carefully, had θ in the wrong place. A very few mixed up the use of sin/cos in resolving. Otherwise very well done.

Question 5 Part (iii), again suggesting a more realistic model, was the only place where a few candidates lost a mark.

Question 6 This was a ‘pump question’ and proved to be the hardest question for most candidates. Candidates do not seem to like working with rates of energy used in questions of this type. Candidates were inclined to get an incorrect rate for the mass moved per second. (see mark scheme) Words on their page, as in the mark scheme, might help them.

Question 7 Many candidates had practically correct scripts up until this question. The question did not give the equation that they had to solve in (ii). So a surprising number used the constant acceleration equations of motion!! Others put the answer to (i) in before the integration or did not use this value of v to get the final answer. Candidates should not rely on the equation modelling the set-up, to be on the question paper.

F 1 Summer Series 2016

F 1 • Candidates seemed to have a good understanding of all of the topics tested. • Only the most able were successful at all questions. • At this level it was disappointing to note that presentation was often poor. • Too many basic algebraic errors were seen. • Some candidates deleted work but did not attempt to do the question again? ?

Question 1 Part (i) of Q 1 was well done but (ii) was poorly done. An algebraic solution was required but many gave a numerical solution. Candidates need to show a clear development of their answer.

Question 2 The usual confusion about the determinant was seen. Is the determinant equal to or not equal to zero? Some candidates multiplied all of the terms out, tidied up but then could not factorise the cubic (see mark scheme).

Question 3 Part (a) was well answered but in (b) the usual confusion between invariant points and invariant line was seen. Poor algebra caused problems for some in this question.

Question 4 Parts (i) and (ii) of Q 4 were generally well done although in (i) some candidates wasted their time by finding all of the eigenvalues when they were only asked for two. In (iii) a number of candidates failed to gain full marks as they did not use the unit eigenvectors in U.

Question 5 Poor algebra and poor presentation caused too many candidates to lose marks in Q 5. Most knew exactly how to tackle both parts but excessively awkward solutions should have led some to think ‘might I have made a mistake’ or ‘misread my previous work’.

Question 6 Candidates do not generally ‘like’ Group questions. The answers to Q 6 split into two groups – those who knew exactly what to do and those who did not. Some did not know how to find the period of an element or complete a group table. Too many did not have a clear idea of what an isomorphism was. They were asked to state an isomorphism and many failed to do so.

Question 7 Parts (i) and (ii) of Q 7 were answered well. However, some candidates did not give the argument in radians or in the correct range. In (ii) diagrams needed to be of a reasonable size, to be neat and clearly labelled. Very few candidates answered the last part of this question correctly. A clear argument should have been given in finding the answer to (iii). (see mark scheme)

F 2 Summer series 2016

General performance • Good performance in general. • Work well presented • Students were clearly well prepared for the paper • Q 1 and 2 gave a solid start for most students • Students generally showed confidence in tackling the more difficult questions

General performance • Answers given on paper for 8 parts helped students not to pursue algebraic lost causes over several pages • However they also meant students made logic defying jumps to get to the answer! • Students should remember to show development of their answers. • Full marks are not always awarded for a correct answer when method is not clear.

General performance • Questions 1, 2, 4 and 6 were generally very well done. • Q 3(a) provided challenge. – Few tried to use R sin(A + B) – Methods using tan, sine and cosine were tried unsuccessfully – Many could not complete this question.

Question 5 • Q 5 (i) was very well done • (ii) much less well done. • Many chose to find point of intersection and then tried to eliminate t -usually without success

Q 7 Differential Equation • (i) Not as well done as usual – Many did not divide through by L and so had incorrect integrating factor – Some did not multiply both sides by integrating factor – The constant L caused confusion • (ii) well done by those who had (i) correct

Q 8 Complex numbers • First parts well done • In (iii) many correctly solved the quadratic equation but gave no justification for taking (3+√ 5)/8 and so lost marks

F 3 Summer Series 2016

General comment • Marks were lower than usual on this paper • Students had difficulty with Q 2 and 3(b), 6 and 7 • Time appeared to be a pressure. Some did not try 7(ii) • Didn’t seem to know when to leave one question and move on to the next e. g 4 pages of work for 5 (ii) for 7 marks

Questions • Q 1, 4 and 5 were generally well done • Q 2 on generalised vectors was poorly done. • Many scored very few marks often none in (i) and 1 in (ii) for a. n = d Slightly more in (iii)

Questions • Q 3 (a) was quite well done • (b) Very few correct solutions. Most tried various substitutions instead of completing the square for term under root sign • Q 6 (ii) was quite well answered – Few had the correct shape for sketch in (i) – Many missed integration by parts in (iii) and so could score very few marks

Question 7 • Many candidates had difficulty with this question. • Many candidates did not attempt (ii) possibly due to time pressure • Those who did usually did well • Some candidates assumed TW was parallel to SV and so lost 4 marks

M 3 Summer Series 2016

M 3 • Candidates taking this module are usually able and well prepared. This was the case in the summer. • Questions 4(ii), 4(iii) and 6 proved to be the most testing for candidates.

Question 1 All parts were well attempted by the majority of candidates. In (i) it was easier to resolve along AP and PB rather than to resolve horizontally and vertically. In (ii) a few used the stretched length rather than the natural length when finding the tension in the string. Some candidates took the modulus of elasticity to be the same in both strings.

Question 2 In Q 2 (i) a number of candidates took the vector AB in the wrong direction. Some omitted the work done by F 1 in (ii). Otherwise the question was well done.

Question 3 Generally well done although a few got confused in (iv) in how to find the time of one oscillation. They had 4 x 4. 5

Question 4 Q 4 was where some candidates’ work was less good. Some did not know where the centre of gravity of the triangle was in (i). In (ii) some did not give the mass of the logo in terms of m and in (iii) the majority of candidates did not have the force at C to be at right angles to BC.

Question 5 All parts of Q 5 were well done although in (ii) a few failed to complete the integration and in (iv) a few failed to check that the 2 nd derivative was positive.

Question 6 • Candidates did not do so well in the relative velocity question Q 6. Those who tried to use an i/j approach were far less successful than those who drew diagrams. • The trigonometry in (i) and (iii) was far more complicated for those who used i s and j s. • The discriminating part of the question was (iii). • A good diagram made it easy but few good diagrams were seen. The answer was dependent on the candidate realising that they had to use the

M 4 Summer Series 2016

M 4 • Again candidates were able and well prepared. • The questions where a number of candidates made mistakes were 1(iii), 3(ii), 4(ii), 5(iv) and 6(iv). On the other questions few mistakes were seen. • In 1(iii), the majority of candidates gave the vertical component of the reaction at B as their final answer. • In 3(ii), some tried to integrate xy not having replaced the x. (see mark scheme)

M 4 Instead of resolving vertically and horizontally, a number resolved along and at right angles to the plane leaving out the components of the force towards the centre in Q 4(ii). Deciding which inequality to use in Q 5(iv) gave some candidates a problem. Only a minority realised what they had to do to answer Q 6(iv); they simply needed to work out the length of the arc of the equator that the satellite could see and divide this into the length of the equator. Some compared the circumferences of Jupiter and the satellite’s orbit whilst others tried to use periodic time to find an answer.

S 4 Summer series 2016

General Comments • Most candidates were well prepared for paper • Many very good answers produced

Some issues • Accuracy – Rounding values and then using these later – Misreading of normal tables or finding these from calculator • Written answers – Answers in words were weak and – Sometimes indicated little understanding of concepts involved • Hypothesis tests – Careful phraseology needed – Test is about deciding if there is sufficient evidence to reject H 0

Comments on questions • Q 1 and 2 were well done • Q 3 not as well done as usual – Some didn’t treat table as frequency distribution – Some didn’t work to 5 or more sig figs to give answer correct to 4 significant figures – Q 4 (ii) generally well answered but – Many students were unfamiliar with tables of random digits – Many showed little knowledge of sampling

S 4 • Q 5 The decision should be – ‘to reject the Null Hypothesis’ or – ‘to not reject the Null Hypothesis’ • Result of test must be related back to the original question

S 4 • Q 6 (i) and (ii) were well answered. – Using summary values in (i) is best – Some accuracy issues in calculations and use of these answers • Some did not read the question carefully and gave the answer ‘outside the range’ for (iii). Maybe this indicated a lack of understanding

S 4 • Q 7 not as well answered as Q 5. In this question, the student had to set up the start of question and ensure alternative hypothesis and critical value were consistent with this. • Those who had learnt the solution by rote found this more difficult than those who clearly understood the process.

S 4 • Q 8 Generally well answered. • Students need to remember to use the normal tables provided • A few had difficulty dealing with the modulus function in (ii)

Contact Details Joe Mc. Gurk – Telephone 9026 1443 – Email jmcgurk@ccea. org. uk Nuala Braniff (Specification Support Officer) –Telephone 9026 1200 extension 2292 –Email nbraniff@ccea. org. uk