1816162d77a1ba1068f5a69959b89e8c.ppt
- Количество слайдов: 86

GBS 520 : FINANCIAL AND MANAGEMENT ACCOUNTING BY BRYSON MUMBA MBA, MAcc, FCCA, FZICA, Di. CG, BSc(Hons) 1

FINANCIAL AND MANAGEMENT ACCOUNTING UNIT 7 : INFORMATION FOR DECISION MAKING 2

Information for Decision Making Unit 7: Information for Decision Making 1. Cost Volume Profit Analysis 2. Product Mix Decisions 3. Relevant costs and Operational Decisions 4. Relevant costs and Strategic Decisions 3

Outline • Pricing • Full cost pricing • Rate of return pricing • Marginal cost pricing • Break even analysis: • Profit/Volume planning • Short term decision making • • • Pricing special orders Maximising profit from scarce resources Multiple scarce resources Closing Down a department or product Product Mix Make or buy decisions 4

Definitions of terms • Pricing • Marginal Cost • Marginal revenue • Margin of safety • Contribution ratio • Break even point 5

Pricing • Full cost pricing • Rate of return pricing • Marginal cost pricing 6

Pricing - Introduction • Pricing – is critical to the health and survival of any firm • Pricing decision factors: • Costs: • Total costs or • Marginal cost • Non-cost factors – e. g. supply demand situation • Aim –: • To set a price that maximises the profits when the Total Revenue less Total Costs is Greatest • At a quantity when Marginal cost = Marginal revenue. • Marginal cost is the cost of one additional unit • Marginal revenue is the revenue of one additional unit 7

Full cost pricing • All costs are charged to products • Selling Price = Direct costs + Share of indirect costs + Profit Margin • Example: • If a company produces and sells a product A with the following costs: • Direct costs per unit = K 10. 00 • Share of indirect costs – at 15% of the direct costs • Profit mark-up is set at 20% of total costs • Required • Calculate the selling Price using the Full cost pricing • Solution • • Selling Price = Direct costs + share of indirect costs + profit margin Full costs = K 10. 00 + 15% x K 10. 00 = K 11. 5 Profit margin = 20% of Full costs = K 2. 30 Selling Price = K 11. 5 + K 2. 30 = K 13. 80 8

Full cost pricing • Advantages • Simple • Disadvantages • Overhead recovery rates set based on budgeted figures and if the actual outcomes differ from the budget or actual costs differ from budget, the recovery rates may be out of date • Not dynamic especially in times of rapid changing demand conditions 9

Rate of return pricing • Another variation of full cost pricing • Instead of profit mark-up the return on capital employed is used to arrive at the selling Price • Selling price = Full costs + ROCE • E. g. Product total costs = K 5. 000 • Capital employed = K 2, 500 • Target ROCE is say 20% • Required • Calculate the selling price • Solution • ROCE = 20% x capital employed = 20% x K 2, 500 = K 500 • Selling Price = K 5, 000 + K 500 = K 5, 500. • What is the mark-up on the costs? 10

Marginal cost pricing • Marginal costing is the term used to the separation of total costs into their fixed costs and variable costs • Costs are analysed based on their behaviour – fixed or variable • In marginal cost pricing, fixed costs are not apportioned to individual products but are left as a total sum for the firm • Avoids arbitrary apportionment of fixed costs inherent in full cost pricing • Main objective in marginal cost pricing is to maximize the contribution • Contribution = sales revenue – Variable costs • Contribution per unit = selling price per unit – variable costs per unit • Selling price = variable costs + contribution 11

Marginal cost pricing • Contribution = sales revenue – Variable costs • Contribution per unit = selling price per unit – variable costs per unit • Selling price = variable costs + contribution 12

Break-even analysis. 13
Introduction to Break Even Analysis • Breakeven analysis is also known as cost-volume profit (CVP) analysis • Breakeven analysis is the study of the relationship between selling prices, sales volumes, fixed costs, variable costs and profits at various levels of activity • Uses of Breakeven analysis: • To find level of activity where total sales = total costs, i. e. company make zero profit i. e. the Break-even Point(BEP) • To find the level of activity where a target level of profits will be achieved • Determine margin of safety • Visualising the effect of a change in any variable on the BEP and profit • Determining contribution levels as a indicators of product profitability • Calculating the level of operating gearing as an indicator of the effect of profit of a given change in sale 14

Application • Breakeven point is a level of activity at which the total revenue is equal to the total costs • At this level, the company makes no profit 15
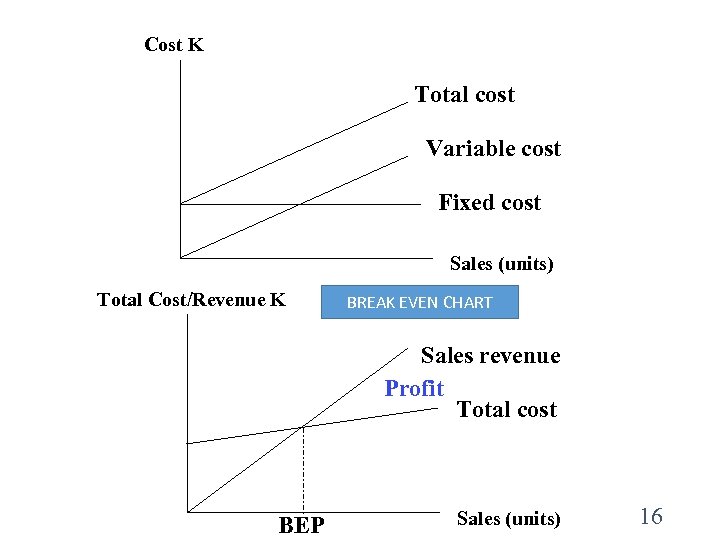
Cost K Total cost Variable cost Fixed cost Sales (units) Total Cost/Revenue K BREAK EVEN CHART Sales revenue Profit Total cost BEP Sales (units) 16
Assumption of breakeven point analysis • Relevant range • The relevant range is the range of an activity over which the fixed cost will remain fixed in total and the variable cost per unit will remain constant • Fixed cost • Total fixed cost are assumed to be constant in total • Variable cost • Total variable cost will increase with increasing number of units produced

Assumption of breakeven point analysis • Sales revenue • The total revenue will increase with the increasing number of units produced

Margin of Safety • Margin of Safety represents the amount by which sales can fall from its current level to the BEP 19

BREAK EVEN CHART Total Cost/Revenue K Sales revenue Profit Total cost Loss Margin of safety BEP Q Sales (units)

Calculations • Methods for: • Contribution • Breakeven point • Contribution ration • Target sales volume and target profit • Margin of safety • Operating gearing • Changes in components of breakeven analysis/multiproducts 21

Calculation method • Contribution is defined as the excess of sales revenue over the variable costs • Contribution = Sales revenue – Variable costs • Contribution per Unit = Selling Price per unit - Variable costs per unit • At BEP , the total contribution is equal to total fixed cost 22

Calculating • Selling Price = K 10 • Variable Costs per unit = 7 • Total fixed costs = K 60, 000 • Required • Calculate contribution per unit • Calculate BEP 23

Contribution per Unit • Contribution per unit = selling price per unit – variable cost per unit = K 10 – K 7 = K 3 24
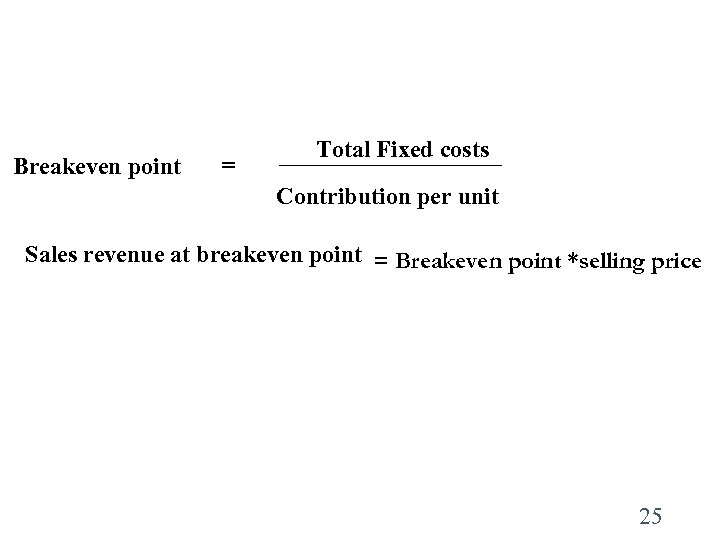
Breakeven point = Total Fixed costs Contribution per unit Sales revenue at breakeven point = Breakeven point *selling price 25

BEP • BEP = K 60, 000/K 3 = 20, 000 units 26

Sales revenue at breakeven point = Breakeven point *selling price = 20, 000 x K 10. = K 200, 000. 27

Example • Selling price per unit • Variable cost per unit • Fixed costs Required: K 12 K 3 K 45000 • Compute the breakeven point • Sales revenue at breakeven point • 28

Breakeven point in units = Fixed costs Contribution per unit = K 45000 K 12 -K 3 = 5000 units Sales revenue at breakeven point = K 12 * 5000 = K 60000 29

Target Volume and Target profit . 30

Formula No. of units at target profit: = Fixed cost + Target profit Contribution per unit Required sales revenue = Contribution per Unit x Selling Price per unit 31

Example • Selling price per unit • Variable cost per unit • Fixed costs • Target profit Required: K 12 K 3 K 45000 K 18000 • Compute the sales volume required to achieve the target profit • Calculate the target sales revenue 32

No. of units at target profit Fixed cost + Target profit = Contribution per unit K 45000 + K 18000 = K 12 - K 3 = 7000 units Required to sales revenue = K 12 *7000 = K 84000 33

Contribution Ratio • Instead of expressing the BEP or target volume in units, it is possible to express them in Sales Revenue terms • This uses the concept of Contribution Ratio • Contribution Ratio = Contribution per unit/Selling Price 34

Example • Selling price per unit • Variable cost per unit • Fixed costs • Target profit Required: K 12 K 3 K 45000 K 18000 • Compute the sales volume required to achieve the target profit • Calculate the target sales revenue 35

Example • • Selling price per unit Variable cost per unit Fixed costs Target profit K 12 K 3 K 45000 K 18000 • Contribution = K 12 - K 3 = K 9 • Contribution to sales ratio = 9/12 = 0. 75 or 75%. 36
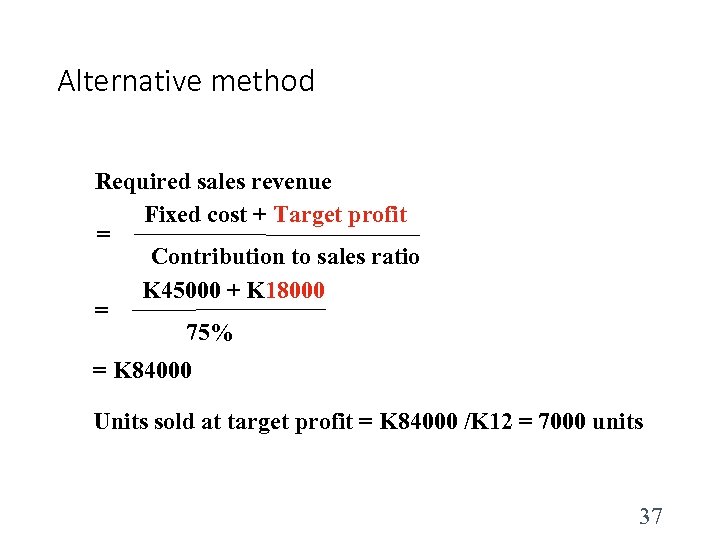
Alternative method Required sales revenue Fixed cost + Target profit = Contribution to sales ratio K 45000 + K 18000 = 75% = K 84000 Units sold at target profit = K 84000 /K 12 = 7000 units 37

Margin of safety. 38

Margin of safety • Margin of safety is a measure of amount by which the sales may decrease before a company suffers a loss. • This can be expressed as a number of units or a percentage of sales 39

Formula Margin of safety in UNITS = Budget sales level – breakeven sales level Margin of safety as % = Margin of safety *100% Budget sales level 40
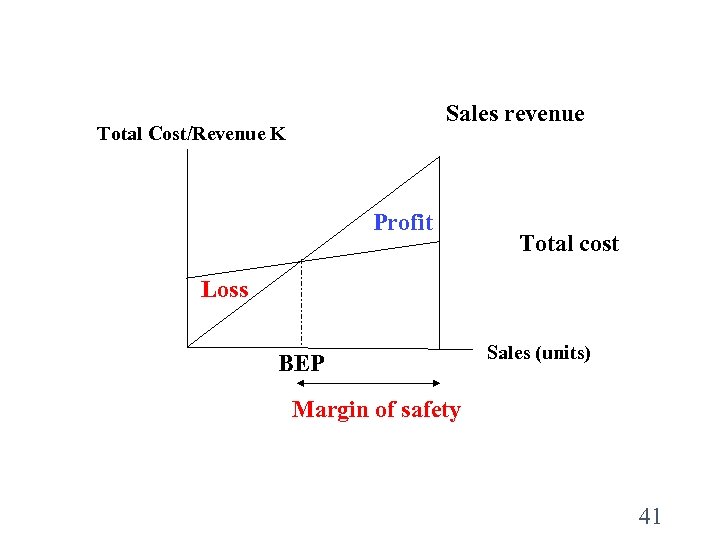
Sales revenue Total Cost/Revenue K Profit Total cost Loss BEP Sales (units) Margin of safety 41

Example • The breakeven sales level is at 5000 units. The company sets the target profit at K 18000 and the budget sales level at 7000 units Required: Calculate the margin of safety in units and express it as a percentage of the budgeted sales revenue 42

Margin of safety in UNITS = Budget sales level – breakeven sales level = 7000 units – 5000 units = 2000 units Margin of safety as % = Margin of safety *100 % Budget sales level = 2000 *100 % 7000 = 28. 6% The margin of safety indicates that the actual sales can fall by 2000 units or 28. 6% from the budgeted level before losses are incurred. 43

Operating Gearing • Operating Gearing quantifies how much effect a given change in sales will have on profit. • Calculating Operating Gearing • Operating Gearing = Contribution /Profit • Example • Assume a company makes a contribution of K 100, 000 and the profit is K 20, 000. • Required • Calculate the Operating Gearing 44

Operating Gearing • Calculating Operating Gearing • Operating Gearing = Contribution /Profit • = 100, 000/20, 000 • =4 • Meaning - a change in sales will have a 4 times change in Profits. 45

Exercise • The following statement relates to a product which sells at K 15. 00 per unit. 46
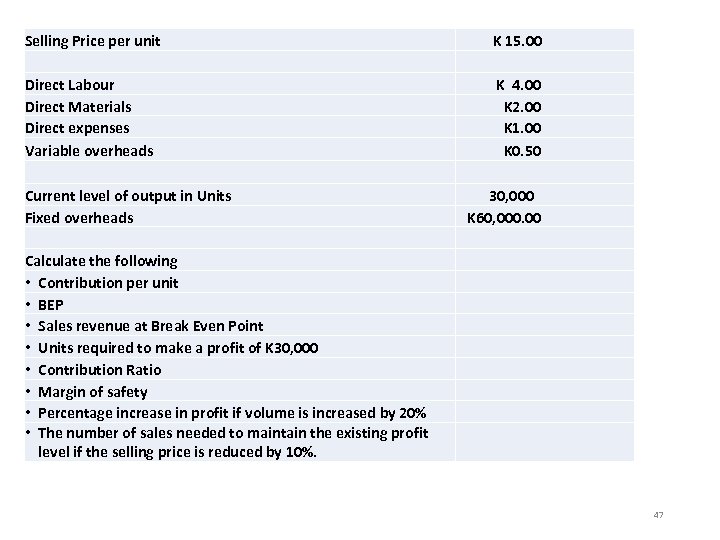
Selling Price per unit K 15. 00 Direct Labour Direct Materials Direct expenses Variable overheads K 4. 00 K 2. 00 K 1. 00 K 0. 50 Current level of output in Units Fixed overheads 30, 000 K 60, 000. 00 Calculate the following • Contribution per unit • BEP • Sales revenue at Break Even Point • Units required to make a profit of K 30, 000 • Contribution Ratio • Margin of safety • Percentage increase in profit if volume is increased by 20% • The number of sales needed to maintain the existing profit level if the selling price is reduced by 10%. 47

Changes in components of breakeven point. 48

Example • Selling price per unit • Variable price per unit • Fixed costs • Current profit K 12 K 3 K 45000 K 18000 49
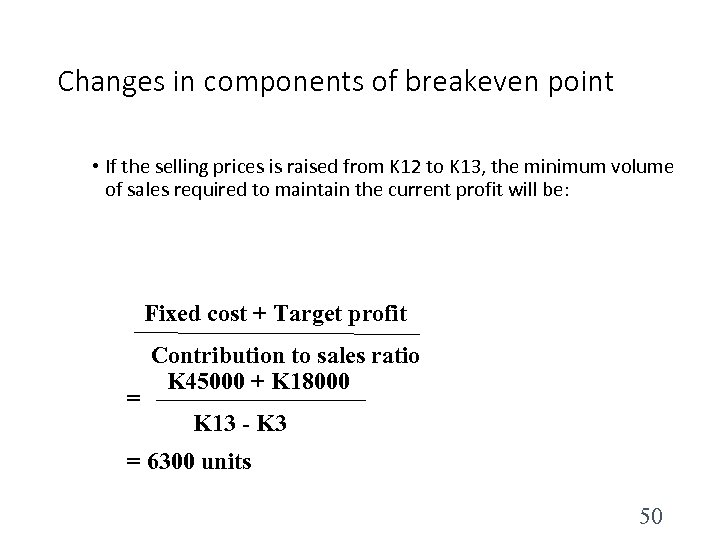
Changes in components of breakeven point • If the selling prices is raised from K 12 to K 13, the minimum volume of sales required to maintain the current profit will be: Fixed cost + Target profit = Contribution to sales ratio K 45000 + K 18000 K 13 - K 3 = 6300 units 50

Changes in components of breakeven point • If the fixed cost fall by K 5000 but the variable costs rise to K 4 per unit, the minimum volume of sales required to maintain the current profit will be: Fixed cost + Target profit Contribution to sales ratio = K 40, 000 + K 18, 000 K 12 - K 4 = 7, 250 units 51

Effect of sales mix on CVP analysis. Unit contribution margin is replaced with contribution margin for a composite unit. A composite unit is made up of specific numbers of each product in proportion to the product sales mix. Sales mix is the ratio of the volumes of the various products.

Computing Multiproduct Break-Even Point A company sells windows and doors. They sell 4 windows for every door. Fixed costs are ZMK 900, 000
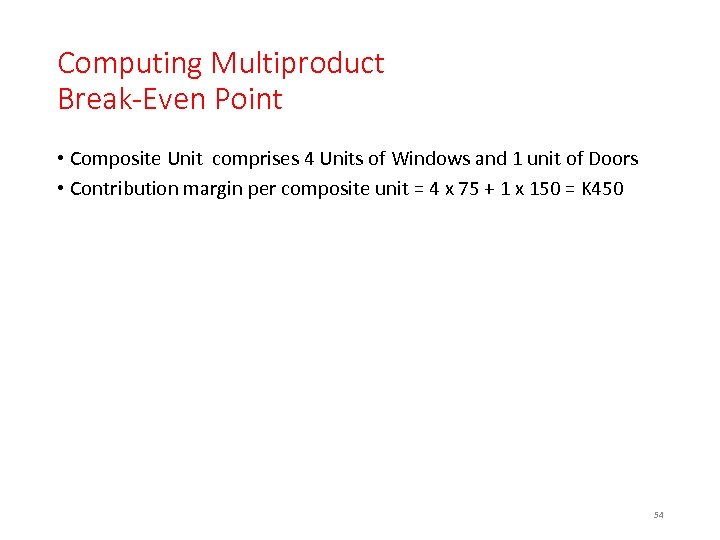
Computing Multiproduct Break-Even Point • Composite Unit comprises 4 Units of Windows and 1 unit of Doors • Contribution margin per composite unit = 4 x 75 + 1 x 150 = K 450 54
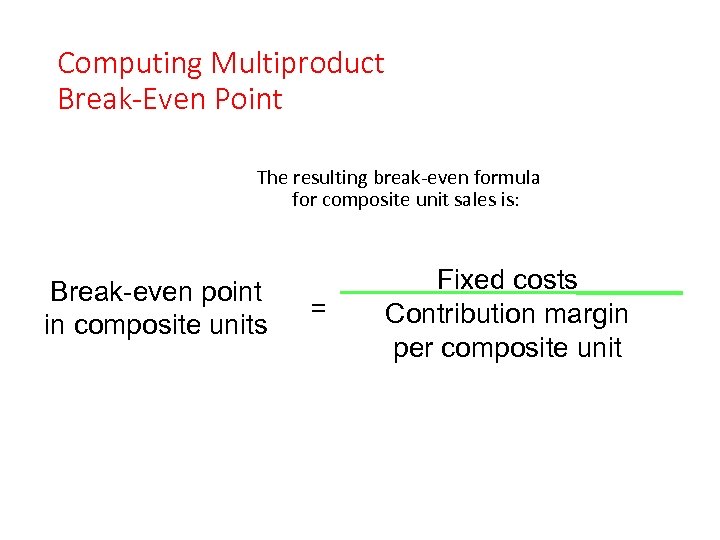
Computing Multiproduct Break-Even Point The resulting break-even formula for composite unit sales is: Break-even point in composite units = Fixed costs Contribution margin per composite unit
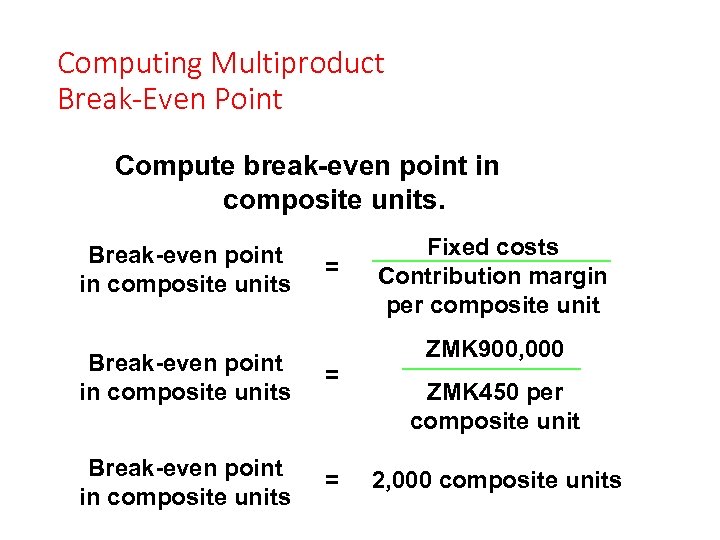
Computing Multiproduct Break-Even Point Compute break-even point in composite units. Break-even point in composite units = Fixed costs Contribution margin per composite unit ZMK 900, 000 ZMK 450 per composite unit 2, 000 composite units
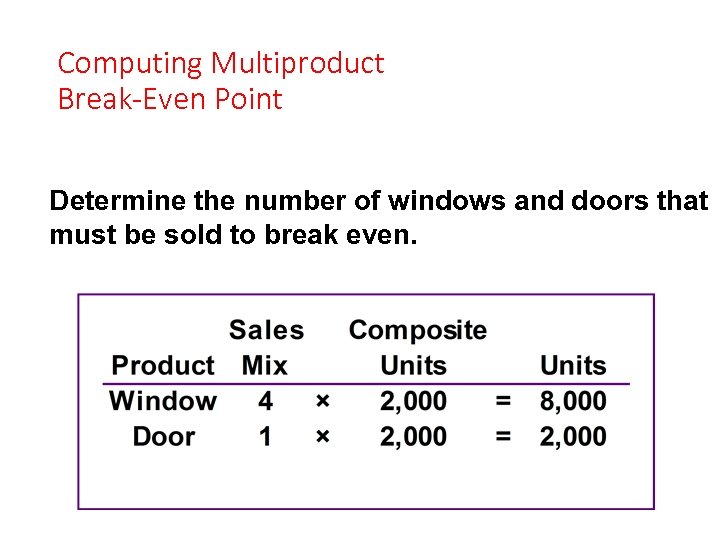
Computing Multiproduct Break-Even Point Determine the number of windows and doors that must be sold to break even.
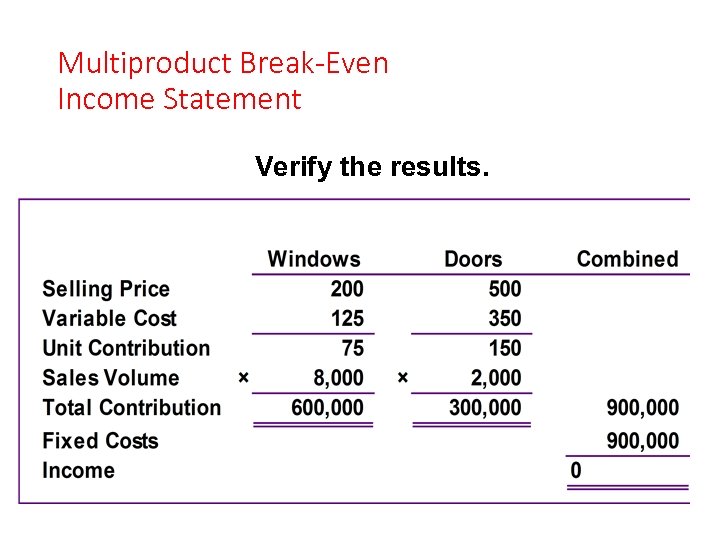
Multiproduct Break-Even Income Statement Verify the results.
Multiproduct Break-Even Analysis Suppose an entity produced two items a and b which had different (avoidable) fixed costs attached to them, such as the Salary of the Product Supervisor. However the entity also has fixed costs which are common to both products and which can only be avoided by not producing either product, such as the salary of the Production Manager. How does one compute the break even point of the entity as a whole? Suppose we use the sample data in the table below
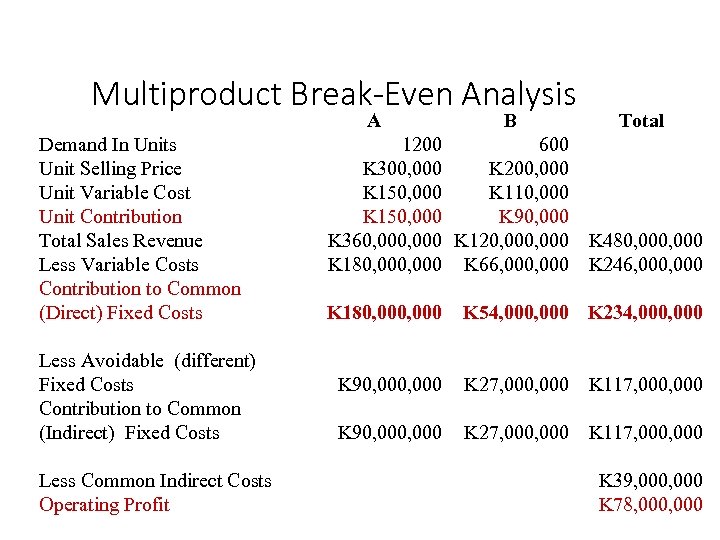
Multiproduct Break-Even Analysis A Demand In Units Unit Selling Price Unit Variable Cost Unit Contribution Total Sales Revenue Less Variable Costs Contribution to Common (Direct) Fixed Costs Less Avoidable (different) Fixed Costs Contribution to Common (Indirect) Fixed Costs Less Common Indirect Costs Operating Profit B 1200 600 K 300, 000 K 200, 000 K 150, 000 K 110, 000 K 150, 000 K 90, 000 K 360, 000 K 120, 000 K 180, 000 K 66, 000 K 180, 000 Total K 480, 000 K 246, 000 K 54, 000 K 234, 000, 000 K 90, 000, 000 K 27, 000, 000 K 117, 000 K 39, 000 K 78, 000
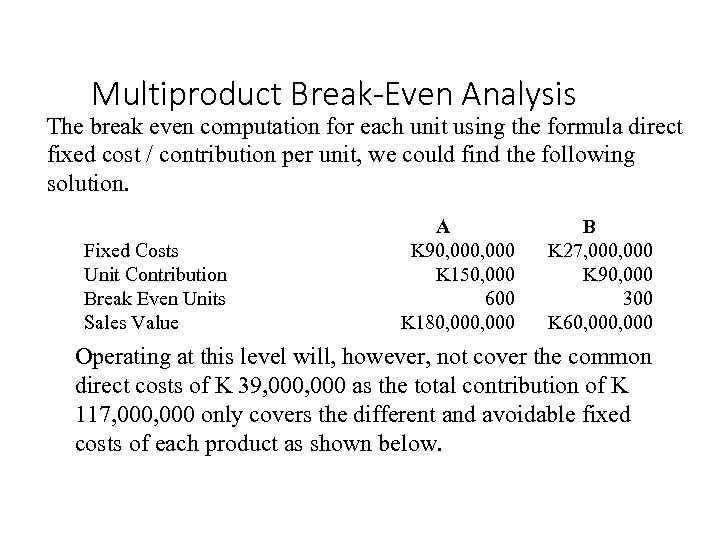
Multiproduct Break-Even Analysis The break even computation for each unit using the formula direct fixed cost / contribution per unit, we could find the following solution. Fixed Costs Unit Contribution Break Even Units Sales Value A K 90, 000 K 150, 000 600 K 180, 000 B K 27, 000 K 90, 000 300 K 60, 000 Operating at this level will, however, not cover the common direct costs of K 39, 000 as the total contribution of K 117, 000 only covers the different and avoidable fixed costs of each product as shown below.
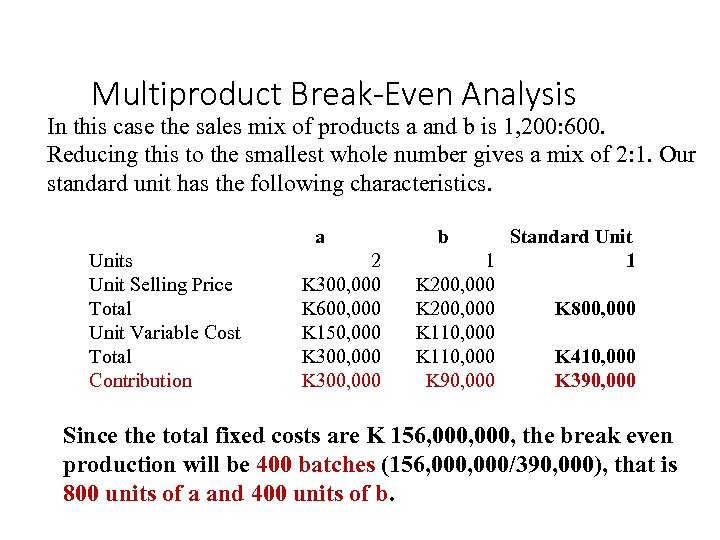
Multiproduct Break-Even Analysis In this case the sales mix of products a and b is 1, 200: 600. Reducing this to the smallest whole number gives a mix of 2: 1. Our standard unit has the following characteristics. a Units Unit Selling Price Total Unit Variable Cost Total Contribution 2 K 300, 000 K 600, 000 K 150, 000 K 300, 000 b Standard Unit 1 1 K 200, 000 K 800, 000 K 110, 000 K 410, 000 K 90, 000 K 390, 000 Since the total fixed costs are K 156, 000, the break even production will be 400 batches (156, 000/390, 000), that is 800 units of a and 400 units of b.
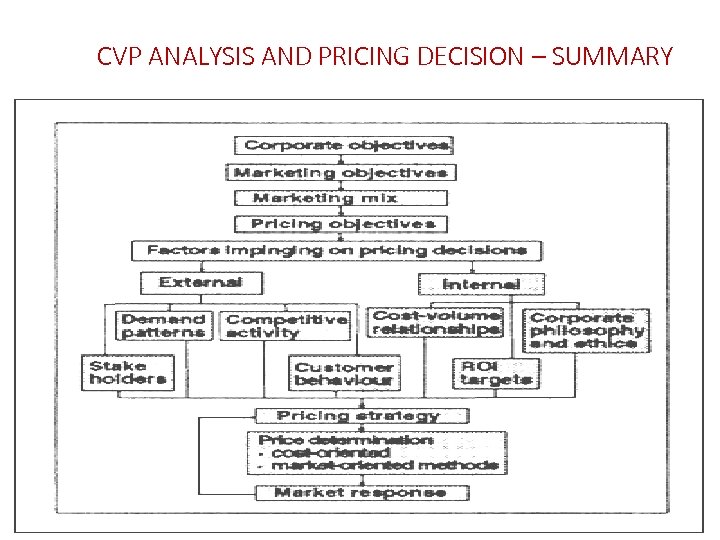
CVP ANALYSIS AND PRICING DECISION – SUMMARY

CVP ANALYSIS CVP analysis involves the analysis of how total costs, total revenues and total profits are related to sales volume, and is therefore concerned with predicting the effects of changes in costs and sales volume on profit. The technique used carefully may be helpful in the following situations: a) Budget planning. The volume of sales required to make a profit (breakeven point) and the 'safety margin' for profits in the budget can be measured. b) Pricing and sales volume decisions. c) Sales mix decisions, to determine in what proportions each product should be sold. d) Decisions that will affect the cost structure and production capacity of the company.
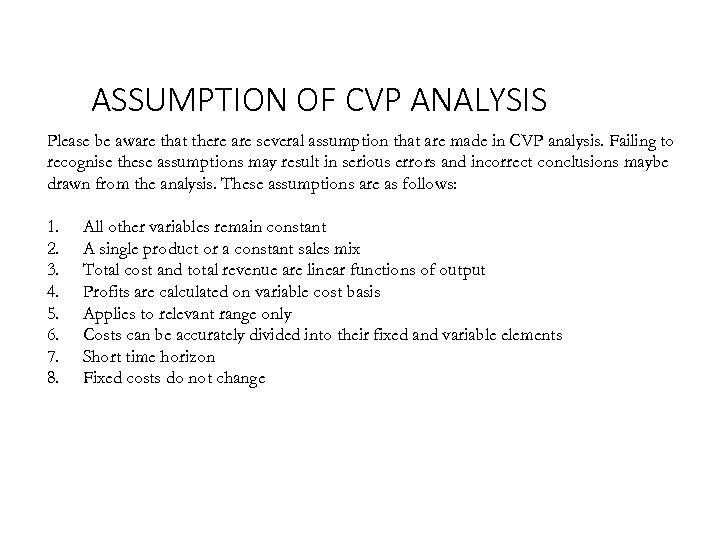
ASSUMPTION OF CVP ANALYSIS Please be aware that there are several assumption that are made in CVP analysis. Failing to recognise these assumptions may result in serious errors and incorrect conclusions maybe drawn from the analysis. These assumptions are as follows: 1. 2. 3. 4. 5. 6. 7. 8. All other variables remain constant A single product or a constant sales mix Total cost and total revenue are linear functions of output Profits are calculated on variable cost basis Applies to relevant range only Costs can be accurately divided into their fixed and variable elements Short time horizon Fixed costs do not change

Limitation of breakeven point. 66
Limitations of breakeven analysis • Breakeven analysis assumes that fixed cost, variable costs and sales revenue behave in linear manner. • However, some overhead costs may be stepped in nature. The straight sales revenue line and total cost line tent to curve beyond certain level of production 67
Limitations of breakeven analysis • It is assumed that all production is sold. The breakeven chart does not take the changes in stock level into account • Breakeven analysis can provide information for small and relatively simple companies that produce same product. It is not useful for the companies producing multiple products 68

Break even Analysis - Exercise Chongwe Real Estates Company was established in 2013 and business looks to be sluggish at the moment. Management are however hopeful that business will pick with the improvement in the income levels among the local population. The company is currently operating below its breakeven point and is targeting to breakeven within six months. The company specializes in residential accommodation in suburbs of Lusaka East and has available 300 housing units of 3 bed rooms each located in Silverest area. The following information has been provided relating to the units. • Maximum Number of Housing Units to let per annum: 300 • Rental Price per house per month: K 6, 500. 00 • Fixed Overhead Costs per annum: K 2, 800, 000. 00 • The contribution sales ratio (C/S): 20% Required: a) Calculate the Break-Even number of housing units. b) Calculate the rental income at break-even point for the company. c) Calculate the housing units required to be rent out to make a target profit of 10% of annual Fixed Costs. d) Briefly explain the term Margin of Safety in this particular case. e) Advise management on whether it should add more housing units than it currently has at the moment and give your reasons. 69

Short Term decision making • Introduction • Short term decision making is involved with making the best use of existing resources • Short-term decision making is based on marginal costing techniques • Requires an examination of how costs and/or revenues will change as a result of any decision • Long term decision making is concerned with long term investments and involves capital appraisal techniques/capital budgeting: • E. g. new product development • Investment in new equipment • Lease or buy decisions 70

Short Term decision making • Outline: • • • Pricing special orders Maximising profit from scarce resources Multiple scarce resources Closing Down a department or product Product Mix Make or buy decisions 71

Short Term decision making • Pricing special orders • Assuming that fixed costs will remain unchanged when we use spare capacity for a special order the decision is to: • Only consider the effects of the order on sales revenue and variable costs • Therefore consider the change in Contribution • If the contribution is increased as a result of the special order then undertake the special order. 72

Short Term decision making • Pricing special orders • Example: • A customer has offered your company a special contract to make equipment for K 20, 000. • The provisional costing to make the equipment has been prepared by the accountant as follows: • Material – K 5, 000 • Direct labour(2, 000 hrs) – K 10, 000 • Variable overhead – K 4, 000 • Allocated fixed overheads - K 8, 000 • Required • Advice your Management as to whether the company should accept the special order or not • Justify your answer 73

Short Term decision making • Maximising profit from scarce resources • Consider the above example • Additional information is that it will be necessary to divert the labour from casual work which takes 2, 000 hours and yields a contribution of K 7. 00 per hour. • Required • Advice your Management as to whether the company should accept the special order or not • Justify your answer 74

Short Term decision making • Maximising profit from scarce resources • Scarce resources can be : • • • Space Equipment Skilled labour Raw materials Working capital 75

Short Term decision making • Maximising profit from scarce resources • When resources are scarce, we need to know how best to allocate them to product lines • In case of Only one scarce resource: • Rank products in order of the contribution they earn per unit of scarce resource 76
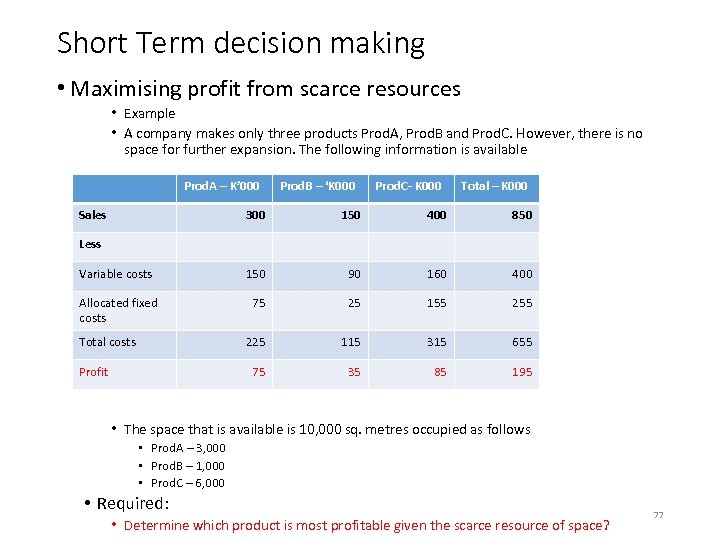
Short Term decision making • Maximising profit from scarce resources • Example • A company makes only three products Prod. A, Prod. B and Prod. C. However, there is no space for further expansion. The following information is available Prod. A – K’ 000 Sales Prod. B – ‘K 000 Prod. C- K 000 Total – K 000 300 150 400 850 Variable costs 150 90 160 400 Allocated fixed costs 75 25 155 225 115 315 655 75 35 85 195 Less Total costs Profit • The space that is available is 10, 000 sq. metres occupied as follows • Prod. A – 3, 000 • Prod. B – 1, 000 • Prod. C – 6, 000 • Required: • Determine which product is most profitable given the scarce resource of space? 77
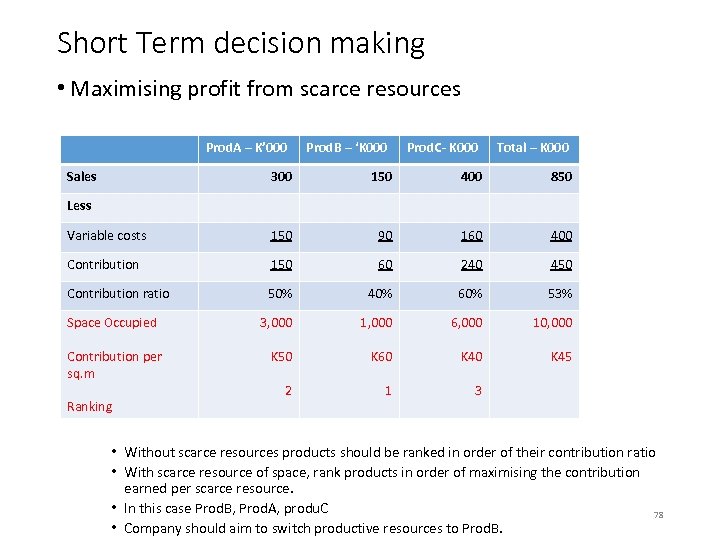
Short Term decision making • Maximising profit from scarce resources Prod. A – K’ 000 Sales Prod. B – ‘K 000 Prod. C- K 000 Total – K 000 300 150 400 850 Variable costs 150 90 160 400 Contribution 150 60 240 450 Contribution ratio 50% 40% 60% 53% 3, 000 1, 000 6, 000 10, 000 K 50 K 60 K 45 2 1 3 Less Space Occupied Contribution per sq. m Ranking • Without scarce resources products should be ranked in order of their contribution ratio • With scarce resource of space, rank products in order of maximising the contribution earned per scarce resource. • In this case Prod. B, Prod. A, produ. C 78 • Company should aim to switch productive resources to Prod. B.

Short Term decision making • Multiple scarce resources • In case of multiple scarce resources: • Identify contributions from each product line for each scarce resource • Use linear programming to techniques to decide how many of each product to make to maximize the contributions 79

Short Term decision making • Closing Down a department or product • Consider only relevant costs • In this case the variable costs • If there are specific fixed costs related to a product, take these into consideration • Decision is not to close when a department or product is making a contribution to the overall company total contribution 80

Short Term decision making Closing Down a product or department Product A B C Total ZMK'000 60. 00 ZMK'000 120. 00 ZMK'000 90. 00 ZMK'000 270. 00 Variable costs Apportioned fixed costs 30. 00 12. 00 75. 00 24. 00 75. 00 180. 00 54. 00 Total costs 42. 00 18. 00 99. 00 21. 00 93. 00 -3. 00 234. 00 36. 00 Sales Less Profit Required • Advise management whether Product C should be discontinued or not. • Justify your answer 81

Short Term decision making • Product Mix • Often managers need to make a decision regarding changes to the product mix in terms of sales volumes • Approach: • Calculate the contributions per each product mix and select the mix that gives the highest contribution 82

Short Term decision making • Make or buy decisions • • May also involve outsource or in-house decisions Compare the costs of buy-in v make or outsource v in-house Only relevant costs of variable costs and direct fixed costs are considered Choose the one with least cost 83

Short Term decision making • Summary • Marginal costing is most useful technique for managers to use in short-tem decision making • Example of short-term decision making include: • • • Pricing special orders Maximising profit from scarce resources Multiple scarce resources Closing Down a department or product Product Mix Make or buy decisions • The concept of contribution is at the heart of the decision making process • Sometimes direct fixed costs specific to a product are also considered relevant • Common fixed costs are never relevant in the above short-term decision making 84

Practice questions 1. Compare and contract ‘profit’ with ‘contribution’ as measures of product profitability giving examples. 2. Compare and contrast the following pricing techniques: • Full cost pricing • Rate of return pricing • Marginal cost pricing 3. Construct a simple example to explain how you would attempt to maximize the profit of your company given that there is limited labour available. 4. List and describe the assumptions made under the break-even analysis 5. Critically analyse the limitations of the break-even analysis 6. Managers are often faced with making decisions that are shortterm or long-term. Discuss the different techniques used in shortterm decision making v those used in long term decision making 85
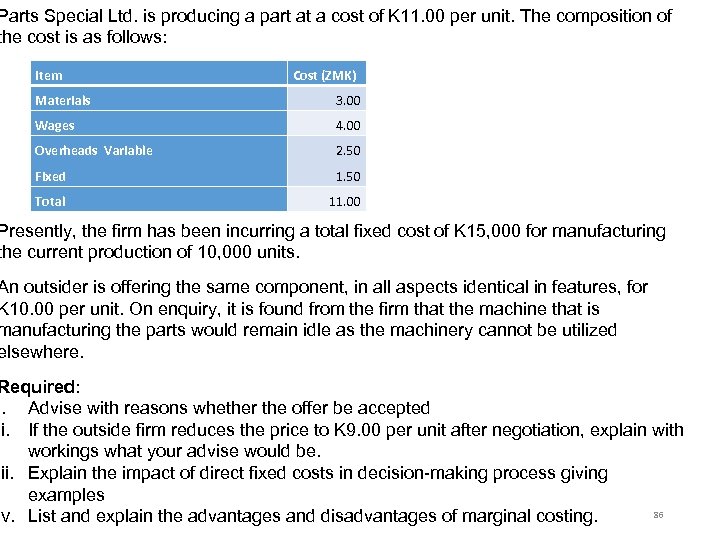
Parts Special Ltd. is producing a part at a cost of K 11. 00 per unit. The composition of the cost is as follows: Item Cost (ZMK) Materials 3. 00 Wages 4. 00 Overheads Variable 2. 50 Fixed 1. 50 Total 11. 00 Presently, the firm has been incurring a total fixed cost of K 15, 000 for manufacturing the current production of 10, 000 units. An outsider is offering the same component, in all aspects identical in features, for K 10. 00 per unit. On enquiry, it is found from the firm that the machine that is manufacturing the parts would remain idle as the machinery cannot be utilized elsewhere. Required: i. Advise with reasons whether the offer be accepted ii. If the outside firm reduces the price to K 9. 00 per unit after negotiation, explain with workings what your advise would be. iii. Explain the impact of direct fixed costs in decision-making process giving examples 86 iv. List and explain the advantages and disadvantages of marginal costing.
1816162d77a1ba1068f5a69959b89e8c.ppt