Everyday examples of the Joule the energy

Everyday examples of the Joule • the energy required to lift a small apple one meter straight up. • the energy released when that same apple falls one meter to the ground. • the energy released as heat by a quiet person, every hundredth of a second. • the energy required to heat one gram of dry, cool air by 1 degree Celsius. • one hundredth of the energy a person can receive by drinking a drop of beer. • the kinetic energy of an adult human moving a distance of about a hand-span every second.

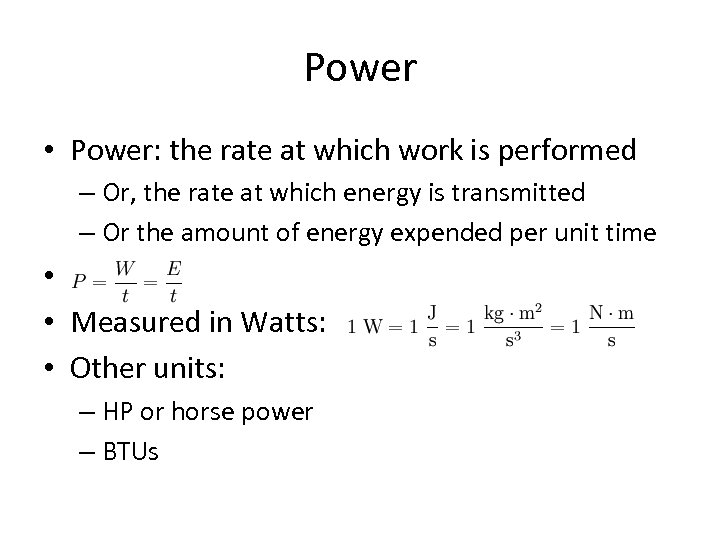Power • Power: the rate at which work is performed – Or, the rate at which energy is transmitted – Or the amount of energy expended per unit time • • Measured in Watts: • Other units: – HP or horse power – BTUs

Horse power • Arose as a result of the invention of the steam engine. People needed a way to compare the power of a steam engine to that of the horses it was replacing. • Confusing unit there are too many different definitions!

BTU • BTU: British Thermal Units - an energy unit – the amount of heat required to raise the temperature of one pound of liquid water by one degree from 60° to 61°Fahrenheit at a constant pressure of one atmosphere • Used in the power, steam generation, heating and air conditioning industries and the energy content of fuels. • However, BTU is often used as a unit of power, where BTU/hour is often abbreviated BTU. – So you need to watch the context!

Back to Watts…. . • A human climbing a flight of stairs is doing work at a rate of about 200 watts. • A typical household incandescent light bulb uses electrical energy at a rate of 25 to 100 watts, while compact fluorescent lights typically consume 5 to 30 watts. • A 100 Watt light bulb consumes energy at the rate of 100 joules/second. • After 1 hour, this light bulb uses 100 watt-hours • 1 kilowatt (kw) is 1000 Watts

Examples • In a certain room in your house, you use a 100 W light bulb. This light is on for 5 hours every day. How much energy does it use? • 1 W = 1 J/s and there are 5 h x 60 min/hour x 60 sec/min = 18, 000 s in 5 hours so the total energy used is 100 j/s *18000 s = 1. 8 x 10 6 J. • Lets assume the same lighting level can be achieved using a 30 W compact florescent bulb. How much energy is used by the compact florescent bulb?

Examples • Total energy = 30 j/s x 18000 s = 5. 4 x 105 j. • So how much energy is saved every day using the compact florescent bulb? Take the difference between the energy used by the two different light bulbs: 1. 8 x 10 6 j - 5. 4 x 105 j = 1. 3 x 106 j. • Lets look at this in something you might be able to relate to better than joules---dollars!

Example continued • After 5 hours, our 100 W light bulb uses 500 Watt-hours, or 0. 5 Kwh. The 30 W bulb will use 150 Watt hours or 0. 15 Kwh. • Assume electricity costs 11 cents/Kwh (average cost in the US in April 2008). So it costs. 5 Kw. H x 11 cents/Kwh = 5. 5 cents every day to run the 100 W light bulb and 0. 15 Kwh x 11 cents = 1. 65 cents every day to run the compact florescent.

Example continued • So in a year, the 100 W light bulb costs you 5. 5 cents/day X 365 days/year = $20. 00 and the 30 W bulb costs you 1. 65 cents/day x 365 days/year = $5. 50.

Types of Energy: kinetic and potential Energy Kinetic energy of a moving object KE=1/2 mv 2 Potential Energy – Energy stored in a system, for example an object of mass m, a distance h above the surface of the earth has a potential energy given by mgh. g is the acceleration due to gravity = 9. 8 m/s 2

More examples of potential energy Another example is a spring, compressed a distance x from its equilibrium point has a potential energy 1/2 kx 2, where k is the spring constant, a property of the spring.

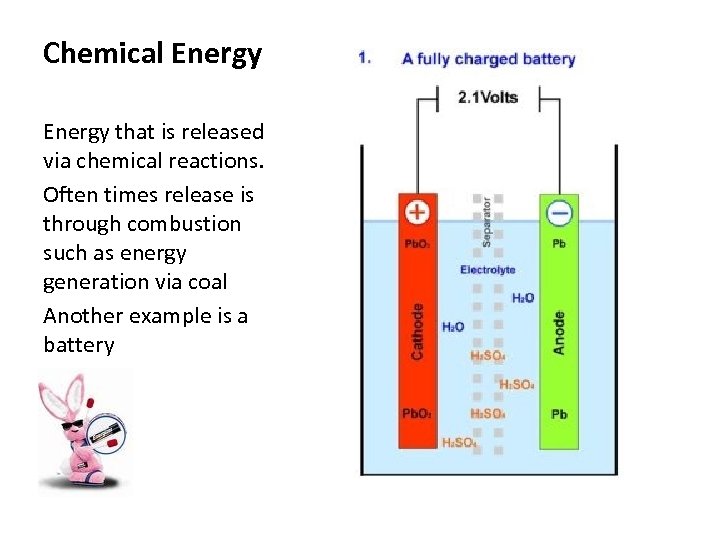Chemical Energy that is released via chemical reactions. Often times release is through combustion such as energy generation via coal Another example is a battery

Heat Energy associated with the random motions of the molecules in a medium. Measured by temperature • Temperature Scales: • Fahrenheit – based on the height of liquid (often mercury or alcohol) in a glass tube. • Celsius – another scale using height of liquid in a tube • Kelvin-absolute scale – True measure of energy

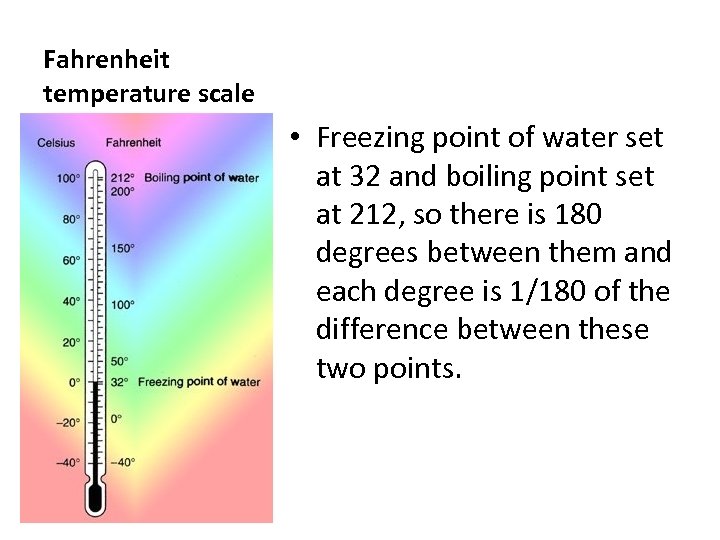Fahrenheit temperature scale • Freezing point of water set at 32 and boiling point set at 212, so there is 180 degrees between them and each degree is 1/180 of the difference between these two points.

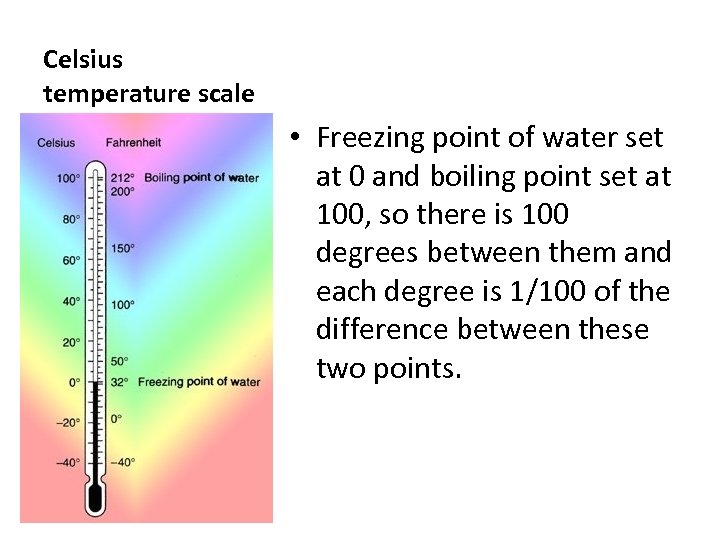Celsius temperature scale • Freezing point of water set at 0 and boiling point set at 100, so there is 100 degrees between them and each degree is 1/100 of the difference between these two points.

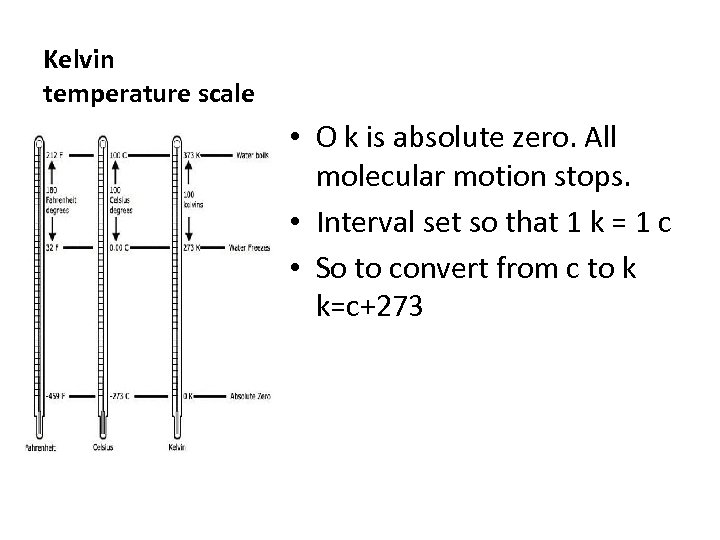Kelvin temperature scale • O k is absolute zero. All molecular motion stops. • Interval set so that 1 k = 1 c • So to convert from c to k k=c+273

Mass Energy • E = mc 2 • Energy and mass are equivalent • C = 3 x 108 m/s. • A big number and its squared! So even if m is small, E is big. • A small mass, converted to energy, gives a lot of energy!

Example