Equation-Based Modeling Copyright 2013 COMSOL COMSOL Multiphysics

Equation-Based Modeling © Copyright 2013 COMSOL, COMSOL Multiphysics, Capture the Concept, COMSOL Desktop, and Live. Link are either registered trademarks or trademarks of COMSOL AB. All other trademarks are the property of their respective owners, and COMSOL AB and its subsidiaries and products are not affiliated with, endorsed by, sponsored by, or supported by those trademark owners. For a list of such trademark owners, see http: //www. comsol. com/tm

Multiphysics and Single-Physics Simulation Platform • Mechanical, Fluid, Electrical, and Chemical Simulations • Multiphysics - Coupled Phenomena – Two or more physics phenomena that affect each other with no limitation on • which combinations • how many combinations • Single Physics – One integrated environment – different physics and applications – One day you work on Heat Transfer, next day Structural Analysis, then Fluid Flow, and so on – Same workflow for any type of modeling • Enables cross-disciplinary product development and a unified simulation platform

Highly Customizable and Adaptable • Create your own multiphysics couplings • Customize material properties and boundary conditions – Type in mathematical expressions, combine with look-up tables and function calls • User-interfaces for differential and algebraic equations • Parameterize on material properties, boundary conditions, geometric dimensions, and more • High-Performance Computing (HPC) – Multicore & Multiprocessor: Included with any license type – Clusters & Cloud: With floating network licenses

The COMSOL Multiphysics® 4. 3 b Product Suite

Equation-Based Modeling • Partial Differential Equations (PDEs) – PDE Interfaces – Boundary Conditions • Ordinary Differential Equations (ODEs) – Global ODEs – Distributed ODEs • Algebraic Equations – Global algebraic equations – Distributed algebraic equations

When is Equation-Based Modeling Needed? • Try to avoid equation-based modeling if possible! – Using built-in physics interfaces enables ready-made postprocessing variables and other tools for faster model setup with much lower risk of human error • Applications that previously required equation-based modeling but now has a dedicated physics interface: – Fluid-Structure Interaction (Structural Mechanics Module, MEMS Module) – Surface adsorption and reactions (Chemical Reaction Engineering Module, Plasma Module) – Shell-Acoustics and Piezo-Acoustics (Acoustics Module) – Thermoacoustics (Acoustics Module) – and many more…

When is Equation-Based Modeling Needed? • Try to avoid equation-based modeling if possible! • But: we don’t have every imaginable physics equation builtinto COMSOL (yet!). So there is sometimes a need for custom modeling.

Custom-Modeling in COMSOL • COMSOL Multiphysics allows you to model with PDEs or ODEs directly: – Use one of the equation-template user interfaces • You do *not* need to write “user-subroutines” in COMSOL to implement your own equation! – Benefit: COMSOL’s nonlinear solver gets all the nonlinear info with gradients and all. Faster and more robust convergence.

Customization Approaches • Four modeling approaches: 1. Ready-made physics interfaces 2. First principles with the equation templates 3. Start with ready-made physics interface and additional terms. 4. Start with a ready-made physics interface and add your own separate equation (PDE, ODE) to represent physics that is not already available as a ready-made application mode • Also: – The Physics Builder lets you create your own user interfaces that hides the mathematics for your colleagues and customers

PDEs

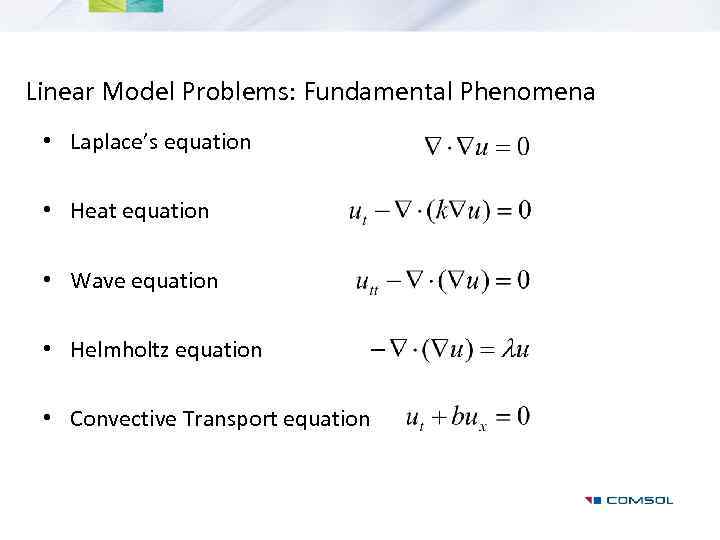
Linear Model Problems: Fundamental Phenomena • Laplace’s equation • Heat equation • Wave equation • Helmholtz equation • Convective Transport equation

COMSOL PDE Modes: Graphical User Interfaces • Coefficient form • General form • Weak form • All these can be used for scalar equations or systems • Which to use? – Whichever is more convenient for you and your simulation needs

Coefficient Form inside domain on boundary • Coefficient Matching Example: Poisson’s equation inside subdomain on subdomain boundary Implies c=f=h=1 and all other coefficients are 0.

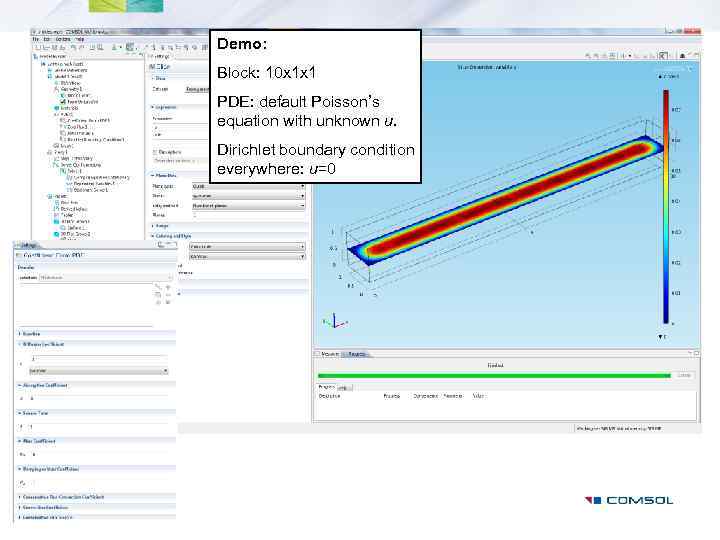
Demo: Block: 10 x 1 x 1 PDE: default Poisson’s equation with unknown u. Dirichlet boundary condition everywhere: u=0

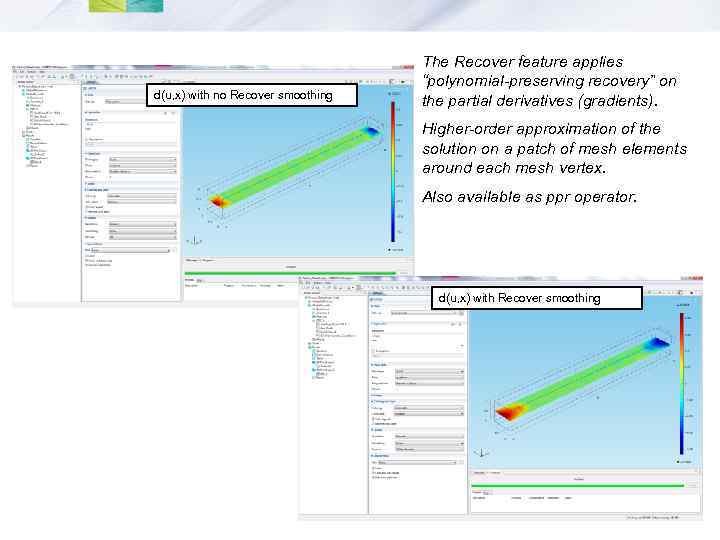
d(u, x) with no Recover smoothing The Recover feature applies “polynomial-preserving recovery” on the partial derivatives (gradients). Higher-order approximation of the solution on a patch of mesh elements around each mesh vertex. Also available as ppr operator. d(u, x) with Recover smoothing

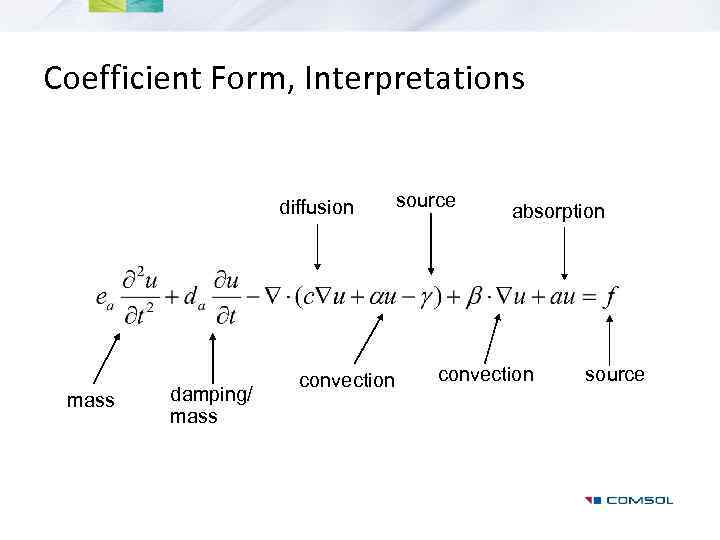
Coefficient Form, Interpretations diffusion mass damping/ mass convection source absorption convection source

Coefficient Form, Structural Analysis Wave Equation elastic stress mass damped mass initial/thermal stress body force (gravitation) density damping coefficient stress, u= displacement vector stiffness, “spring constant”

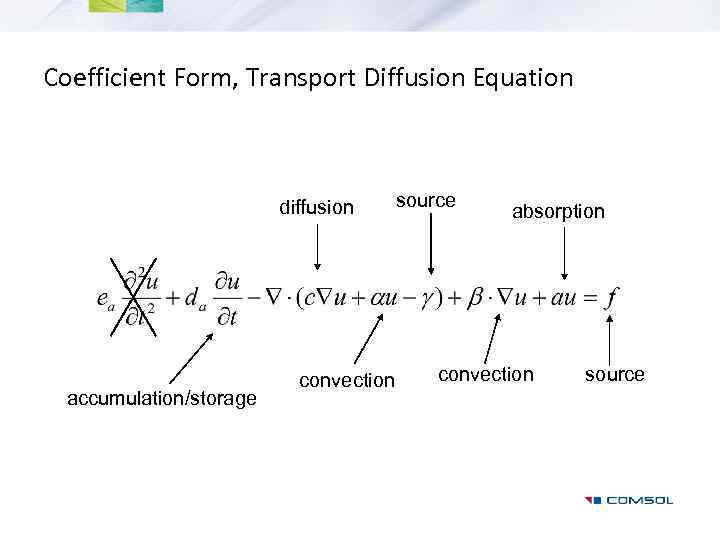
Coefficient Form, Transport Diffusion Equation diffusion accumulation/storage convection source absorption convection source

Coefficient Form, Steady-State Equation

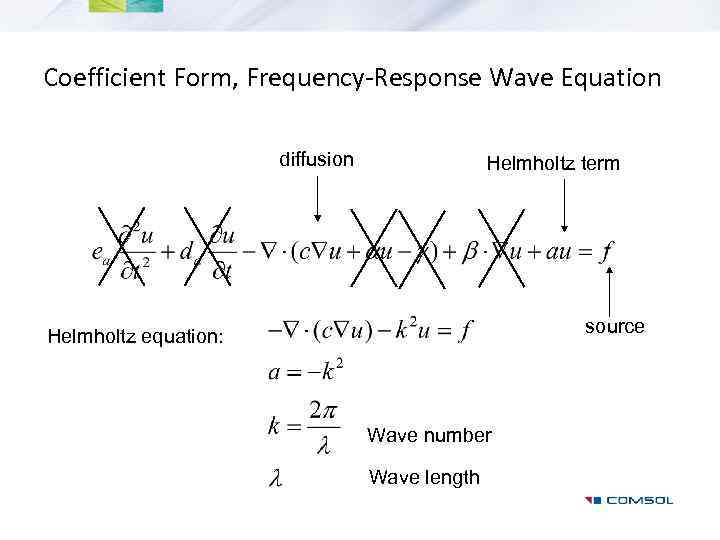
Coefficient Form, Frequency-Response Wave Equation diffusion Helmholtz term source Helmholtz equation: Wave number Wave length

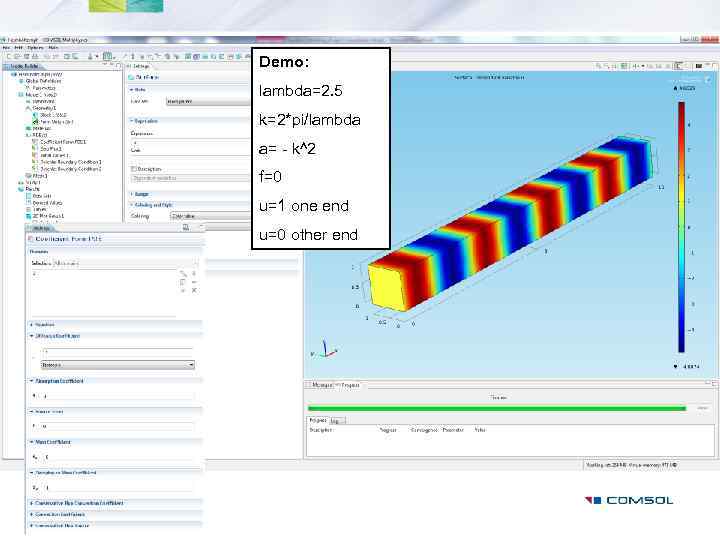
Demo: lambda=2. 5 k=2*pi/lambda a= - k^2 f=0 u=1 one end u=0 other end

Complex Arithmetics • Can compute: real(w) imag(w) abs(w) arg(w) conj(w)

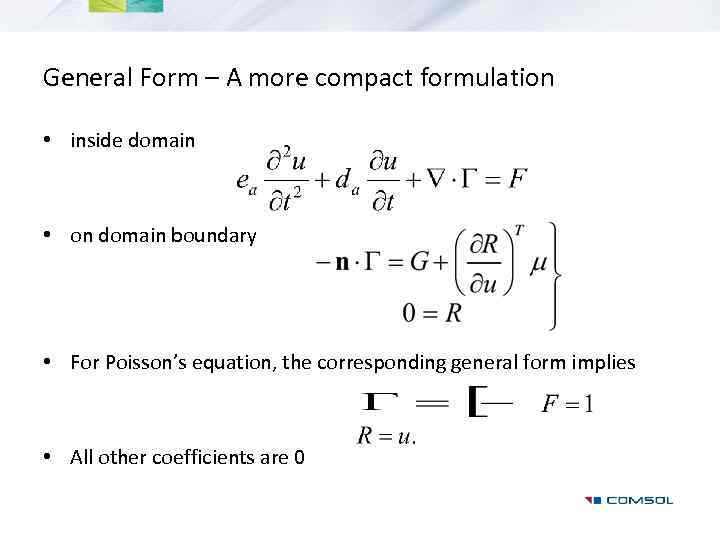
General Form – A more compact formulation • inside domain • on domain boundary • For Poisson’s equation, the corresponding general form implies • All other coefficients are 0

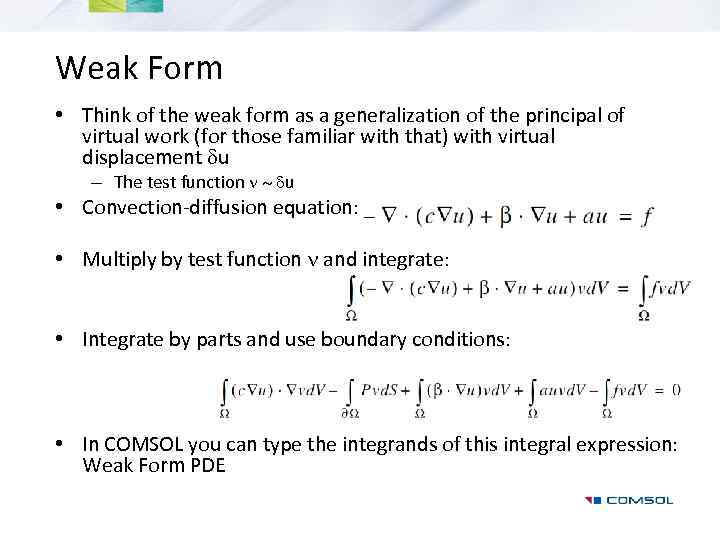
Weak Form • Think of the weak form as a generalization of the principal of virtual work (for those familiar with that) with virtual displacement du – The test function n ~ du • Convection-diffusion equation: • Multiply by test function n and integrate: • Integrate by parts and use boundary conditions: • In COMSOL you can type the integrands of this integral expression: Weak Form PDE

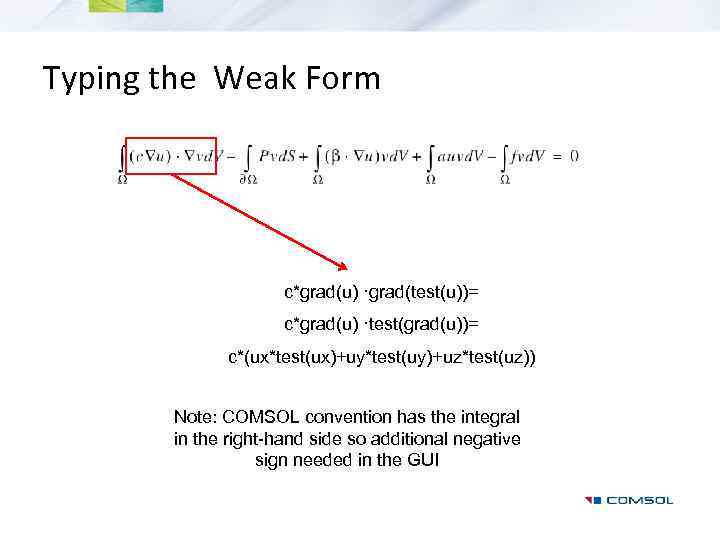
Typing the Weak Form c*grad(u) ·grad(test(u))= c*grad(u) ·test(grad(u))= c*(ux*test(ux)+uy*test(uy)+uz*test(uz)) Note: COMSOL convention has the integral in the right-hand side so additional negative sign needed in the GUI

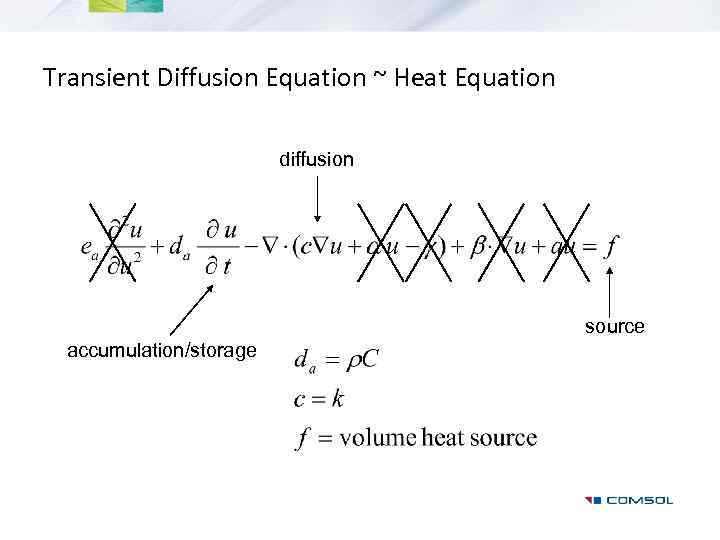
Transient Diffusion Equation ~ Heat Equation diffusion source accumulation/storage

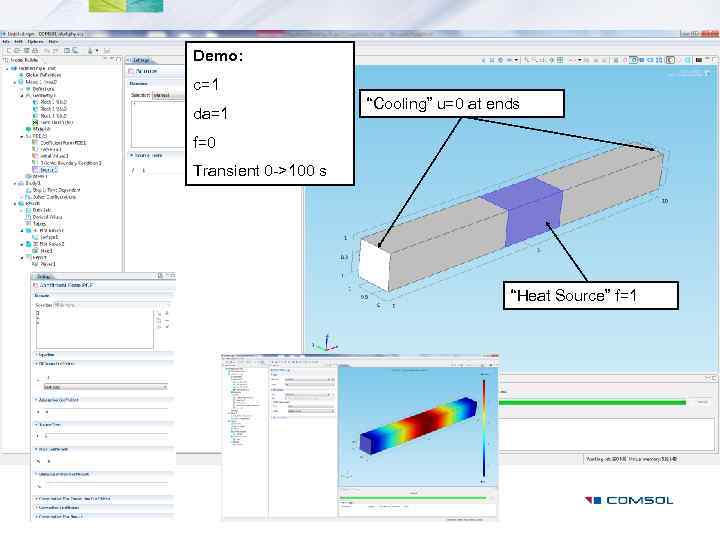
Demo: c=1 da=1 “Cooling” u=0 at ends f=0 Transient 0 ->100 s “Heat Source” f=1

PDEs+ODEs

Transient Diffusion Equation + ODE What if we wish to measure the global accumulation of “heat” over time?

Transient Diffusion Equation + ODE What if we wish to measure the global accumulation of “heat” over time? => This is a Global ODE in the global state variable w

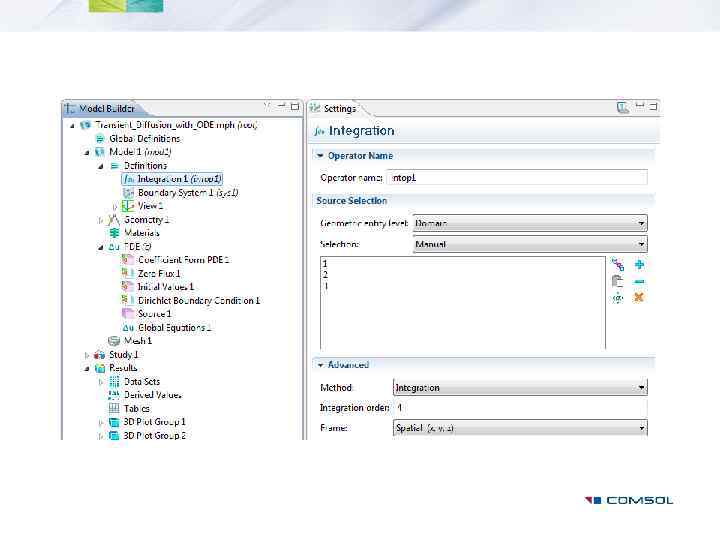
Demo: Global Equation ODE Same time-dependent problem as earlier Time-dependent 0 -100 Volume integration of u ODE: wt-U

PDEs + Distributed ODEs

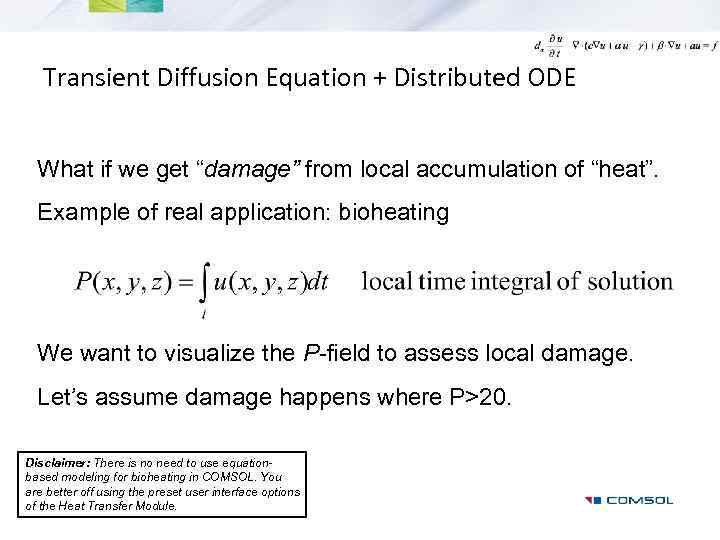
Transient Diffusion Equation + Distributed ODE What if we get “damage” from local accumulation of “heat”. Example of real application: bioheating We want to visualize the P-field to assess local damage. Let’s assume damage happens where P>20. Disclaimer: There is no need to use equationbased modeling for bioheating in COMSOL. You are better off using the preset user interface options of the Heat Transfer Module.

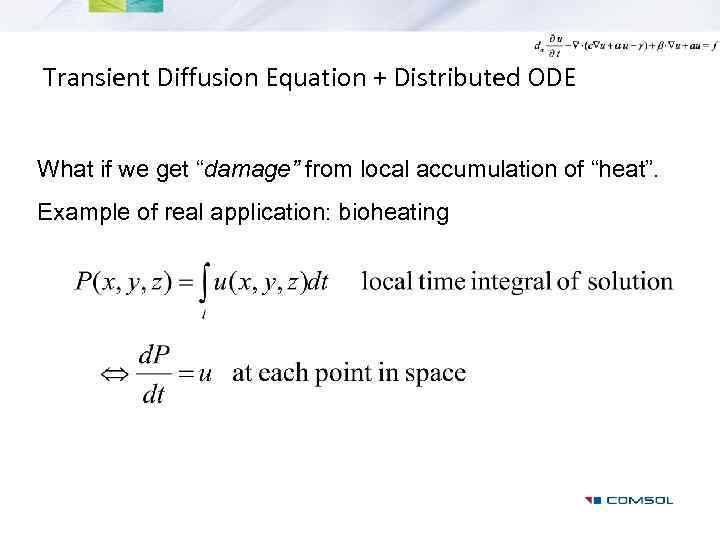
Transient Diffusion Equation + Distributed ODE What if we get “damage” from local accumulation of “heat”. Example of real application: bioheating

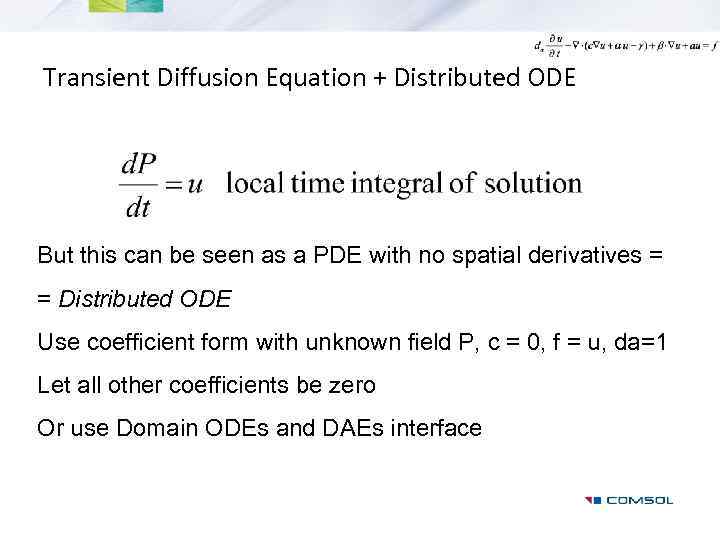
Transient Diffusion Equation + Distributed ODE But this can be seen as a PDE with no spatial derivatives = = Distributed ODE Use coefficient form with unknown field P, c = 0, f = u, da=1 Let all other coefficients be zero Or use Domain ODEs and DAEs interface

Demo: Distributed ODE Same time-dependent problem as earlier Time-dependent 0 -100 Volume integration of u ODE: wt-U Volume where P>20 and we get damage

Distributed Algebraic Equations

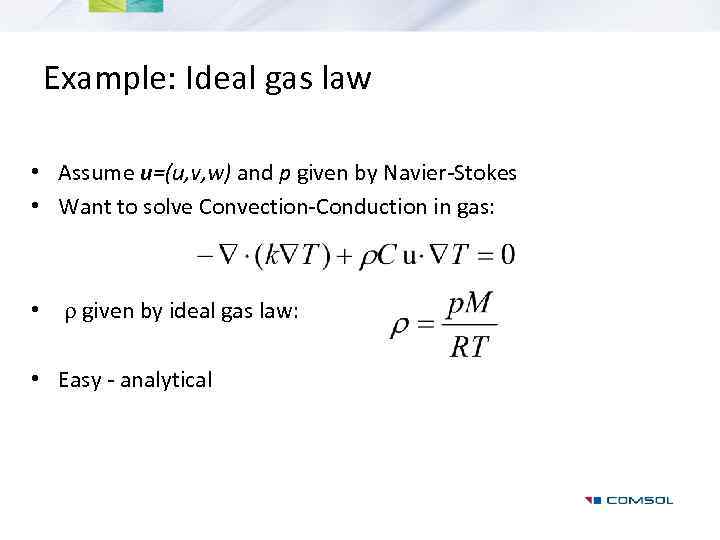
Example: Ideal gas law • Assume u=(u, v, w) and p given by Navier-Stokes • Want to solve Convection-Conduction in gas: • r given by ideal gas law: • Easy - analytical

Example: Non-ideal gas law • Assume u=(u, v, w) and p given by Navier-Stokes • Want to solve Convection-Conduction in gas: • • r given by non-ideal gas law: Needed for high molecular weight at very high pressures Difficult – implicit equation How to proceed?

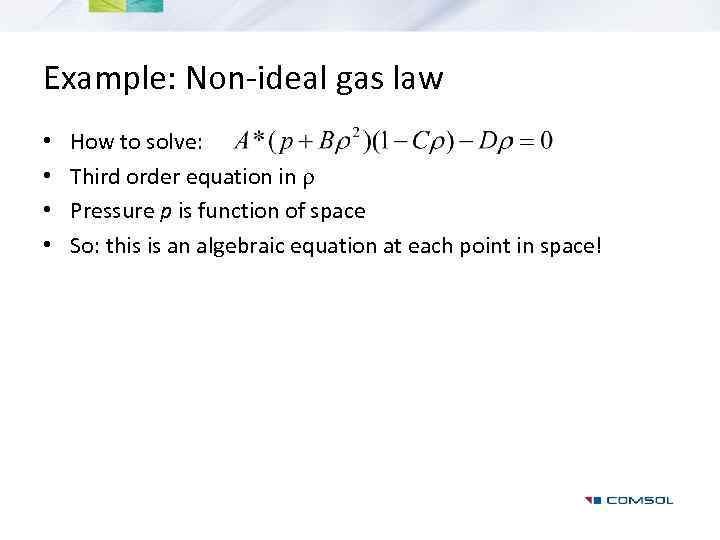
Example: Non-ideal gas law • • How to solve: Third order equation in r Pressure p is function of space So: this is an algebraic equation at each point in space!

Distributed Algebraic Equation • So: this is an algebraic equation at each point in space • See as PDE with no space or time derivatives! – A*(p+B*u^2)*(1 -C*u)-D*u – Here we let: A=1, B=2, C=3, D=4, p=x*y • How: Put the entire equation in the source (f) term and zero out the rest • Or, user interface for Domain ODEs and DAEs

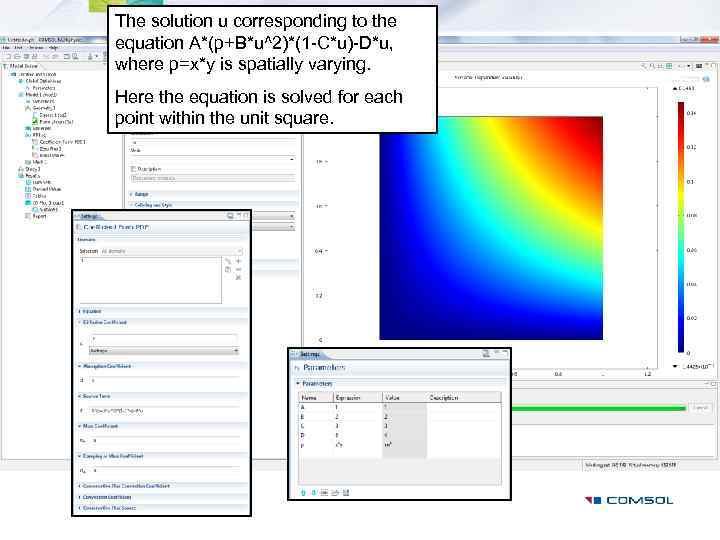
The solution u corresponding to the equation A*(p+B*u^2)*(1 -C*u)-D*u, where p=x*y is spatially varying. Here the equation is solved for each point within the unit square.

Distributed Algebraic Equation • • • What about nonlinear equations with multiple solutions? Which solution do you get? For simplicity, consider the equation (u-2)^2 -p=0, where p is a constant This can be entered as earlier with an f=(u-2)^2 -p The solution is easy to get analytically: u=2±sqrt(p) The solution you get will depend on the Initial Guess given by the PDE Physics Interface • If we let p=x*y and let our modeling region be the unit square, then at (x, y)=(0, 0) we should get the unique solution u=2 but at (x, y)=(1, 1) we get 1 or 3 depending on our starting guess (and also the convergence region of the solver). See next slide.

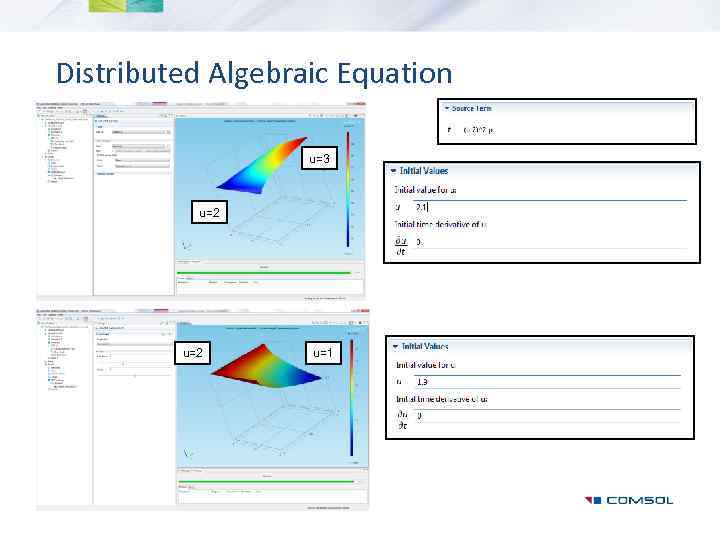
Distributed Algebraic Equation u=3 u=2 u=1

End of Presentation