Ea. ES 455 -11 1 Contents •




















- Размер: 2.6 Mегабайта
- Количество слайдов: 37
Описание презентации Ea. ES 455 -11 1 Contents • по слайдам
 Ea. ES 455 -11 1 Contents • Introduction • Sedimentology – concepts • Fluvial environments • Deltaic environments • Coastal environments • Offshore marine environments • Sea-level change • Sequence stratigraphy – concepts • Marine sequence stratigraphy • Nonmarine sequence stratigraphy • Basin and reservoir modeling • Reflection
Ea. ES 455 -11 1 Contents • Introduction • Sedimentology – concepts • Fluvial environments • Deltaic environments • Coastal environments • Offshore marine environments • Sea-level change • Sequence stratigraphy – concepts • Marine sequence stratigraphy • Nonmarine sequence stratigraphy • Basin and reservoir modeling • Reflection
 Ea. ES 455 -11 2 Basin and reservoir modeling What is a model?
Ea. ES 455 -11 2 Basin and reservoir modeling What is a model?
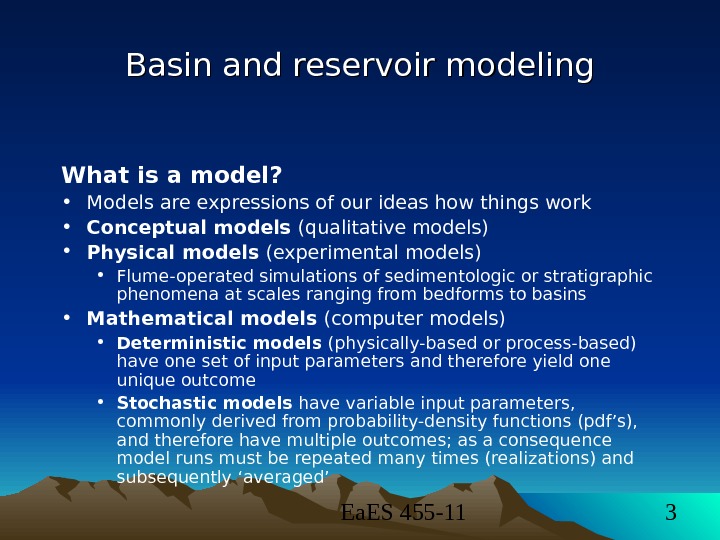
 Ea. ES 455 -11 3 Basin and reservoir modeling What is a model? • Models are expressions of our ideas how things work • Conceptual models (qualitative models) • Physical models (experimental models) • Flume-operated simulations of sedimentologic or stratigraphic phenomena at scales ranging from bedforms to basins • Mathematical models (computer models) • Deterministic models (physically-based or process-based) have one set of input parameters and therefore yield one unique outcome • Stochastic models have variable input parameters, commonly derived from probability-density functions (pdf’s), and therefore have multiple outcomes; as a consequence model runs must be repeated many times (realizations) and subsequently ‘averaged’
Ea. ES 455 -11 3 Basin and reservoir modeling What is a model? • Models are expressions of our ideas how things work • Conceptual models (qualitative models) • Physical models (experimental models) • Flume-operated simulations of sedimentologic or stratigraphic phenomena at scales ranging from bedforms to basins • Mathematical models (computer models) • Deterministic models (physically-based or process-based) have one set of input parameters and therefore yield one unique outcome • Stochastic models have variable input parameters, commonly derived from probability-density functions (pdf’s), and therefore have multiple outcomes; as a consequence model runs must be repeated many times (realizations) and subsequently ‘averaged’
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
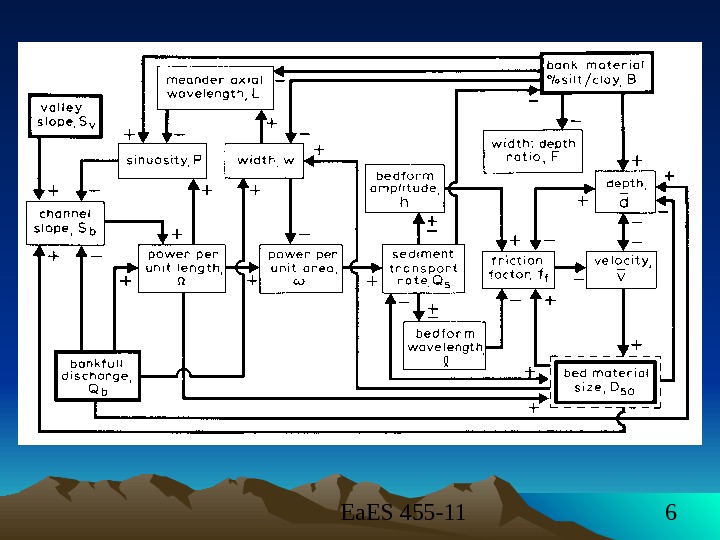
 Ea. ES 455 -11 8 Basin and reservoir modeling • Forward models simulate sets of processes and responses in a system that has specified (assumed) initial boundary conditions (e. g. , the evolution of a sedimentary basin given an initial configuration) • Inverse models use observations as a starting point and aim to estimate initial boundary conditions and combinations of processes and responses that have operated to produce the observed conditions (i. e. , flip side of forward models) • What is the goal of modeling in sedimentary geology? • Understanding processes and responses in sedimentary systems (experimental and process-based models) • Prediction of sedimentary architecture and stratigraphy (primarily stochastic models)
Ea. ES 455 -11 8 Basin and reservoir modeling • Forward models simulate sets of processes and responses in a system that has specified (assumed) initial boundary conditions (e. g. , the evolution of a sedimentary basin given an initial configuration) • Inverse models use observations as a starting point and aim to estimate initial boundary conditions and combinations of processes and responses that have operated to produce the observed conditions (i. e. , flip side of forward models) • What is the goal of modeling in sedimentary geology? • Understanding processes and responses in sedimentary systems (experimental and process-based models) • Prediction of sedimentary architecture and stratigraphy (primarily stochastic models)
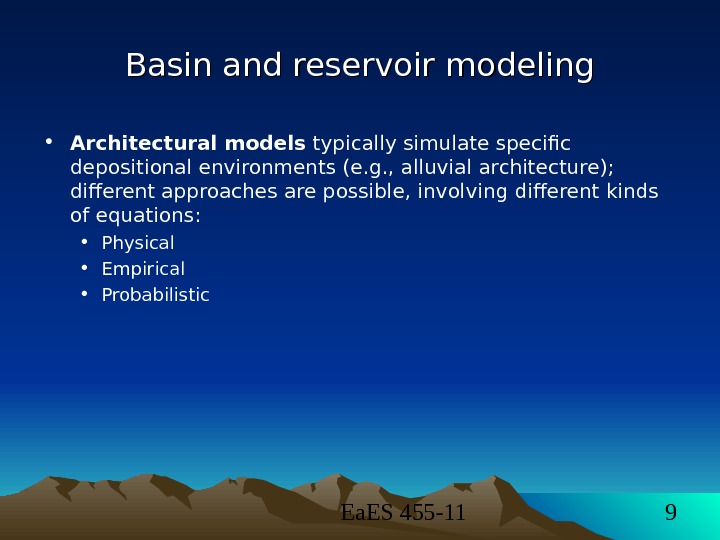
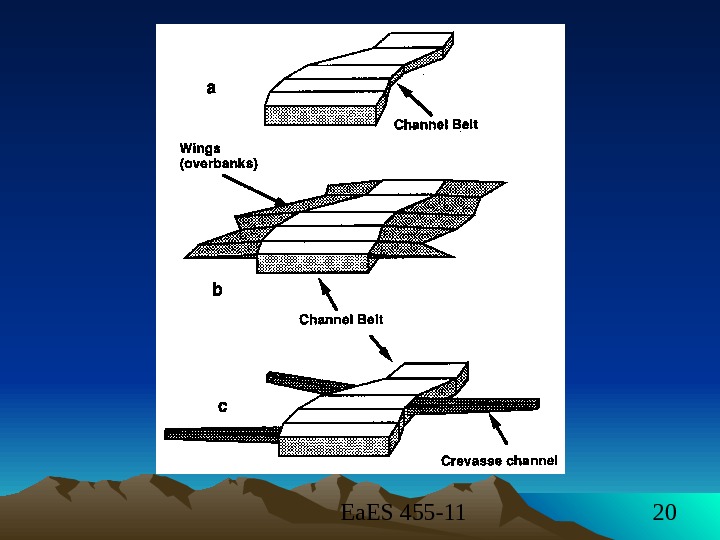
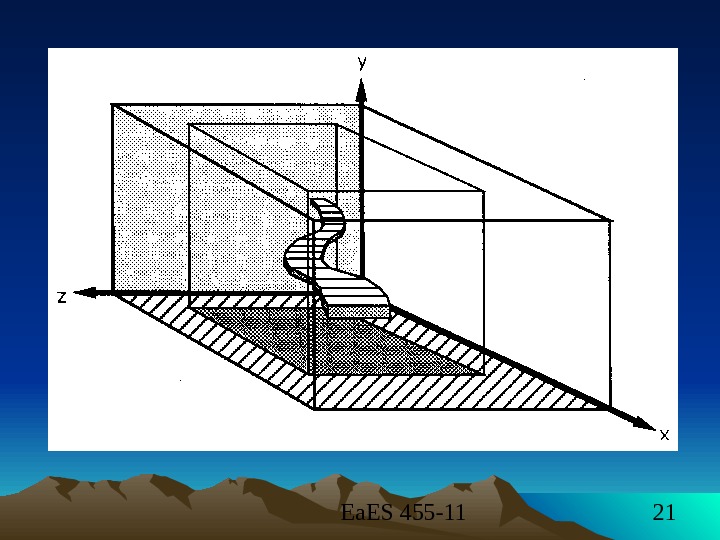
 Ea. ES 455 -11 9 Basin and reservoir modeling • Architectural models typically simulate specific depositional environments (e. g. , alluvial architecture); different approaches are possible, involving different kinds of equations: • Physical • Empirical • Probabilistic
Ea. ES 455 -11 9 Basin and reservoir modeling • Architectural models typically simulate specific depositional environments (e. g. , alluvial architecture); different approaches are possible, involving different kinds of equations: • Physical • Empirical • Probabilistic
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
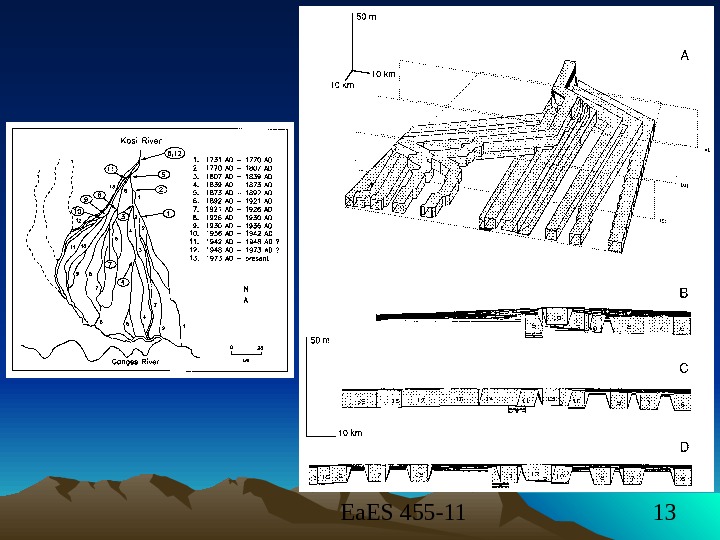
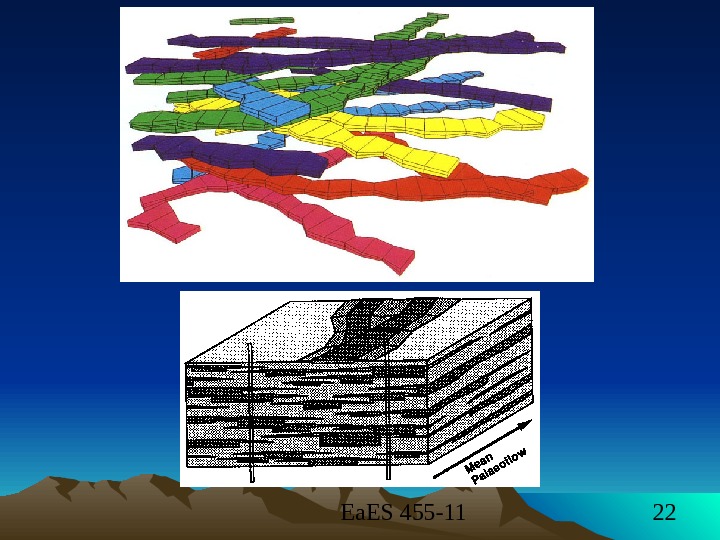
 Ea. ES 455 -11 14 Basin and reservoir modeling • Stratigraphic models are widely used to simulate basin-scale stratal patterns (e. g. , sequence stratigraphy): • In geometric models the sediment surface is represented by one or more surfaces with predetermined geometry • Many models are based on a diffusion equation that relates rates of sediment transport to topographic slopes Slideshow
Ea. ES 455 -11 14 Basin and reservoir modeling • Stratigraphic models are widely used to simulate basin-scale stratal patterns (e. g. , sequence stratigraphy): • In geometric models the sediment surface is represented by one or more surfaces with predetermined geometry • Many models are based on a diffusion equation that relates rates of sediment transport to topographic slopes Slideshow
 Ea. ES 455 -11 15 Animation 1 Animation
Ea. ES 455 -11 15 Animation 1 Animation
 Ea. ES 455 -11 16 Basin and reservoir modeling • A classical approach in sedimentologic/stratigraphic modeling has been to start from first principles (i. e. , basic, small-scale processes of sediment transport) and multiply this to the desired spatial and temporal scale (‘upscaling’) • The outcomes of this approach have been very disappointing (i. e. , upscaling is a very complicated procedure) • There is no law of nature that says that “complexity + complexity = greater complexity”!
Ea. ES 455 -11 16 Basin and reservoir modeling • A classical approach in sedimentologic/stratigraphic modeling has been to start from first principles (i. e. , basic, small-scale processes of sediment transport) and multiply this to the desired spatial and temporal scale (‘upscaling’) • The outcomes of this approach have been very disappointing (i. e. , upscaling is a very complicated procedure) • There is no law of nature that says that “complexity + complexity = greater complexity”!
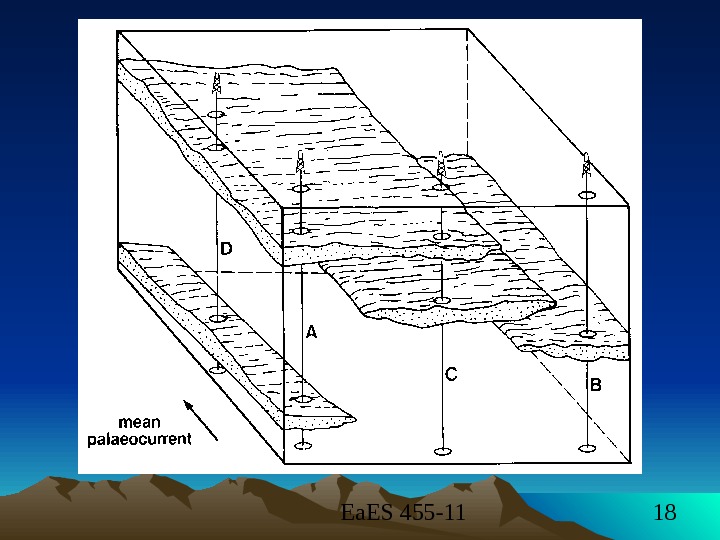
 Ea. ES 455 -11 17 Basin and reservoir modeling • Reservoir characterization is the analysis of subsurface sediments or sedimentary rocks from the perspective of fluid flow through porous media, including issues related to resource recovery (e. g. , groundwater, hydrocarbons) • The net-to-gross ratio (proportion of permeable units) is one of the most basic parameters in reservoir studies • The connectedness between permeable units is another important parameter
Ea. ES 455 -11 17 Basin and reservoir modeling • Reservoir characterization is the analysis of subsurface sediments or sedimentary rocks from the perspective of fluid flow through porous media, including issues related to resource recovery (e. g. , groundwater, hydrocarbons) • The net-to-gross ratio (proportion of permeable units) is one of the most basic parameters in reservoir studies • The connectedness between permeable units is another important parameter
 Ea. ES 455 —
Ea. ES 455 —
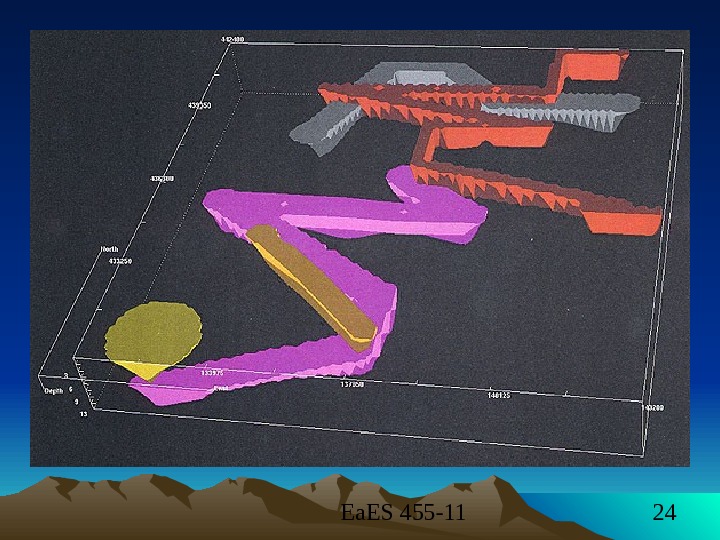
 Ea. ES 455 -11 19 Basin and reservoir modeling • Many reservoir models operate on the scale of sedimentary architecture; they are mostly stochastic • Object-based models simulate the distribution of objects, defined by specified geometries, in 3 D space; simulations are usually constrained by well data • Geostatistical models predict sedimentary facies at unvisited sites, based on the quantified spatial facies variability derived from well data (e. g. , sequential indicator simulation) • Conditioning model output to observations is more easily done in stochastic models, but process-based models have the advantage that they tend to provide sedimentologically more realistic output
Ea. ES 455 -11 19 Basin and reservoir modeling • Many reservoir models operate on the scale of sedimentary architecture; they are mostly stochastic • Object-based models simulate the distribution of objects, defined by specified geometries, in 3 D space; simulations are usually constrained by well data • Geostatistical models predict sedimentary facies at unvisited sites, based on the quantified spatial facies variability derived from well data (e. g. , sequential indicator simulation) • Conditioning model output to observations is more easily done in stochastic models, but process-based models have the advantage that they tend to provide sedimentologically more realistic output
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
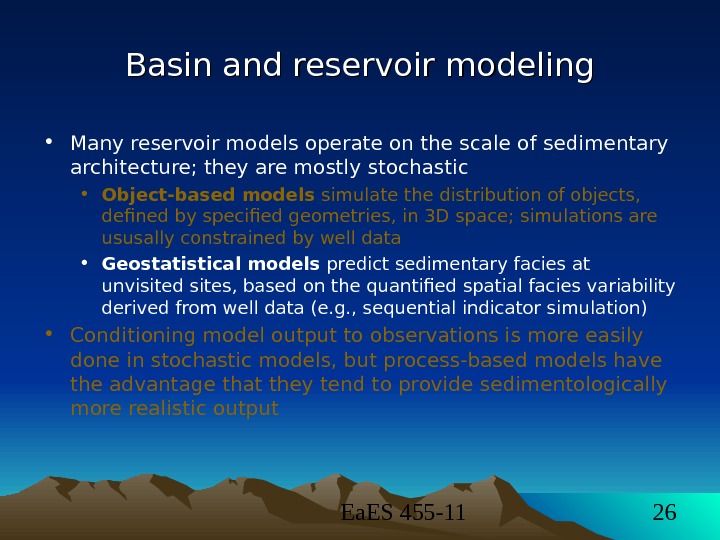
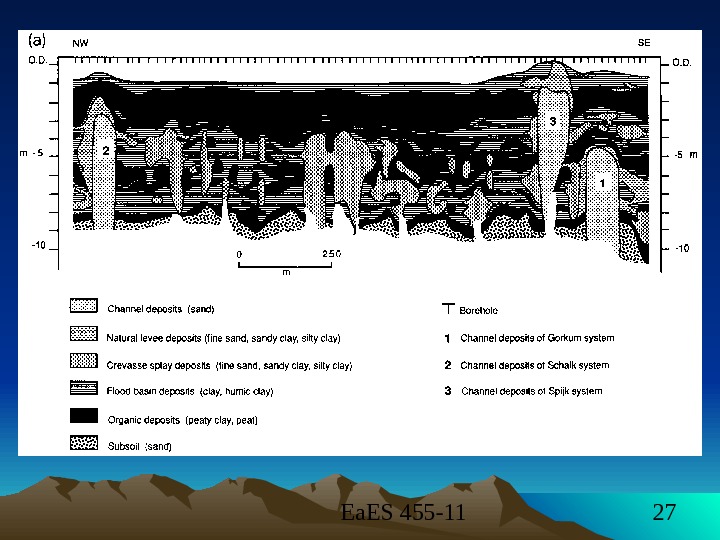
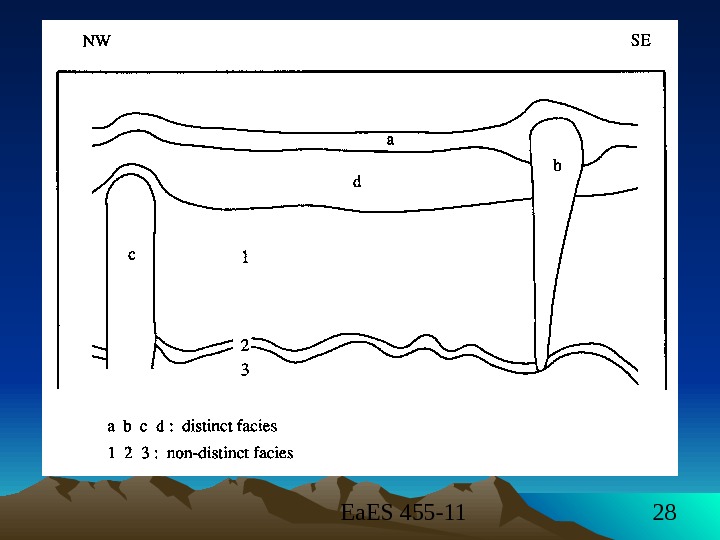
 Ea. ES 455 -11 26 Basin and reservoir modeling • Many reservoir models operate on the scale of sedimentary architecture; they are mostly stochastic • Object-based models simulate the distribution of objects, defined by specified geometries, in 3 D space; simulations are ususally constrained by well data • Geostatistical models predict sedimentary facies at unvisited sites, based on the quantified spatial facies variability derived from well data (e. g. , sequential indicator simulation) • Conditioning model output to observations is more easily done in stochastic models, but process-based models have the advantage that they tend to provide sedimentologically more realistic output
Ea. ES 455 -11 26 Basin and reservoir modeling • Many reservoir models operate on the scale of sedimentary architecture; they are mostly stochastic • Object-based models simulate the distribution of objects, defined by specified geometries, in 3 D space; simulations are ususally constrained by well data • Geostatistical models predict sedimentary facies at unvisited sites, based on the quantified spatial facies variability derived from well data (e. g. , sequential indicator simulation) • Conditioning model output to observations is more easily done in stochastic models, but process-based models have the advantage that they tend to provide sedimentologically more realistic output
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
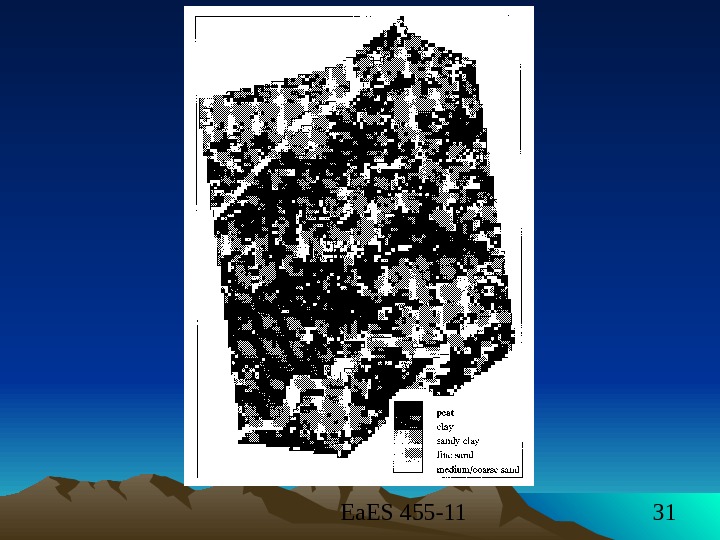
 Ea. ES 455 -11 32 Basin and reservoir modeling • Many reservoir models operate on the scale of sedimentary architecture; they are mostly stochastic • Object-based models simulate the distribution of objects, defined by specified geometries, in 3 D space; simulations are usually constrained by well data • Geostatistical models predict sedimentary facies at unvisited sites, based on the quantified spatial facies variability derived from well data (e. g. , sequential indicator simulation) • Conditioning model output to observations is more easily done in stochastic models, but process-based models have the advantage that they tend to provide sedimentologically more realistic output
Ea. ES 455 -11 32 Basin and reservoir modeling • Many reservoir models operate on the scale of sedimentary architecture; they are mostly stochastic • Object-based models simulate the distribution of objects, defined by specified geometries, in 3 D space; simulations are usually constrained by well data • Geostatistical models predict sedimentary facies at unvisited sites, based on the quantified spatial facies variability derived from well data (e. g. , sequential indicator simulation) • Conditioning model output to observations is more easily done in stochastic models, but process-based models have the advantage that they tend to provide sedimentologically more realistic output
 Ea. ES 455 -11 33 Basin and reservoir modeling • The challenge for experimental models is to mimic real-world conditions as well as possible (scaling); this becomes increasingly difficult with increasing spatial and temporal scales (compare bedforms vs. sedimentary basins) • Grain size (e. g. , how to simulate clays? ) • Grain properties (e. g. , how to simulate cohesion of sediment grains? ) • Fluid mechanics (e. g. , how to keep the Froude number reasonable? ) • Experimental models are increasingly used to simulate sedimentary architecture and basin-scale stratigraphy • One important outcome of experimental modeling is the recognition of non-linear responses
Ea. ES 455 -11 33 Basin and reservoir modeling • The challenge for experimental models is to mimic real-world conditions as well as possible (scaling); this becomes increasingly difficult with increasing spatial and temporal scales (compare bedforms vs. sedimentary basins) • Grain size (e. g. , how to simulate clays? ) • Grain properties (e. g. , how to simulate cohesion of sediment grains? ) • Fluid mechanics (e. g. , how to keep the Froude number reasonable? ) • Experimental models are increasingly used to simulate sedimentary architecture and basin-scale stratigraphy • One important outcome of experimental modeling is the recognition of non-linear responses
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 —
Ea. ES 455 —
 Ea. ES 455 -11 36 Animation 1 Animation 2 Animation
Ea. ES 455 -11 36 Animation 1 Animation 2 Animation
 Ea. ES 455 -11 37 Basin and reservoir modeling • The challenge for experimental models is to mimic real-world conditions as well as possible (scaling); this becomes increasingly difficult with increasing spatial and temporal scales (compare bedforms vs. sedimentary basins) • Grain size (e. g. , how to simulate clays? ) • Grain properties (e. g. , how to simulate cohesion of sediment grains? ) • Fluid mechanics (e. g. , how to keep the Froude number reasonable? ) • Experimental models are increasingly used to simulate sedimentary architecture and basin-scale stratigraphy • One important outcome of experimental modeling is the recognition of non-linear responses Animation
Ea. ES 455 -11 37 Basin and reservoir modeling • The challenge for experimental models is to mimic real-world conditions as well as possible (scaling); this becomes increasingly difficult with increasing spatial and temporal scales (compare bedforms vs. sedimentary basins) • Grain size (e. g. , how to simulate clays? ) • Grain properties (e. g. , how to simulate cohesion of sediment grains? ) • Fluid mechanics (e. g. , how to keep the Froude number reasonable? ) • Experimental models are increasingly used to simulate sedimentary architecture and basin-scale stratigraphy • One important outcome of experimental modeling is the recognition of non-linear responses Animation

