d561ccd319de7cb7d9e91520b536eb57.ppt
- Количество слайдов: 29

Corporate Finance: Securities Professor Scott Hoover Business Administration 221 1
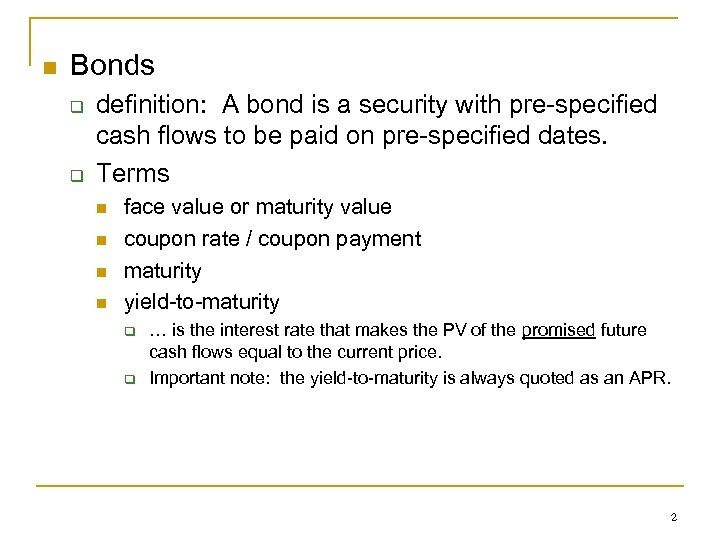
n Bonds q q definition: A bond is a security with pre-specified cash flows to be paid on pre-specified dates. Terms n n face value or maturity value coupon rate / coupon payment maturity yield-to-maturity q q … is the interest rate that makes the PV of the promised future cash flows equal to the current price. Important note: the yield-to-maturity is always quoted as an APR. 2
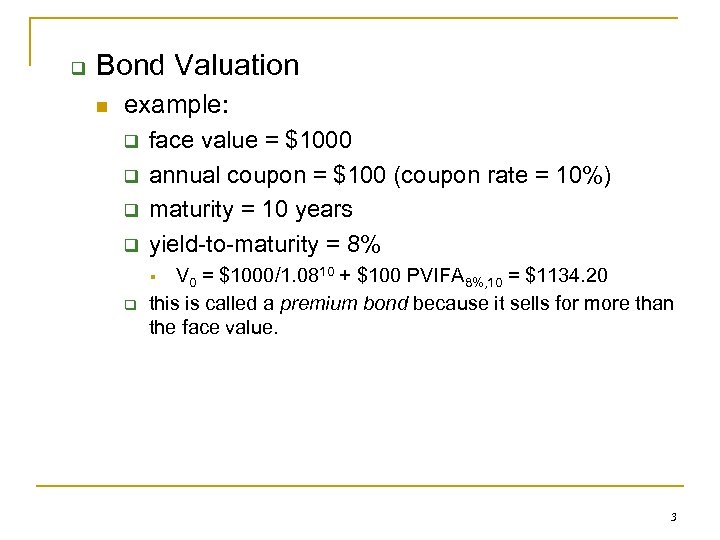
q Bond Valuation n example: q q face value = $1000 annual coupon = $100 (coupon rate = 10%) maturity = 10 years yield-to-maturity = 8% V 0 = $1000/1. 0810 + $100 PVIFA 8%, 10 = $1134. 20 this is called a premium bond because it sells for more than the face value. § q 3
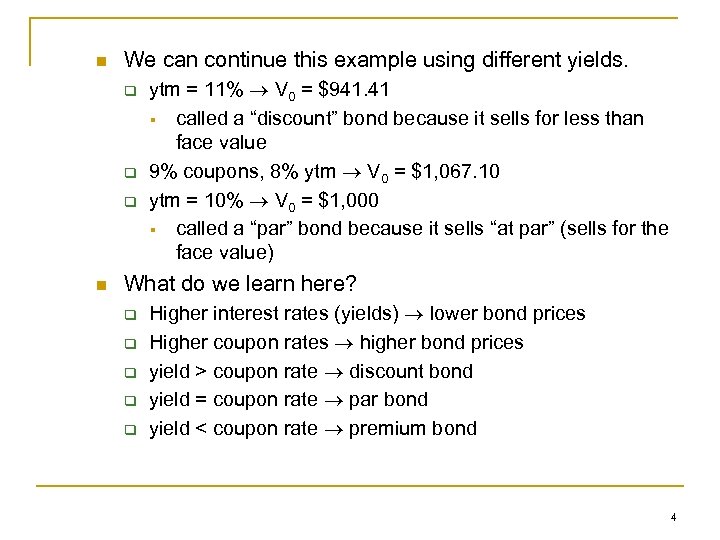
n We can continue this example using different yields. q q q n ytm = 11% V 0 = $941. 41 § called a “discount” bond because it sells for less than face value 9% coupons, 8% ytm V 0 = $1, 067. 10 ytm = 10% V 0 = $1, 000 § called a “par” bond because it sells “at par” (sells for the face value) What do we learn here? q q q Higher interest rates (yields) lower bond prices Higher coupon rates higher bond prices yield > coupon rate discount bond yield = coupon rate par bond yield < coupon rate premium bond 4
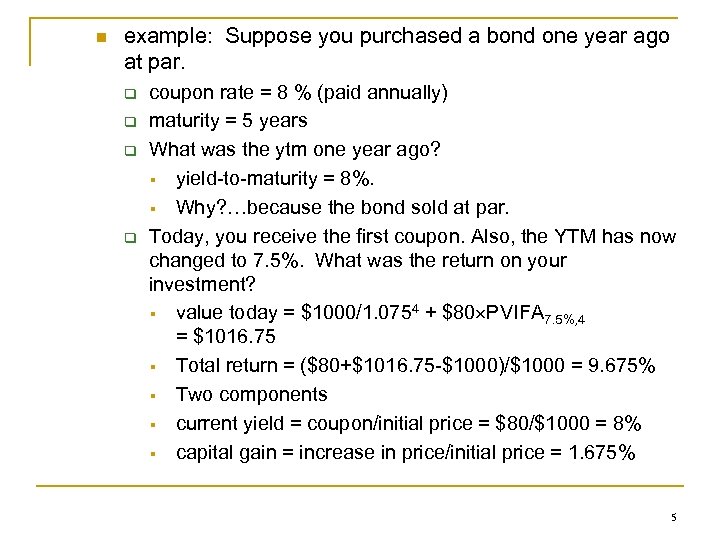
n example: Suppose you purchased a bond one year ago at par. q q coupon rate = 8 % (paid annually) maturity = 5 years What was the ytm one year ago? § yield-to-maturity = 8%. § Why? …because the bond sold at par. Today, you receive the first coupon. Also, the YTM has now changed to 7. 5%. What was the return on your investment? § value today = $1000/1. 0754 + $80 PVIFA 7. 5%, 4 = $1016. 75 § Total return = ($80+$1016. 75 -$1000)/$1000 = 9. 675% § Two components § current yield = coupon/initial price = $80/$1000 = 8% § capital gain = increase in price/initial price = 1. 675% 5

n Two sources of returns q q interest (coupon) payments changes in interest rates § changes in risk § changes in macroeconomic rates 6

n example: Suppose you observe the following bond information: q q q n I = $50 (annual coupons, coupon rate = 5%) n = 6 years price = $975 What is the yield-to-maturity on the bond? q q q bond selling at a discount Rd > 5%. guess: 6% V 0 = 1000/1. 066 + 50 PVIFA 6%, 6 = $950. 83 § too low…. need to decrease the interest rate guess: 5. 6% V 0 = 1000/1. 0566 + 50 PVIFA 5. 6%, 6 = $970. 12 § …a bit too low again guess: 5. 5% V 0 = 1000/1. 0556 + 50 PVIFA 5. 5%, 6 = $975. 02 close enough…. the actual yield is 5. 50045% 7

q Non-Annual Coupons n Background: EAR vs. APR q q q Annual Percentage Rate (APR) period rate # of periods per year. § ignores compounding of interest Effective Annual Rate (EAR) actual annual interest rate. § assumes interest is compounded Example: Suppose the semi-annual rate is 6%. § The APR is 6%× 2 = 12% § The EAR is 1. 062 -1 = 12. 36%. § Why? § If we invest a dollar, we will have $1. 06 in six months. If we reinvest that money for another six months, we will have $1. 06× 1. 06 = $1. 1236, for a 12. 36% return. 8

q q q The APR is often a misleading indictor of the true interest rate. § Why? §. . . then why is it used? Converting from APR to EAR (and vice versa) is easy if we remember the definitions. We might even have an infinite number of compounding periods per year (“continuous compounding”). § In that case, we find that EAR = e. APR – 1, where e is the constant e = 2. 7182818… 9

n example: q q q q coupon rate = 12%, paid semi-annually 5 years to maturity. appropriate discount rate = 10% EAR What is the price of the bond? § Coupons and coupon rates are calculated on an annual basis, so a 12% coupon rate is 6% every six months. V 0 = 60/1. 10. 5 + 60/1. 1 + … + 60/1. 14. 5 + 1000/1. 15 = $1086. 92 Alternatively, we can use the semi-annual rate. § Rsa = 1. 10. 5 – 1 = 4. 88% V 0 = 60 PVIFA 4. 88%, 10 + 1000/1. 048810 = $1086. 92 10
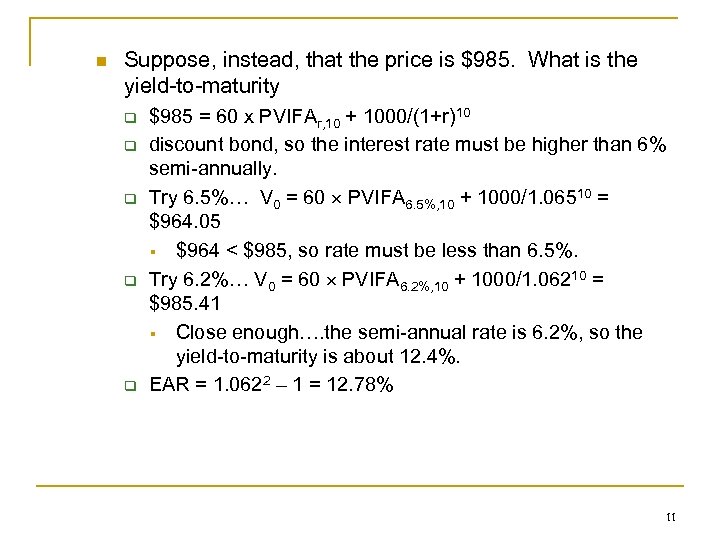
n Suppose, instead, that the price is $985. What is the yield-to-maturity q q q $985 = 60 x PVIFAr, 10 + 1000/(1+r)10 discount bond, so the interest rate must be higher than 6% semi-annually. Try 6. 5%… V 0 = 60 PVIFA 6. 5%, 10 + 1000/1. 06510 = $964. 05 § $964 < $985, so rate must be less than 6. 5%. Try 6. 2%… V 0 = 60 PVIFA 6. 2%, 10 + 1000/1. 06210 = $985. 41 § Close enough…. the semi-annual rate is 6. 2%, so the yield-to-maturity is about 12. 4%. EAR = 1. 0622 – 1 = 12. 78% 11
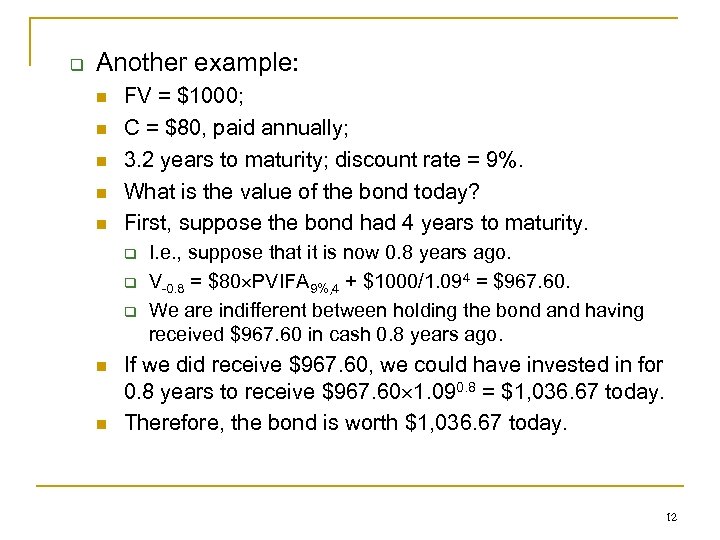
q Another example: n n n FV = $1000; C = $80, paid annually; 3. 2 years to maturity; discount rate = 9%. What is the value of the bond today? First, suppose the bond had 4 years to maturity. q q q n n I. e. , suppose that it is now 0. 8 years ago. V-0. 8 = $80 PVIFA 9%, 4 + $1000/1. 094 = $967. 60. We are indifferent between holding the bond and having received $967. 60 in cash 0. 8 years ago. If we did receive $967. 60, we could have invested in for 0. 8 years to receive $967. 60 1. 090. 8 = $1, 036. 67 today. Therefore, the bond is worth $1, 036. 67 today. 12

n Bond Provisions q Convertibility n q Warrants n n n q the purchaser can exchange the bond for a pre-specified number of shares of stock during a pre-specified period. often "attached" to a bond the purchaser can buy a pre-specified number of shares of stock for a pre-specified price during a pre-specified period. In contrast to a convertible, the warrantholder does not give up the bond when warrants are exercised. Call provision n the seller can buy back the bond for a pre-specified price during a pre-specified period 13

q Other Covenants n force the issuer to maintain a certain level of firm stability q q usually specified through financial ratio restrictions If a covenant is violated, the bond purchaser has the right to demand immediate repayment. Think about how each of these is likely to affect bond values. Why would a company choose to issue a bond with one of these provisions? 14

n Derivatives q Call option: n n n The buyer of an American call option has the right (but not the obligation) to purchase the underlying asset on or before a pre-specified date for a pre-specified price. The seller (writer) of the option has the obligation to sell the underlying asset if the buyer chooses to buy it. For European options, one can only exercise on the expiration date. 15

q Put option: n n q The buyer of an American put option has the right (but not the obligation) to sell the underlying asset on or before a pre-specified date for a pre-specified price. The seller (writer) of the option has the obligation to buy the underlying asset if the buyer chooses to sell it. Forward: n The buyer in a forward contract has the obligation to purchase the underlying asset from the seller for a prespecified price on a pre-specified date. 16

q When would a firm want to use derivative securities? n n Primarily, derivatives are used to reduce price risk. Example: Suppose a gold mine is planning to produce 10, 000 ounces of gold next quarter. q q q n It might lock in a price for those ounces by selling a forward contract. Alternatively, it might buy put options that allow the firm to sell at a pre-specified price if need be. In both cases, the firm is using derivatives as insurance against declines in price. In contrast, the buyer of a commodity might buy forward or buy call options to hedge the risk. 17

q How does a firm choose between forwards and options? n Generally speaking, … q q forwards are preferred when the firm wants to hedge a certain cash flow. § e. g. , the firm has a contract in place that will require the firm to pay ¥ 100, 000 in one year. The firm might buy yen forwards to hedge that risk. options are preferred when the firm wants to hedge an uncertain cash flow. § e. g. , the firm is negotiating a deal in which the firm would have to manufacture a product. The manufacturing process requires oil. The firm might buy call options on oil to hedge the risk. 18

n Stocks q definition: A residual claim on firm cash flows. q Notation n n q R = required rate of return on the stock Dt = dividend paid at date t Pt = price of the stock at date t g = expected rate of growth in dividends Definitions n n n dividend yield = D 1/P 0 capital gains yield = (P 1 - P 0)/P 0 total return = dividend yield + capital gains yield. q Note that this is analogous to bond returns 19
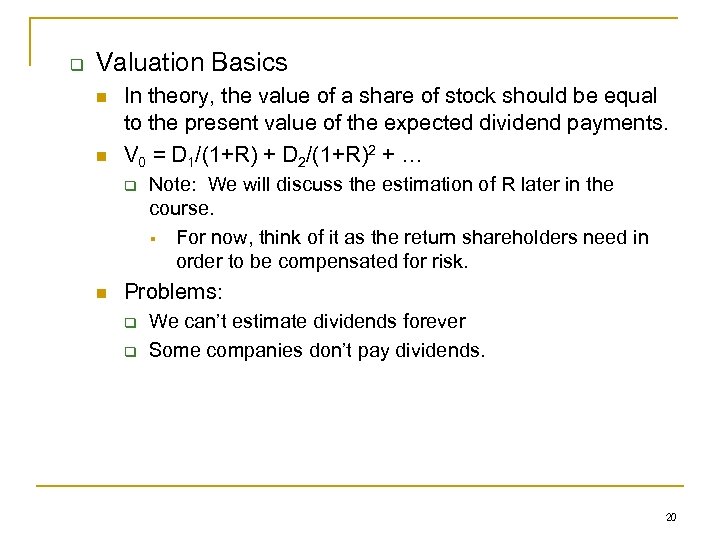
q Valuation Basics n n In theory, the value of a share of stock should be equal to the present value of the expected dividend payments. V 0 = D 1/(1+R) + D 2/(1+R)2 + … q n Note: We will discuss the estimation of R later in the course. § For now, think of it as the return shareholders need in order to be compensated for risk. Problems: q q We can’t estimate dividends forever Some companies don’t pay dividends. 20

q The Malkiel Model: A solution to the problems? n n n We value the stock as if it will be sold at some date (T). V 0 = D 1/(1+R) + D 2/(1+R)2 + … + DT/(1+R)T + PT /(1+R)T How might we estimate PT (the price at date T)? q q Multiples Perpetual growth n Using multiples…. n 1. Forecast XT, where XT is a cash flow-related variable (earnings, sales, etc. ) at date T q 2. Estimate (P/X)T, the price-to-X ratio at date T q 3. PT = (P/X)T XT Perpetual Growth q q q 1. Estimate a long-term growth rate (g) 2. Use PT = DT+1/(R-g) 21
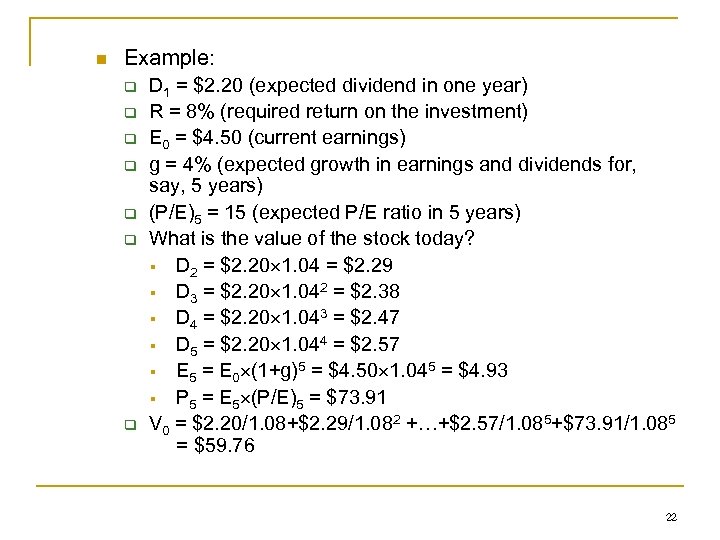
n Example: q q q q D 1 = $2. 20 (expected dividend in one year) R = 8% (required return on the investment) E 0 = $4. 50 (current earnings) g = 4% (expected growth in earnings and dividends for, say, 5 years) (P/E)5 = 15 (expected P/E ratio in 5 years) What is the value of the stock today? § D 2 = $2. 20 1. 04 = $2. 29 § D 3 = $2. 20 1. 042 = $2. 38 § D 4 = $2. 20 1. 043 = $2. 47 § D 5 = $2. 20 1. 044 = $2. 57 § E 5 = E 0 (1+g)5 = $4. 50 1. 045 = $4. 93 § P 5 = E 5 (P/E)5 = $73. 91 V 0 = $2. 20/1. 08+$2. 29/1. 082 +…+$2. 57/1. 085+$73. 91/1. 085 = $59. 76 22
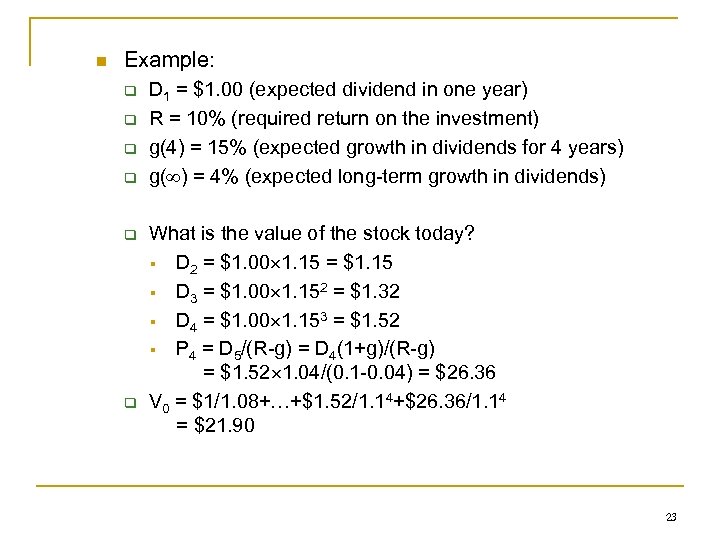
n Example: q q q D 1 = $1. 00 (expected dividend in one year) R = 10% (required return on the investment) g(4) = 15% (expected growth in dividends for 4 years) g( ) = 4% (expected long-term growth in dividends) What is the value of the stock today? § D 2 = $1. 00 1. 15 = $1. 15 § D 3 = $1. 00 1. 152 = $1. 32 § D 4 = $1. 00 1. 153 = $1. 52 § P 4 = D 5/(R-g) = D 4(1+g)/(R-g) = $1. 52 1. 04/(0. 1 -0. 04) = $26. 36 V 0 = $1/1. 08+…+$1. 52/1. 14+$26. 36/1. 14 = $21. 90 23
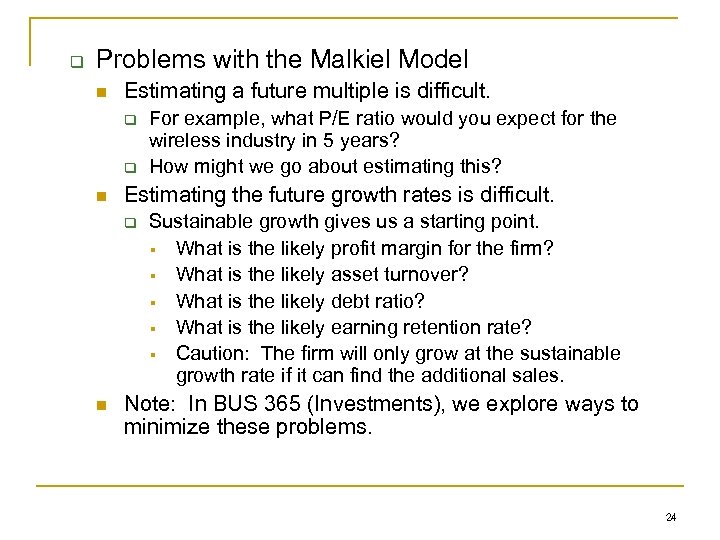
q Problems with the Malkiel Model n Estimating a future multiple is difficult. q q n Estimating the future growth rates is difficult. q n For example, what P/E ratio would you expect for the wireless industry in 5 years? How might we go about estimating this? Sustainable growth gives us a starting point. § What is the likely profit margin for the firm? § What is the likely asset turnover? § What is the likely debt ratio? § What is the likely earning retention rate? § Caution: The firm will only grow at the sustainable growth rate if it can find the additional sales. Note: In BUS 365 (Investments), we explore ways to minimize these problems. 24
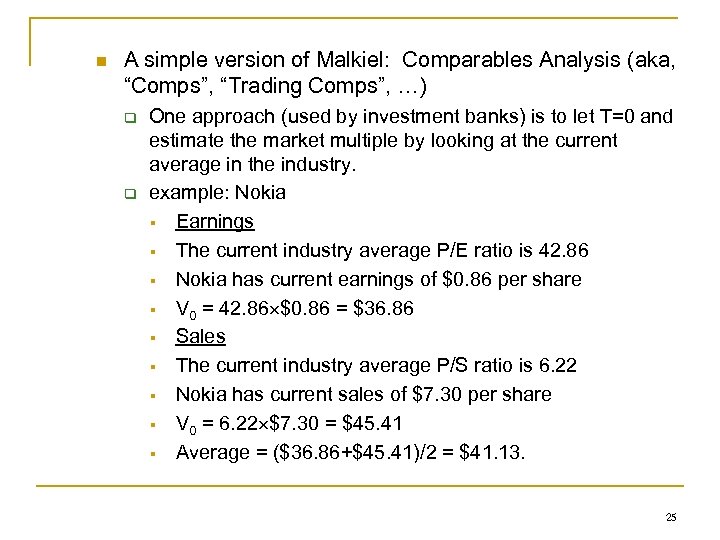
n A simple version of Malkiel: Comparables Analysis (aka, “Comps”, “Trading Comps”, …) q q One approach (used by investment banks) is to let T=0 and estimate the market multiple by looking at the current average in the industry. example: Nokia § Earnings § The current industry average P/E ratio is 42. 86 § Nokia has current earnings of $0. 86 per share § V 0 = 42. 86 $0. 86 = $36. 86 § Sales § The current industry average P/S ratio is 6. 22 § Nokia has current sales of $7. 30 per share § V 0 = 6. 22 $7. 30 = $45. 41 § Average = ($36. 86+$45. 41)/2 = $41. 13. 25

q n A related technique is called “M&A Analysis” or “Transaction Comps. ” § This is identical to Comps except that… - We examine historical mergers and acquisitions involving similar companies. - In our market multiples, we use the transaction purchase price instead of the market price Problems with “Comps” q q q Ignores growth Ignores debt structure Biased by our choice of similar companies 26
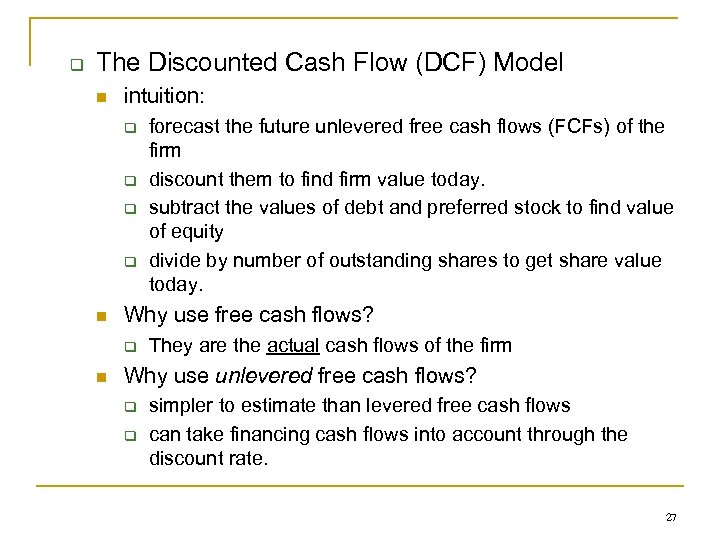
q The Discounted Cash Flow (DCF) Model n intuition: q q n Why use free cash flows? q n forecast the future unlevered free cash flows (FCFs) of the firm discount them to find firm value today. subtract the values of debt and preferred stock to find value of equity divide by number of outstanding shares to get share value today. They are the actual cash flows of the firm Why use unlevered free cash flows? q q simpler to estimate than levered free cash flows can take financing cash flows into account through the discount rate. 27
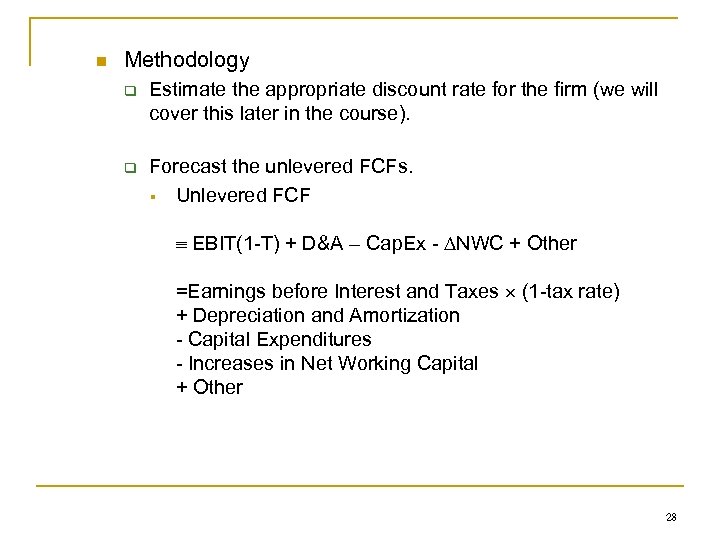
n Methodology q Estimate the appropriate discount rate for the firm (we will cover this later in the course). q Forecast the unlevered FCFs. § Unlevered FCF EBIT(1 -T) + D&A – Cap. Ex - NWC + Other =Earnings before Interest and Taxes (1 -tax rate) + Depreciation and Amortization - Capital Expenditures - Increases in Net Working Capital + Other 28

q q Estimate the terminal value of the FCFs at the end of the forecast period. § E. g. , if we have forecast 5 years of unlevered FCFs, we estimate the value at year 5 of the unlevered FCFs from years 6 on. Discount the expected FCFs to the present using the Weighted Average Cost of Capital (WACC). § This gives us our estimate of Firm Value. § We will cover the WACC in detail later. § For now, view it as the weighted average return needed by the company’s investors. Subtract the values of debt and preferred stock to get the value of equity. Divide by the number of shares outstanding to get the per share value. 29
d561ccd319de7cb7d9e91520b536eb57.ppt