Complex Numbers Loci Problems Objectives To

Complex Numbers Loci Problems

Objectives • To use the modulus to define a locus of points P(x, y) • To use the argument to define a locus of points P(x, y) (described as a half line) • To identify regions on a diagram defined by a locus of points P(x, y)

Definition of a locus of points • A locus is the path formed as a point follows a rule. P(x, y) denotes the set of points. • The plural of locus is loci. • The modulus and arguments of complex numbers can be used to define a locus of points P(x, y).

Definition of a locus of points • When z = x +yi the point P(x, y) can be anywhere on the Argand diagram. • If a condition is imposed on z then this affects the possible positions of P and so all possible P’s, i. e. P(x, y), becomes the locus of points. • For example the locus of points exactly 4 cm from a point (x, y) will be a circle with centre (x, y) and radius 4 cm.

Loci using the modulus of z • Considering the above example, the modulus of a complex number can be used to define a locus of points since the modulus of a complex number is the length of the position vector on the Argand diagram and therefore could be the radius of a circle with centre (0, 0).

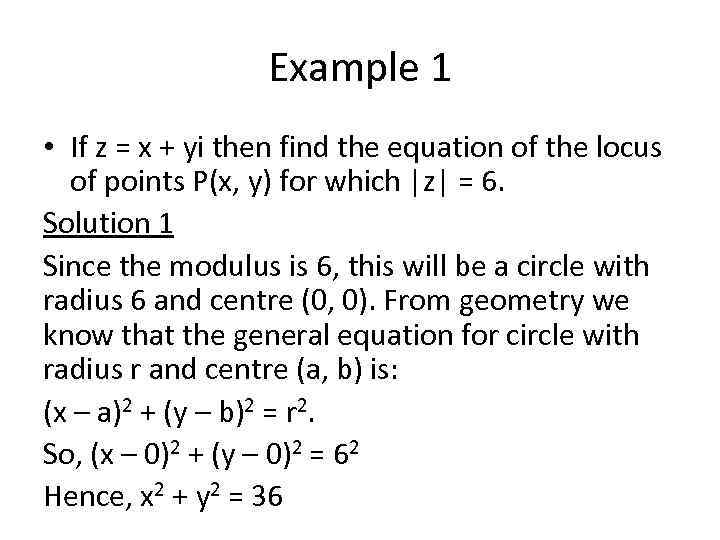
Example 1 • If z = x + yi then find the equation of the locus of points P(x, y) for which |z| = 6. Solution 1 Since the modulus is 6, this will be a circle with radius 6 and centre (0, 0). From geometry we know that the general equation for circle with radius r and centre (a, b) is: (x – a)2 + (y – b)2 = r 2. So, (x – 0)2 + (y – 0)2 = 62 Hence, x 2 + y 2 = 36

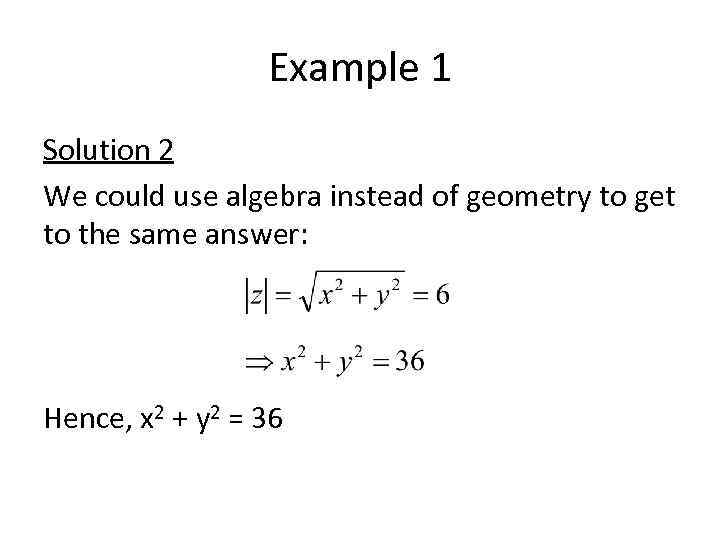
Example 1 Solution 2 We could use algebra instead of geometry to get to the same answer: Hence, x 2 + y 2 = 36

Loci using the modulus of (z – z 1) where z = x + yi and z 1 = x 1 + y 1 i • We can use the method used in solution 2 to find the equation of a locus of points for which |z – z 1| = r

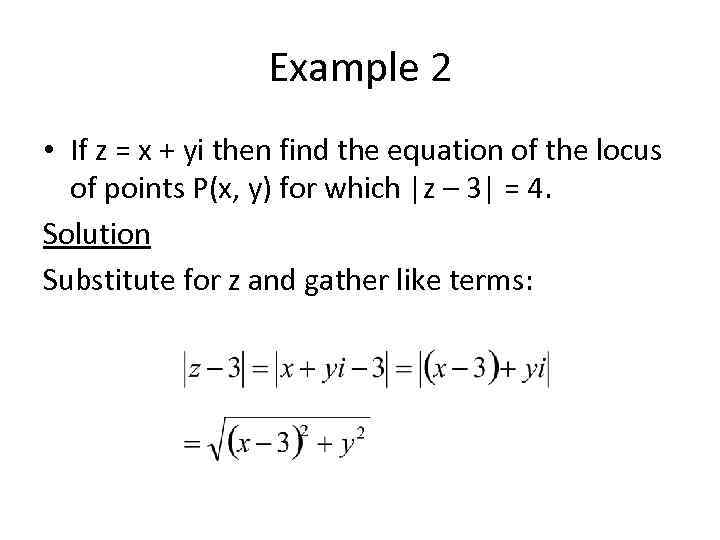
Example 2 • If z = x + yi then find the equation of the locus of points P(x, y) for which |z – 3| = 4. Solution Substitute for z and gather like terms:

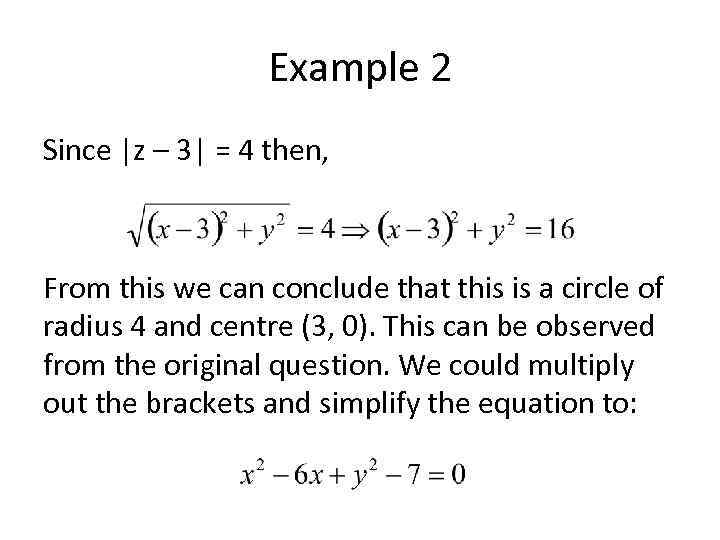
Example 2 Since |z – 3| = 4 then, From this we can conclude that this is a circle of radius 4 and centre (3, 0). This can be observed from the original question. We could multiply out the brackets and simplify the equation to:

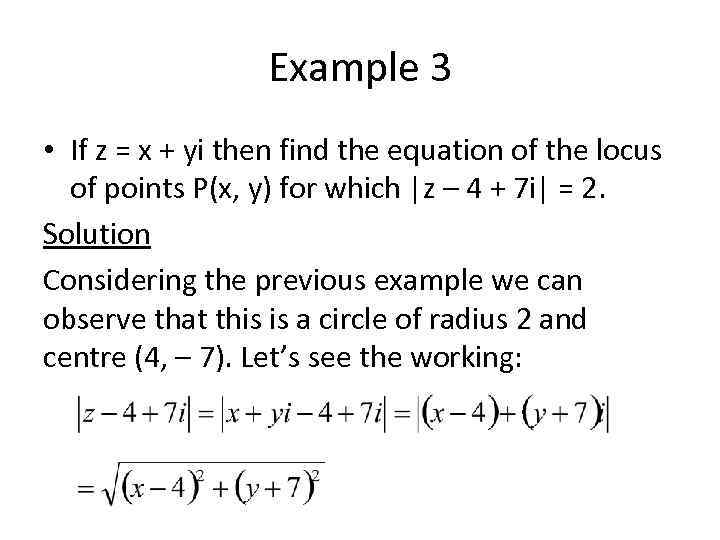
Example 3 • If z = x + yi then find the equation of the locus of points P(x, y) for which |z – 4 + 7 i| = 2. Solution Considering the previous example we can observe that this is a circle of radius 2 and centre (4, – 7). Let’s see the working:

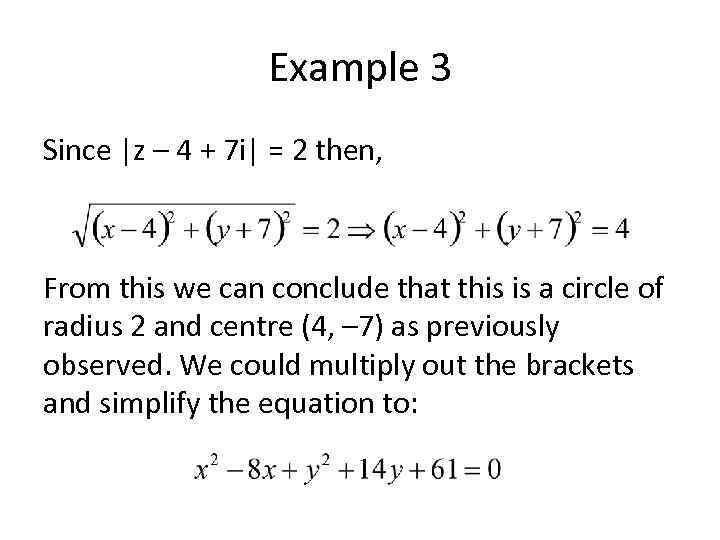
Example 3 Since |z – 4 + 7 i| = 2 then, From this we can conclude that this is a circle of radius 2 and centre (4, – 7) as previously observed. We could multiply out the brackets and simplify the equation to:

Example 3 Also consider the centre of the circle |z – z 1 |where z = x + yi and z 1 = x 1 + y 1 i by considering the above example 3. |z| has a centre of (0, 0) and |z – 4 + 7 i| has a centre of (4, – 7). So in this example z 1 = 4 – 7 i. Therefore we conclude that the centre of the circle with radius |z – z 1 |where z = x + yi and z 1 = x 1 + y 1 i is (x 1, y 1). (Be careful with the signs)

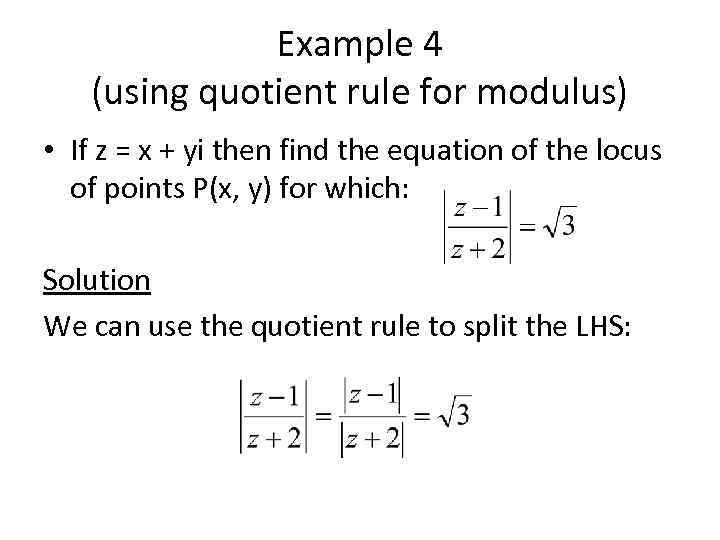
Example 4 (using quotient rule for modulus) • If z = x + yi then find the equation of the locus of points P(x, y) for which: Solution We can use the quotient rule to split the LHS:

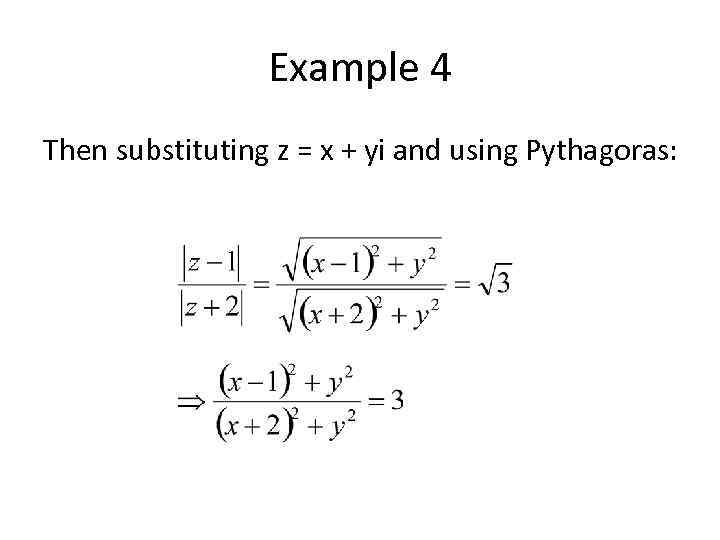
Example 4 Then substituting z = x + yi and using Pythagoras:

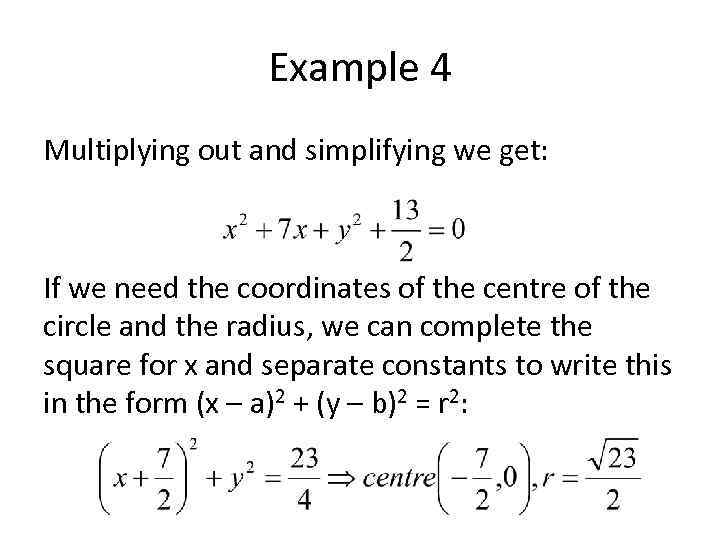
Example 4 Multiplying out and simplifying we get: If we need the coordinates of the centre of the circle and the radius, we can complete the square for x and separate constants to write this in the form (x – a)2 + (y – b)2 = r 2:

Loci using the argument of z • If we are given the argument of z then this tells us the angle the straight line on the Argand diagram makes with the positive x axis. • Therefore, if we are given the argument of z then the locus of points forms a straight line.

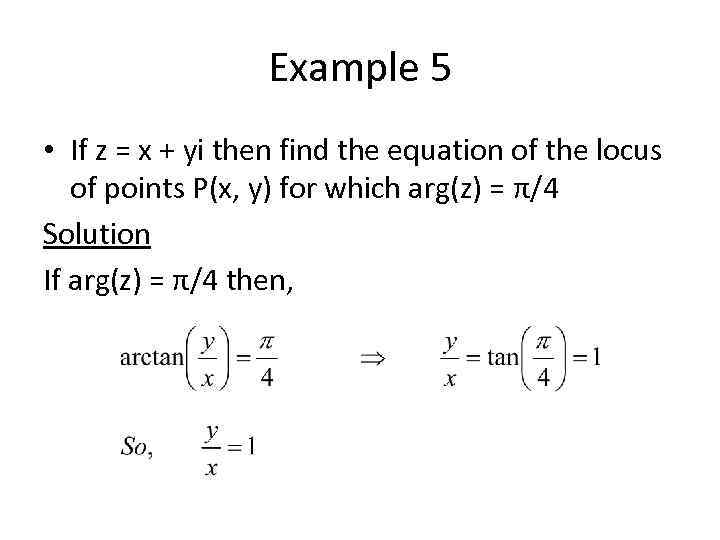
Example 5 • If z = x + yi then find the equation of the locus of points P(x, y) for which arg(z) = π/4 Solution If arg(z) = π/4 then,

Example 5 Hence, the equation of the locus of points is: y=x However, remember that when we think about the argument of a complex number the quadrant is important. In this case, the argument was positive and acute so it is in the 1 st quadrant which means that x > 0 and y > 0.

Loci using the argument of z • So, this means that when finding the equations of loci using the argument we must consider whether x and y are positive or negative. • It is also worth noting here that when considering arg(z) we consider the straight line from the origin (0, 0) and so the straight line has a starting point of the origin and the argument is measured at that starting point.

Loci using the argument Terminology • The locus of points, P(x, y), where arg(z) = θ is described as the part line or half line of P(x, y) since the line representing the locus of points has a starting point and so is not a complete line.

Loci using the argument of (z – z 1) • Now consider the argument of (z – z 1) where z = x + yi and z 1 = x 1 + y 1 i. • When we considered the modulus of (z – z 1) we found that the centre of the circle moved from (0, 0) to (x 1, y 1). • This means that the argument of (z – z 1) is measured at the point (x 1, y 1). • (This can also be seen from considering the vector of (z – z 1). )

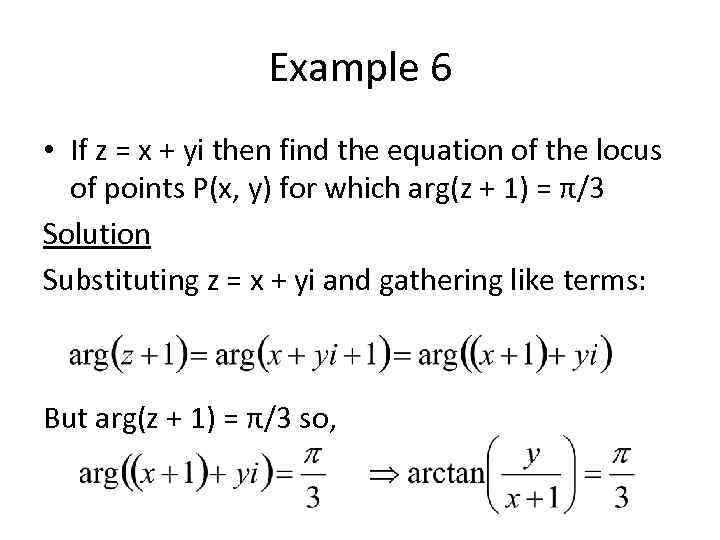
Example 6 • If z = x + yi then find the equation of the locus of points P(x, y) for which arg(z + 1) = π/3 Solution Substituting z = x + yi and gathering like terms: But arg(z + 1) = π/3 so,

Example 6 Solving this equation: So we have an equation for our locus of points but we must consider the constraints for x and y by considering whether the argument is acute, obtuse, positive or negative.

Example 6 Going back to a previous step: π/3 is positive and acute which is first quadrant which means that if z = a + bi then a > 0 and b > 0. Considering ‘a’ and ‘b’ here we have: x + 1 > 0 and y > 0, i. e. x > – 1 and y > 0. Hence the equation of the locus is:

Loci using the argument of (z – z 1) Therefore, to find the constraints for x and y for a loci where arg(z – z 1) = θ, Consider which quadrant θ is in to decide whether x – x 1 > 0 or x – x 1 < 0 and whether y – y 1 > 0 or y – y 1 < 0

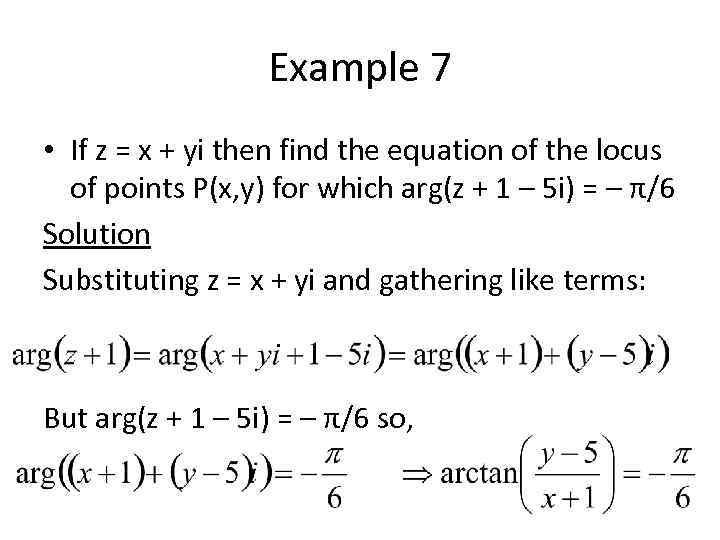
Example 7 • If z = x + yi then find the equation of the locus of points P(x, y) for which arg(z + 1 – 5 i) = – π/6 Solution Substituting z = x + yi and gathering like terms: But arg(z + 1 – 5 i) = – π/6 so,

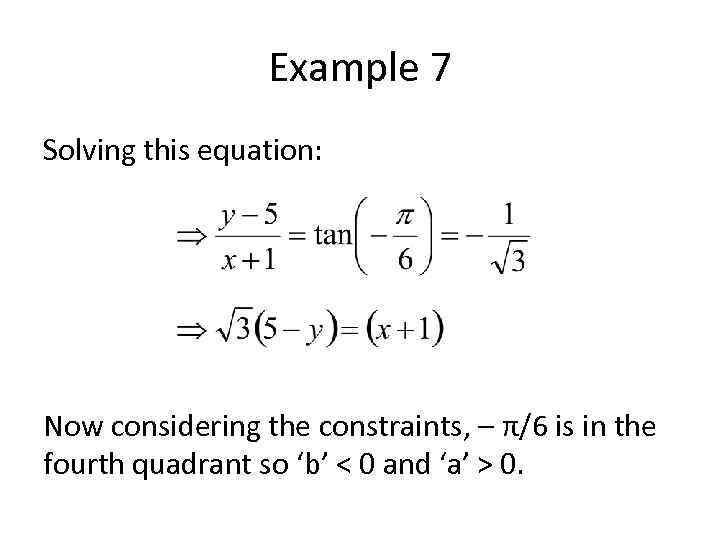
Example 7 Solving this equation: Now considering the constraints, – π/6 is in the fourth quadrant so ‘b’ < 0 and ‘a’ > 0.

Example 7 So, considering a previous step: We have y – 5 < 0 and x + 1 > 0. Hence, the equation of the locus of points is,

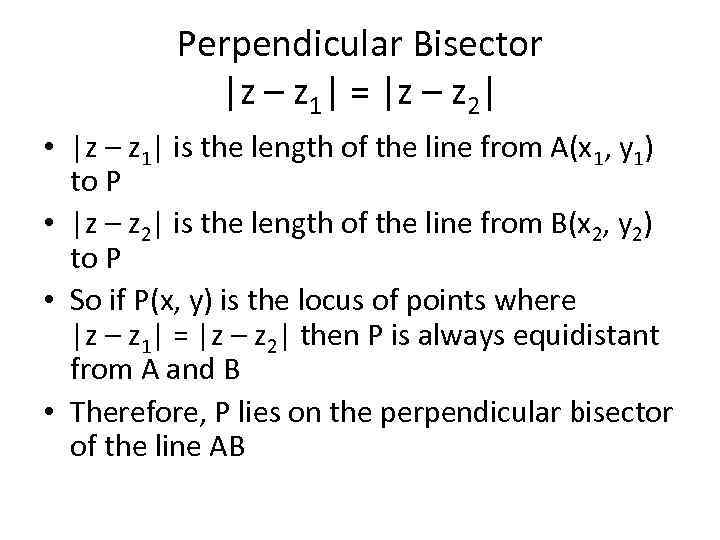
Perpendicular Bisector |z – z 1| = |z – z 2| • |z – z 1| is the length of the line from A(x 1, y 1) to P • |z – z 2| is the length of the line from B(x 2, y 2) to P • So if P(x, y) is the locus of points where |z – z 1| = |z – z 2| then P is always equidistant from A and B • Therefore, P lies on the perpendicular bisector of the line AB

Example 8 • If z 1 = 3 + i and z 2 = – 3 – i and z = x + yi determine the locus of the set of points P(x, y) for which |z – z 1| = |z – z 2|. Solution If |z – z 1| = |z – z 2| then the locus of points P(x, y) will form the perpendicular bisector of the line from A(x 1, y 1) to B(x 2, y 2). So we need the gradient of the line AB to find the equation of the perpendicular bisector to AB.

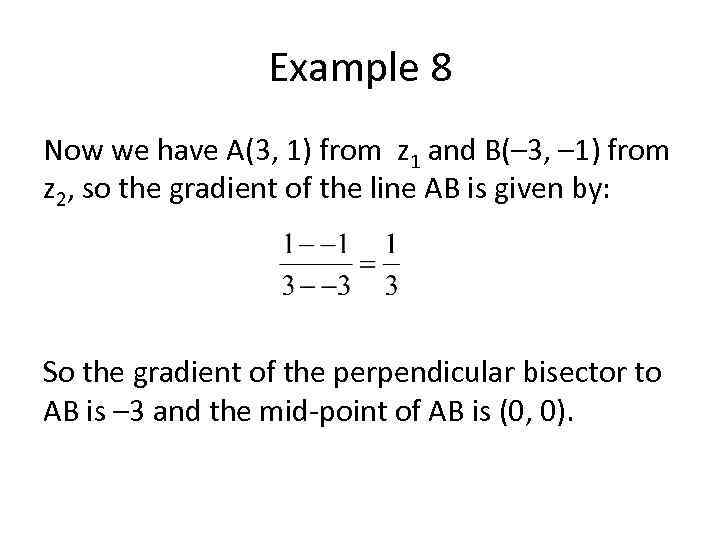
Example 8 Now we have A(3, 1) from z 1 and B(– 3, – 1) from z 2, so the gradient of the line AB is given by: So the gradient of the perpendicular bisector to AB is – 3 and the mid-point of AB is (0, 0).

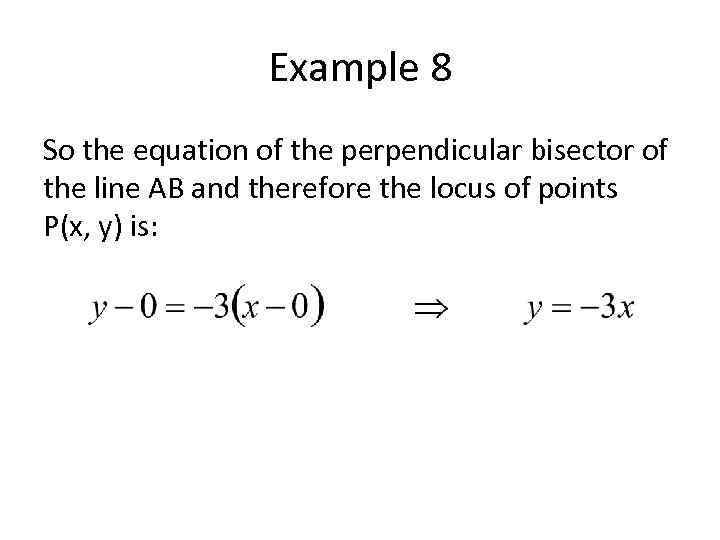
Example 8 So the equation of the perpendicular bisector of the line AB and therefore the locus of points P(x, y) is:

Example 9 (using quotient rule for argument) • Remember that: • Whether the question gives the LHS format or the RHS format, the same method can be used. • The resulting locus will form the arc of a circle (not a complete circle) • See scanned question and solution.

Identifying regions on a diagram • We can use the above methods to find loci to identify regions defined by inequalities. • Instead of an equal sign as all of the examples above there will be an inequality sign. • The same methods as above are used to find the loci and draw the half lines, circles or part circles. • Then we shade the diagram to indicate the region defined by the inequality.

Examples 10 & 11 • See scanned questions and solutions

Example 12 (greatest and least values) • We can also use loci to identify the greatest and least values of the modulus of a complex number. • See scanned question and solution