9cef657eff7c05e2a81f0aee536170f6.ppt
- Количество слайдов: 21
 Chapter Thirteen: Term Structure of Interest Rates (Econ 512): Economics of Financial Markets Dr. Reyadh Faras Econ 512 Dr. Reyadh Faras
Chapter Thirteen: Term Structure of Interest Rates (Econ 512): Economics of Financial Markets Dr. Reyadh Faras Econ 512 Dr. Reyadh Faras
 Overview § One of the dimensions relevant for distinguishing between bonds, the time to maturity is one of the most important. § It is on the relationship between each bond’s time to maturity and its rate of return that analysis of the term structure of interest rates focuses. Econ 512 Dr. Reyadh Faras
Overview § One of the dimensions relevant for distinguishing between bonds, the time to maturity is one of the most important. § It is on the relationship between each bond’s time to maturity and its rate of return that analysis of the term structure of interest rates focuses. Econ 512 Dr. Reyadh Faras
 13. 1 Yield Curves 13. 1. 1 Yield Curves in Principle § Diversity among bond contracts can be narrowed because yield curves are almost always constructed for government bonds, for which the risk of default is negligible. Thus, their differences are: time to maturity, n, and coupon rate, c/m. § Yield curves are constructed for ZC bonds, the spot yield is plotted against the time to maturity. § Panel (a) in figure 13. 1 depicts an upward sloping yield curve, which is the conventional and most observed shape, with bonds of longer maturities attracting higher yields. Econ 512 Dr. Reyadh Faras
13. 1 Yield Curves 13. 1. 1 Yield Curves in Principle § Diversity among bond contracts can be narrowed because yield curves are almost always constructed for government bonds, for which the risk of default is negligible. Thus, their differences are: time to maturity, n, and coupon rate, c/m. § Yield curves are constructed for ZC bonds, the spot yield is plotted against the time to maturity. § Panel (a) in figure 13. 1 depicts an upward sloping yield curve, which is the conventional and most observed shape, with bonds of longer maturities attracting higher yields. Econ 512 Dr. Reyadh Faras
 § The rationale is that bonds with many years to redemption are riskier than bonds near maturity. § Hence, L-T bonds command premia, as reflected in higher yields, relative to S-T bonds. Consequently, the yield curve is positively sloped. § Yield curves are not necessarily positively sloped. § They are, on occasion, observed to slope downwards, with S-T bond yields exceed L-T ones. § Yield curves can also be flat, i. e. same y for all n. § In principle there is no reason why yield curves should be monotonic throughout. Econ 512 Dr. Reyadh Faras
§ The rationale is that bonds with many years to redemption are riskier than bonds near maturity. § Hence, L-T bonds command premia, as reflected in higher yields, relative to S-T bonds. Consequently, the yield curve is positively sloped. § Yield curves are not necessarily positively sloped. § They are, on occasion, observed to slope downwards, with S-T bond yields exceed L-T ones. § Yield curves can also be flat, i. e. same y for all n. § In principle there is no reason why yield curves should be monotonic throughout. Econ 512 Dr. Reyadh Faras
 § The curve could be negatively sloped for some maturities and positively sloped for others (panel (b) in figure 13. 1). § Even though this is uncommon, but when observed they are usually attributed to specific or peculiar (unusual) events in bond markets, often following an abrupt (sudden) reversal or intensification of monetary policy. Econ 512 Dr. Reyadh Faras
§ The curve could be negatively sloped for some maturities and positively sloped for others (panel (b) in figure 13. 1). § Even though this is uncommon, but when observed they are usually attributed to specific or peculiar (unusual) events in bond markets, often following an abrupt (sudden) reversal or intensification of monetary policy. Econ 512 Dr. Reyadh Faras
 13. 3 Implicit Forward Rates § Implicit forward rates are indicators of future interest rates inferred from observed bond prices. § They provide a way of characterizing the term structure of interest rates that is equivalent to the yield curve: for each sequence of spot yields (one for each maturity) there exists a unique sequence of implicit forward rates, and vice versa. § Explicit forward rates are interest rates relevant for agreements made today on loans that begin and end at specific future dates. § Implicit forward rates are forecasts of interest rates on loans that will begin and end in the future. Econ 512 Dr. Reyadh Faras
13. 3 Implicit Forward Rates § Implicit forward rates are indicators of future interest rates inferred from observed bond prices. § They provide a way of characterizing the term structure of interest rates that is equivalent to the yield curve: for each sequence of spot yields (one for each maturity) there exists a unique sequence of implicit forward rates, and vice versa. § Explicit forward rates are interest rates relevant for agreements made today on loans that begin and end at specific future dates. § Implicit forward rates are forecasts of interest rates on loans that will begin and end in the future. Econ 512 Dr. Reyadh Faras
 § Being forecasts, there is no guarantee that the implicit forward rates will be realized when the future arrives. § To define an implicit forward rate, consider the investment of $1 in a ZC bond that matures in 5 years from the present. § Alternatively, suppose that $1 is invested in a 4 year ZC bond, followed by an investment of the proceeds in a 1 -year bond. § The 1 -year rate that results in the same payoff after five years for both strategies is the implicit forward rate between years 4 and 5. § Note: Markets are assumed to be frictionless. Econ 512 Dr. Reyadh Faras
§ Being forecasts, there is no guarantee that the implicit forward rates will be realized when the future arrives. § To define an implicit forward rate, consider the investment of $1 in a ZC bond that matures in 5 years from the present. § Alternatively, suppose that $1 is invested in a 4 year ZC bond, followed by an investment of the proceeds in a 1 -year bond. § The 1 -year rate that results in the same payoff after five years for both strategies is the implicit forward rate between years 4 and 5. § Note: Markets are assumed to be frictionless. Econ 512 Dr. Reyadh Faras
 § More formally, consider the investment of $1 in a ZC bond that matures in n years from the present. § After n years, when the bond matures, the investment will be worth (1+yn)n, where yn is the spot yield on an n-period bond purchased today. § Similarly, $1 invested in a ZC bond that matures n -1 years from today will accumulate to (1+yn-1)n-1 , after n-1 years. § The implicit forward rate between n-1 and n years is the interest rate that equates the payoffs from the two strategies. Econ 512 Dr. Reyadh Faras
§ More formally, consider the investment of $1 in a ZC bond that matures in n years from the present. § After n years, when the bond matures, the investment will be worth (1+yn)n, where yn is the spot yield on an n-period bond purchased today. § Similarly, $1 invested in a ZC bond that matures n -1 years from today will accumulate to (1+yn-1)n-1 , after n-1 years. § The implicit forward rate between n-1 and n years is the interest rate that equates the payoffs from the two strategies. Econ 512 Dr. Reyadh Faras
 § In other words, the implicit forward rate of return that would be received from investing the proceeds of the (n-1)-year ZC bond, at maturity, for one more year if the rate of return over n=(n-1)+1 years exactly equals the yield from holding an n-year ZC bond to maturity. § In symbols, the implicit one-year interest rate beginning n-1 years from today, n-1 fn, is defined to satisfy: (1+yn)n = (1+yn-1)n-1 (1+ n-1 fn) (13. 1) § Rearranging (13. 1), the implicit forward rate is: n-1 fn = § That’s how the implicit forward rate is calculated from the prices of ZC bond with the 2 relevant maturities. Econ 512 Dr. Reyadh Faras
§ In other words, the implicit forward rate of return that would be received from investing the proceeds of the (n-1)-year ZC bond, at maturity, for one more year if the rate of return over n=(n-1)+1 years exactly equals the yield from holding an n-year ZC bond to maturity. § In symbols, the implicit one-year interest rate beginning n-1 years from today, n-1 fn, is defined to satisfy: (1+yn)n = (1+yn-1)n-1 (1+ n-1 fn) (13. 1) § Rearranging (13. 1), the implicit forward rate is: n-1 fn = § That’s how the implicit forward rate is calculated from the prices of ZC bond with the 2 relevant maturities. Econ 512 Dr. Reyadh Faras
 Example If: P 5 =60, p 4 = 66, m = 100. Then: 4 f 5 = (66/60) – 1 = 10% § If the investor expects the 1 -year interest rate 4 years from now to exceed 10%, he can make a speculative profit by selling 5 -year bonds, and investing the funds in 4 -year bonds. After 4 years, invest the payoff at the 1 -year interest rate. § If the investor’s expectations turned out true, a profit would be made after 5 years following the redemption of the 5 -year bonds. § This investment strategy is risky: there is no guarantee that the investor’s expectations will be realized. § Thus, implicit forward rates are important as they enable inferences about interest rates applicable for loans over intervals in the future, which are used to determine which bonds to buy or sell. Econ 512 Dr. Reyadh Faras
Example If: P 5 =60, p 4 = 66, m = 100. Then: 4 f 5 = (66/60) – 1 = 10% § If the investor expects the 1 -year interest rate 4 years from now to exceed 10%, he can make a speculative profit by selling 5 -year bonds, and investing the funds in 4 -year bonds. After 4 years, invest the payoff at the 1 -year interest rate. § If the investor’s expectations turned out true, a profit would be made after 5 years following the redemption of the 5 -year bonds. § This investment strategy is risky: there is no guarantee that the investor’s expectations will be realized. § Thus, implicit forward rates are important as they enable inferences about interest rates applicable for loans over intervals in the future, which are used to determine which bonds to buy or sell. Econ 512 Dr. Reyadh Faras
 13. 4 The Expectations Hypothesis of the Term Structure § The expectations hypothesis provides a starting point for all explanations of the term structure. § It asserts that expectations about future bond yields determine the shape of the yield curve. § Consider a world in which 1 -year (S-T) and 2 -year (L -T) ZC bonds are traded. § Suppose that the yields on both are equal. § If the yield on 1 -year bonds is expected to rise in the future, investors may prefer to hold 1 -year bonds so that, when they mature, the proceeds can be reinvested in one year bonds commencing next year, thus benefiting from the higher expected yield in the future. Econ 512 Dr. Reyadh Faras
13. 4 The Expectations Hypothesis of the Term Structure § The expectations hypothesis provides a starting point for all explanations of the term structure. § It asserts that expectations about future bond yields determine the shape of the yield curve. § Consider a world in which 1 -year (S-T) and 2 -year (L -T) ZC bonds are traded. § Suppose that the yields on both are equal. § If the yield on 1 -year bonds is expected to rise in the future, investors may prefer to hold 1 -year bonds so that, when they mature, the proceeds can be reinvested in one year bonds commencing next year, thus benefiting from the higher expected yield in the future. Econ 512 Dr. Reyadh Faras
 § This preference would be expressed by investors selling 2 -year bonds and buying 1 -year bonds, hence leading to an equilibrium in which the price of the 2 year bonds is lower, and the price of the 1 -year bonds is higher, than otherwise. § Given the inverse relationship between yields and prices, the equilibrium yield on 2 -year bonds becomes higher than the yield on one 1 -year bonds. § Hence, theory predict that the yield curve has a: ü Positive slope if investors expect interest rates to rise. ü Negative slope if investors expect interest rates to fall. ü Be flat if the 1 -year yield is expected to remain at its current level. Econ 512 Dr. Reyadh Faras
§ This preference would be expressed by investors selling 2 -year bonds and buying 1 -year bonds, hence leading to an equilibrium in which the price of the 2 year bonds is lower, and the price of the 1 -year bonds is higher, than otherwise. § Given the inverse relationship between yields and prices, the equilibrium yield on 2 -year bonds becomes higher than the yield on one 1 -year bonds. § Hence, theory predict that the yield curve has a: ü Positive slope if investors expect interest rates to rise. ü Negative slope if investors expect interest rates to fall. ü Be flat if the 1 -year yield is expected to remain at its current level. Econ 512 Dr. Reyadh Faras
 § Let pn, t denote the price today, t, of a ZC bond with n years to maturity. Let yn, t denote its spot yield. § After one year, at t+1, the same bond will have n-1 years to maturity. § Its price and spot yields are then written as pn-1, t+1 and yn-1, t+1. § Now compare ZC bonds with 1 -year and 2 -years to maturity. § There are three bonds: (i) 1 -year bonds available today, yielding y 1, t; (ii) 1 -year bonds issued at t+1, yielding y 1, t+1; and (iii) 2 -year bond available today, yielding y 2, t. Econ 512 Dr. Reyadh Faras
§ Let pn, t denote the price today, t, of a ZC bond with n years to maturity. Let yn, t denote its spot yield. § After one year, at t+1, the same bond will have n-1 years to maturity. § Its price and spot yields are then written as pn-1, t+1 and yn-1, t+1. § Now compare ZC bonds with 1 -year and 2 -years to maturity. § There are three bonds: (i) 1 -year bonds available today, yielding y 1, t; (ii) 1 -year bonds issued at t+1, yielding y 1, t+1; and (iii) 2 -year bond available today, yielding y 2, t. Econ 512 Dr. Reyadh Faras
 § By assumption all 3 rates are known today. § Hence, it seems reasonable to claim that in market equilibrium the payoff from investing $1 in a 2 -year bond must equal that from investing $1 in a 1 -year bond and then reinvesting the proceeds in another 1 year bond for the second year: (1+y 2, t)2 = (1+y 1, t) (1+ y 1, t+1) (13. 8) § If this equality does not hold, then investors have an incentive either to issue 2 -year bonds and invest the proceeds in two successive 1 -year bonds, or to issue two successive 1 -year bonds and invest the proceeds in 2 -year bonds. Econ 512 Dr. Reyadh Faras
§ By assumption all 3 rates are known today. § Hence, it seems reasonable to claim that in market equilibrium the payoff from investing $1 in a 2 -year bond must equal that from investing $1 in a 1 -year bond and then reinvesting the proceeds in another 1 year bond for the second year: (1+y 2, t)2 = (1+y 1, t) (1+ y 1, t+1) (13. 8) § If this equality does not hold, then investors have an incentive either to issue 2 -year bonds and invest the proceeds in two successive 1 -year bonds, or to issue two successive 1 -year bonds and invest the proceeds in 2 -year bonds. Econ 512 Dr. Reyadh Faras
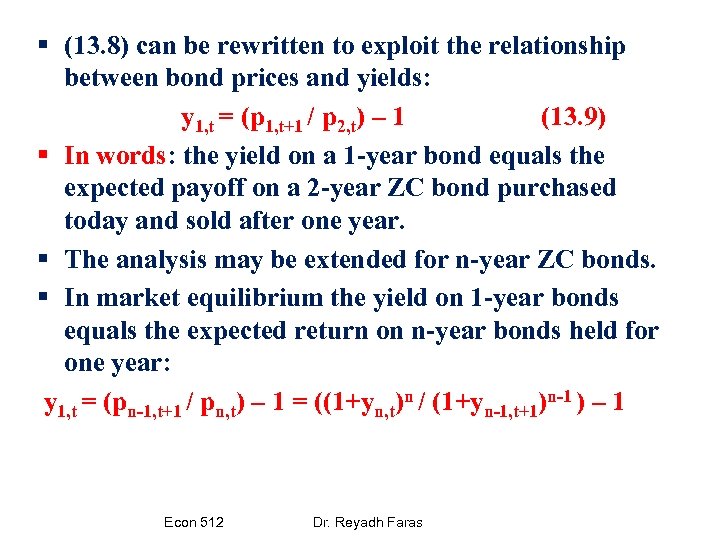 § (13. 8) can be rewritten to exploit the relationship between bond prices and yields: y 1, t = (p 1, t+1 / p 2, t) – 1 (13. 9) § In words: the yield on a 1 -year bond equals the expected payoff on a 2 -year ZC bond purchased today and sold after one year. § The analysis may be extended for n-year ZC bonds. § In market equilibrium the yield on 1 -year bonds equals the expected return on n-year bonds held for one year: y 1, t = (pn-1, t+1 / pn, t) – 1 = ((1+yn, t)n / (1+yn-1, t+1)n-1 ) – 1 Econ 512 Dr. Reyadh Faras
§ (13. 8) can be rewritten to exploit the relationship between bond prices and yields: y 1, t = (p 1, t+1 / p 2, t) – 1 (13. 9) § In words: the yield on a 1 -year bond equals the expected payoff on a 2 -year ZC bond purchased today and sold after one year. § The analysis may be extended for n-year ZC bonds. § In market equilibrium the yield on 1 -year bonds equals the expected return on n-year bonds held for one year: y 1, t = (pn-1, t+1 / pn, t) – 1 = ((1+yn, t)n / (1+yn-1, t+1)n-1 ) – 1 Econ 512 Dr. Reyadh Faras
 13. 5 Allowing for risk preferences in the term structure 13. 5. 1 The liquidity preference theory of term structure § Hicks was the first to modify the expectations hypothesis, it is known as the liquidity preference, liquidity premium or risk premium theory. § The theory asserts that, while investors are influenced by expectations of future rates of return, they are riskaverse when making decisions about which bonds to hold (or to issue). § Risk aversion may imply that investors prefer to hold short-term, liquid, assets unless a premium is included in the return expected from long-term assets: bonds nearing maturity would be preferred to bonds for which maturity is far off. Econ 512 Dr. Reyadh Faras
13. 5 Allowing for risk preferences in the term structure 13. 5. 1 The liquidity preference theory of term structure § Hicks was the first to modify the expectations hypothesis, it is known as the liquidity preference, liquidity premium or risk premium theory. § The theory asserts that, while investors are influenced by expectations of future rates of return, they are riskaverse when making decisions about which bonds to hold (or to issue). § Risk aversion may imply that investors prefer to hold short-term, liquid, assets unless a premium is included in the return expected from long-term assets: bonds nearing maturity would be preferred to bonds for which maturity is far off. Econ 512 Dr. Reyadh Faras
 § Consequently, long-term bonds would be held only if the expected payoff from holding them exceeds that on short term bonds. § Thus, the bond price pattern reflects the premia demanded by investors if the aggregate demand to hold bonds with different maturities is to match the supply. § The premia are referred to as risk, liquidity or term premia. § Two characterizations of term premia deserve mention: First: Premia are defined as differences between implicit forward rates and relevant expected future interest rate: λ 2= 1 f 2 - Et[y 1, t+1] Econ 512 Dr. Reyadh Faras
§ Consequently, long-term bonds would be held only if the expected payoff from holding them exceeds that on short term bonds. § Thus, the bond price pattern reflects the premia demanded by investors if the aggregate demand to hold bonds with different maturities is to match the supply. § The premia are referred to as risk, liquidity or term premia. § Two characterizations of term premia deserve mention: First: Premia are defined as differences between implicit forward rates and relevant expected future interest rate: λ 2= 1 f 2 - Et[y 1, t+1] Econ 512 Dr. Reyadh Faras
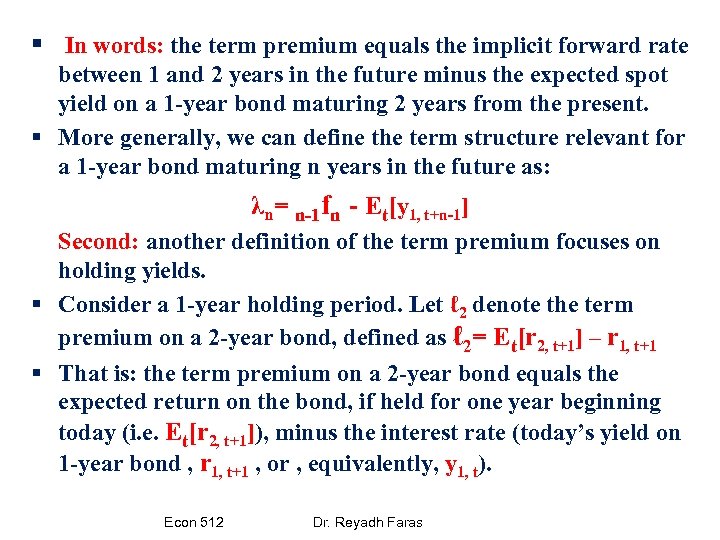 § In words: the term premium equals the implicit forward rate between 1 and 2 years in the future minus the expected spot yield on a 1 -year bond maturing 2 years from the present. § More generally, we can define the term structure relevant for a 1 -year bond maturing n years in the future as: λn= n-1 fn - Et[y 1, t+n-1] Second: another definition of the term premium focuses on holding yields. § Consider a 1 -year holding period. Let ℓ 2 denote the term premium on a 2 -year bond, defined as ℓ 2= Et[r 2, t+1] – r 1, t+1 § That is: the term premium on a 2 -year bond equals the expected return on the bond, if held for one year beginning today (i. e. Et[r 2, t+1]), minus the interest rate (today’s yield on 1 -year bond , r 1, t+1 , or , equivalently, y 1, t). Econ 512 Dr. Reyadh Faras
§ In words: the term premium equals the implicit forward rate between 1 and 2 years in the future minus the expected spot yield on a 1 -year bond maturing 2 years from the present. § More generally, we can define the term structure relevant for a 1 -year bond maturing n years in the future as: λn= n-1 fn - Et[y 1, t+n-1] Second: another definition of the term premium focuses on holding yields. § Consider a 1 -year holding period. Let ℓ 2 denote the term premium on a 2 -year bond, defined as ℓ 2= Et[r 2, t+1] – r 1, t+1 § That is: the term premium on a 2 -year bond equals the expected return on the bond, if held for one year beginning today (i. e. Et[r 2, t+1]), minus the interest rate (today’s yield on 1 -year bond , r 1, t+1 , or , equivalently, y 1, t). Econ 512 Dr. Reyadh Faras
![§ More generally, the term premium on n-year bond is: ℓn= Et[rn, t+1] – § More generally, the term premium on n-year bond is: ℓn= Et[rn, t+1] –](https://present5.com/presentation/9cef657eff7c05e2a81f0aee536170f6/image-19.jpg) § More generally, the term premium on n-year bond is: ℓn= Et[rn, t+1] – r 1, t+1 § The term structure can be expressed as: Et[rn, t+1] - ℓn = Et[rn-1, t+1] - ℓn-1 = …= Et[r 2, t+1] - ℓ 2 = r 1, t+1 § Hicks argues that the 5 -year bond, being riskier than the 1 -year bond over the ensuing year, has a higher expected holding period yield (positive term premium): Et[r 5, t+1] > r 1, t+1 § The difference being the term premium, ℓ 5. § Hicks theory predicts that term premia have a positive relationship with time to maturity: ℓn > ℓn -1 > …> ℓ 2> 0. § There is asymmetry in preferences: lenders prefer to hold short-term bonds, while borrowers prefer to issue long-term bonds. Econ 512 Dr. Reyadh Faras
§ More generally, the term premium on n-year bond is: ℓn= Et[rn, t+1] – r 1, t+1 § The term structure can be expressed as: Et[rn, t+1] - ℓn = Et[rn-1, t+1] - ℓn-1 = …= Et[r 2, t+1] - ℓ 2 = r 1, t+1 § Hicks argues that the 5 -year bond, being riskier than the 1 -year bond over the ensuing year, has a higher expected holding period yield (positive term premium): Et[r 5, t+1] > r 1, t+1 § The difference being the term premium, ℓ 5. § Hicks theory predicts that term premia have a positive relationship with time to maturity: ℓn > ℓn -1 > …> ℓ 2> 0. § There is asymmetry in preferences: lenders prefer to hold short-term bonds, while borrowers prefer to issue long-term bonds. Econ 512 Dr. Reyadh Faras
 13. 5. 2 The preferred habitat theory of the term structure § The preferred habitat or hedging pressure theory refines the liquidity preference theory to allow for differing preferences among lenders and borrowers with respect to the maturity of the bonds they hold or issue. § The main implication of this theory is that expectations of future interest rates are not exclusively responsible for the pattern of current bond yields: the stocks of bonds, and investor’s demands to hold them, also influence the term structure. § If the differentials in bond prices are large enough then investors may choose maturities different from those that they most prefer, but they have to be offered an incentive to do so. Econ 512 Dr. Reyadh Faras
13. 5. 2 The preferred habitat theory of the term structure § The preferred habitat or hedging pressure theory refines the liquidity preference theory to allow for differing preferences among lenders and borrowers with respect to the maturity of the bonds they hold or issue. § The main implication of this theory is that expectations of future interest rates are not exclusively responsible for the pattern of current bond yields: the stocks of bonds, and investor’s demands to hold them, also influence the term structure. § If the differentials in bond prices are large enough then investors may choose maturities different from those that they most prefer, but they have to be offered an incentive to do so. Econ 512 Dr. Reyadh Faras
 § The dispersion (spreading) of risk preferences and stocks of bonds with different maturities can exert an impact on the term structure. § As extreme version of theory – the segmented markets hypothesis – asserts that bonds with different times to maturity can be grouped together, such that prices of bonds with each group are related to one another but not to the prices of bonds belonging to other groups. § For example, monetary policy (acting on S-T interest rates) might impact upon bond prices with shorter maturities only. While actions of pension funds (holding long-term bonds) might impact prices of longer term bond but not those with shorter maturities. Econ 512 Dr. Reyadh Faras
§ The dispersion (spreading) of risk preferences and stocks of bonds with different maturities can exert an impact on the term structure. § As extreme version of theory – the segmented markets hypothesis – asserts that bonds with different times to maturity can be grouped together, such that prices of bonds with each group are related to one another but not to the prices of bonds belonging to other groups. § For example, monetary policy (acting on S-T interest rates) might impact upon bond prices with shorter maturities only. While actions of pension funds (holding long-term bonds) might impact prices of longer term bond but not those with shorter maturities. Econ 512 Dr. Reyadh Faras


