Chapter 9 The Time Value of Money

Chapter 9 The Time Value of Money

Chapter 9 - Outline Time Value of Money Perpetuity Future Value and Present Value Effective Annual Rate (EAR) Annuity

Time Value of Money The basic idea behind the concept of time value of money is: – $1 received today is worth more than $1 in the future OR – $1 received in the future is worth less than $1 today Why? – because interest can be earned on the money The connecting piece or link between present (today) and future is the interest rate

2 Questions to Ask in Time Value of Money Problems Future Value or Present Value? Future Value: Present (Now) Future Present Value: Future Present (Now) Single amount or Annuity? Single amount: one-time (or lump) sum Annuity: same amount per year for a number of years

Perpetuity: Constant Payment Forever PV = PMT/i This is the present value of receiving a constant payment forever.

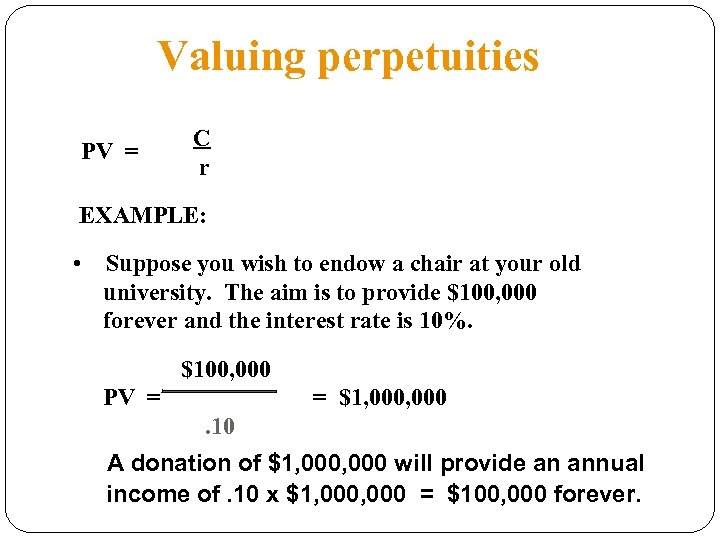Valuing perpetuities PV = C r EXAMPLE: • Suppose you wish to endow a chair at your old university. The aim is to provide $100, 000 forever and the interest rate is 10%. $100, 000 PV = = $1, 000. 10 A donation of $1, 000 will provide an annual income of. 10 x $1, 000 = $100, 000 forever.

Future Value and Present Value Future Value (FV) is what money today will be worth at some point in the future FV = PV x FVIF is the future value interest factor Present Value (PV) is what money at some point in the future is worth today PV = FV x PVIF is the present value interest factor

Future Value of a Lump Sum FV = PV * (1+i)n Why is this formula correct? This is the amount that will be accumulated by investing a given amount today for n periods at a given interest rate.

Simple & compound interest Simple interest rates) are calculated by multiplying the rate period by the number of periods. Compound interest rates recognize the opportunity to earn interest on interest. i ii Periods Interest per year period 1 6% iii APR (i x ii) iv Value after one year 6% 1. 06 v Annually compounded interest rate 6. 000% 2 3 6 1. 032 = 1. 0609 6. 090 4 1. 5 6 1. 0154 = 1. 06136 6. 136 12 . 5 6 1. 00512 = 1. 06168 6. 168 52 . 1154 6 1. 00115452 = 1. 06180 6. 180 365 . 0164 6 1. 000164365 = 1. 06183 6. 183

Effective Annual Rate (EAR) or Yield (EAY) EAR or EAY = (1+inom/m)m-1 This is used to calculate the compounded yearly rate. It considers interest being earned on interest.

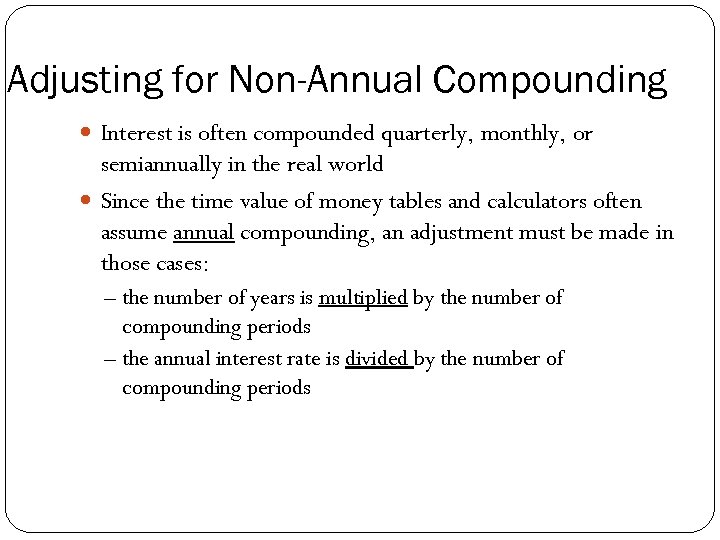Adjusting for Non-Annual Compounding Interest is often compounded quarterly, monthly, or semiannually in the real world Since the time value of money tables and calculators often assume annual compounding, an adjustment must be made in those cases: – the number of years is multiplied by the number of compounding periods – the annual interest rate is divided by the number of compounding periods

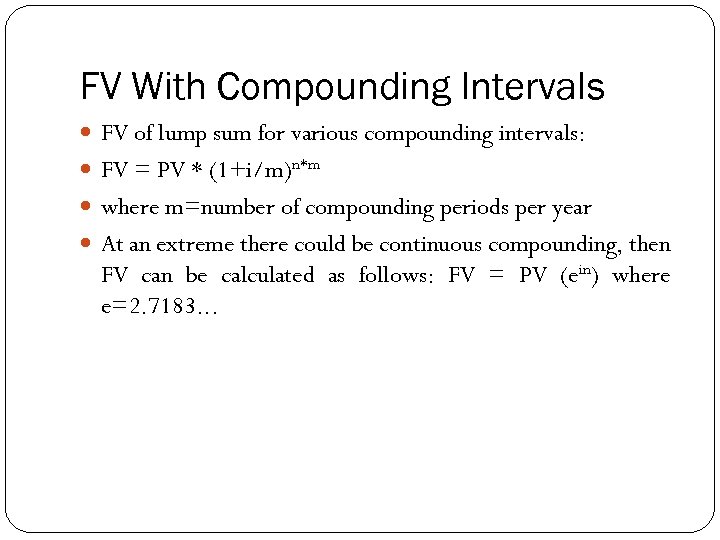FV With Compounding Intervals FV of lump sum for various compounding intervals: FV = PV * (1+i/m)n*m where m=number of compounding periods per year At an extreme there could be continuous compounding, then FV can be calculated as follows: FV = PV (ein) where e=2. 7183. . .

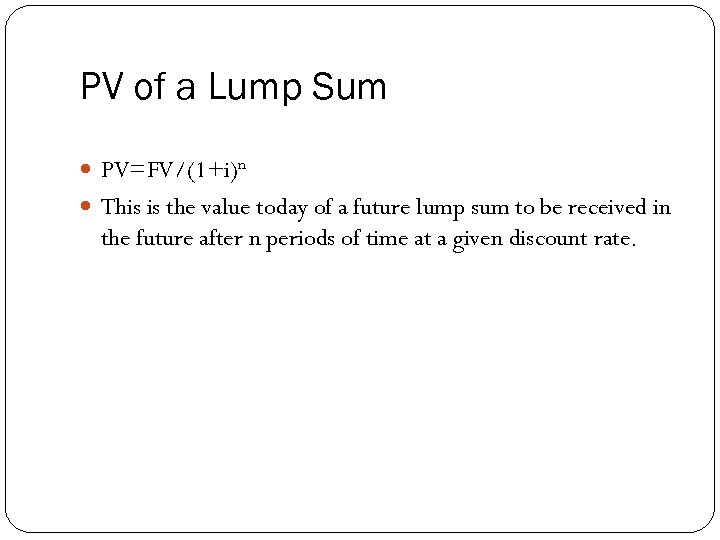PV of a Lump Sum PV=FV/(1+i)n This is the value today of a future lump sum to be received in the future after n periods of time at a given discount rate.

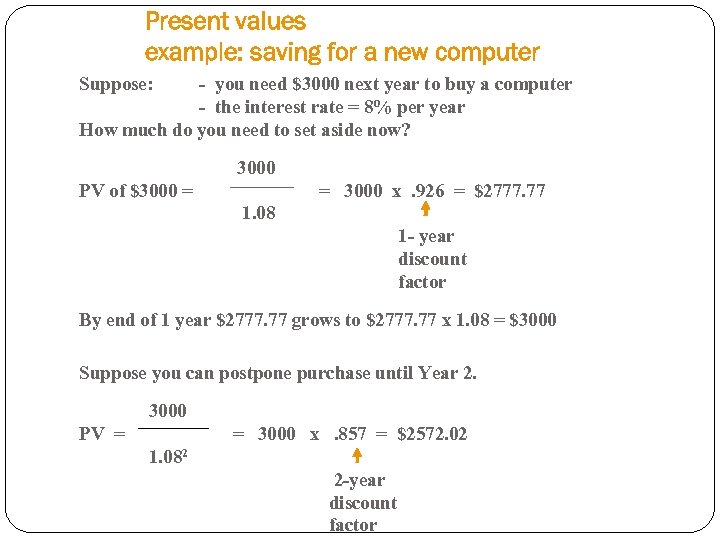Present values example: saving for a new computer Suppose: - you need $3000 next year to buy a computer - the interest rate = 8% per year How much do you need to set aside now? 3000 PV of $3000 = = 3000 x. 926 = $2777. 77 1. 08 1 - year discount factor By end of 1 year $2777. 77 grows to $2777. 77 x 1. 08 = $3000 Suppose you can postpone purchase until Year 2. 3000 PV = = 3000 x. 857 = $2572. 02 1. 082 2 -year discount factor

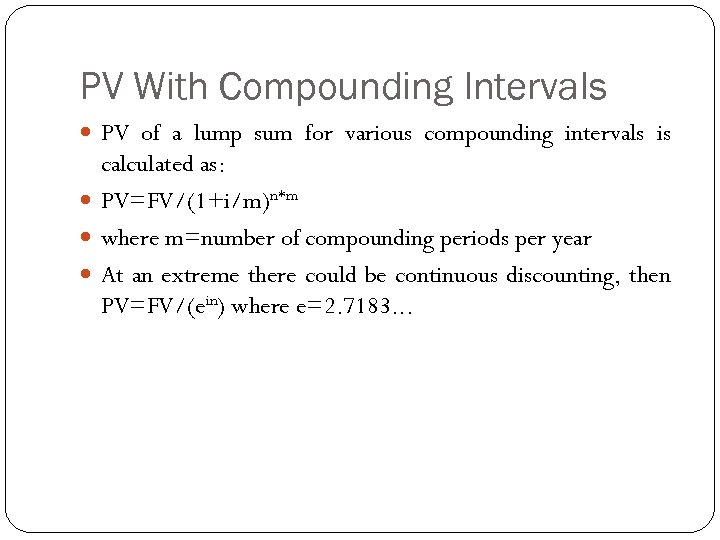PV With Compounding Intervals PV of a lump sum for various compounding intervals is calculated as: PV=FV/(1+i/m)n*m where m=number of compounding periods per year At an extreme there could be continuous discounting, then PV=FV/(ein) where e=2. 7183. . .

PV of an Annuity PV=SA/(1+i)n = A*{(1/i) - (1/i) [1/(1+i)n]} This is the value today of a series of equal payments to be received at the end of each period for n periods at a given interest rate.

An annuity is equal to the difference between two perpetuities Asset Year of payment PV 1 2. . t t+1. . C r Perpetuity (first payment year 1) Perpetuity (first payment year t + 1) Annuity from year 1 to year t C r ( ) C r 1 (1+r) t ( ) 1 (1+r) t

Using the annuity formula Example: valuing an 'easy payment' scheme Suppose: n a car purchase involves 3 annual payments of $4000 n the interest rate is 10% a year 1 PV = $4000 x 1 - . 10(1. 10)3 = $4000 x 2. 487 = $9947. 41 ANNUITY TABLE Number of years 1 2 3 5 10 Interest Rate 5%. 952 1. 859 2. 723 4. 329 7. 722 8% 10% . 926 1. 783 2. 577 3. 993 6. 710 . 909 1. 736 2. 487 3. 791 6. 145

FV of an Annuity FV=SA* (1+i)n = A*{[(1+i)n -1]/i} This is the accumulated value of equal payments for n years at a given interest rate.

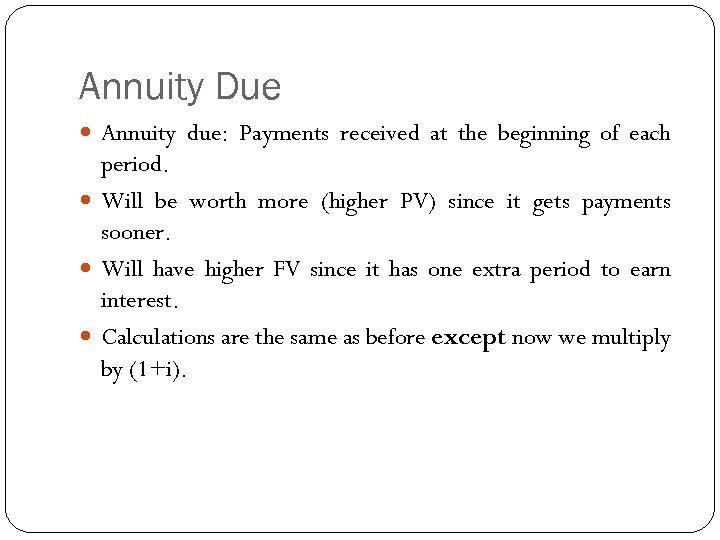Annuity Due Annuity due: Payments received at the beginning of each period. Will be worth more (higher PV) since it gets payments sooner. Will have higher FV since it has one extra period to earn interest. Calculations are the same as before except now we multiply by (1+i).

Solving for Annuity Payments (Present Value) Recall that PV=A*{(1/i) - (1/i) [1/(1+i)n]}, then A=PV/{(1/i) - (1/i) [1/(1+i)n]} A is the payment necessary for n years at given interest rate to amortize a present (loan) amount.

Solving for Annuity Payments (Future Value) Recall that FV=A*{[(1+i)n-1]/i}, then A=FV/{[(1+i)n-1]/i} A is the amount needed to be invested each period at a given interest rate to accumulate a desired future amount at the end of n years.

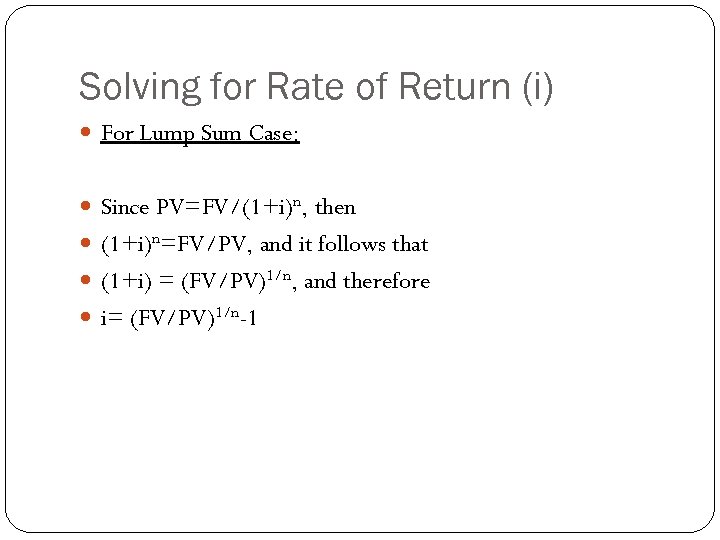Solving for Rate of Return (i) For Lump Sum Case: Since PV=FV/(1+i)n, then (1+i)n=FV/PV, and it follows that (1+i) = (FV/PV)1/n, and therefore i= (FV/PV)1/n-1

Solving for Rate of Return (i) Annuities: In the annuity case, you could also solve for i using annuity relationship once you know the annuity. You do not need a cash flow register.

Solving for Rate of Return (r) with uneven cash flows 0 = C 0 + C 1 (1 + r)1 + C 2 +. . . (1 + r)2 • Spreadsheets (use financial function =IRR) • Financial calculators (IRR using cash flow register) • Manual (Trial and error until PV of all cash flows equal zero)

Solving for Number of Periods (n) Since PV=FV/(1+i)n, then (1+i)n=FV/PV, and it follows that n. LN(1+i)=LN(FV/PV), and therefore n=LN(FV/PV)/LN(1+i)

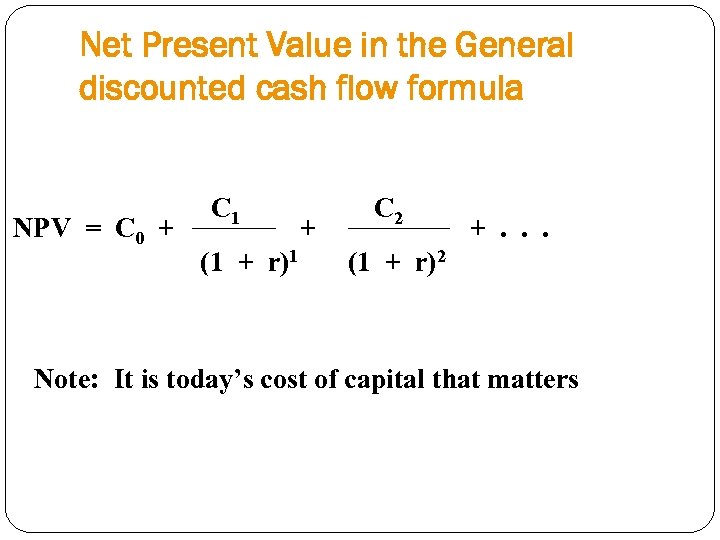Net Present Value in the General discounted cash flow formula NPV = C 0 + C 1 (1 + r)1 + C 2 +. . . (1 + r)2 Note: It is today’s cost of capital that matters

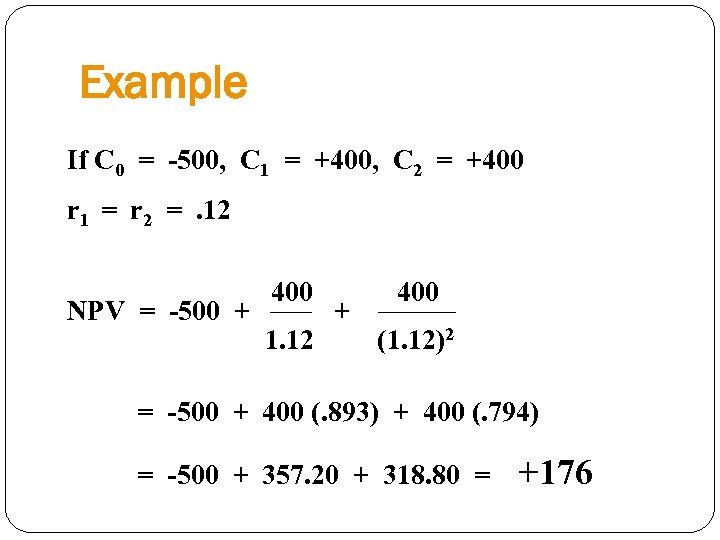Example If C 0 = -500, C 1 = +400, C 2 = +400 r 1 = r 2 =. 12 NPV = -500 + 400 1. 12 + 400 (1. 12)2 = -500 + 400 (. 893) + 400 (. 794) = -500 + 357. 20 + 318. 80 = +176

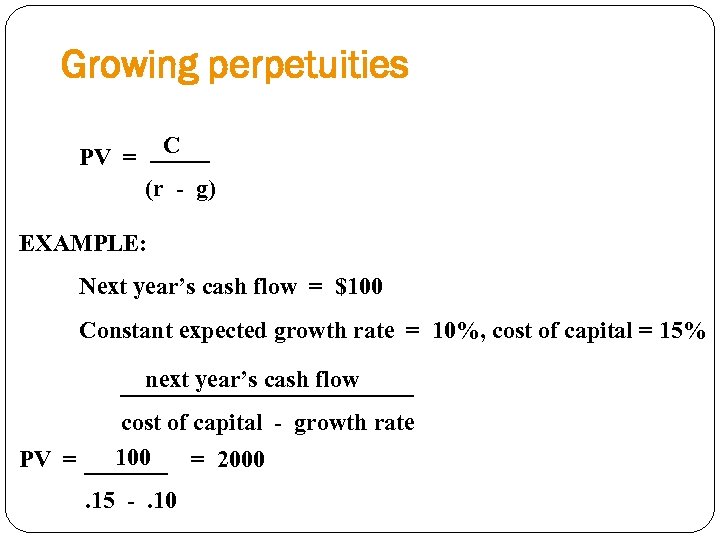Growing perpetuities C PV = (r - g) EXAMPLE: Next year’s cash flow = $100 Constant expected growth rate = 10%, cost of capital = 15% next year’s cash flow PV = cost of capital - growth rate 100 = 2000. 15 -. 10