Chapter 9 Corporate Finance Value of Bond and

Key Concepts and Skills Ø Know the important bond features and bond types Ø Understand bond values and why they fluctuate Ø Understand how stock prices depend on future dividends and dividend growth Ø Be able to compute stock prices using the dividend growth model Ø Understand how growth opportunities affect stock values Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Chapter Outline 9. 1 9. 2 9. 3 9. 4 9. 5 Definitions and Example of a Bond How to Value Bonds Bond Concepts The Present Value of Common Stocks Estimates of Parameters in the Dividend. Discount Model 9. 6 Growth Opportunities 9. 7 The Dividend Growth Model and the NPVGO Model 9. 8 Price-Earnings Ratio 9. 9 Stock Market Reporting Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

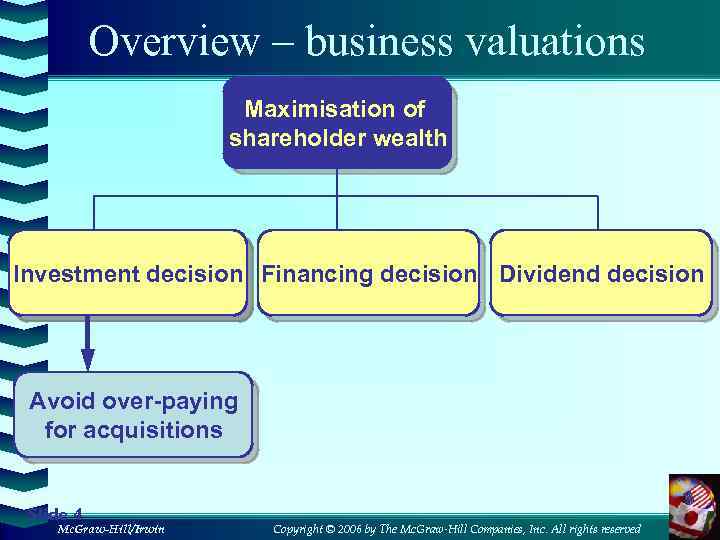
Overview – business valuations Maximisation of shareholder wealth Investment decision Financing decision Dividend decision Avoid over-paying for acquisitions Slide 4 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Review : Time Value 1 Valuation: The One-Period Case 2 The Multiperiod Case 3 Compounding Periods 4 Simplifications 6 What Is a Firm Worth? Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

1 The One-Period Case ØIf you were to invest $10, 000 at 5 -percent interest for one year, your investment would grow to $10, 500. $500 would be interest ($10, 000 ×. 05) $10, 000 is the principal repayment ($10, 000 × 1) $10, 500 is the total due. It can be calculated as: $10, 500 = $10, 000×(1. 05) q. The total amount due at the end of the investment is call the Future Value (FV). Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

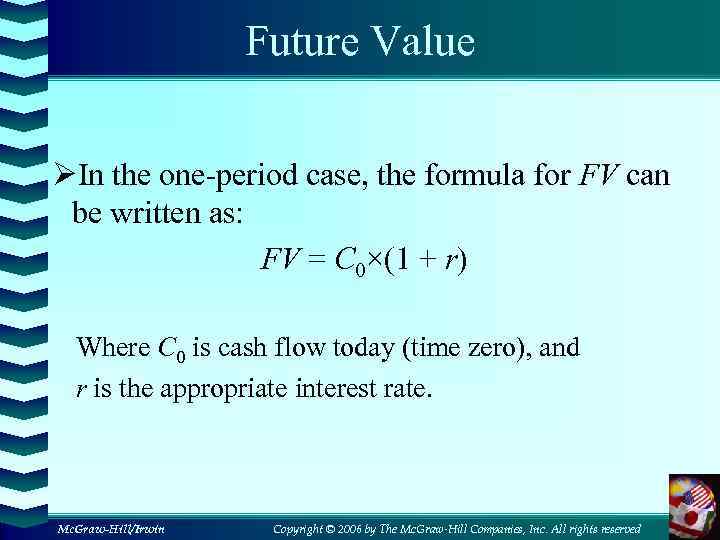
Future Value ØIn the one-period case, the formula for FV can be written as: FV = C 0×(1 + r) Where C 0 is cash flow today (time zero), and r is the appropriate interest rate. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Present Value ØIf you were to be promised $10, 000 due in one year when interest rates are 5 -percent, your investment would be worth $9, 523. 81 in today’s dollars. The amount that a borrower would need to set aside today to be able to meet the promised payment of $10, 000 in one year is called the Present Value (PV). Note that $10, 000 = $9, 523. 81×(1. 05). Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

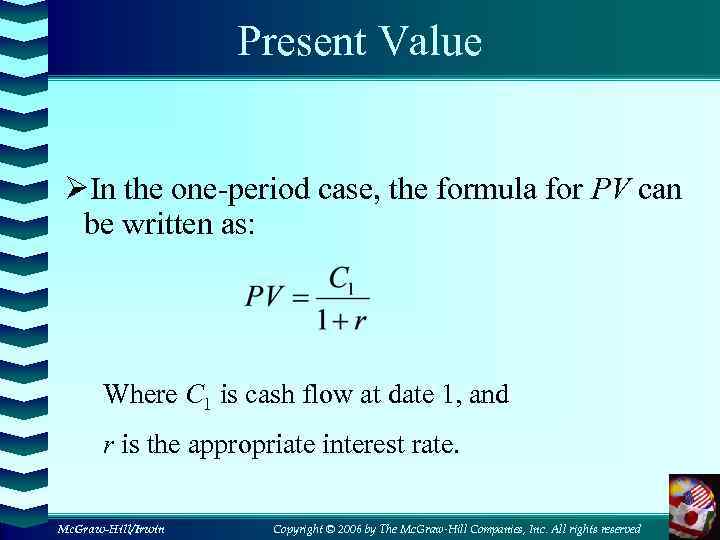
Present Value ØIn the one-period case, the formula for PV can be written as: Where C 1 is cash flow at date 1, and r is the appropriate interest rate. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Net Present Value ØThe Net Present Value (NPV) of an investment is the present value of the expected cash flows, less the cost of the investment. ØSuppose an investment that promises to pay $10, 000 in one year is offered for sale for $9, 500. Your interest rate is 5%. Should you buy? Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Net Present Value The present value of the cash inflow is greater than the cost. In other words, the Net Present Value is positive, so the investment should be purchased. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Net Present Value In the one-period case, the formula for NPV can be written as: NPV = –Cost + PV If we had not undertaken the positive NPV project considered on the last slide, and instead invested our $9, 500 elsewhere at 5 percent, our FV would be less than the $10, 000 the investment promised, and we would be worse off in FV terms : $9, 500×(1. 05) = $9, 975 < $10, 000 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

4. 2 The Multiperiod Case ØThe general formula for the future value of an investment over many periods can be written as: FV = C 0×(1 + r)T Where C 0 is cash flow at date 0, r is the appropriate interest rate, and T is the number of periods over which the cash is invested. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Future Value ØSuppose a stock currently pays a dividend of $1. 10, which is expected to grow at 40% per year for the next five years. ØWhat will the dividend be in five years? FV = C 0×(1 + r)T $5. 92 = $1. 10×(1. 40)5 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Future Value and Compounding ØNotice that the dividend in year five, $5. 92, is considerably higher than the sum of the original dividend plus five increases of 40 percent on the original $1. 10 dividend: $5. 92 > $1. 10 + 5×[$1. 10×. 40] = $3. 30 This is due to compounding. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

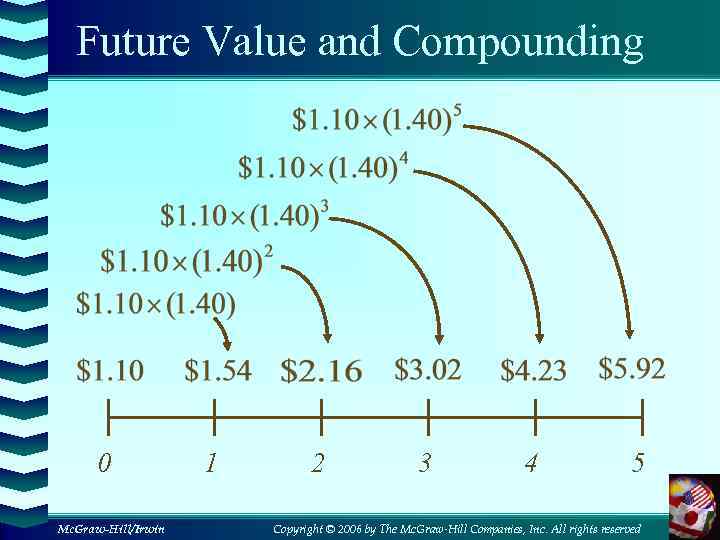
Future Value and Compounding 0 Mc. Graw-Hill/Irwin 1 2 3 4 5 Copyright ©

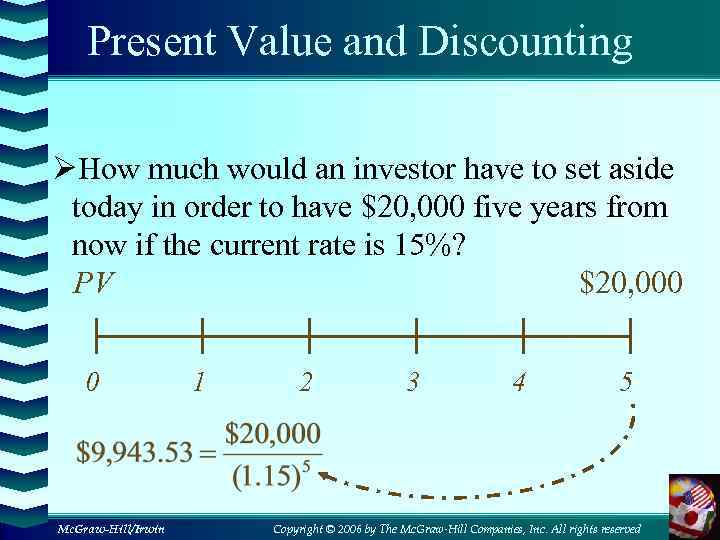
Present Value and Discounting ØHow much would an investor have to set aside today in order to have $20, 000 five years from now if the current rate is 15%? PV $20, 000 0 Mc. Graw-Hill/Irwin 1 2 3 4 5 Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

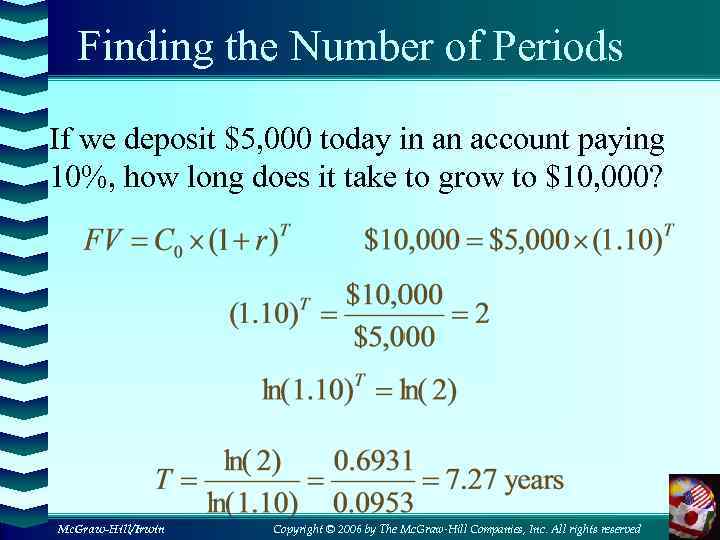
Finding the Number of Periods If we deposit $5, 000 today in an account paying 10%, how long does it take to grow to $10, 000? Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

What Rate Is Enough? Assume the total cost of a college education will be $50, 000 when your child enters college in 12 years. You have $5, 000 to invest today. What rate of interest must you earn on your investment to cover the cost of your child’s education? About 21. 15%. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Multiple Cash Flows ØConsider an investment that pays $200 one year from now, with cash flows increasing by $200 per year through year 4. If the interest rate is 12%, what is the present value of this stream of cash flows? ØIf the issuer offers this investment for $1, 500, should you purchase it? Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

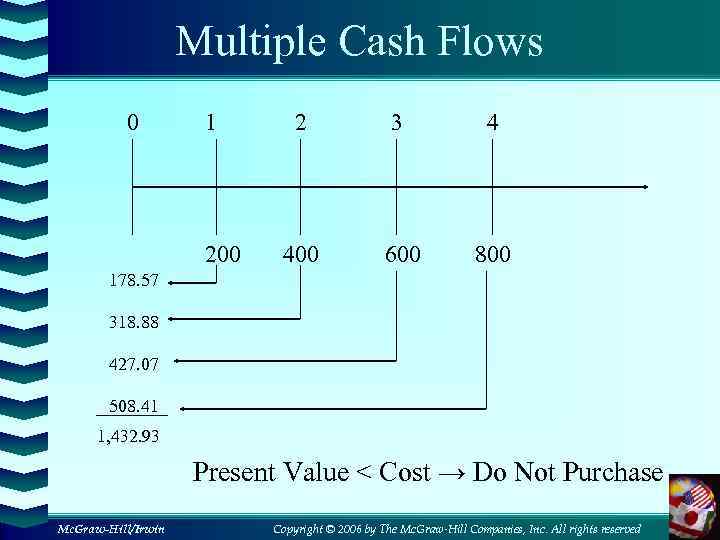
Multiple Cash Flows 0 1 200 2 3 4 400 600 800 178. 57 318. 88 427. 07 508. 41 1, 432. 93 Present Value < Cost → Do Not Purchase Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

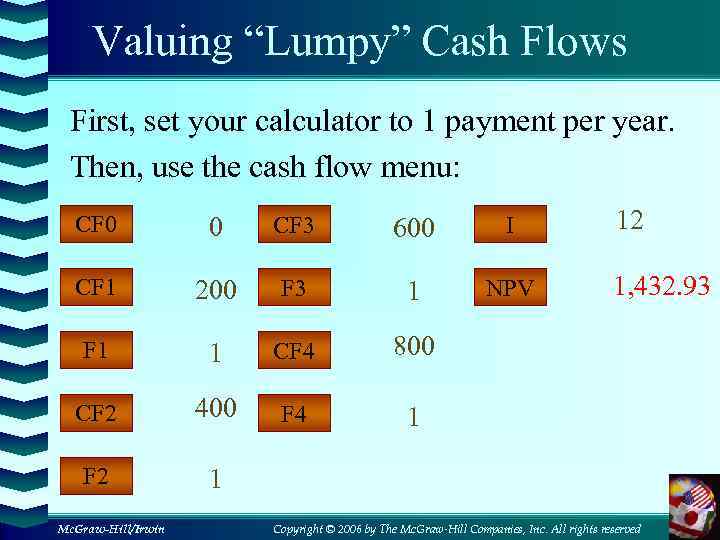
Valuing “Lumpy” Cash Flows First, set your calculator to 1 payment per year. Then, use the cash flow menu: CF 0 0 CF 3 600 I CF 1 200 F 3 1 NPV F 1 1 CF 4 800 CF 2 400 F 4 1 F 2 12 1 Mc. Graw-Hill/Irwin 1, 432. 93 Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

4. 3 Compounding Periods Compounding an investment m times a year for T years provides for future value of wealth: Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Compounding Periods q For example, if you invest $50 for 3 years at 12% compounded semi-annually, your investment will grow to Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Effective Annual Rates of Interest A reasonable question to ask in the above example is “what is the effective annual rate of interest on that investment? ” The Effective Annual Rate (EAR) of interest is the annual rate that would give us the same endof-investment wealth after 3 years: Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Effective Annual Rates of Interest So, investing at 12. 36% compounded annually is the same as investing at 12% compounded semi-annually. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

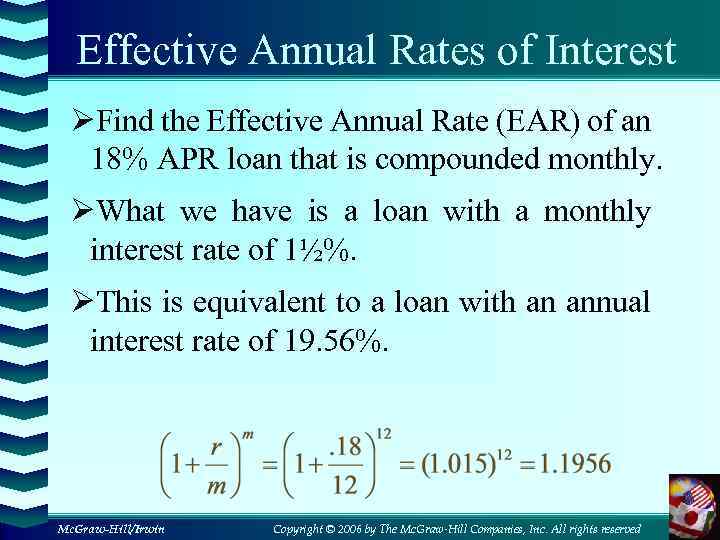
Effective Annual Rates of Interest ØFind the Effective Annual Rate (EAR) of an 18% APR loan that is compounded monthly. ØWhat we have is a loan with a monthly interest rate of 1½%. ØThis is equivalent to a loan with an annual interest rate of 19. 56%. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

EAR on a Financial Calculator Texas Instruments BAII Plus keys: description: [2 nd] [ICONV] Opens interest rate conversion menu Sets 12 payments per year [↑] [C/Y=] 12 [ENTER] Sets 18 APR. [↓][NOM=] 18 [ENTER] [↓] [EFF=] [CPT] Mc. Graw-Hill/Irwin 19. 56 Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

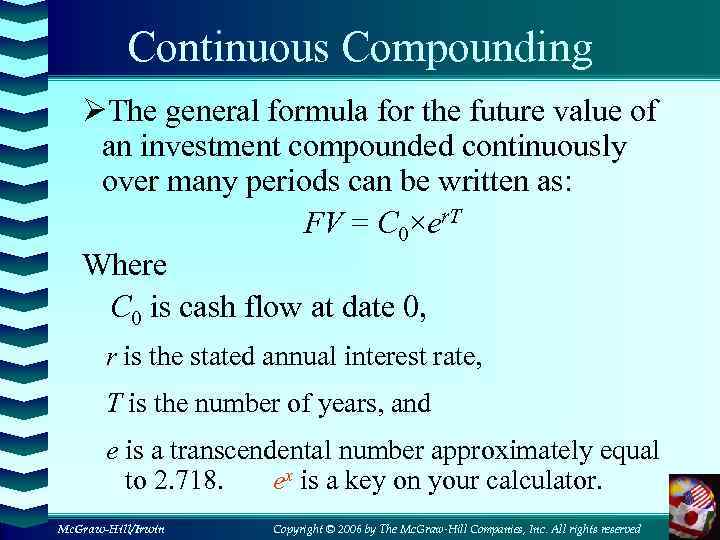
Continuous Compounding ØThe general formula for the future value of an investment compounded continuously over many periods can be written as: FV = C 0×er. T Where C 0 is cash flow at date 0, r is the stated annual interest rate, T is the number of years, and e is a transcendental number approximately equal to 2. 718. ex is a key on your calculator. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

4. 4 Simplifications ØAnnuity – A stream of constant cash flows that lasts for a fixed number of periods ØGrowing annuity – A stream of cash flows that grows at a constant rate for a fixed number of periods ØPerpetuity – A constant stream of cash flows that lasts forever ØGrowing perpetuity – A stream of cash flows that grows at a constant rate forever Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

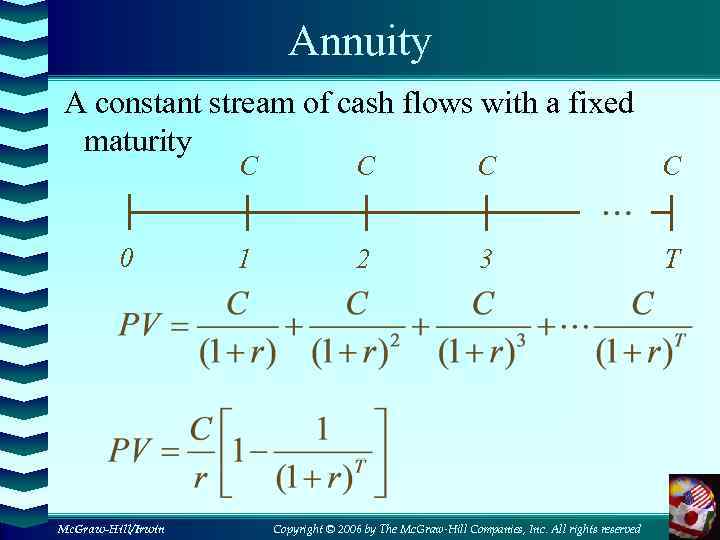
Annuity A constant stream of cash flows with a fixed maturity C 0 Mc.

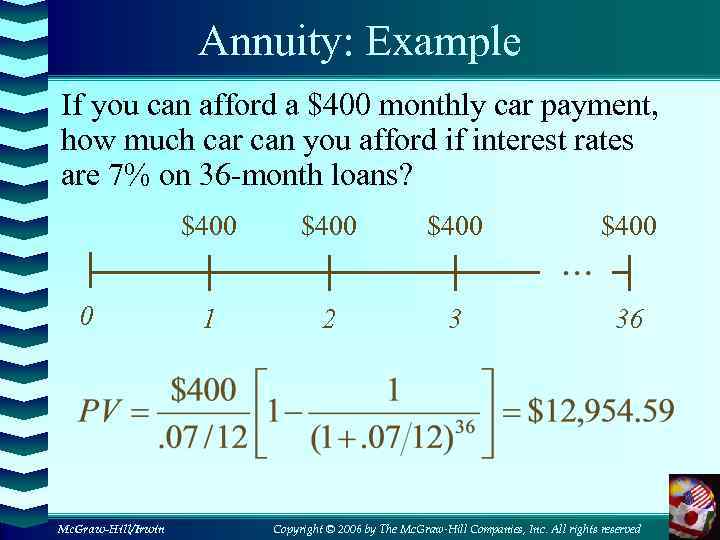
Annuity: Example If you can afford a $400 monthly car payment, how much car can you afford if interest rates are 7% on 36 -month loans? $400 0 Mc. Graw-Hill/Irwin $400 1 2 3 36 Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

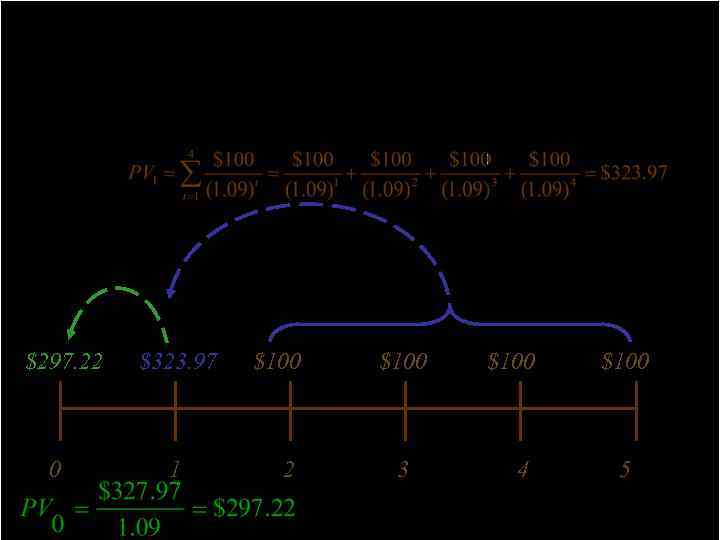
What is the present value of a four-year annuity of $100 per year that makes its first payment two years from today if the discount rate is 9%? $297. 22 $323. 97 0 Mc. Graw-Hill/Irwin 1 $100 2 $100 3 $100 4 $100 5 Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 2 -33

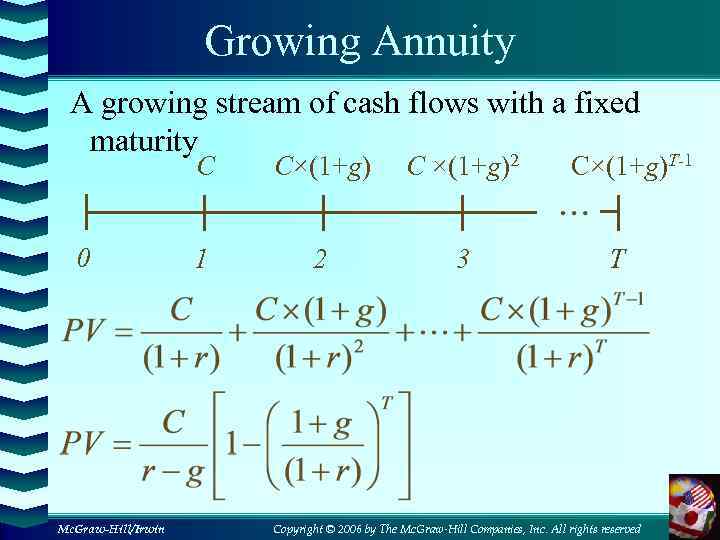
Growing Annuity A growing stream of cash flows with a fixed maturity 2 C 0 Mc. Graw-Hill/Irwin C×(1+g) C ×(1+g) 1 2 3 C×(1+g)T-1 T Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

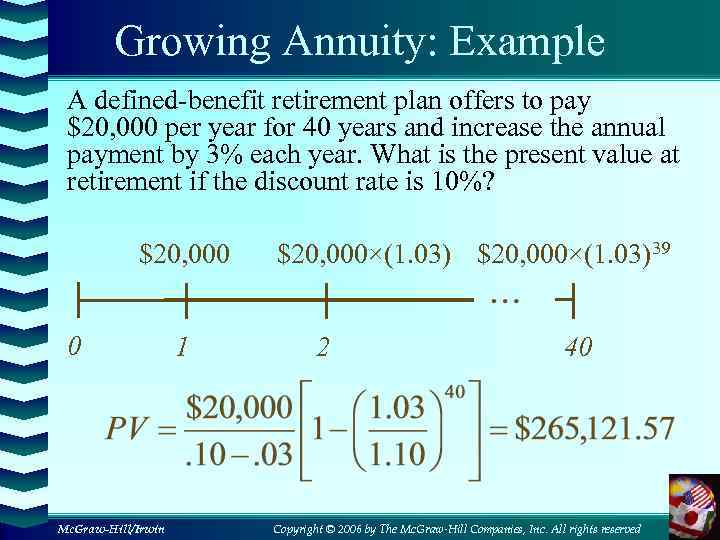
Growing Annuity: Example A defined-benefit retirement plan offers to pay $20, 000 per year for 40 years and increase the annual payment by 3% each year. What is the present value at retirement if the discount rate is 10%? $20, 000 0 Mc. Graw-Hill/Irwin 1 $20, 000×(1. 03)39 2 40 Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Perpetuity A constant stream of cash flows that lasts forever C 0 Mc. Graw-Hill/Irwin

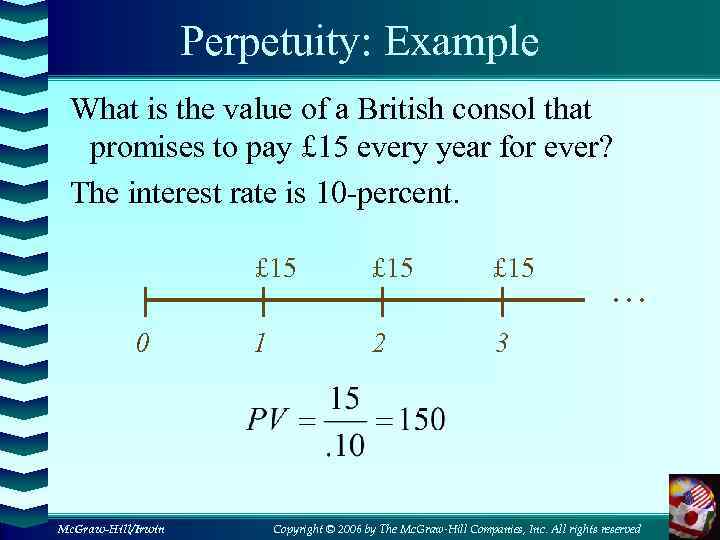
Perpetuity: Example What is the value of a British consol that promises to pay £ 15 every year for ever? The interest rate is 10 -percent. £ 15 0 Mc. Graw-Hill/Irwin £ 15 1 2 3 … Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

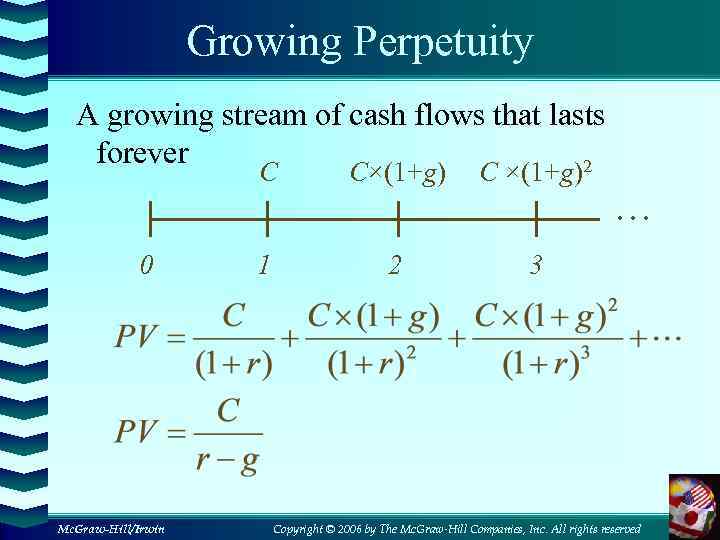
Growing Perpetuity A growing stream of cash flows that lasts forever 2 C 0

Growing Perpetuity: Example The expected dividend next year is $1. 30, and dividends are expected to grow at 5% forever. If the discount rate is 10%, what is the value of this promised dividend stream? $1. 30 0 Mc. Graw-Hill/Irwin 1 $1. 30×(1. 05) 2 $1. 30 ×(1. 05)2 … 3 Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

After reviewing the Time Value, then we go to the main body of this

9. 1 Definition of a Bond Ø A bond is a legally binding agreement between a borrower and a lender that specifies the: – Par (face) value – Coupon rate – Coupon payment – Maturity Date Ø The yield to maturity is the required market interest rate on the bond. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

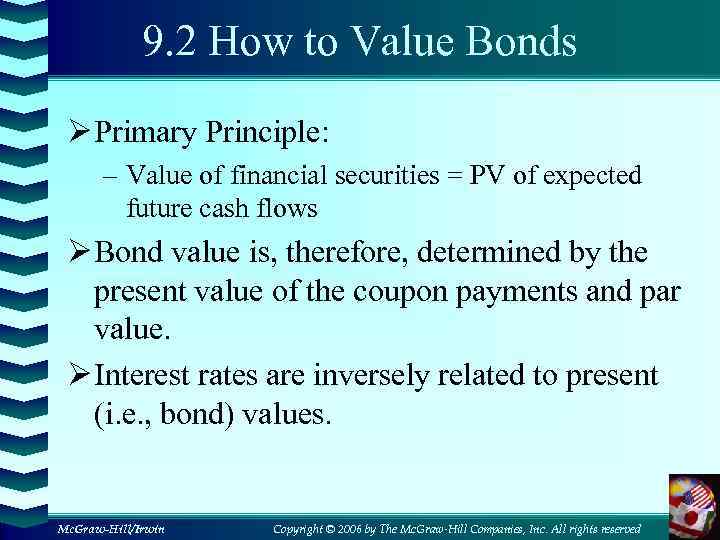
9. 2 How to Value Bonds Ø Primary Principle: – Value of financial securities = PV of expected future cash flows Ø Bond value is, therefore, determined by the present value of the coupon payments and par value. Ø Interest rates are inversely related to present (i. e. , bond) values. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

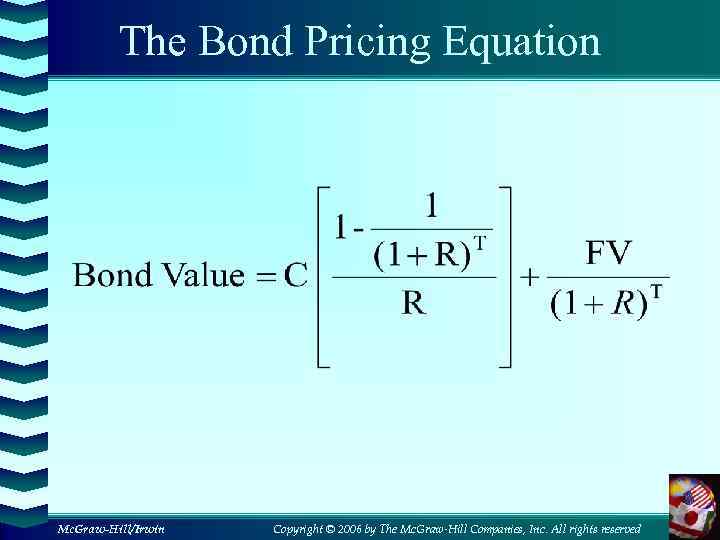
The Bond Pricing Equation Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies,

Pure Discount Bonds Ø Make no periodic interest payments (coupon rate = 0%) Ø The entire yield to maturity comes from the difference between the purchase price and the par value. Ø Cannot sell for more than par value Ø Sometimes called zeroes, deep discount bonds, or original issue discount bonds (OIDs) Ø Treasury Bills and principal-only Treasury strips are good examples of zeroes. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

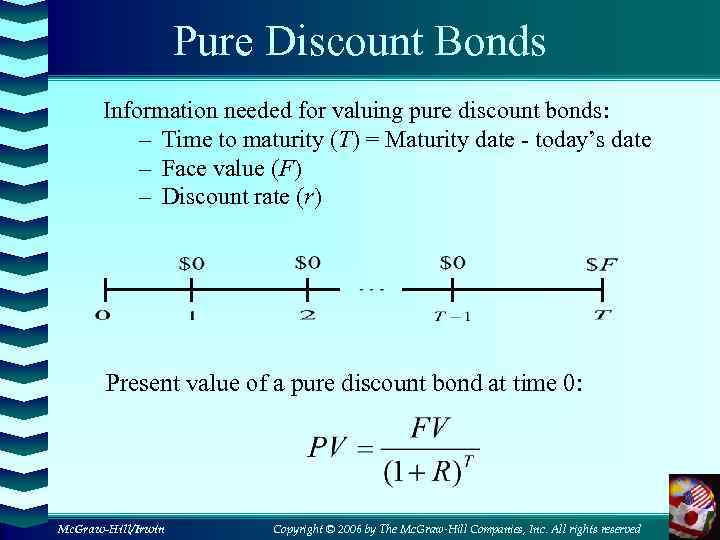
Pure Discount Bonds Information needed for valuing pure discount bonds: – Time to maturity (T) = Maturity date - today’s date – Face value (F) – Discount rate (r) Present value of a pure discount bond at time 0: Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

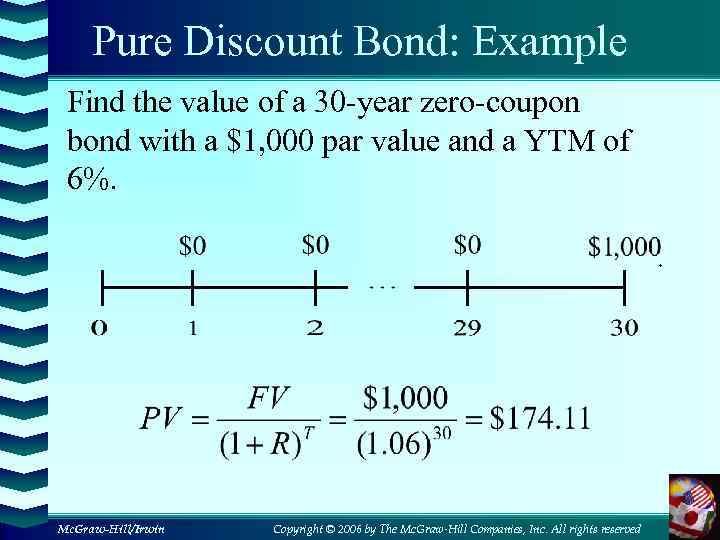
Pure Discount Bond: Example Find the value of a 30 -year zero-coupon bond with a $1, 000 par value and a YTM of 6%. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Level Coupon Bonds Ø Make periodic coupon payments in addition to the maturity value Ø The payments are equal each period. Therefore, the bond is just a combination of an annuity and a terminal (maturity) value. Ø Coupon payments are typically semiannual. Ø Effective annual rate (EAR) = (1 + R/m)m – 1 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Level Coupon Bond: Example Ø Consider a U. S. government bond with a 6 3/8% coupon that expires in December 2010. – The Par Value of the bond is $1, 000. – Coupon payments are made semi-annually (June 30 and December 31 for this particular bond). – Since the coupon rate is 6 3/8%, the payment is $31. 875. – On January 1, 2006 the size and timing of cash flows are: Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Level Coupon Bond: Example Ø On January 1, 2010, the required annual yield is

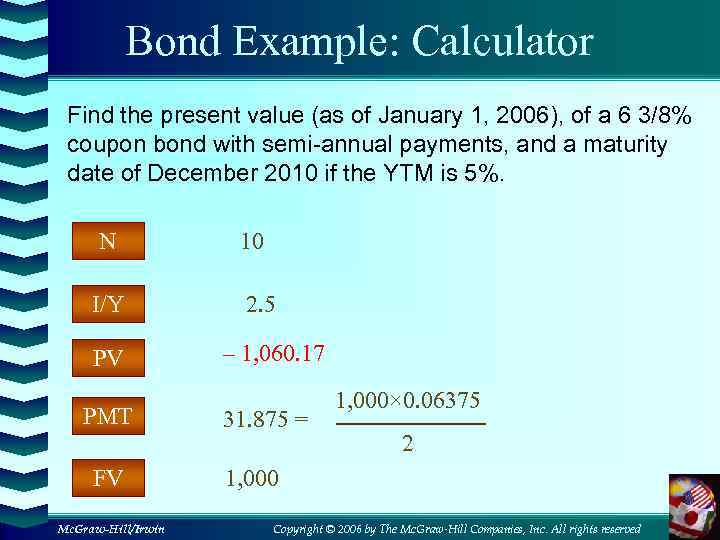
Bond Example: Calculator Find the present value (as of January 1, 2006), of a 6 3/8% coupon bond with semi-annual payments, and a maturity date of December 2010 if the YTM is 5%. N I/Y PV PMT FV Mc. Graw-Hill/Irwin 10 2. 5 – 1, 060. 17 31. 875 = 1, 000× 0. 06375 2 1, 000 Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Bond Pricing with a Spreadsheet Ø There are specific formulas for finding bond prices and yields on a spreadsheet. – PRICE(Settlement, Maturity, Rate, Yld, Redemption, Frequency, Basis) – YIELD(Settlement, Maturity, Rate, Pr, Redemption, Frequency, Basis) – Settlement and maturity need to be actual dates – The redemption and Pr need to be given as % of par value Ø Click on the Excel icon for an example. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

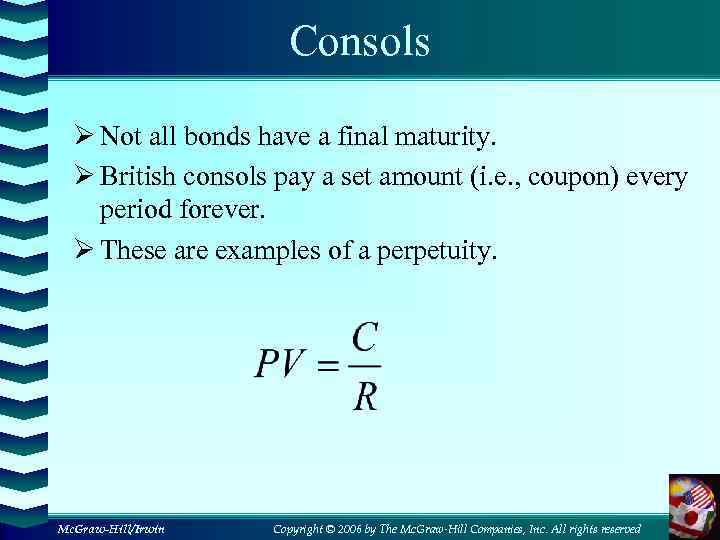
Consols Ø Not all bonds have a final maturity. Ø British consols pay a set amount (i. e. , coupon) every period forever. Ø These are examples of a perpetuity. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

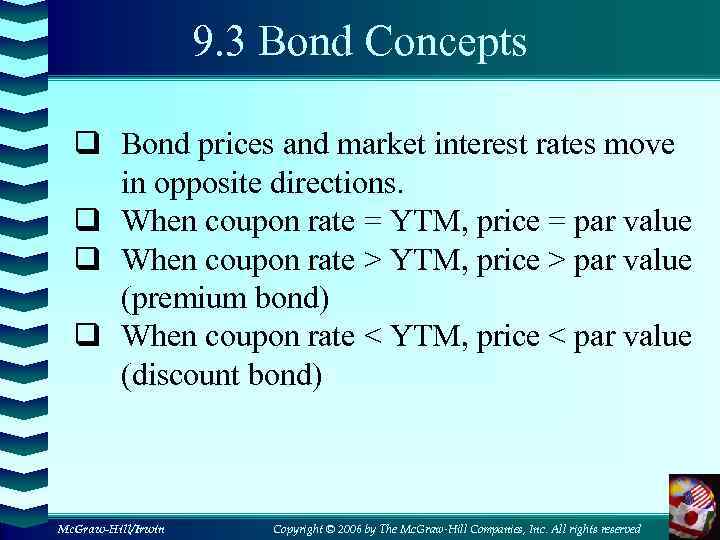
9. 3 Bond Concepts q Bond prices and market interest rates move in opposite directions. q When coupon rate = YTM, price = par value q When coupon rate > YTM, price > par value (premium bond) q When coupon rate < YTM, price < par value (discount bond) Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

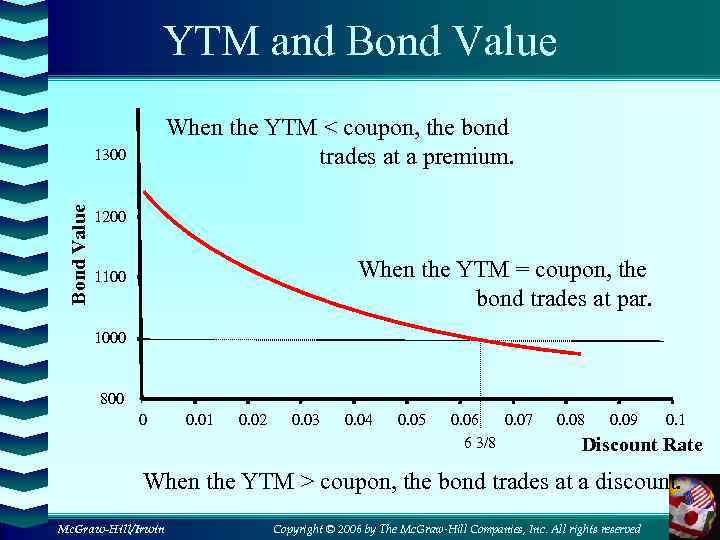
YTM and Bond Value When the YTM < coupon, the bond trades at a premium. Bond Value 1300 1200 When the YTM = coupon, the bond trades at par. 1100 1000 800 0 0. 01 0. 02 0. 03 0. 04 0. 05 0. 06 0. 07 6 3/8 0. 09 0. 1 Discount Rate When the YTM > coupon, the bond trades at a discount. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

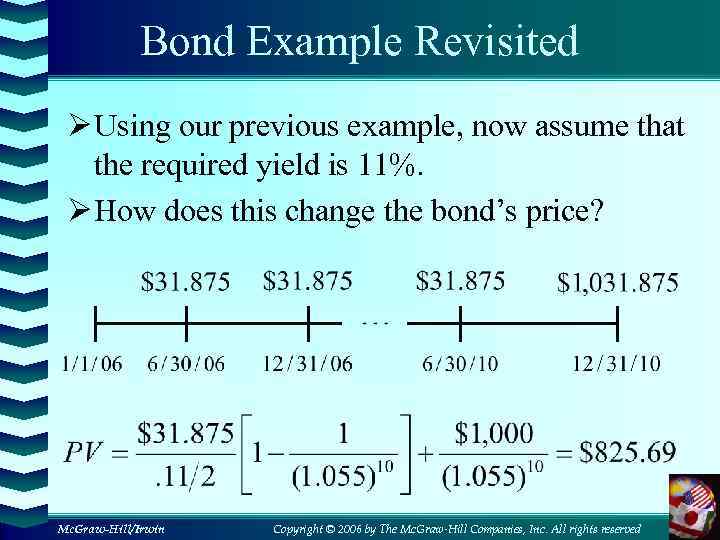
Bond Example Revisited Ø Using our previous example, now assume that the required yield is 11%. Ø How does this change the bond’s price? Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Computing Yield to Maturity Ø Yield to maturity is the rate implied by the current bond price. Ø Finding the YTM requires trial and error if you do not have a financial calculator and is similar to the process for finding R with an annuity. Ø If you have a financial calculator, enter N, PV, PMT, and FV, remembering the sign convention (PMT and FV need to have the same sign, PV the opposite sign). Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

YTM with Annual Coupons Ø Consider a bond with a 10% annual coupon rate, 15 years to maturity, and a par value of $1, 000. The current price is $928. 09. – Will the yield be more or less than 10%? – N = 15; PV = -928. 09; FV = 1, 000; PMT = 100 – CPT I/Y = 11% Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

YTM with Semiannual Coupons Ø Suppose a bond with a 10% coupon rate and semiannual coupons has a face value of $1, 000, 20 years to maturity, and is selling for $1, 197. 93. – Is the YTM more or less than 10%? – What is the semiannual coupon payment? – How many periods are there? – N = 40; PV = -1, 197. 93; PMT = 50; FV = 1, 000; CPT I/Y = 4% (Is this the YTM? ) – YTM = 4%*2 = 8% Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Bond Market Reporting Ø Primarily over-the-counter transactions with dealers connected electronically Ø Extremely large number of bond issues, but generally low daily volume in single issues Ø Makes getting up-to-date prices difficult, particularly on a small company or municipal issues Ø Treasury securities are an exception Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Treasury Quotations 8 Nov 21 132: 23 132: 24 -12 5. 14 Ø What is the coupon rate on the bond? Ø When does the bond mature? Ø What is the bid price? What does this mean? Ø What is the ask price? What does this mean? Ø How much did the price change from the previous day? Ø What is the yield based on the ask price? Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Range of values for equity Maximum value • Earnings/cash flows under new ownership • Dividends under existing ownership • Assets basis Minimum value Slide 61 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Asset valuation bases Possible bases of valuation Historic Realisable (unlikey to be realistic) Slide 62 Replacement (asset used on ongoing basis) (asset sold/ business broken up) Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Assets basis If a business is difficult to sell, its owners may be prepared to accept a minimum bid that matched the value that they get from a liquidation. There are 2 ways of assessing this: (a) Balance sheet value - but the book value of assets will differ from their market value (b) Realisable value - better, but harder to calculate. Slide 63 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

9. 4 The Present Value of Common Stocks Ø The value of any asset is the present value of its expected future cash flows. Ø Stock ownership produces cash flows from: – Dividends – Capital Gains Ø Valuation of Different Types of Stocks – Zero Growth – Constant Growth – Differential Growth Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Dividend basis Max Value the dividends under the existing management Min Slide 65 Mc.

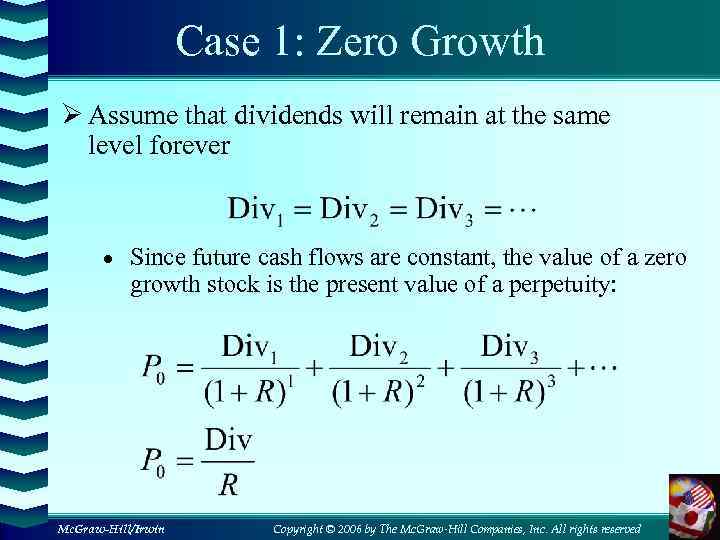
Case 1: Zero Growth Ø Assume that dividends will remain at the same level forever · Since future cash flows are constant, the value of a zero growth stock is the present value of a perpetuity: Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

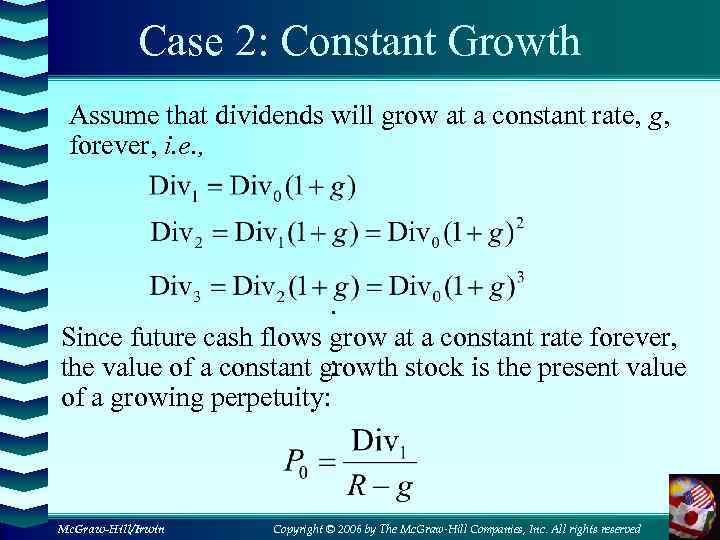
Case 2: Constant Growth Assume that dividends will grow at a constant rate, g, forever, i. e. , . Since future cash flows grow at a constant rate forever, . . the value of a constant growth stock is the present value of a growing perpetuity: Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Constant Growth Example Ø Suppose Big D, Inc. , just paid a dividend of $. 50. It is expected to increase its dividend by 2% per year. If the market requires a return of 15% on assets of this risk level, how much should the stock be selling for? Ø P 0 =. 50(1+. 02) / (. 15 -. 02) = $3. 92 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Case 3: Differential Growth Ø Assume that dividends will grow at different rates in the foreseeable future and then will grow at a constant rate thereafter. Ø To value a Differential Growth Stock, we need to: – Estimate future dividends in the foreseeable future. – Estimate the future stock price when the stock becomes a Constant Growth Stock (case 2). – Compute the total present value of the estimated future dividends and future stock price at the appropriate discount rate. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

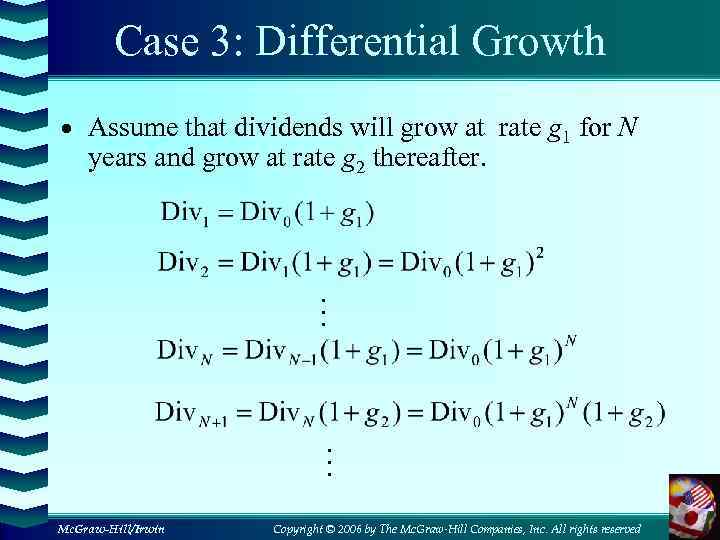
Case 3: Differential Growth · Assume that dividends will grow at rate g 1 for N years and grow at rate g 2 thereafter. . . . Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

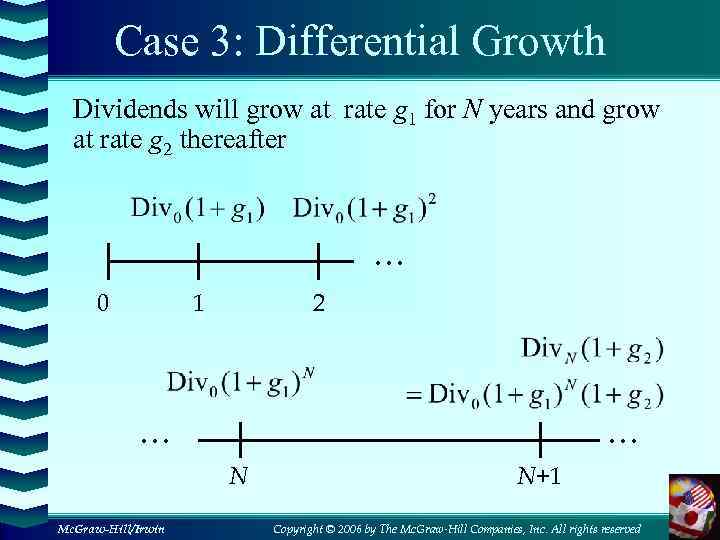
Case 3: Differential Growth Dividends will grow at rate g 1 for N years and grow at rate g 2 thereafter … 0 1 2 … … N Mc. Graw-Hill/Irwin N+1 Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Case 3: Differential Growth We can value this as the sum of: § an N-year annuity growing at rate g 1 § plus the discounted value of a perpetuity growing at rate g 2 that starts in year N+1 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

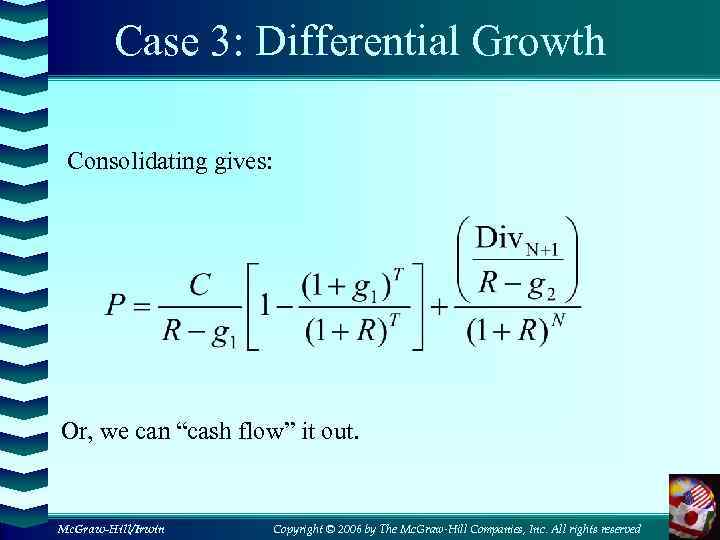
Case 3: Differential Growth Consolidating gives: Or, we can “cash flow” it out. Mc.

A Differential Growth Example A common stock just paid a dividend of $2. The dividend is expected to grow at 8% for 3 years, then it will grow at 4% in perpetuity. What is the stock worth? The discount rate is 12%. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

With the Formula Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc.

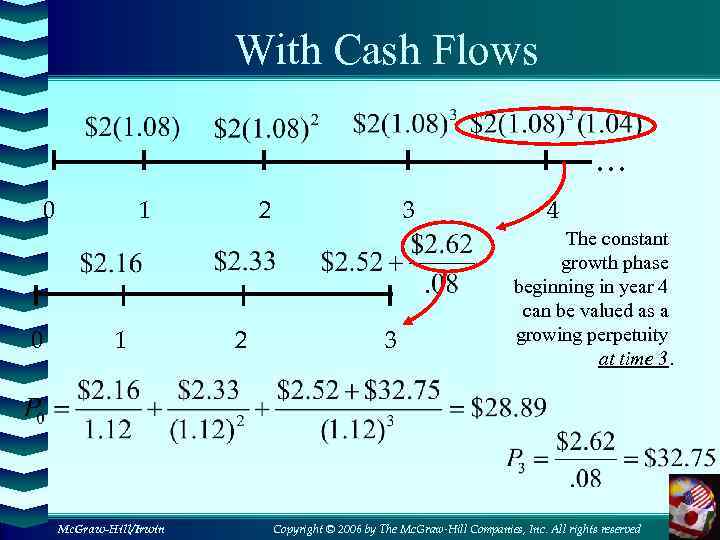
With Cash Flows … 0 0 1 1 Mc. Graw-Hill/Irwin 2 2 3 3 4 The constant growth phase beginning in year 4 can be valued as a growing perpetuity at time 3. Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Disadvantages (a) It is difficult to estimating future dividend growth (b) It is inaccurate to assume that growth will be constant (c) It creates zero values for zero dividend companies. (d) It creates negative values for high growth companies, if g > Ke Slide 77 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Ø The Red Bud Co. just paid a dividend of $1. 20 a share. The company announced today that it will continue to pay this constant dividend for the next 3 years after which time it will discontinue paying dividends permanently. What is one share of this stock worth today if the required rate of return is 7%? Ø a. $2. 94 Ø b. $3. 15 Ø c. $3. 23 Ø d. $3. 44 Ø e. $3. 60 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

9. 5 Estimates of Parameters Ø The value of a firm depends upon its growth rate, g, and its discount rate, R. – Where does g come from? g = Retention ratio × Return on retained earnings Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Where does R come from? Ø The discount rate can be broken into two parts. – The dividend yield – The growth rate (in dividends) Ø In practice, there is a great deal of estimation error involved in estimating R. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

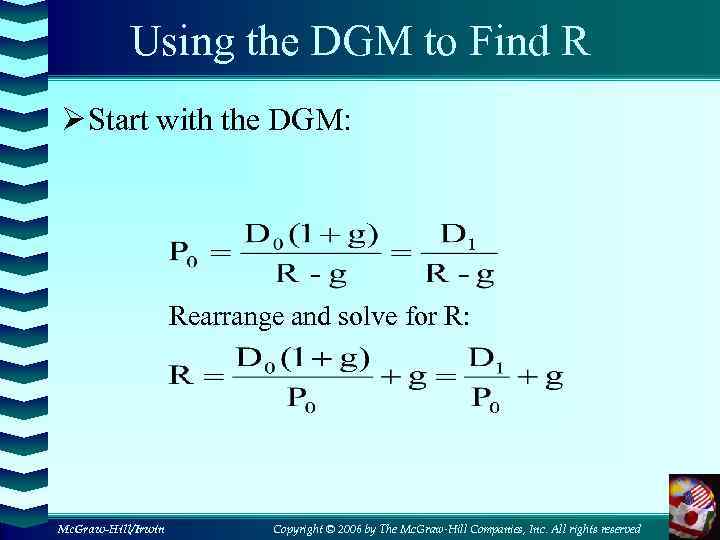
Using the DGM to Find R Ø Start with the DGM: Rearrange and solve

Ø what should be paid for Overland common stock? Overland has just paid a dividend of $2. 25. These dividends are expected to grow at a rate of 5% in the foreseeable future. The required rate of return is 11%. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

9. 6 Growth Opportunities Ø Growth opportunities are opportunities to invest in positive NPV projects. Ø The value of a firm can be conceptualized as the sum of the value of a firm that pays out 100% of its earnings as dividends and the net present value of the growth opportunities. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

9. 7 The Dividend Growth Model and the NPVGO Model Ø We have two ways to value a stock: – The dividend discount model – The sum of its price as a “cash cow” plus the per share value of its growth opportunities Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

The NPVGO Model: Example Consider a firm that has EPS of $5 at the end of the first year, a dividend-payout ratio of 30%, a discount rate of 16%, and a return on retained earnings of 20%. Ø The dividend at year one will be $5 ×. 30 = $1. 50 per share. Ø The retention ratio is. 70 ( = 1 -. 30), implying a growth rate in dividends of 14% =. 70 × 20%. From the dividend growth model, the price of a share is: Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

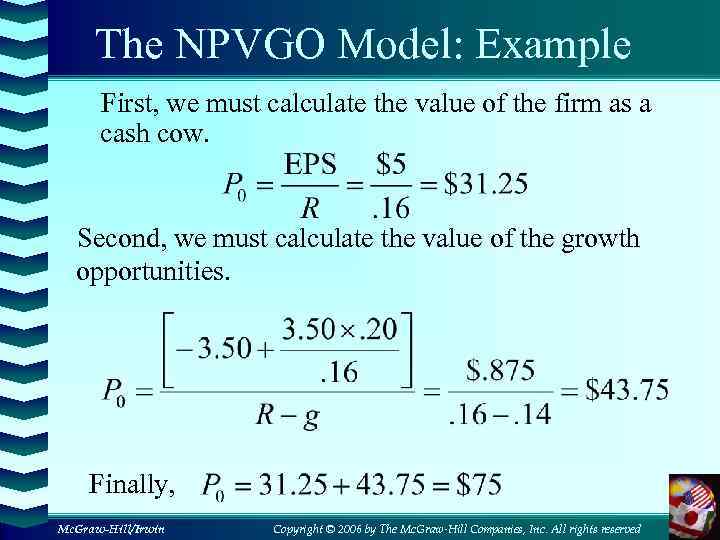
The NPVGO Model: Example First, we must calculate the value of the firm as a cash cow. Second, we must calculate the value of the growth opportunities. Finally, Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

9. 8 Price-Earnings Ratio Ø Many analysts frequently relate earnings per share to price. Ø The price-earnings ratio is calculated as the current stock price divided by annual EPS. – The Wall Street Journal uses last 4 quarter’s earnings Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

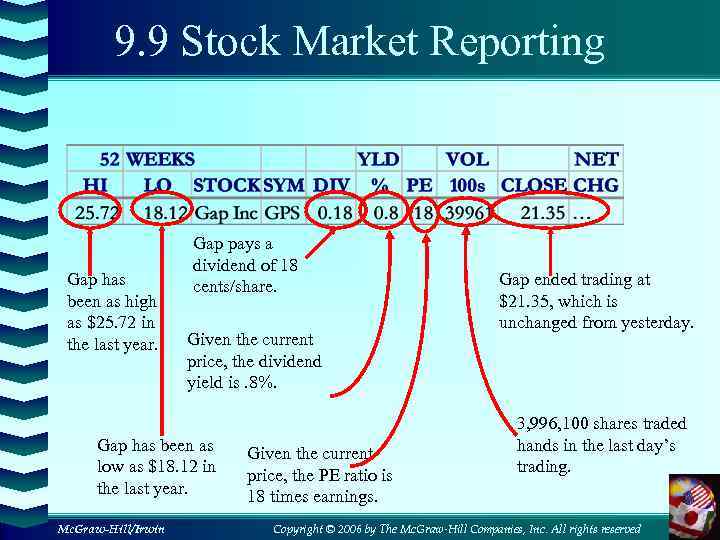
9. 9 Stock Market Reporting Gap has been as high as $25. 72 in the last year. Gap pays a dividend of 18 cents/share. Given the current price, the dividend yield is. 8%. Gap has been as low as $18. 12 in the last year. Mc. Graw-Hill/Irwin Given the current price, the PE ratio is 18 times earnings. Gap ended trading at $21. 35, which is unchanged from yesterday. 3, 996, 100 shares traded hands in the last day’s trading. Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Earnings basis Market value = P/E Growth prospects Slide 89 Mc. Graw-Hill/Irwin x earnings

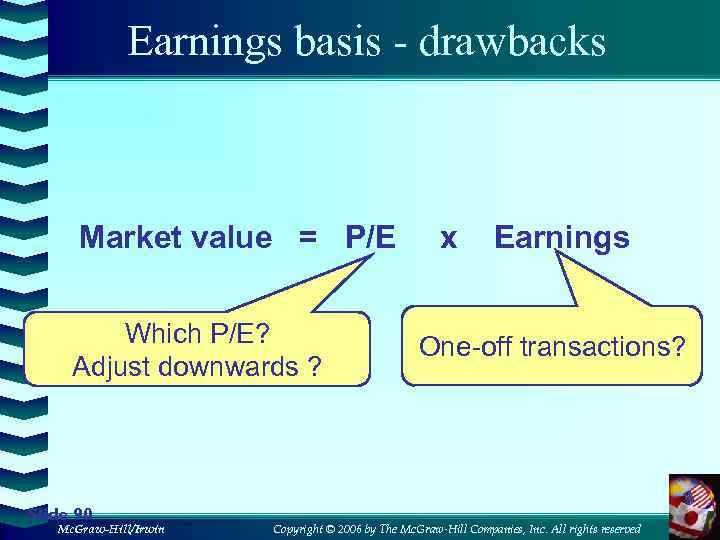
Earnings basis - drawbacks Market value = P/E Which P/E? Adjust downwards ? Slide 90 Mc. Graw-Hill/Irwin x Earnings One-off transactions? Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Earnings yield = EPS Market price per share Market value = Earnings yield Slide

Valuation of other securities Ø Discounted cash flow techniques can be used to value irredeemable debt, convertible debt and preference shares. Slide 92 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Quick Quiz Ø How do you find the value of a bond, and why do bond prices change? Ø What is a bond indenture, and what are some of the important features? Ø What determines the price of a share of stock? Ø What determines g and R in the DGM? Ø Decompose a stock’s price into constant growth and NPVGO values. Ø Discuss the importance of the PE ratio. Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

Lecture 9 Prospective Analysis – Valuation theory and concepts (part 2) Chapter 7 – Palepu, Healy & Peek IFRS Edition Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

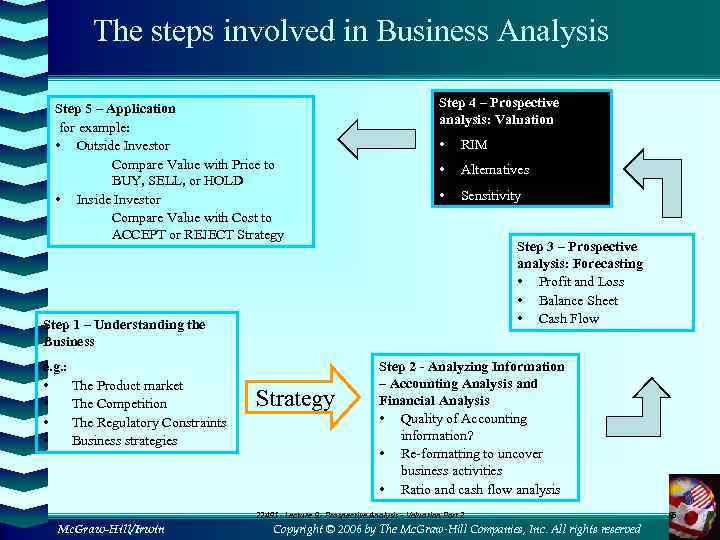
The steps involved in Business Analysis Step 5 – Application for example: • Outside Investor Compare Value with Price to BUY, SELL, or HOLD • Inside Investor Compare Value with Cost to ACCEPT or REJECT Strategy Step 4 – Prospective analysis: Valuation • RIM • Alternatives • Sensitivity Step 3 – Prospective analysis: Forecasting • Profit and Loss • Balance Sheet • Cash Flow Step 1 – Understanding the Business e. g. : • The Product market • The Competition • The Regulatory Constraints • Business strategies Strategy Step 2 - Analyzing Information – Accounting Analysis and Financial Analysis • Quality of Accounting information? • Re-formatting to uncover business activities • Ratio and cash flow analysis 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 95

Learning Objectives At the conclusion of this lecture you should understand: How to value a firm using the following methods: Ø Residual income (Abnormal Earnings) Ø Residual operating income Ø Discounted cash flow 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 96

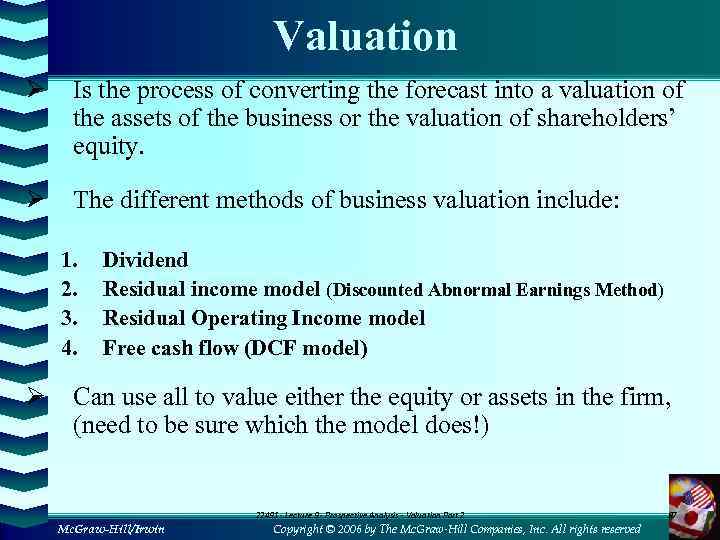
Valuation Ø Is the process of converting the forecast into a valuation of the assets of the business or the valuation of shareholders’ equity. Ø The different methods of business valuation include: 1. 2. 3. 4. Ø Dividend Residual income model (Discounted Abnormal Earnings Method) Residual Operating Income model Free cash flow (DCF model) Can use all to value either the equity or assets in the firm, (need to be sure which the model does!) 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 97

Earnings ØAccountants and analysts focus on earnings ØEarnings in the income statement represent the flow of value “created” between two points in time: NI 1 ØDistinguishable from dividends which are (net) flows paid back to the owners between two points in time: DIV 1 and dividends (and Book Value) – Relies on CSP (Clean Surplus Profit): There is a relation between earnings BVE 1 = BVE 0 + CSP 1 - DIV 1 Or: n DIV 1 = CSP 1 + BVE 0 - BVE 1 CSP 1 = BVE 1 + DIV 1 - BVE 0 = Book Value of Equity at START of Year BVE 1 = Book Value of Equity at END of Year CSP 1 = Clean Surplus Profit DIV 1 = Dividend Paid during year 1 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 98

Residual Income Model Where Ø Normal Earnings Capitalised re is the cost of equity capital n Abnormal Earnings (Residual Income) Capitalised 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 99

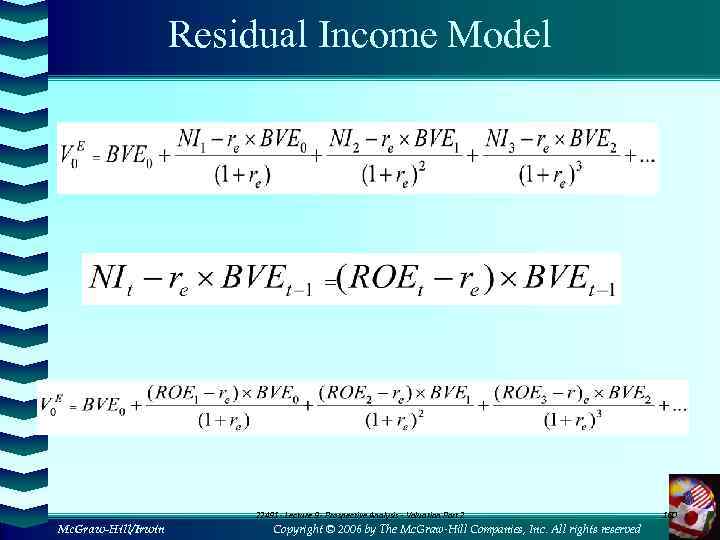
Residual Income Model 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2

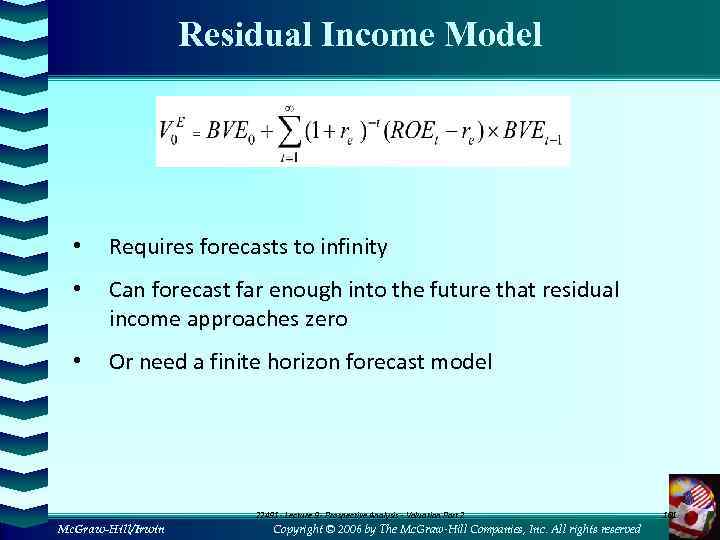
Residual Income Model • Requires forecasts to infinity • Can forecast far enough into the future that residual income approaches zero • Or need a finite horizon forecast model 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 101

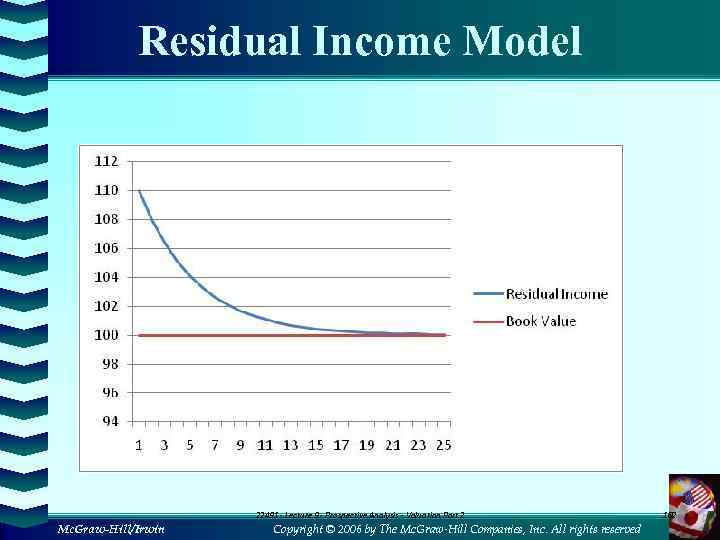
Residual Income Model – Terminal Values Ø Choice of 3 simple ways to calculate a terminal value at some time in the future – our forecast horizon Ø 3 choices: 1. Residual Income = 0 2. Residual Income in perpetuity 3. Residual Income continues in perpetuity, with growth Ø Choice dependent on what we know of the firm, and therefore our forecasts 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 103

Residual Income Model– steps in valuation 1. Forecast CSP (Clean Surplus Profit) and book values of equity 2. Estimate cost of capital for equity 3. Calculate Residual Income = CSP – opening equity (for that year) x cost of capital for equity 4. Calculate forecast Residual Income growth patterns to estimate Terminal Value (TV) calculation method (0, perpetuity or perpetuity with growth) 5. Calculate TV at time T (where growth pattern stabilises) 6. Discount forecast Residual Income to TV year and the TV, add together + opening book value for total value 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 104

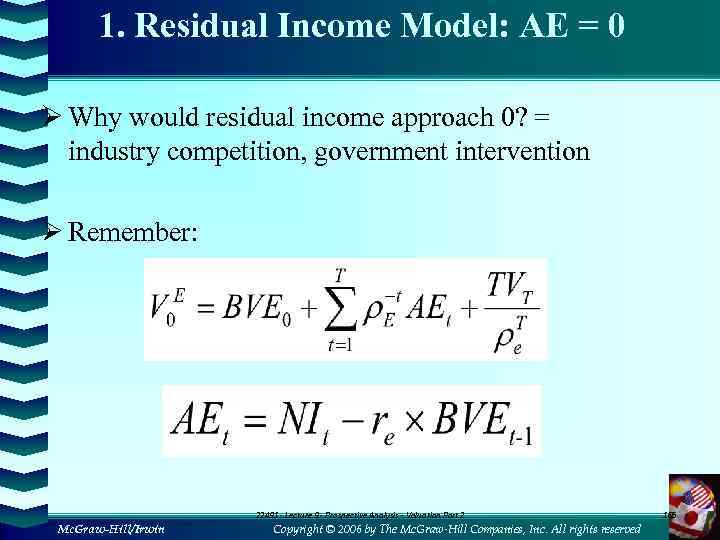
1. Residual Income Model: AE = 0 Ø Why would residual income approach 0? = industry competition, government intervention Ø Remember: 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 105

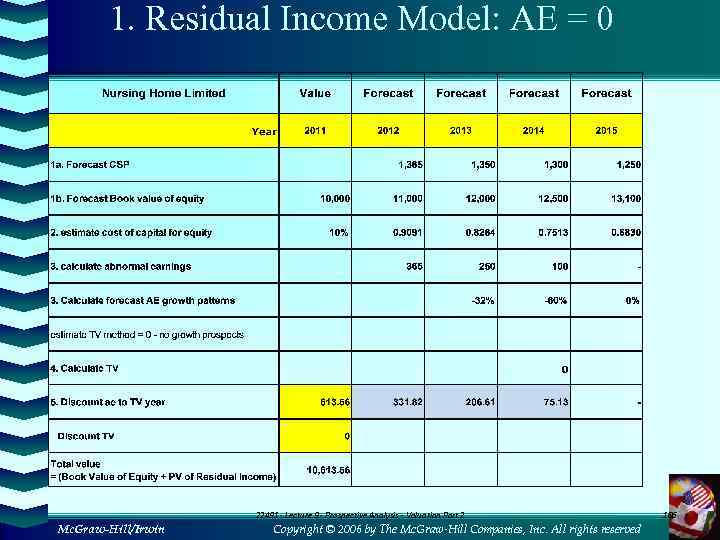
1. Residual Income Model: AE = 0 22491 - Lecture 9 - Prospective Analysis

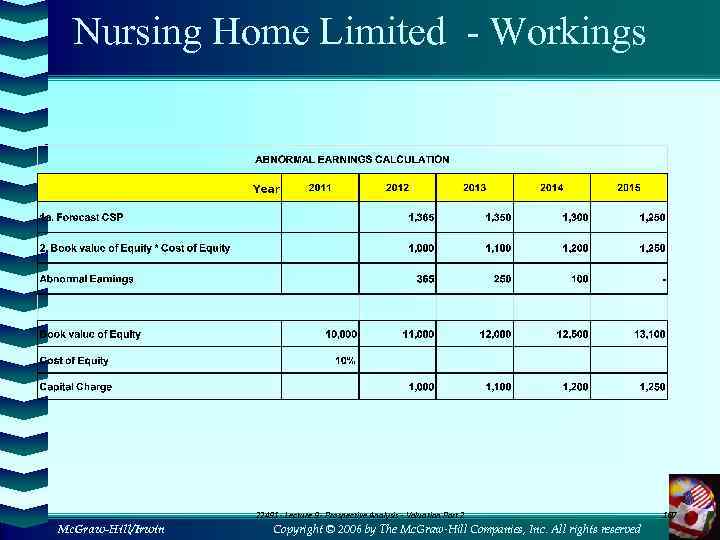
Nursing Home Limited - Workings 22491 - Lecture 9 - Prospective Analysis - Valuation

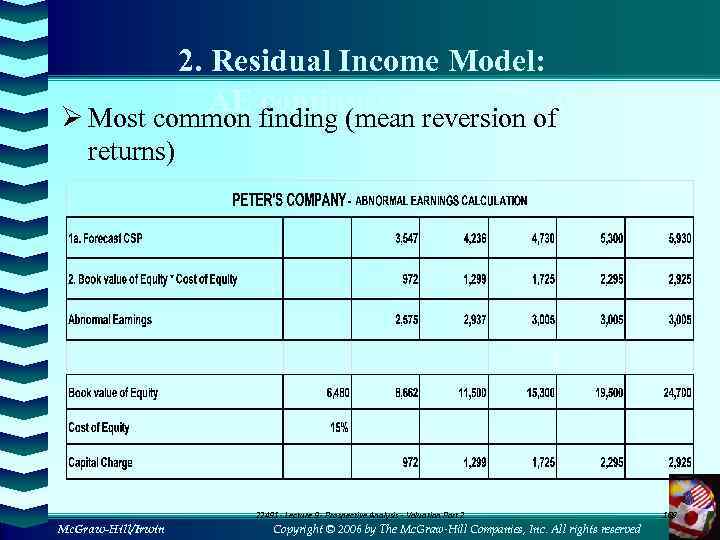
2. Residual Income Model: AE continues in perpetuity Ø Most common finding (mean reversion of returns) 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 108

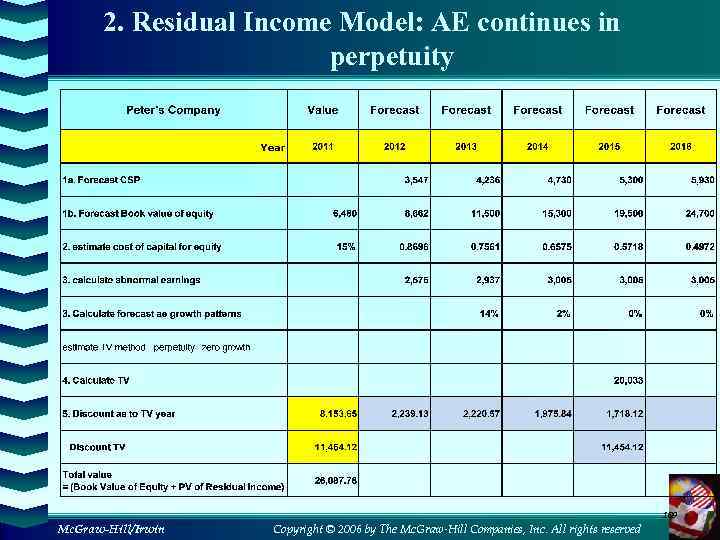
2. Residual Income Model: AE continues in perpetuity 109 Mc. Graw-Hill/Irwin Copyright © 2006

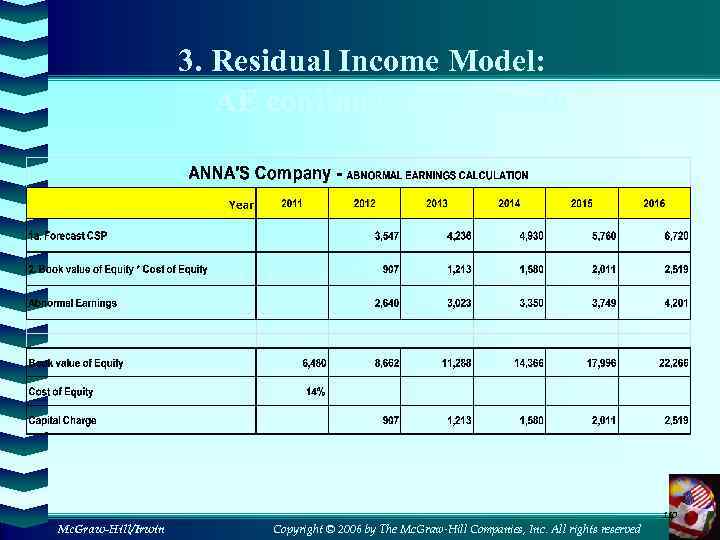
3. Residual Income Model: AE continues with growth 110 Mc. Graw-Hill/Irwin Copyright © 2006

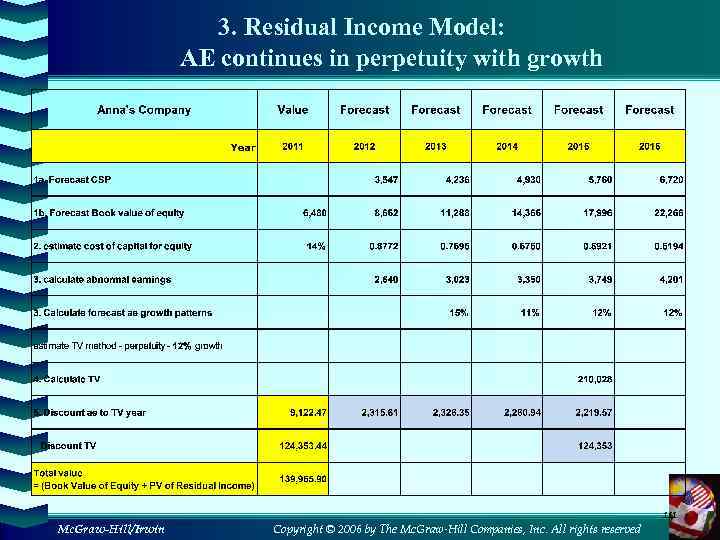
3. Residual Income Model: AE continues in perpetuity with growth 111 Mc. Graw-Hill/Irwin Copyright

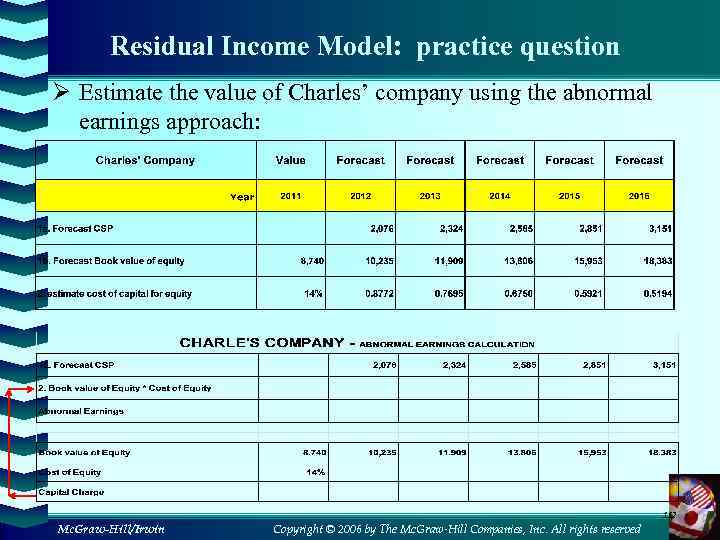
Residual Income Model: practice question Ø Estimate the value of Charles’ company using the abnormal earnings approach: 112 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved

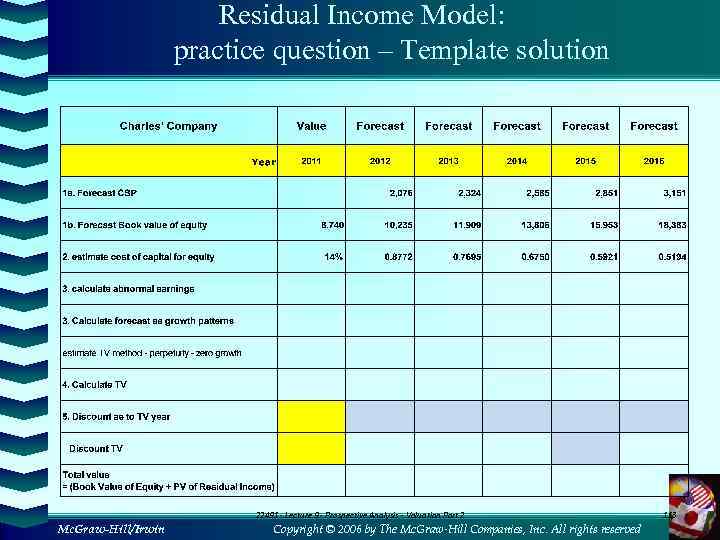
Residual Income Model: practice question – Template solution 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 113

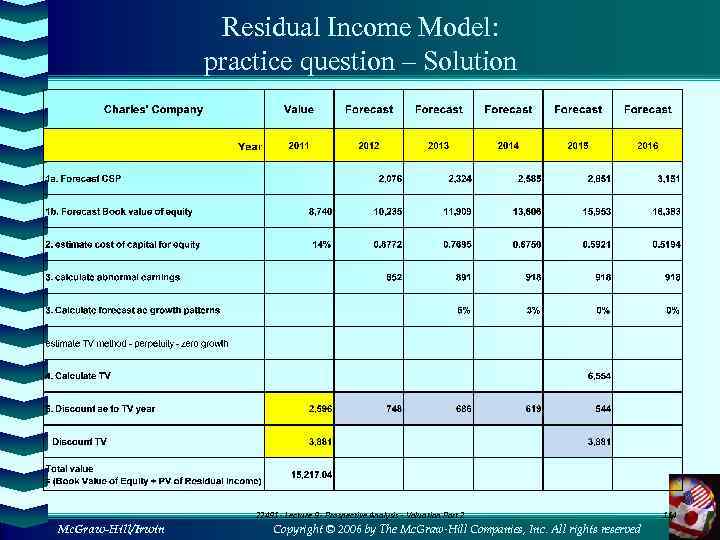
Residual Income Model: practice question – Solution 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 114

Residual Income Model Advantages: Disadvantages: Ø Academic research shows that this method out performs many of the other models Ø Focus on value drivers § Profitability of investment and growth in investment § Directs strategic thinking Ø Incorporates the financial statements § Incorporates the balance sheet (book value) § Forecasts the income statement and the balance sheet Ø Uses accrual accounting § Recognizes value added § Matches value added to value lost § Treats investment as an asset Ø Versatility § Can be used with a wide variety of accounting principles Ø Aligned with what people forecast Ø Can be validated Ø Accounting Complexity § Requires understanding of how accounting works Ø Suspect accounting § Accounting numbers can be suspect Ø Forecast Horizon § Forecast horizon depends on the quality of the accounting 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 115

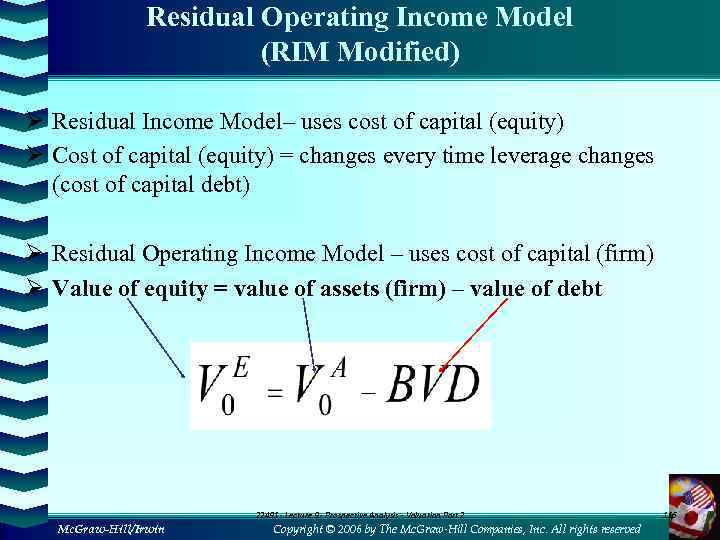
Residual Operating Income Model (RIM Modified) Ø Residual Income Model– uses cost of capital (equity) Ø Cost of capital (equity) = changes every time leverage changes (cost of capital debt) Ø Residual Operating Income Model – uses cost of capital (firm) Ø Value of equity = value of assets (firm) – value of debt 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 116

Residual Operating Income Model Ø Therefore - can also estimate equity values by calculating the value of assets and subtracting the book value of debt (often simpler – no need to think about leverage) Value of Equity = Value of Assets – Book Value of Debt Ø Question: So how to value the assets (firm)? Ø Equity = NOA – Net debt (NFL) Ø CSP = NOPAT – Net financing expenses NOA = Net Operating Assets CSP = Clean Surplus Profit Net Debt = Net Financial Liabilities = Interest Bearing Debt (Current + Non Current) – (Cash +Cash Equivalents) NOPAT = Net Operating Profit after tax Net Financing Expense = Interest Expense – Interest Revenue 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 117

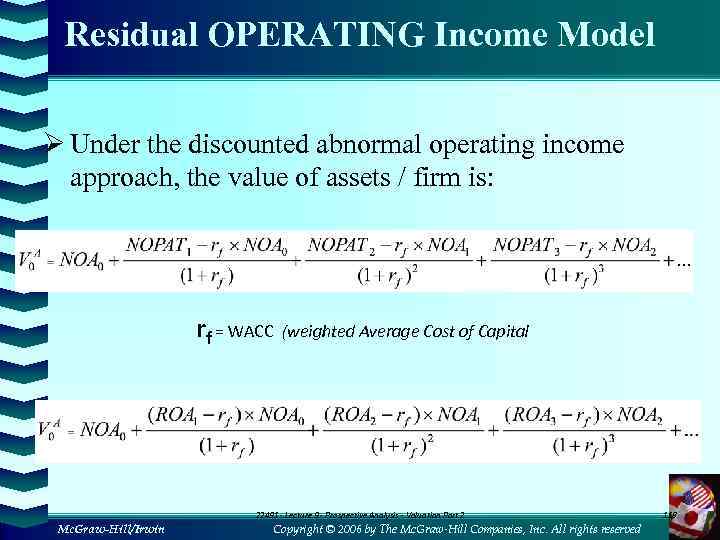
Residual OPERATING Income Model Ø Under the discounted abnormal operating income approach, the value of assets / firm is: rf = WACC (weighted Average Cost of Capital 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 118

Residual Operating Income Model Ø Gives the same result as abnormal earnings valuation (as long as cost of capital for equity is adjusted each time leverage changes) 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 119

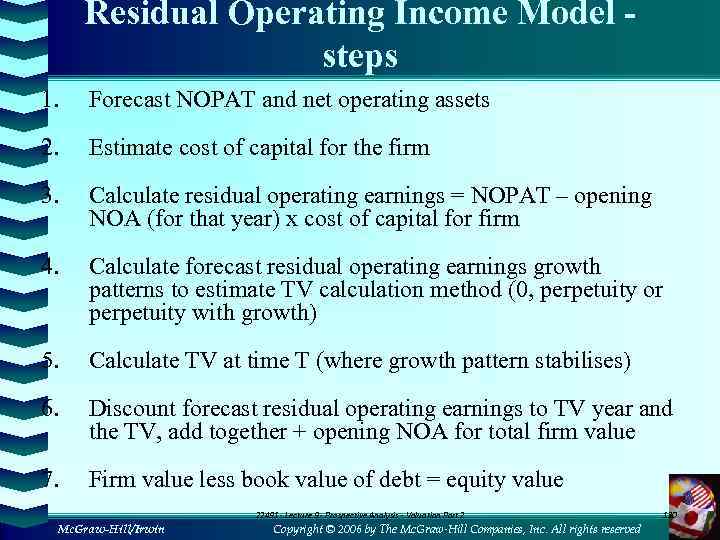
Residual Operating Income Model - steps 1. Forecast NOPAT and net operating assets 2. Estimate cost of capital for the firm 3. Calculate residual operating earnings = NOPAT – opening NOA (for that year) x cost of capital for firm 4. Calculate forecast residual operating earnings growth patterns to estimate TV calculation method (0, perpetuity or perpetuity with growth) 5. Calculate TV at time T (where growth pattern stabilises) 6. Discount forecast residual operating earnings to TV year and the TV, add together + opening NOA for total firm value 7. Firm value less book value of debt = equity value 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 120

Residual Operating Income Model - question 1. Estimate the value of the assets and the value of the equity of following firm: 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 121

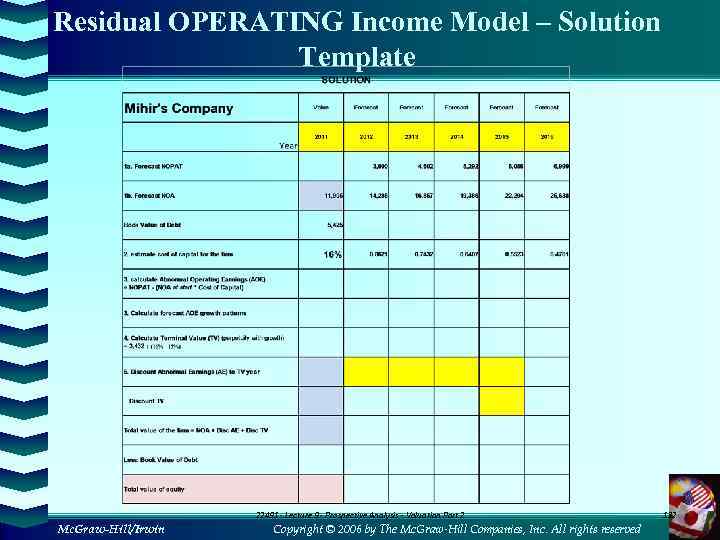
Residual OPERATING Income Model – Solution Template 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 122

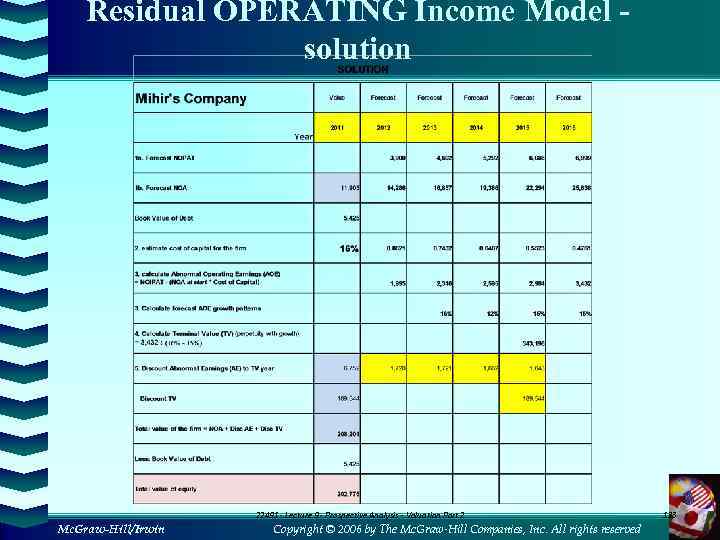
Residual OPERATING Income Model - solution 22491 - Lecture 9 - Prospective Analysis -

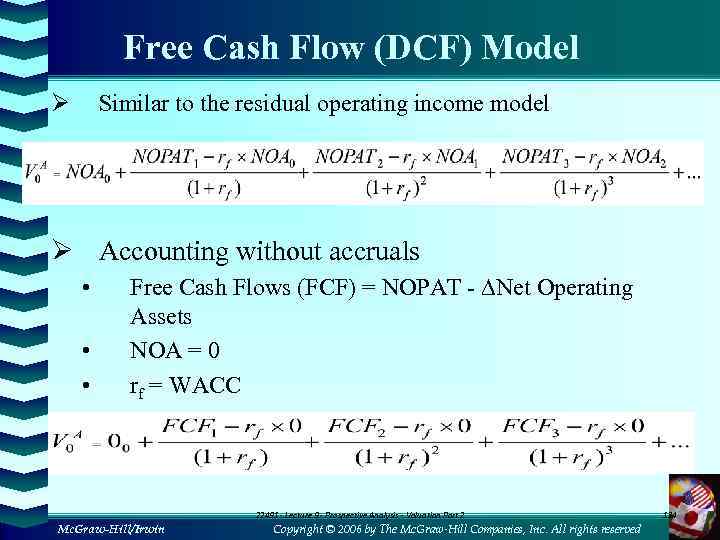
Free Cash Flow (DCF) Model Ø Similar to the residual operating income model Ø Accounting without accruals • • • Free Cash Flows (FCF) = NOPAT - ∆Net Operating Assets NOA = 0 rf = WACC 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 124

The Continuing Value for the DCF Ø Also forecasts over an infinite horizon: need to make a terminal assumption: 1. No FCF past horizon 2. Capitalise terminal free cash flow as a perpetuity, or 3. Capitalise terminal free cash flow as a perpetuity with growth 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 125

DCF valuation method - steps 1. Forecast free cash flow 2. Estimate cost of capital for the firm 3. Calculate forecast FCF growth patterns to estimate Terminal Value (TV) calculation method (0, perpetuity or perpetuity with growth) 4. Calculate TV at time T (where growth pattern stabilises) 5. Discount forecast FCF to TV year and the TV, add together for total firm value 6. Firm value less Book Value of DEBT = Equity Value 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 126

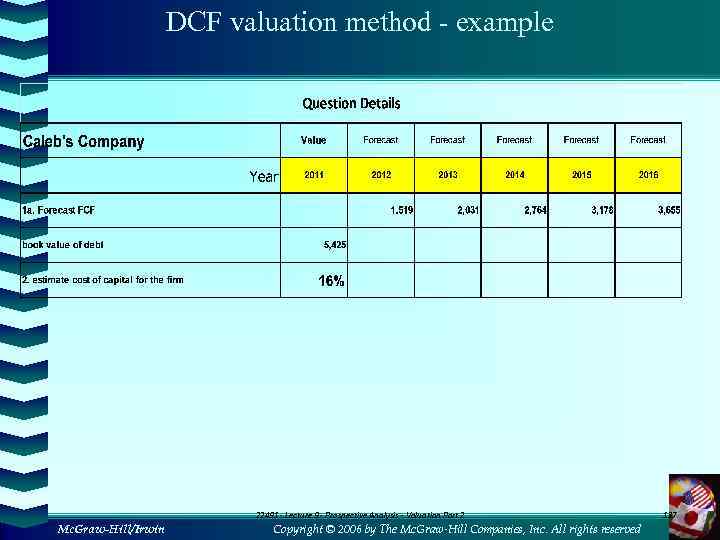
DCF valuation method - example 22491 - Lecture 9 - Prospective Analysis - Valuation

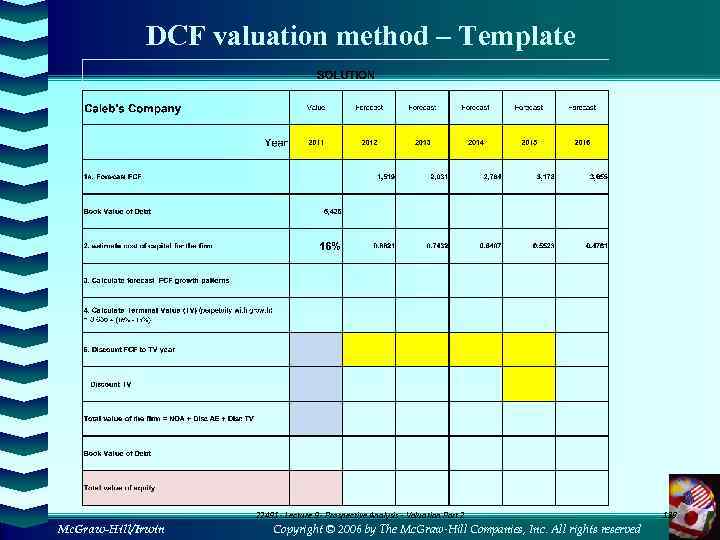
DCF valuation method – Template 22491 - Lecture 9 - Prospective Analysis - Valuation

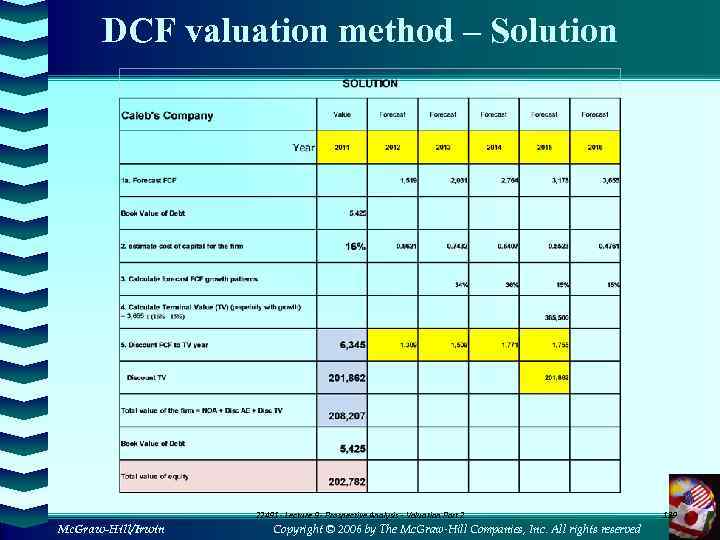
DCF valuation method – Solution 22491 - Lecture 9 - Prospective Analysis - Valuation

What are the problems with DCF? Ø Cash flow from operations (value added) is reduced by investments (which also add value): investments are treated as value losses Ø Value received is not matched against value surrendered to generate value - except for long forecast horizons Note: analysts forecast earnings, not cash flows 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 130

Advantages Easy concept: Familiarity: DCF Analysis cash flows are “real” and easy to think about; they are not affected by accounting rules is a straight application of familiar net present value techniques Suspect concept: Disadvantages → free cash flow does not measure value added in the short run; value gained is not matched with value given up → free cash flow fails to recognize value generated that does not involve cash flows → investment is treated as a loss of value → free cash flow is partly a liquidation concept; firms increase free cash flow by cutting back on investments Forecast horizons: can require long forecast horizons to recognize cash inflows from investments, particularly when investments are growing Validation: it is hard to validate free cash flow forecasts Not aligned with what people forecast: When It Works Best analysts forecast earnings, not free cash flow; adjusting earnings forecasts to free cash forecasts requires further forecasting of accruals 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 131 Copyright © to produce Mc. Graw-Hill Companies, free cash flow growing Mc. Graw-Hill/Irwin the investment pattern is such as 2006 by Theconstant free cash flow or. Inc. All rights reserved at a When

Comparing the models – DDM, DCF and RIM Ø All derive from the dividend discount model Ø Differences: 1. Focus on different issues 2. Require different levels of structure 3. Have different implications for terminal values 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 132

Comparing the models – DDM, DCF and RIM(DAE) – research results Penman and Suogiannis (1998) CAR Dechow Hutton and Sloan (1999) JAE Francis, Olsson and Oswald (2000) JAR Ø Over relatively short forecast horizons (ten years or less) valuation estimates using DAE are more precise than DDM or DCF Ø Advantage persists over both conservative and aggressive accounting = US results that accrual accounting reflects future cash flows 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 133

Summary Ø Valuation converts forecasts of performance into approximated market price Ø There are 3 methods derived from DDM, namely Residual Income model, Residual Operating Income model and the DCF model (using Free Cash Flows) each has advantages and disadvantages so there are gains in considering using all of the approaches Ø Price multiples can also be used. These have been popular as there is no need for multi year forecasts. However, finding benchmark firms is difficult 22491 - Lecture 9 - Prospective Analysis - Valuation Part 2 Mc. Graw-Hill/Irwin Copyright © 2006 by The Mc. Graw-Hill Companies, Inc. All rights reserved 134