Chapter 8 Mechanical Failure Failure Analysis ISSUES

Chapter 8: Mechanical Failure & Failure Analysis ISSUES TO ADDRESS. . . • How do flaws in a material initiate failure? • How is fracture resistance quantified; how do different material classes compare? • How do we estimate the stress to fracture? • How do loading rate, loading history, and temperature affect the failure stress? Ship-cyclic loading from waves. Adapted from chapter-opening photograph, Chapter 8, Callister 7 e. (by Neil Boenzi, The New York Times. ) Computer chip-cyclic thermal loading. Adapted from Fig. 22. 30(b), Callister 7 e. (Fig. 22. 30(b) is courtesy of National Semiconductor Corporation. ) Hip implant-cyclic loading from walking. Adapted from Fig. 22. 26(b), Callister 7 e.

Fracture mechanisms • Ductile fracture – Occurs with plastic deformation • Brittle fracture – Occurs with Little or no plastic deformation – Thus they are Catastrophic meaning they occur without warning!

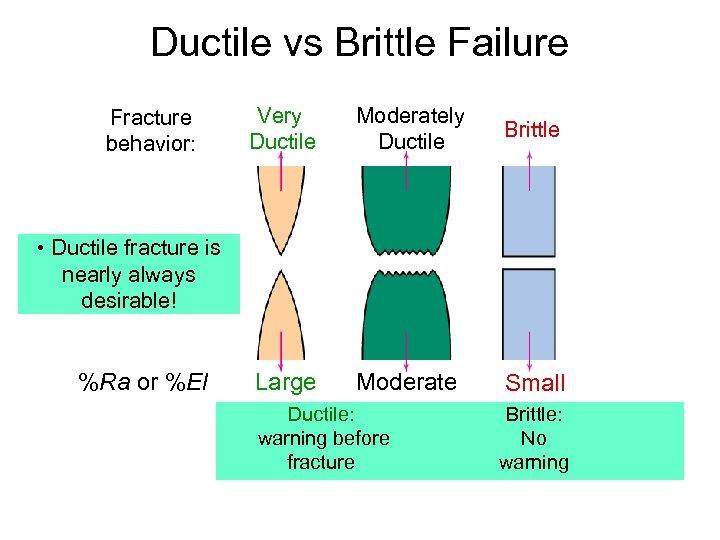Ductile vs Brittle Failure Fracture behavior: Very Ductile Moderately Ductile Brittle Large Moderate Small • Ductile fracture is nearly always desirable! %Ra or %El Ductile: warning before fracture Brittle: No warning

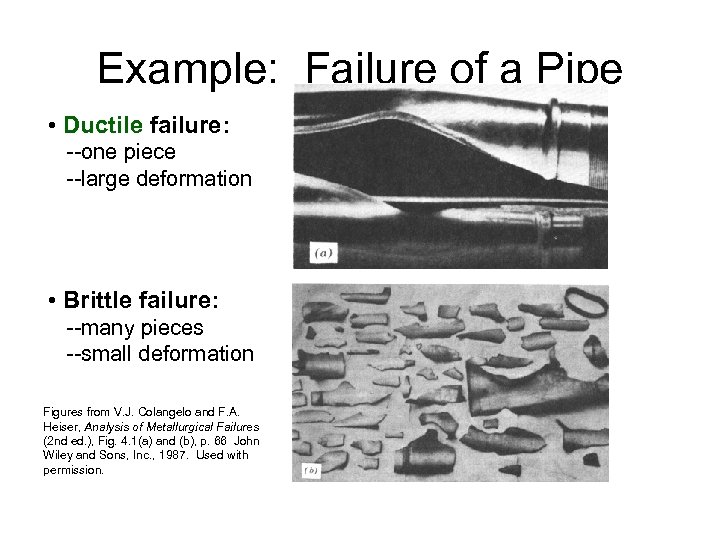Example: Failure of a Pipe • Ductile failure: --one piece --large deformation • Brittle failure: --many pieces --small deformation Figures from V. J. Colangelo and F. A. Heiser, Analysis of Metallurgical Failures (2 nd ed. ), Fig. 4. 1(a) and (b), p. 66 John Wiley and Sons, Inc. , 1987. Used with permission.

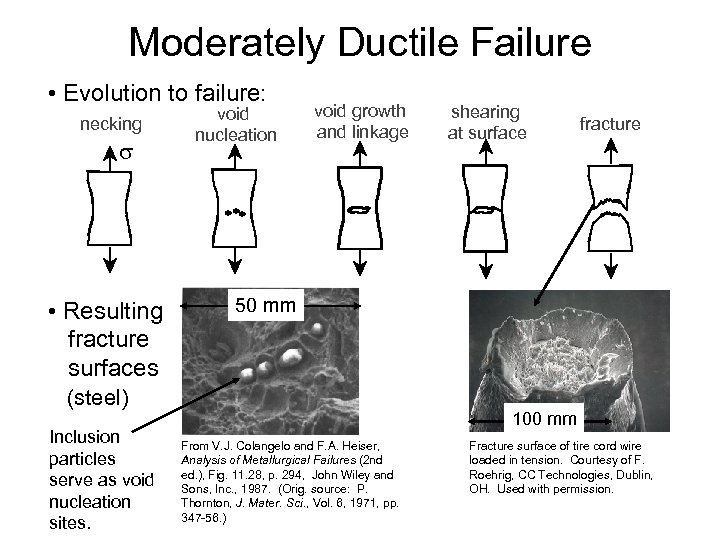Moderately Ductile Failure • Evolution to failure: necking • Resulting fracture surfaces void nucleation void growth and linkage fracture 50 mm (steel) Inclusion particles serve as void nucleation sites. shearing at surface 100 mm From V. J. Colangelo and F. A. Heiser, Analysis of Metallurgical Failures (2 nd ed. ), Fig. 11. 28, p. 294, John Wiley and Sons, Inc. , 1987. (Orig. source: P. Thornton, J. Mater. Sci. , Vol. 6, 1971, pp. 347 -56. ) Fracture surface of tire cord wire loaded in tension. Courtesy of F. Roehrig, CC Technologies, Dublin, OH. Used with permission.

Ductile vs. Brittle Failure cup-and-cone fracture Adapted from Fig. 8. 3, Callister 7 e. brittle fracture

Brittle Failure Arrows indicate point at which failure originated Adapted from Fig. 8. 5(a), Callister 7 e.

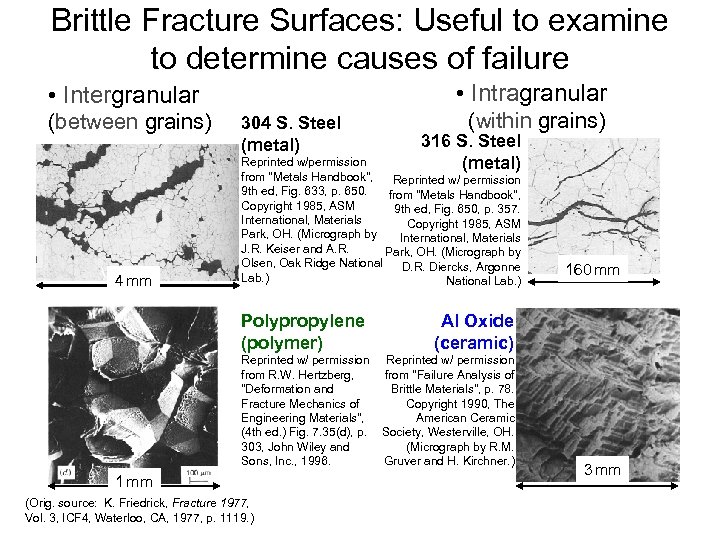Brittle Fracture Surfaces: Useful to examine to determine causes of failure • Intragranular • Intergranular (between grains) 4 mm 304 S. Steel (metal) (within grains) 316 S. Steel (metal) Reprinted w/permission from "Metals Handbook", Reprinted w/ permission 9 th ed, Fig. 633, p. 650. from "Metals Handbook", Copyright 1985, ASM 9 th ed, Fig. 650, p. 357. International, Materials Copyright 1985, ASM Park, OH. (Micrograph by International, Materials J. R. Keiser and A. R. Park, OH. (Micrograph by Olsen, Oak Ridge National D. R. Diercks, Argonne Lab. ) National Lab. ) Polypropylene (polymer) Al Oxide (ceramic) Reprinted w/ permission from R. W. Hertzberg, from "Failure Analysis of "Deformation and Brittle Materials", p. 78. Fracture Mechanics of Copyright 1990, The Engineering Materials", American Ceramic (4 th ed. ) Fig. 7. 35(d), p. Society, Westerville, OH. 303, John Wiley and (Micrograph by R. M. Sons, Inc. , 1996. Gruver and H. Kirchner. ) 1 mm (Orig. source: K. Friedrick, Fracture 1977, Vol. 3, ICF 4, Waterloo, CA, 1977, p. 1119. ) 160 mm 3 mm

Failure Analysis – Failure Avoidance • Most failure occur due to the presence of defects in materials – Cracks or Flaws (stress concentrators) – Voids or inclusions • Presence of defects is best found before hand they should be determined non-destructively – X-Ray analysis – Ultra-Sonic Inspection – Surface inspection • Magna-flux • Dye Penetrant

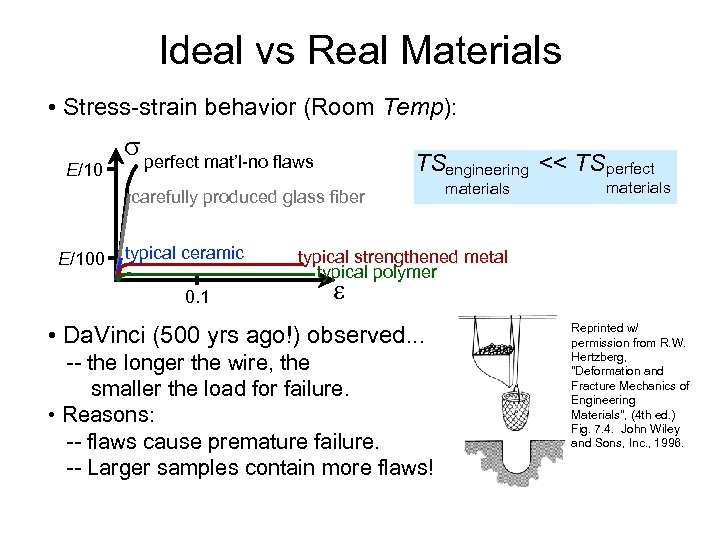Ideal vs Real Materials • Stress-strain behavior (Room Temp): E/10 perfect mat’l-no flaws TSengineering << TS perfect carefully produced glass fiber E/100 typical ceramic 0. 1 materials typical strengthened metal typical polymer e • Da. Vinci (500 yrs ago!) observed. . . -- the longer the wire, the smaller the load for failure. • Reasons: -- flaws cause premature failure. -- Larger samples contain more flaws! Reprinted w/ permission from R. W. Hertzberg, "Deformation and Fracture Mechanics of Engineering Materials", (4 th ed. ) Fig. 7. 4. John Wiley and Sons, Inc. , 1996.

Considering Loading Rate Effect • Increased loading rate. . . -- increases y and TS -- decreases %EL y TS • Why? An increased rate allows less time for dislocations to move past obstacles. e larger TS e smaller y e

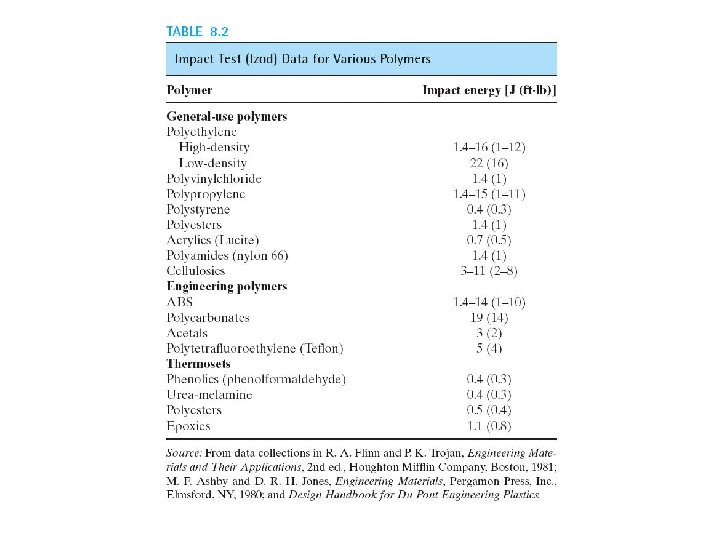
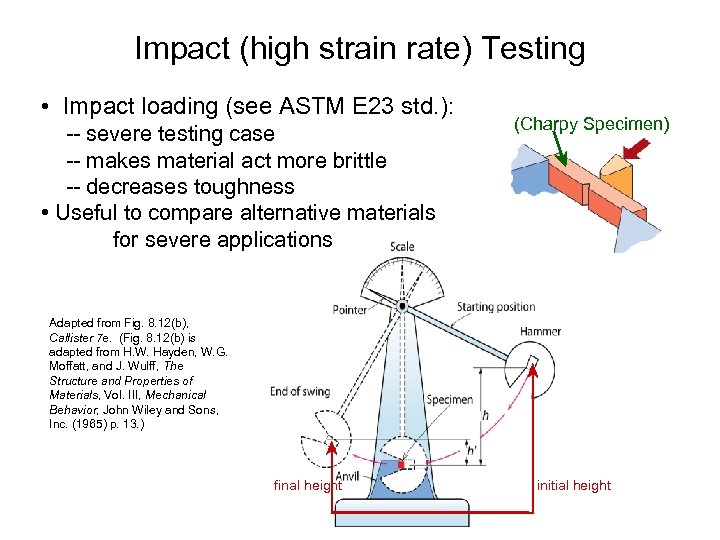Impact (high strain rate) Testing • Impact loading (see ASTM E 23 std. ): -- severe testing case -- makes material act more brittle -- decreases toughness • Useful to compare alternative materials for severe applications (Charpy Specimen) Adapted from Fig. 8. 12(b), Callister 7 e. (Fig. 8. 12(b) is adapted from H. W. Hayden, W. G. Moffatt, and J. Wulff, The Structure and Properties of Materials, Vol. III, Mechanical Behavior, John Wiley and Sons, Inc. (1965) p. 13. ) final height initial height

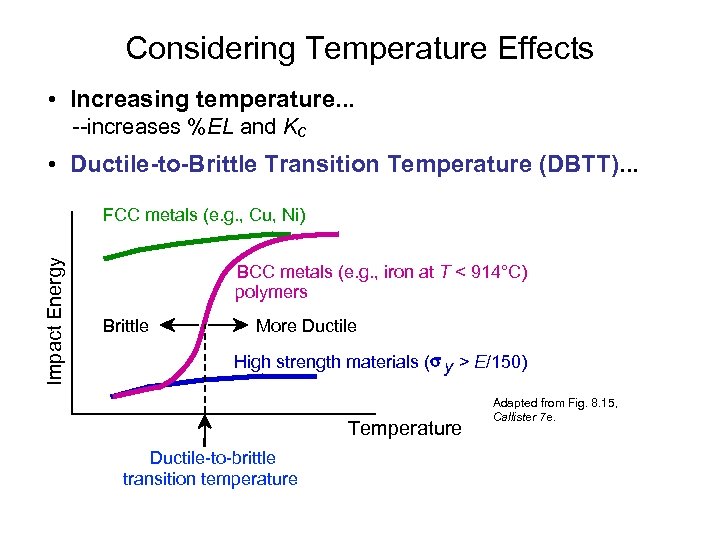Considering Temperature Effects • Increasing temperature. . . --increases %EL and Kc • Ductile-to-Brittle Transition Temperature (DBTT). . . Impact Energy FCC metals (e. g. , Cu, Ni) BCC metals (e. g. , iron at T < 914°C) polymers Brittle More Ductile High strength materials ( y > E/150) Temperature Ductile-to-brittle transition temperature Adapted from Fig. 8. 15, Callister 7 e.

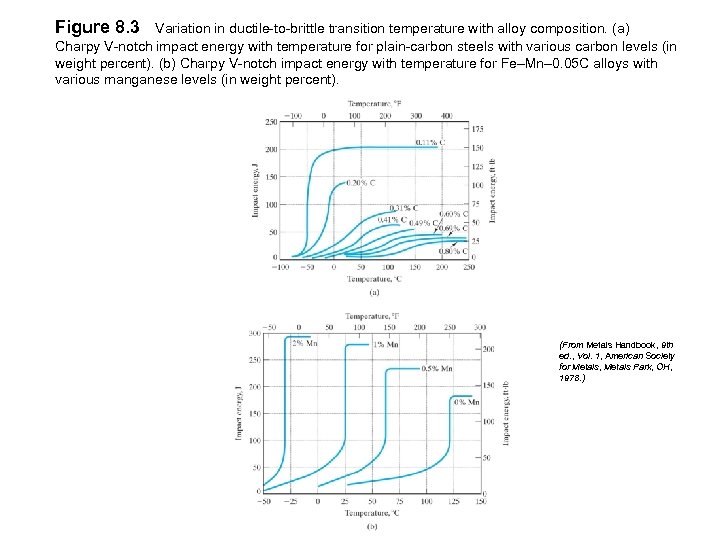Figure 8. 3 Variation in ductile-to-brittle transition temperature with alloy composition. (a) Charpy V-notch impact energy with temperature for plain-carbon steels with various carbon levels (in weight percent). (b) Charpy V-notch impact energy with temperature for Fe–Mn– 0. 05 C alloys with various manganese levels (in weight percent). (From Metals Handbook, 9 th ed. , Vol. 1, American Society for Metals, Metals Park, OH, 1978. )

Design Strategy: Build Steel Ships Quickly! • Pre-WWI: The Titanic Reprinted w/ permission from R. W. Hertzberg, "Deformation and Fracture Mechanics of Engineering Materials", (4 th ed. ) Fig. 7. 1(a), p. 262, John Wiley and Sons, Inc. , 1996. (Orig. source: Dr. Robert D. Ballard, The Discovery of the Titanic. ) • WWII: Liberty ships Reprinted w/ permission from R. W. Hertzberg, "Deformation and Fracture Mechanics of Engineering Materials", (4 th ed. ) Fig. 7. 1(b), p. 262, John Wiley and Sons, Inc. , 1996. (Orig. source: Earl R. Parker, "Behavior of Engineering Structures", Nat. Acad. Sci. , Nat. Res. Council, John Wiley and Sons, Inc. , NY, 1957. ) • Problem: Used a type of steel with a DBTT ~ Room temp. As a Designer: Stay Above The DBTT!

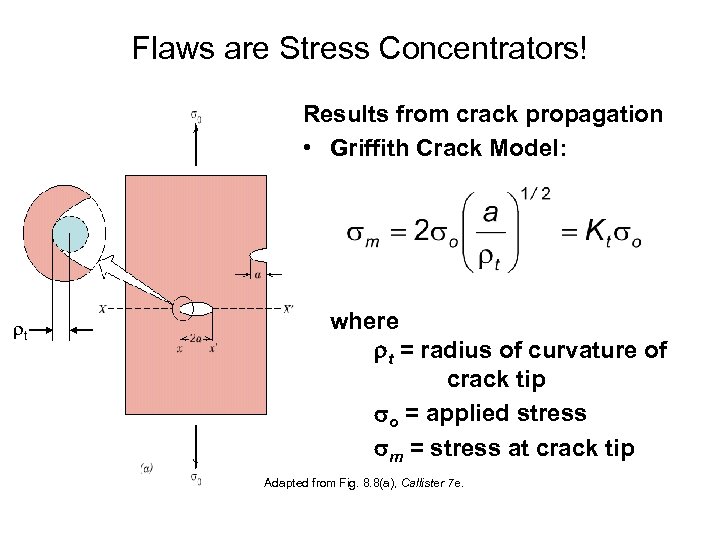Flaws are Stress Concentrators! Results from crack propagation • Griffith Crack Model: t where t = radius of curvature of crack tip o = applied stress m = stress at crack tip Adapted from Fig. 8. 8(a), Callister 7 e.

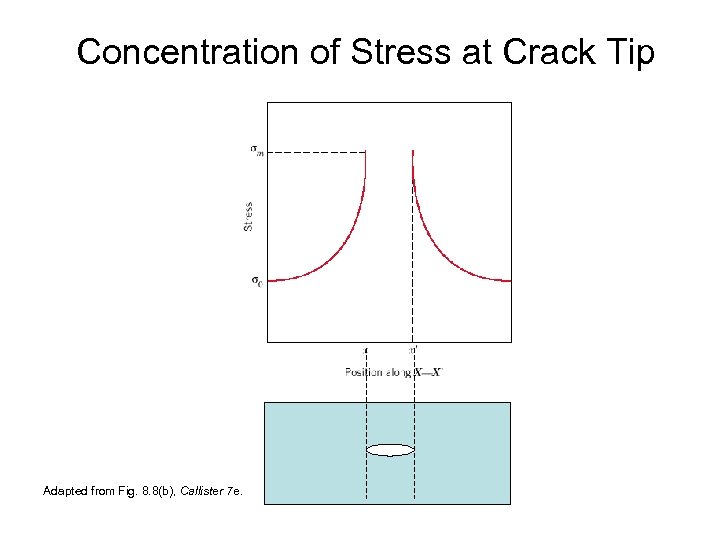Concentration of Stress at Crack Tip Adapted from Fig. 8. 8(b), Callister 7 e.

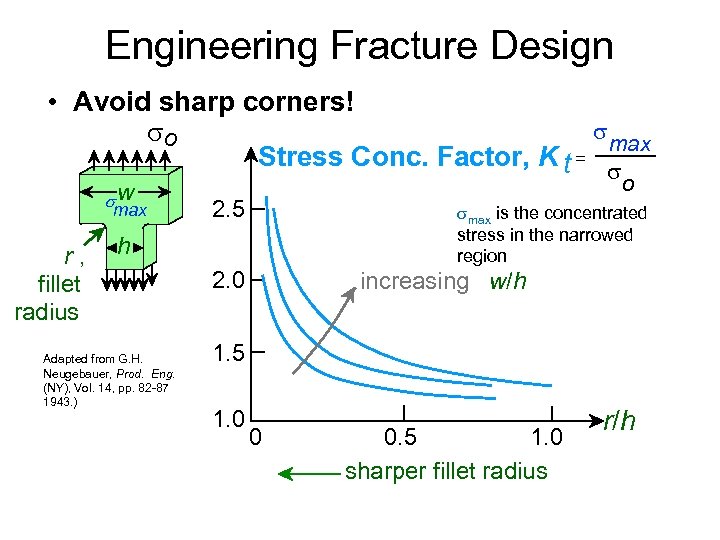Engineering Fracture Design • Avoid sharp corners! o max Stress Conc. Factor, K t = sw max r, fillet radius o 2. 5 max is the concentrated stress in the narrowed region h Adapted from G. H. Neugebauer, Prod. Eng. (NY), Vol. 14, pp. 82 -87 1943. ) 2. 0 increasing w/h 1. 5 1. 0 0 0. 5 1. 0 sharper fillet radius r/h

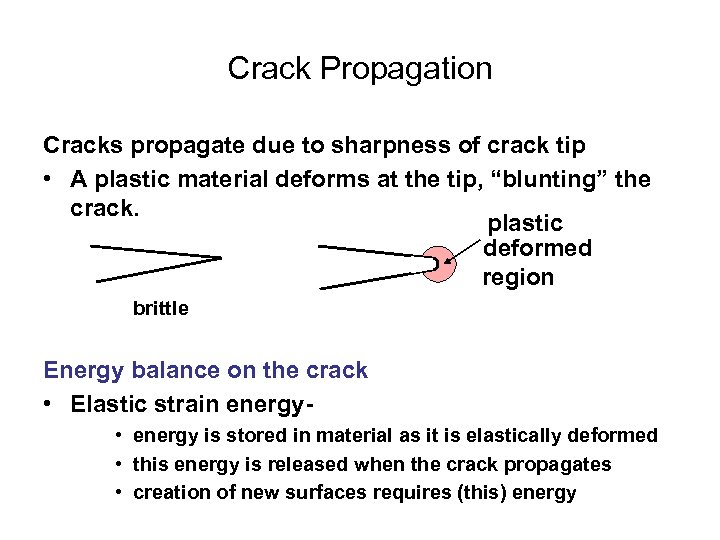Crack Propagation Cracks propagate due to sharpness of crack tip • A plastic material deforms at the tip, “blunting” the crack. plastic deformed region brittle Energy balance on the crack • Elastic strain energy • energy is stored in material as it is elastically deformed • this energy is released when the crack propagates • creation of new surfaces requires (this) energy

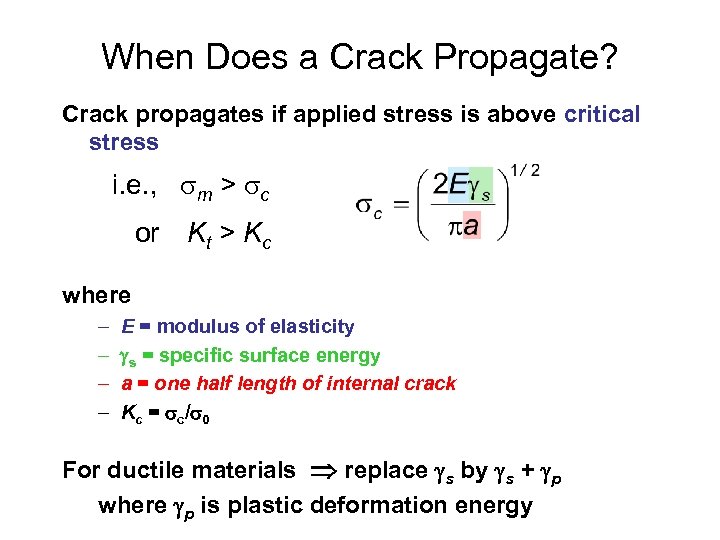When Does a Crack Propagate? Crack propagates if applied stress is above critical stress i. e. , m > c or Kt > Kc where – – E = modulus of elasticity s = specific surface energy a = one half length of internal crack Kc = c/ 0 For ductile materials replace s by s + p where p is plastic deformation energy

Fracture Toughness Metals/ Alloys 100 K Ic (MPa · m 0. 5 ) 70 60 50 40 30 Graphite/ Ceramics/ Semicond Polymers C-C(|| fibers) 1 Steels Ti alloys Al alloys Mg alloys 20 Al/Al oxide(sf) 2 Y 2 O 3 /Zr. O 2 (p) 4 C/C( fibers) 1 Al oxid/Si. C(w) 3 Si nitr/Si. C(w) 5 Al oxid/Zr. O 2 (p) 4 Glass/Si. C(w) 6 10 7 6 5 4 Diamond Si carbide Al oxide Si nitride 3 0. 7 0. 6 0. 5 PET PP PVC 2 1 Composites/ fibers PC <100> Si crystal <111> Glass -soda Concrete PS Polyester Glass 6 K 1 c – plane strain stress concentration factor – with edge crack; A Material Property we use for design, developed using ASTM Std: ASTM E 399 - 09 Standard Test Method for Linear. Elastic Plane-Strain Fracture Toughness K Ic of Metallic Materials Composite reinforcement geometry is: f = fibers; sf = short fibers; w = whiskers; p = particles. Addition data as noted (vol. fraction of reinforcement): 1. (55 vol%) ASM Handbook, Vol. 21, ASM Int. , Materials Park, OH (2001) p. 606. 2. (55 vol%) Courtesy J. Cornie, MMC, Inc. , Waltham, MA. 3. (30 vol%) P. F. Becher et al. , Fracture Mechanics of Ceramics, Vol. 7, Plenum Press (1986). pp. 61 -73. 4. Courtesy Coors. Tek, Golden, CO. 5. (30 vol%) S. T. Buljan et al. , "Development of Ceramic Matrix Composites for Application in Technology for Advanced Engines Program", ORNL/Sub/85 -22011/2, ORNL, 1992. 6. (20 vol%) F. D. Gace et al. , Ceram. Eng. Sci. Proc. , Vol. 7 (1986) pp. 978 -82.

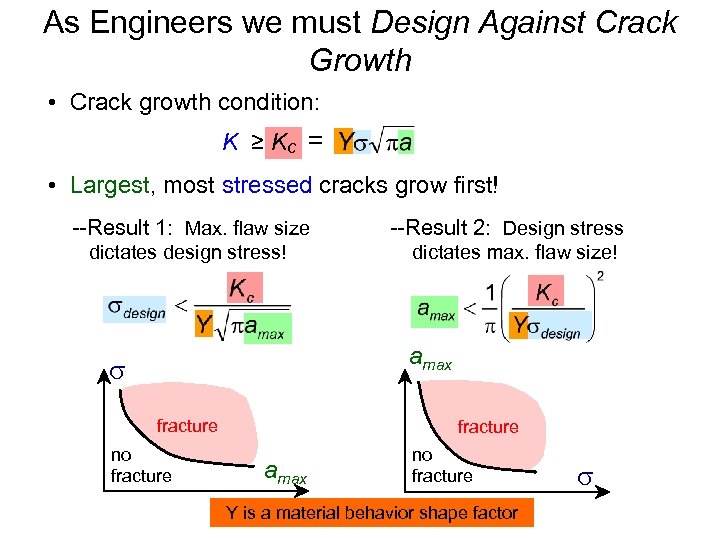As Engineers we must Design Against Crack Growth • Crack growth condition: K ≥ Kc = • Largest, most stressed cracks grow first! --Result 1: Max. flaw size dictates design stress! --Result 2: Design stress dictates max. flaw size! amax fracture no fracture amax no fracture Y is a material behavior shape factor

Design Example: Aircraft Wing • Material has Kc = 26 MPa-m 0. 5 • Two designs to consider. . . Design A --largest flaw is 9 mm --failure occurs at stress = 112 MPa Design B --use same material --largest flaw is 4 mm --failure stress = ? • Use. . . • Key point: Y and Kc are the same in both designs! --Result: 112 MPa 9 mm 4 mm Answer: • Reducing flaw size pays off!

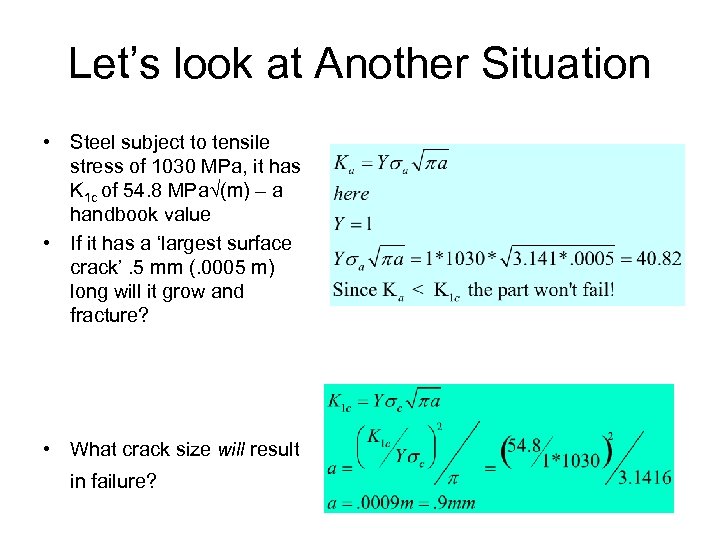Let’s look at Another Situation • Steel subject to tensile stress of 1030 MPa, it has K 1 c of 54. 8 MPa (m) – a handbook value • If it has a ‘largest surface crack’. 5 mm (. 0005 m) long will it grow and fracture? • What crack size will result in failure?

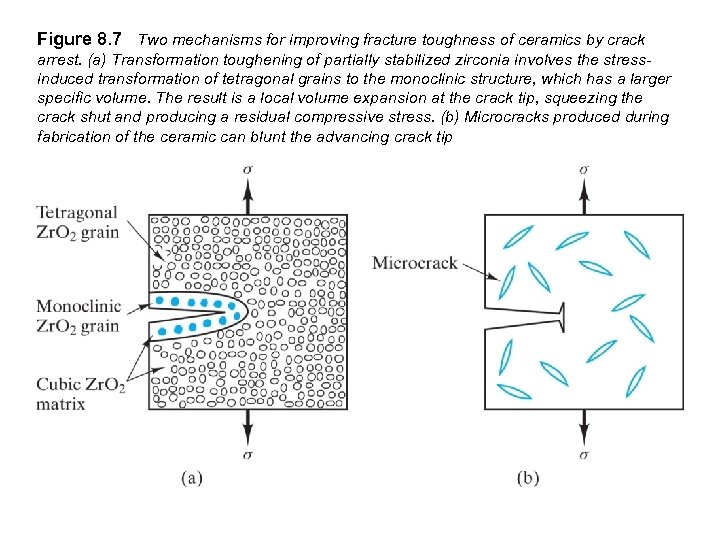Figure 8. 7 Two mechanisms for improving fracture toughness of ceramics by crack arrest. (a) Transformation toughening of partially stabilized zirconia involves the stressinduced transformation of tetragonal grains to the monoclinic structure, which has a larger specific volume. The result is a local volume expansion at the crack tip, squeezing the crack shut and producing a residual compressive stress. (b) Microcracks produced during fabrication of the ceramic can blunt the advancing crack tip

Fatigue behavior: • Fatigue = failure under cyclic stress specimen compression on top bearing motor counter flex coupling tension on bottom • Stress varies with time. -- key parameters are S (stress amplitude), m, and frequency max m min (Fig. 8. 18 is from Materials Science in Engineering, 4/E by Carl. A. Keyser, Pearson Education, Inc. , Upper Saddle River, NJ. ) S time • Key points when designing in Fatigue inducing situations: -- fatigue can cause part failure, even though max < c. -- fatigue causes ~ 90% of mechanical engineering failures. • Because of its importance, ASTM and ISO have developed many special standards to assess Fatigue Strength of materials

Some important Calculations in Fatigue Testing

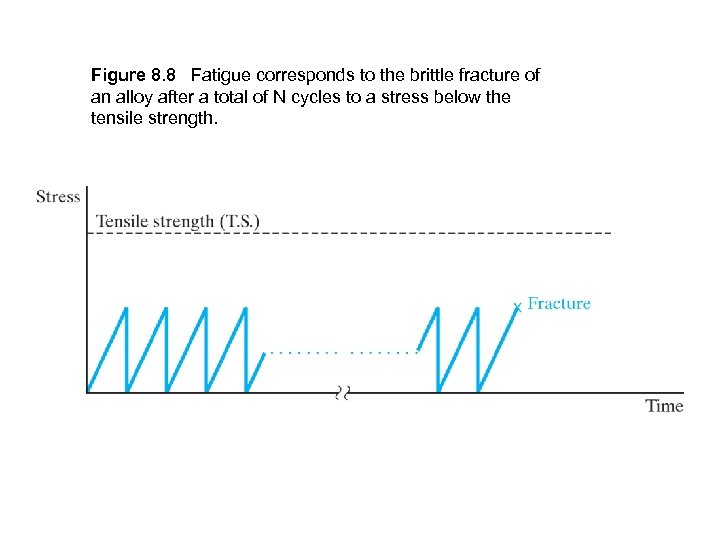Figure 8. 8 Fatigue corresponds to the brittle fracture of an alloy after a total of N cycles to a stress below the tensile strength.

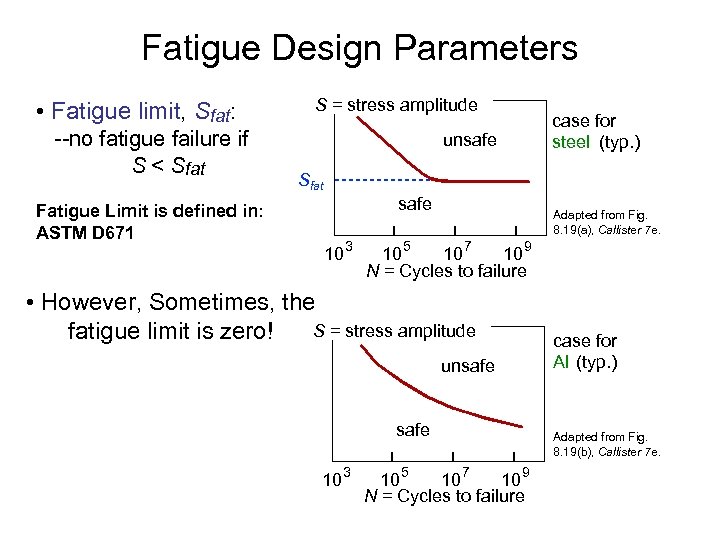Fatigue Design Parameters • Fatigue limit, Sfat: --no fatigue failure if S < Sfat Fatigue Limit is defined in: ASTM D 671 S = stress amplitude unsafe case for steel (typ. ) Sfat safe 10 3 Adapted from Fig. 8. 19(a), Callister 7 e. 10 5 10 7 10 9 N = Cycles to failure • However, Sometimes, the S = stress amplitude fatigue limit is zero! unsafe 10 3 10 5 10 7 10 9 N = Cycles to failure case for Al (typ. ) Adapted from Fig. 8. 19(b), Callister 7 e.

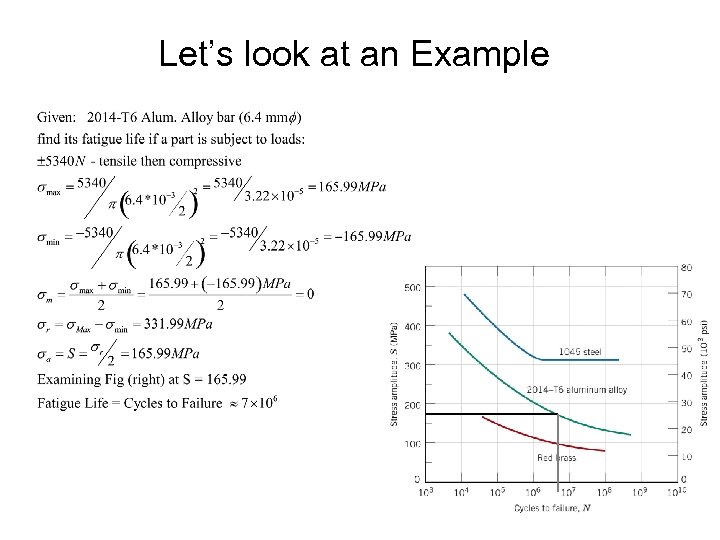Let’s look at an Example

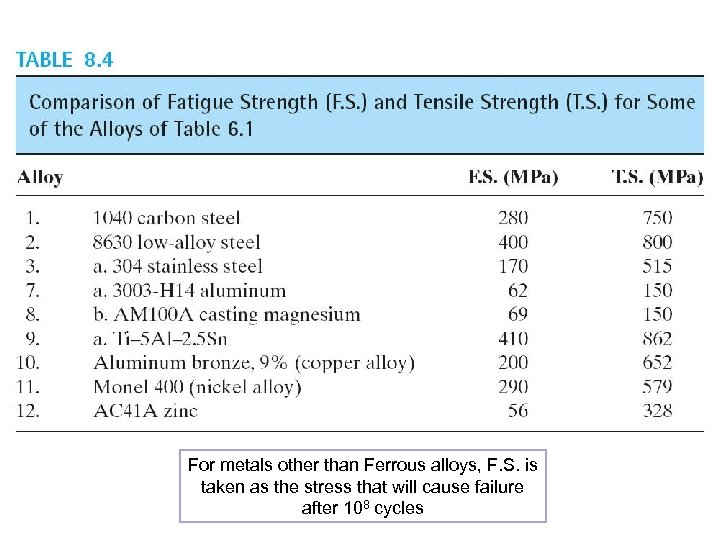For metals other than Ferrous alloys, F. S. is taken as the stress that will cause failure after 108 cycles

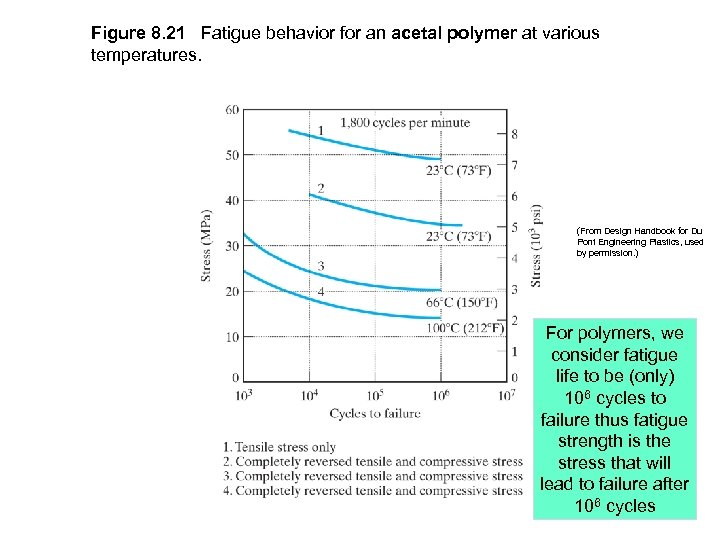Figure 8. 21 Fatigue behavior for an acetal polymer at various temperatures. (From Design Handbook for Du Pont Engineering Plastics, used by permission. ) For polymers, we consider fatigue life to be (only) 106 cycles to failure thus fatigue strength is the stress that will lead to failure after 106 cycles

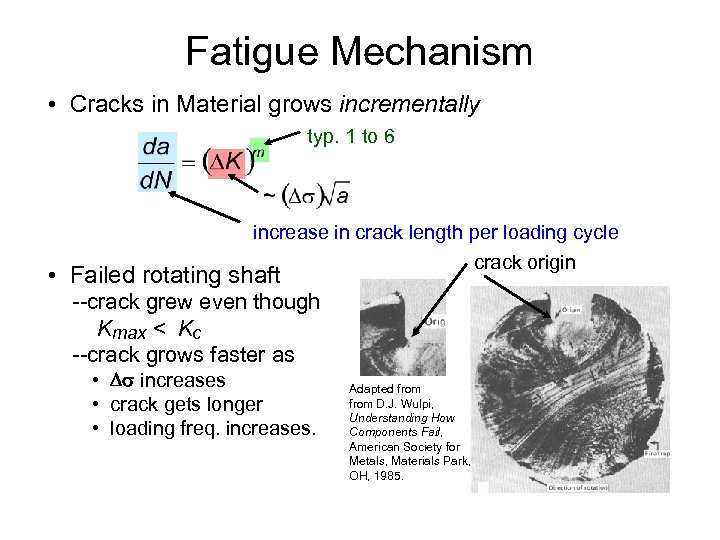Fatigue Mechanism • Cracks in Material grows incrementally typ. 1 to 6 increase in crack length per loading cycle crack origin • Failed rotating shaft --crack grew even though Kmax < Kc --crack grows faster as • D increases • crack gets longer • loading freq. increases. Adapted from D. J. Wulpi, Understanding How Components Fail, American Society for Metals, Materials Park, OH, 1985.

Figure 8. 11 An illustration of how repeated stress applications can generate localized plastic deformation at the alloy surface leading eventually to sharp discontinuities.

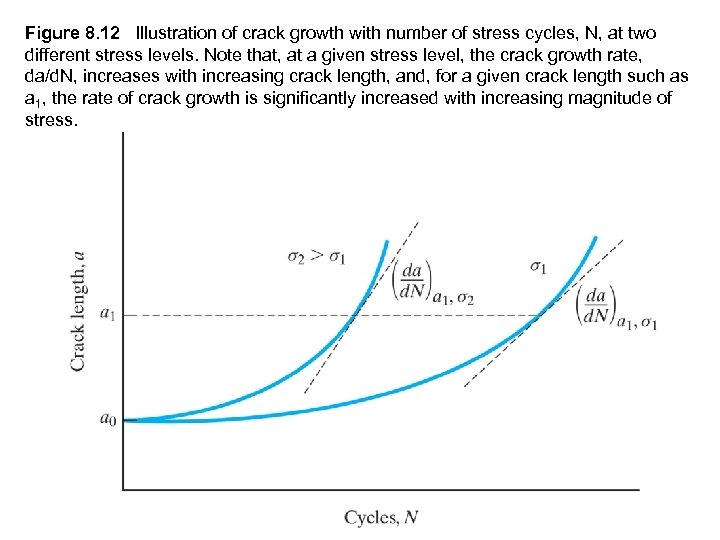Figure 8. 12 Illustration of crack growth with number of stress cycles, N, at two different stress levels. Note that, at a given stress level, the crack growth rate, da/d. N, increases with increasing crack length, and, for a given crack length such as a 1, the rate of crack growth is significantly increased with increasing magnitude of stress.

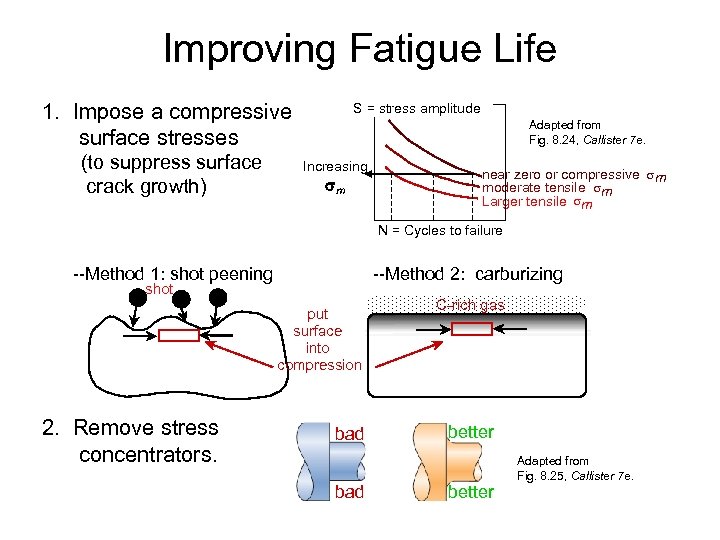Improving Fatigue Life 1. Impose a compressive surface stresses (to suppress surface crack growth) S = stress amplitude Adapted from Fig. 8. 24, Callister 7 e. Increasing m near zero or compressive m moderate tensile m Larger tensile m N = Cycles to failure --Method 1: shot peening --Method 2: carburizing shot put surface into compression 2. Remove stress concentrators. bad C-rich gas better Adapted from Fig. 8. 25, Callister 7 e.

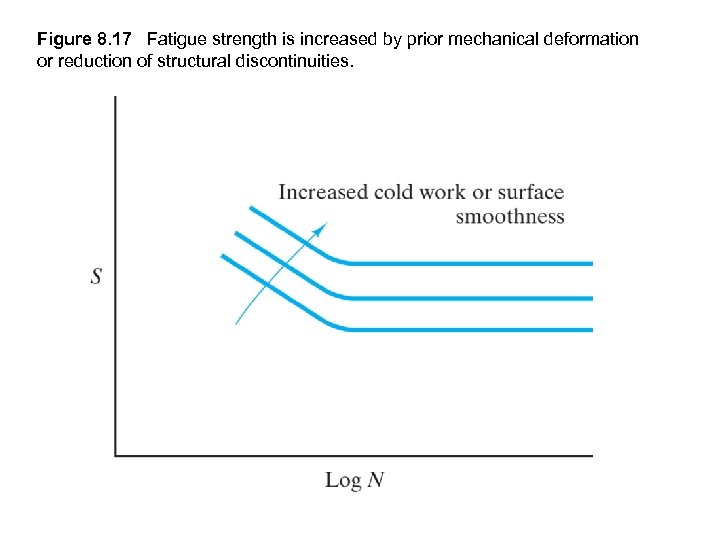Figure 8. 17 Fatigue strength is increased by prior mechanical deformation or reduction of structural discontinuities.

Other Issues in Failure – Stress Corrosion Cracking • Water can greatly accelerate crack growth and shorten life performance – in metals, ceramics and glasses • Other chemicals – that can generate (or provide H+ or O 2 -) ions – also effectively reduce fatigue life as these ions react with the metal or oxide in the material

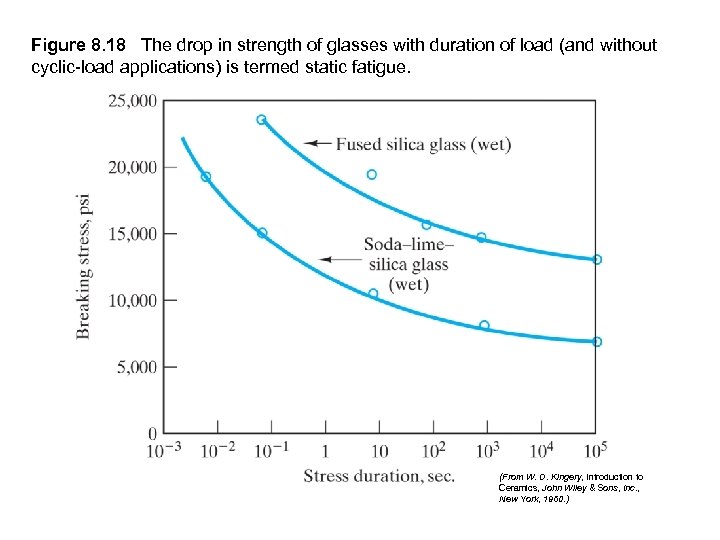Figure 8. 18 The drop in strength of glasses with duration of load (and without cyclic-load applications) is termed static fatigue. (From W. D. Kingery, Introduction to Ceramics, John Wiley & Sons, Inc. , New York, 1960. )

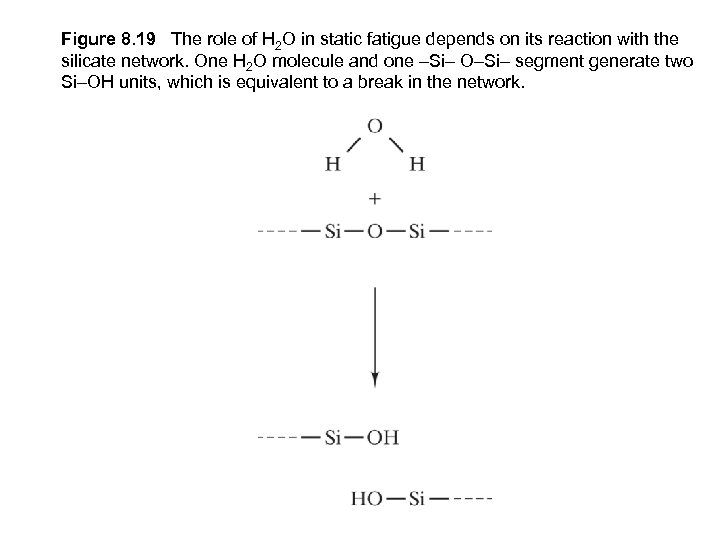Figure 8. 19 The role of H 2 O in static fatigue depends on its reaction with the silicate network. One H 2 O molecule and one –Si– O–Si– segment generate two Si–OH units, which is equivalent to a break in the network.

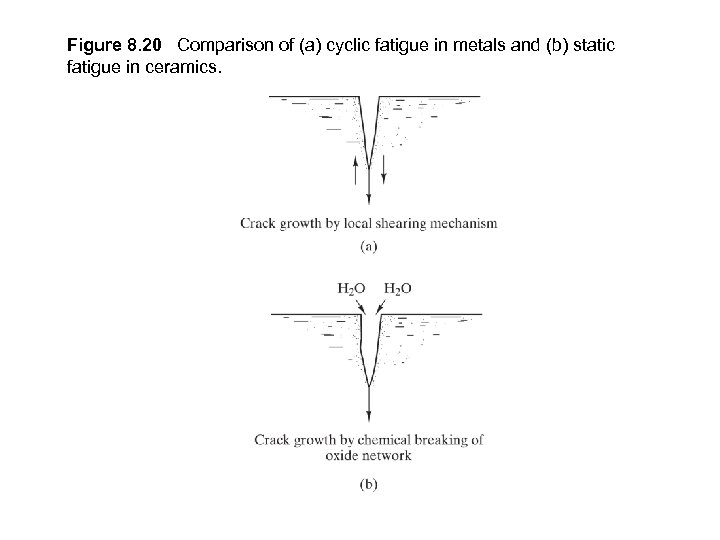Figure 8. 20 Comparison of (a) cyclic fatigue in metals and (b) static fatigue in ceramics.

SUMMARY • Engineering materials don't reach theoretical strength. • Flaws produce stress concentrations that cause premature failure. • Sharp corners produce large stress concentrations and premature failure. • Failure type depends on T and stress: - for noncyclic and T < 0. 4 Tm, failure stress decreases with: - increased maximum flaw size, - decreased T, - increased rate of loading. - for cyclic : - cycles to fail decreases as D increases. - for higher T (T > 0. 4 Tm): - time to fail decreases as or T increases.