CHAPTER 5 Probability What Are the Chances 5

CHAPTER 5 Probability: What Are the Chances? 5. 1 Randomness, Probability, and Simulation The Practice of Statistics, 5 th Edition Starnes, Tabor, Yates, Moore Bedford Freeman Worth Publishers

Randomness, Probability, and Simulation Learning Objectives After this section, you should be able to: ü INTERPRET probability as a long-run relative frequency. ü USE simulation to MODEL chance behavior. The Practice of Statistics, 5 th Edition 2

Aim – How do we interpret the meaning of probability? H. W. – Read section 5. 1 and do pages 300 – 302 #1, 3, 5, 8, 11, 17, 19, 21, 24 and 27 Do Now – Suppose you have a coin. 1. What is the probability of tossing a coin and getting heads? 2. What is the probability of tossing a coin and getting tails? The Practice of Statistics, 5 th Edition 3

The Idea of Probability Chance behavior is unpredictable in the short run, but has a regular and predictable pattern in the long run. The law of large numbers says that if we observe more and more repetitions of any chance process, the proportion of times that a specific outcome occurs approaches a single value. The probability of any outcome of a chance process is a number between 0 and 1 that describes the proportion of times the outcome would occur in a very long series of repetitions. The Practice of Statistics, 5 th Edition 4

Myths About Randomness The idea of probability seems straightforward. However, there are several myths of chance behavior we must address. The myth of short-run regularity: The idea of probability is that randomness is predictable in the long run. Our intuition tries to tell us random phenomena should also be predictable in the short run. However, probability does not allow us to make short-run predictions. The myth of the “law of averages”: Probability tells us random behavior evens out in the long run. Future outcomes are not affected by past behavior. That is, past outcomes do not influence the likelihood of individual outcomes occurring in the future. The Practice of Statistics, 5 th Edition 5

Check our understanding: 1. According to a recent study, the probability that a randomly selected U. S. adult usually eats breakfast is 0. 61. a. Explain what probability of 0. 61 means in this setting. Solution: • IF you ask a large sample of U. S. adults whether they eat breakfast about 61% of them will answer yes (how large is large? ? ? ) The Practice of Statistics, 5 th Edition 6

Check our understanding continued • Why doesn’t this probability say that if 100 U. S. adults are chosen at random, exactly 61 of them usually eat breakfast? • Solution: • The exact number of breakfast eaters will vary from sample to sample. The Practice of Statistics, 5 th Edition 7

Simulation The imitation of chance behavior, based on a model that accurately reflects the situation, is called a simulation. Performing a Simulation State: Ask a question of interest about some chance process. Plan: Describe how to use a chance device to imitate one repetition of the process. Tell what you will record at the end of each repetition. Do: Perform many repetitions of the simulation. Conclude: Use the results of your simulation to answer the question of interest. We can use physical devices, random numbers (e. g. Table D), and technology to perform simulations. The Practice of Statistics, 5 th Edition 8

Example: Simulations with technology In an attempt to increase sales, a breakfast cereal company decides to offer a NASCAR promotion. Each box of cereal will contain a collectible card featuring one of these NASCAR drivers: Jeff Gordon, Dale Earnhardt, Jr. , Tony Stewart, Danica Patrick, or Jimmie Johnson. The company says that each of the 5 cards is equally likely to appear in any box of cereal. A NASCAR fan decides to keep buying boxes of the cereal until she has all 5 drivers’ cards. She is surprised when it takes her 23 boxes to get the full set of cards. Should she be surprised? Problem: What is the probability that it will take 23 or more boxes to get a full set of 5 NASCAR collectible cards? The Practice of Statistics, 5 th Edition 9

Example: Simulations with technology Plan: We need five numbers to represent the five possible cards. Let’s let 1 = Jeff Gordon, 2 = Dale Earnhardt, Jr. , 3 = Tony Stewart, 4 = Danica Patrick, and 5 = Jimmie Johnson. We’ll use rand. Int(1, 5) to simulate buying one box of cereal and looking at which card is inside. Because we want a full set of cards, we’ll keep pressing Enter until we get all five of the labels from 1 to 5. We’ll record the number of boxes that we had to open. The Practice of Statistics, 5 th Edition 10

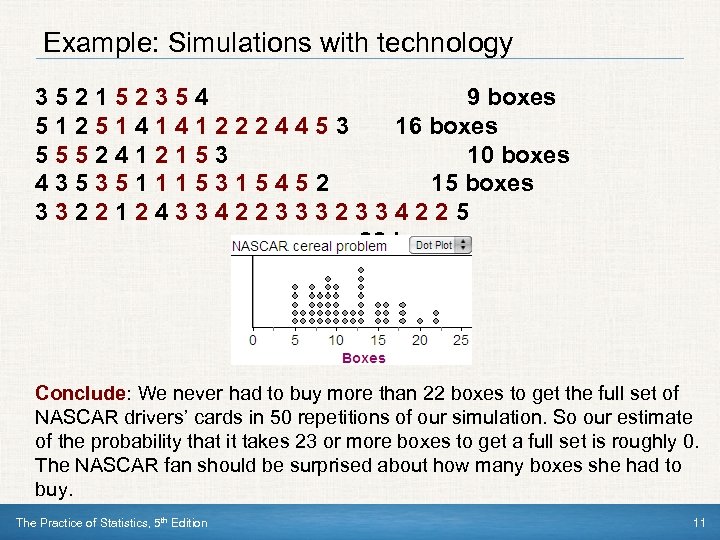
Example: Simulations with technology 352152354 9 boxes 5125141412224453 16 boxes 5552412153 10 boxes 435351115315452 15 boxes 3322124334223332334225 22 boxes Conclude: We never had to buy more than 22 boxes to get the full set of NASCAR drivers’ cards in 50 repetitions of our simulation. So our estimate of the probability that it takes 23 or more boxes to get a full set is roughly 0. The NASCAR fan should be surprised about how many boxes she had to buy. The Practice of Statistics, 5 th Edition 11

Using a table for simulation: • Textbook: The Practice of Statistics, 5 th Edition 12

Another example: • Suppose I want to choose a simple random sample of size 6 from a group of 60 seniors and 30 juniors. To do this, I write each person’s name on an equally-sized piece of paper and mix the papers in a large grocery bag. Just as I am about to select the first name, a thoughtful student suggests that I should stratify by class. I agree, and we decide it would be appropriate to select 4 seniors and 2 juniors. However, because I have already mixed up the names, I don’t want to have to separate them all again. Instead, I will select names one at a time from the bag until I get 4 seniors and 2 juniors. Design and carry out a simulation using Table D to estimate the probability that you must draw 10 or more names to get 4 seniors and 2 juniors. • State: What is the probability that it takes 10 or more selections to get 4 seniors and 2 juniors? • What would a possible simulation look like? The Practice of Statistics, 5 th Edition 13

Another example continued: • Plan: Using pairs of digits from Table D: • We’ll label the 60 seniors 01– 60 and the 30 juniors 61– 90. Numbers 00 and 91– 99 will be skipped. Moving left to right across a row, we’ll look at pairs of digits until we have 4 different labels from 01– 60 and 2 different labels from 61– 90. Then we will count how many different labels from 01– 90 we looked at. The Practice of Statistics, 5 th Edition 14

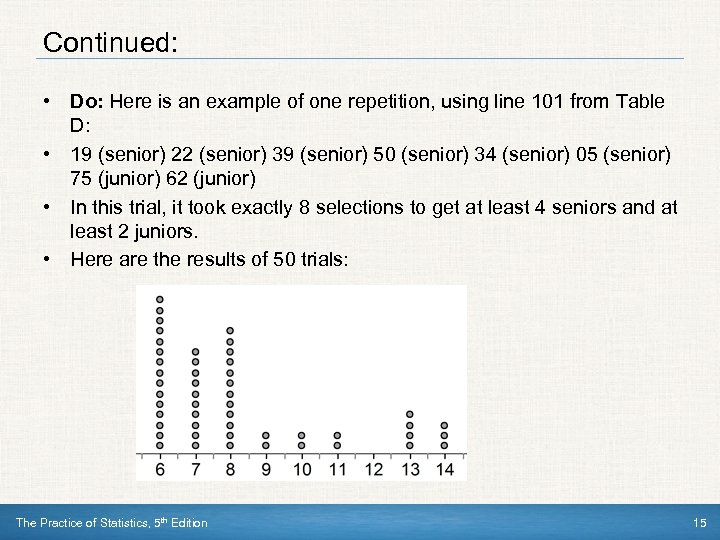
Continued: • Do: Here is an example of one repetition, using line 101 from Table D: • 19 (senior) 22 (senior) 39 (senior) 50 (senior) 34 (senior) 05 (senior) 75 (junior) 62 (junior) • In this trial, it took exactly 8 selections to get at least 4 seniors and at least 2 juniors. • Here are the results of 50 trials: The Practice of Statistics, 5 th Edition 15

Conclusion: • Conclude: In the simulation, 11 of the 50 trials required 10 or more selections to get 4 seniors and 2 juniors, so the probability that it takes 10 or more selections is approximately 0. 22. The Practice of Statistics, 5 th Edition 16

A. P. Exam tip • When students make conclusions, they often lose credit for suggesting that a claim is definitely true or saying that the evidence proves that a claim is incorrect. If the conclusion is based on a probability, students should always acknowledge that they can’t be certain that their conclusion is correct. A better response would be to say that there is convincing evidence (or there isn’t convincing evidence) to support a particular claim. The Practice of Statistics, 5 th Edition 17

Randomness, Probability, and Simulation Section Summary In this section, we learned how to… ü INTERPRET probability as a long-run relative frequency. ü USE simulation to MODEL chance behavior. The Practice of Statistics, 5 th Edition 18