cdc8b2f39b7923bbaadc80d40fa93002.ppt
- Количество слайдов: 30
 Chapter 5 Interest Rates Copyright © 2010 Pearson Prentice Hall. All rights reserved.
Chapter 5 Interest Rates Copyright © 2010 Pearson Prentice Hall. All rights reserved.
 Learning Objectives 1. Discuss how interest rates are quoted, and compute the effective annual rate (EAR) on a loan or investment. 2. Apply the TVM equations by accounting for the compounding periods per year. 3. Set up monthly amortization tables for consumer loans, and illustrate the payment changes as the compounding or annuity period changes. 4. Explain the real rate of interest and the effect of inflation on nominal interest rates. 5. Summarize the two major premiums that differentiate interest rates: the default premium and the maturity premium. 6. Amaze your family and friends with your knowledge of interest rate history. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -2
Learning Objectives 1. Discuss how interest rates are quoted, and compute the effective annual rate (EAR) on a loan or investment. 2. Apply the TVM equations by accounting for the compounding periods per year. 3. Set up monthly amortization tables for consumer loans, and illustrate the payment changes as the compounding or annuity period changes. 4. Explain the real rate of interest and the effect of inflation on nominal interest rates. 5. Summarize the two major premiums that differentiate interest rates: the default premium and the maturity premium. 6. Amaze your family and friends with your knowledge of interest rate history. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -2
 5. 1 How Interest Rates Are Quoted: Annual and Periodic Interest Rates • The most commonly quoted rate is the annual percentage rate (APR), It is the annual rate based on interest being computed once a year. • Lenders often charge interest on a non-annual basis. e. g. although 5% is quoted in annual basis, interest is in fact paid quarterly or monthly. • The period in which interest is applied or the frequency of times interest is added to an account each year is called compounding period. (m) • In such a case, the APR is divided by the number of compounding periods per year (C/Y or “m”) to calculate the periodic interest rate, so that the compounding period is taken into account. • For example: APR = 12%; m=12; i%=12%/12= 1% Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -3
5. 1 How Interest Rates Are Quoted: Annual and Periodic Interest Rates • The most commonly quoted rate is the annual percentage rate (APR), It is the annual rate based on interest being computed once a year. • Lenders often charge interest on a non-annual basis. e. g. although 5% is quoted in annual basis, interest is in fact paid quarterly or monthly. • The period in which interest is applied or the frequency of times interest is added to an account each year is called compounding period. (m) • In such a case, the APR is divided by the number of compounding periods per year (C/Y or “m”) to calculate the periodic interest rate, so that the compounding period is taken into account. • For example: APR = 12%; m=12; i%=12%/12= 1% Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -3
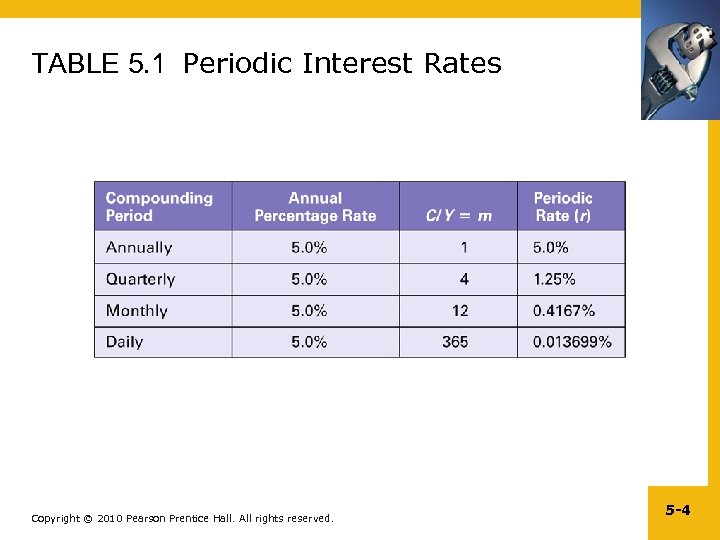 TABLE 5. 1 Periodic Interest Rates Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -4
TABLE 5. 1 Periodic Interest Rates Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -4
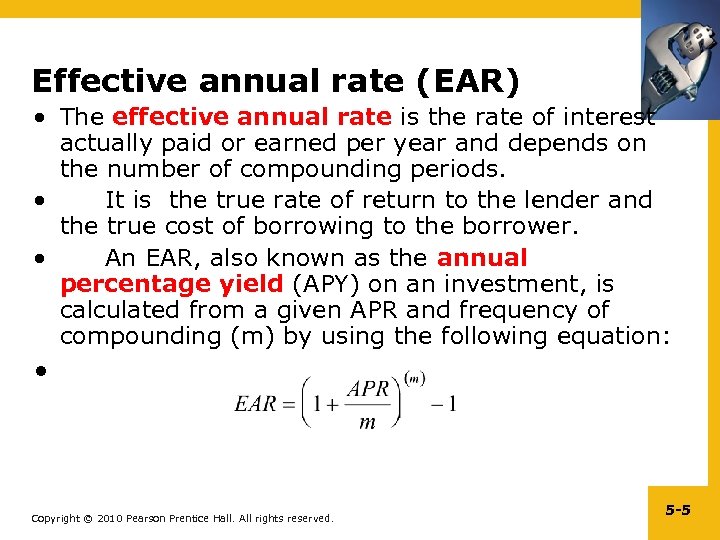 Effective annual rate (EAR) • The effective annual rate is the rate of interest actually paid or earned per year and depends on the number of compounding periods. • It is the true rate of return to the lender and the true cost of borrowing to the borrower. • An EAR, also known as the annual percentage yield (APY) on an investment, is calculated from a given APR and frequency of compounding (m) by using the following equation: • Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -5
Effective annual rate (EAR) • The effective annual rate is the rate of interest actually paid or earned per year and depends on the number of compounding periods. • It is the true rate of return to the lender and the true cost of borrowing to the borrower. • An EAR, also known as the annual percentage yield (APY) on an investment, is calculated from a given APR and frequency of compounding (m) by using the following equation: • Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -5
 5. 1 How Interest Rates Are Quoted: Annual and Periodic Interest Rates (continued) Example 1: Calculating an EAR or APY The First Common Bank has advertised one of its loan offerings as follows: “We will lend you $100, 000 for up to 3 years at an APR of 8. 5% (interest compounded monthly). ” If you borrow $100, 000 for 1 year, how much interest will you have paid and what is the bank’s APY? Nominal annual rate = APR = 8. 5% Frequency of compounding = C/Y = m = 12 Periodic interest rate = APR/m = 8. 5%/12 = 0. 70833% = . 0070833 APY or EAR = (1. 0070833)12 - 1 = 1. 08839 - 1 = 8. 839% Total interest paid after 1 year =. 08839*$100, 000 = $8, 839. 05 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -6
5. 1 How Interest Rates Are Quoted: Annual and Periodic Interest Rates (continued) Example 1: Calculating an EAR or APY The First Common Bank has advertised one of its loan offerings as follows: “We will lend you $100, 000 for up to 3 years at an APR of 8. 5% (interest compounded monthly). ” If you borrow $100, 000 for 1 year, how much interest will you have paid and what is the bank’s APY? Nominal annual rate = APR = 8. 5% Frequency of compounding = C/Y = m = 12 Periodic interest rate = APR/m = 8. 5%/12 = 0. 70833% = . 0070833 APY or EAR = (1. 0070833)12 - 1 = 1. 08839 - 1 = 8. 839% Total interest paid after 1 year =. 08839*$100, 000 = $8, 839. 05 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -6
 5. 2 Effect of Compounding Periods on the Time Value of Money Equations(TVM) TVM equations require the periodic rate (r%) and the number of periods (n) to be entered as inputs. The greater the frequency of payments made per year, the lower the total amount paid. More money goes to principal and less interest is charged. The interest rate entered should be consistent with the frequency of compounding and the number of payments involved. In other words, r and n must agree in terms of periods in the equation. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -7
5. 2 Effect of Compounding Periods on the Time Value of Money Equations(TVM) TVM equations require the periodic rate (r%) and the number of periods (n) to be entered as inputs. The greater the frequency of payments made per year, the lower the total amount paid. More money goes to principal and less interest is charged. The interest rate entered should be consistent with the frequency of compounding and the number of payments involved. In other words, r and n must agree in terms of periods in the equation. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -7
 • Assume that you purchase a 6 -year, 8 percent savings certificate for $1, 000. If interest is compounded semiannually, what will be the value of the certificate when it matures? • FV = 1000(1+8% )^ 6*2 2 = 1601. 03 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -8
• Assume that you purchase a 6 -year, 8 percent savings certificate for $1, 000. If interest is compounded semiannually, what will be the value of the certificate when it matures? • FV = 1000(1+8% )^ 6*2 2 = 1601. 03 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -8
 5. 2 Effect of Compounding Periods on the Time Value of Money Equations Example 2: Effect of Payment Frequency on Total Payment Jim needs to borrow $50, 000 for a business expansion project. His bank agrees to lend him the money over a 5 year term at an APR of 9% and will accept annual, quarterly, or monthly payments with no change in the quoted APR. Calculate the periodic payment under each alternative and compare the total amount paid each year under each option. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -9
5. 2 Effect of Compounding Periods on the Time Value of Money Equations Example 2: Effect of Payment Frequency on Total Payment Jim needs to borrow $50, 000 for a business expansion project. His bank agrees to lend him the money over a 5 year term at an APR of 9% and will accept annual, quarterly, or monthly payments with no change in the quoted APR. Calculate the periodic payment under each alternative and compare the total amount paid each year under each option. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -9
 5. 2 Effect of Compounding Periods on the Time Value of Money Equations (Example 2 Answer) Loan amount = $50, 000 Loan period = 5 years APR = 9% Annual payments: PV = 50000; n=5; i = 9; FV=0; P/Y=1; CPT PMT = $12, 854. 62 Quarterly payments: PV = 50000; n=20; i = 9; FV=0; P/Y=4; CPT PMT = $3132. 10 Total annual payment = $3132. 1*4 = $12, 528. 41 Monthly payments: PV = 50000; n=60; i = 9; FV=0; P/Y=12; CPT PMT = $1037. 92 Total annual payment = $1037. 92*12 = $12, 455. 04 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -10
5. 2 Effect of Compounding Periods on the Time Value of Money Equations (Example 2 Answer) Loan amount = $50, 000 Loan period = 5 years APR = 9% Annual payments: PV = 50000; n=5; i = 9; FV=0; P/Y=1; CPT PMT = $12, 854. 62 Quarterly payments: PV = 50000; n=20; i = 9; FV=0; P/Y=4; CPT PMT = $3132. 10 Total annual payment = $3132. 1*4 = $12, 528. 41 Monthly payments: PV = 50000; n=60; i = 9; FV=0; P/Y=12; CPT PMT = $1037. 92 Total annual payment = $1037. 92*12 = $12, 455. 04 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -10
 5. 2 Effect of Compounding Periods on the Time Value of Money Equations Example 3: Comparing Annual and Monthly Deposits Joshua, who is currently 25 years old, wants to invest money into a retirement fund so as to have $2, 000 saved up when he retires at age 65. If he can earn 12% per year in an equity fund, calculate the amount of money he would have to invest in equal annual amounts and alternatively, in equal monthly amounts starting at the end of the current year or month, respectively. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -11
5. 2 Effect of Compounding Periods on the Time Value of Money Equations Example 3: Comparing Annual and Monthly Deposits Joshua, who is currently 25 years old, wants to invest money into a retirement fund so as to have $2, 000 saved up when he retires at age 65. If he can earn 12% per year in an equity fund, calculate the amount of money he would have to invest in equal annual amounts and alternatively, in equal monthly amounts starting at the end of the current year or month, respectively. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -11
 5. 2 Effect of Compounding Periods on the Time Value of Money Equations (Example 3 Answer) With annual deposits: FV = $2, 000; N = 40 years; I/Y = APR = 12%; PV = 0; C/Y=1; PMT = $2, 607. 25 With monthly deposits: (Using the APR as the interest rate) FV = $2, 000; N = 12*40=480; I/Y = APR = 12%; PV = 0; C/Y = 12 PMT = $169. 99 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -12
5. 2 Effect of Compounding Periods on the Time Value of Money Equations (Example 3 Answer) With annual deposits: FV = $2, 000; N = 40 years; I/Y = APR = 12%; PV = 0; C/Y=1; PMT = $2, 607. 25 With monthly deposits: (Using the APR as the interest rate) FV = $2, 000; N = 12*40=480; I/Y = APR = 12%; PV = 0; C/Y = 12 PMT = $169. 99 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -12
 5. 3 Consumer Loans and Amortization Schedules Interest is charged only on the outstanding balance of a typical consumer loan. Increases in frequency and size of payments result in reduced interest charges and quicker payoff due to more being applied to loan balance. Amortization schedules help in planning and analysis of consumer loans. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -13
5. 3 Consumer Loans and Amortization Schedules Interest is charged only on the outstanding balance of a typical consumer loan. Increases in frequency and size of payments result in reduced interest charges and quicker payoff due to more being applied to loan balance. Amortization schedules help in planning and analysis of consumer loans. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -13
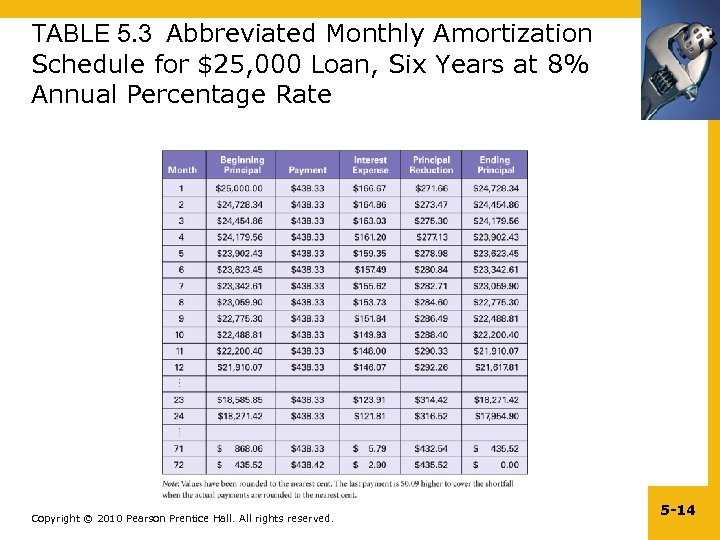 TABLE 5. 3 Abbreviated Monthly Amortization Schedule for $25, 000 Loan, Six Years at 8% Annual Percentage Rate Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -14
TABLE 5. 3 Abbreviated Monthly Amortization Schedule for $25, 000 Loan, Six Years at 8% Annual Percentage Rate Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -14
 5. 3 Consumer Loans and Amortization Schedules (continued) Example 4: Paying Off a Loan Early! Kay has just taken out a $200, 000, 30 -year, 5% mortgage. She has heard from friends that if she increases the size of her monthly payment by one-twelfth of the monthly payment, she will be able to pay off the loan much earlier and save a bundle on interest costs. She is not convinced. Use the necessary calculations to help convince her that this is, in fact, true. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -15
5. 3 Consumer Loans and Amortization Schedules (continued) Example 4: Paying Off a Loan Early! Kay has just taken out a $200, 000, 30 -year, 5% mortgage. She has heard from friends that if she increases the size of her monthly payment by one-twelfth of the monthly payment, she will be able to pay off the loan much earlier and save a bundle on interest costs. She is not convinced. Use the necessary calculations to help convince her that this is, in fact, true. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -15
 5. 3 Consumer Loans and Amortization Schedules (continued) Example 4 (Answer) We first solve for the required minimum monthly payment: PV = $200, 000; I/Y=5; N=30*12=360; FV=0; C/Y=12; P/Y=12; PMT = ? $1073. 64 Next, we calculate the number of payments required to pay off the loan, if the monthly payment is increased by 1/12*$1073. 64 i. e. by $89. 47 PMT = 1163. 11; PV=$200, 000; FV=0; I/Y=5; C/Y=12; P/Y=12; N = ? N= 303. 13 months, or 303. 13/12 = 25. 26 years. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -16
5. 3 Consumer Loans and Amortization Schedules (continued) Example 4 (Answer) We first solve for the required minimum monthly payment: PV = $200, 000; I/Y=5; N=30*12=360; FV=0; C/Y=12; P/Y=12; PMT = ? $1073. 64 Next, we calculate the number of payments required to pay off the loan, if the monthly payment is increased by 1/12*$1073. 64 i. e. by $89. 47 PMT = 1163. 11; PV=$200, 000; FV=0; I/Y=5; C/Y=12; P/Y=12; N = ? N= 303. 13 months, or 303. 13/12 = 25. 26 years. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -16
 5. 3 Consumer Loans and Amortization Schedules (continued) Example 4 (Answer—continued) With minimum monthly payments: Total paid = 360*$1073. 64 = $386, 510. 4 Amount borrowed = $200, 000. 0 Interest paid = $186, 510. 4 With higher monthly payments: Total paid = 303. 13*$1163. 11 = $352, 573. 53 Amount borrowed = $200, 000. 00 Interest paid = $152, 573. 53 Interest saved=$186, 510. 4 -$152, 573. 53 = $33, 936. 87 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -17
5. 3 Consumer Loans and Amortization Schedules (continued) Example 4 (Answer—continued) With minimum monthly payments: Total paid = 360*$1073. 64 = $386, 510. 4 Amount borrowed = $200, 000. 0 Interest paid = $186, 510. 4 With higher monthly payments: Total paid = 303. 13*$1163. 11 = $352, 573. 53 Amount borrowed = $200, 000. 00 Interest paid = $152, 573. 53 Interest saved=$186, 510. 4 -$152, 573. 53 = $33, 936. 87 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -17
 5. 4 Nominal and Real Interest Rates • Nominal interest rates are made up of two primary components inflation and the real interest rate. • It is essentially a compensation paid for the giving up of current consumption by the investor. • The real rate of interest(reward for waiting) adjusts for the erosion of purchasing power caused by inflation. • Real interest rate(r*) =nominal rate –expected inflation • The Fisher Effect shown below is the equation that shows the relationship between the real rate (r*), the inflation rate (h), and the nominal interest rate (r): (1 + r) = (1 + r*) x (1 + h) r = (1 + r*) x (1 + h) – 1 r = r* + h + (r* x h) Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -18
5. 4 Nominal and Real Interest Rates • Nominal interest rates are made up of two primary components inflation and the real interest rate. • It is essentially a compensation paid for the giving up of current consumption by the investor. • The real rate of interest(reward for waiting) adjusts for the erosion of purchasing power caused by inflation. • Real interest rate(r*) =nominal rate –expected inflation • The Fisher Effect shown below is the equation that shows the relationship between the real rate (r*), the inflation rate (h), and the nominal interest rate (r): (1 + r) = (1 + r*) x (1 + h) r = (1 + r*) x (1 + h) – 1 r = r* + h + (r* x h) Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -18
 5. 5 Risk-Free Rate and Premiums • A risk free rate (rf) is the rate of return for an investment with zero risk. • The nominal risk-free rate of interest such as the rate of return on a Treasury bill includes the real rate of interest and the inflation premium. • Rf=r*+inf • The rate of return on all other riskier investments (r) would have to include a default risk premium (dp)and a maturity risk premium (mp): i. e. r = r* + inf + dp + mp. • 30 -year corporate bond yield > 30 -year T-bond yield – Due to the higher default risk on the corporate bond investment. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -19
5. 5 Risk-Free Rate and Premiums • A risk free rate (rf) is the rate of return for an investment with zero risk. • The nominal risk-free rate of interest such as the rate of return on a Treasury bill includes the real rate of interest and the inflation premium. • Rf=r*+inf • The rate of return on all other riskier investments (r) would have to include a default risk premium (dp)and a maturity risk premium (mp): i. e. r = r* + inf + dp + mp. • 30 -year corporate bond yield > 30 -year T-bond yield – Due to the higher default risk on the corporate bond investment. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -19
 Risk Premiums • Default premium compensates the lender for the risk if the borrower is unable to repay or risk associated with the varying types of collateral. The higher the risk, the higher the premium. • Maturity premium compensates the investor for the additional waiting time or the lender for the additional time it takes to receive repayment in full. The longer the period the higher the premium. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -20
Risk Premiums • Default premium compensates the lender for the risk if the borrower is unable to repay or risk associated with the varying types of collateral. The higher the risk, the higher the premium. • Maturity premium compensates the investor for the additional waiting time or the lender for the additional time it takes to receive repayment in full. The longer the period the higher the premium. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -20
 FIGURE 5. 1 Interest rate dimensions Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -21
FIGURE 5. 1 Interest rate dimensions Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -21
 ADDITIONAL PROBLEMS WITH ANSWERS Problem 1 Calculating APY or EAR. The First Federal Bank has advertised one of its loan offerings as follows: “We will lend you $100, 000 for up to 5 years at an APR of 9. 5% (interest compounded monthly. )” If you borrow $100, 000 for 1 year and pay it off in one lump sum at the end of the year, how much interest will you have paid and what is the bank’s APY? Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -22
ADDITIONAL PROBLEMS WITH ANSWERS Problem 1 Calculating APY or EAR. The First Federal Bank has advertised one of its loan offerings as follows: “We will lend you $100, 000 for up to 5 years at an APR of 9. 5% (interest compounded monthly. )” If you borrow $100, 000 for 1 year and pay it off in one lump sum at the end of the year, how much interest will you have paid and what is the bank’s APY? Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -22
 ADDITIONAL PROBLEMS WITH ANSWERS Problem 1 (ANSWER) Nominal annual rate = APR = 9. 5% Frequency of compounding = C/Y = m = 12 Periodic interest rate = APR/m = 9. 5%/12 = 0. 79167% =. 0079167 APY or EAR = (1. 0079167)12 - 1 = 1. 099247 - 1 9. 92% Amount of interest paid =9. 92%*100, 000=$9920. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -23
ADDITIONAL PROBLEMS WITH ANSWERS Problem 1 (ANSWER) Nominal annual rate = APR = 9. 5% Frequency of compounding = C/Y = m = 12 Periodic interest rate = APR/m = 9. 5%/12 = 0. 79167% =. 0079167 APY or EAR = (1. 0079167)12 - 1 = 1. 099247 - 1 9. 92% Amount of interest paid =9. 92%*100, 000=$9920. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -23
 ADDITIONAL PROBLEMS WITH ANSWERS Problem 2 EAR with Monthly Compounding If First Federal offers to structure the 9. 5%, $100, 000, 1 year loan on a monthly payment basis, calculate your monthly payment and the amount of interest paid at the end of the year. What is your EAR? Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -24
ADDITIONAL PROBLEMS WITH ANSWERS Problem 2 EAR with Monthly Compounding If First Federal offers to structure the 9. 5%, $100, 000, 1 year loan on a monthly payment basis, calculate your monthly payment and the amount of interest paid at the end of the year. What is your EAR? Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -24
 ADDITIONAL PROBLEMS WITH ANSWERS Problem 2 (ANSWER) Calculate monthly payment: Total interest paid after 1 year = 12*$8, 768. 35 - $100, 000 = $105, 220. 20 -$100, 000 = $5, 220. 20 EAR is still 9. 92%, since the APR and m are the same as #1 above, APY or EAR = (1. 0079167)12 - 1 = 1. 099247 - 1 =9. 92% Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -25
ADDITIONAL PROBLEMS WITH ANSWERS Problem 2 (ANSWER) Calculate monthly payment: Total interest paid after 1 year = 12*$8, 768. 35 - $100, 000 = $105, 220. 20 -$100, 000 = $5, 220. 20 EAR is still 9. 92%, since the APR and m are the same as #1 above, APY or EAR = (1. 0079167)12 - 1 = 1. 099247 - 1 =9. 92% Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -25
 ADDITIONAL PROBLEMS WITH ANSWERS Problem 3 Monthly versus Quarterly Payments: Patrick needs to borrow $70, 000 to start a business expansion project. His bank agrees to lend him the money over a 5 -year term at an APR of 9. 25% and will accept either monthly or quarterly payments with no change in the quoted APR. Calculate the periodic payment under each alternative and compare the total amount paid each year under each option. Which payment term should Patrick accept and why? Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -26
ADDITIONAL PROBLEMS WITH ANSWERS Problem 3 Monthly versus Quarterly Payments: Patrick needs to borrow $70, 000 to start a business expansion project. His bank agrees to lend him the money over a 5 -year term at an APR of 9. 25% and will accept either monthly or quarterly payments with no change in the quoted APR. Calculate the periodic payment under each alternative and compare the total amount paid each year under each option. Which payment term should Patrick accept and why? Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -26
 ADDITIONAL PROBLEMS WITH ANSWERS Problem 3 (ANSWER) Calculate monthly payment: n=60; i/y = 9. 25%/12; PV = 70000; FV=0; PMT= -1, 461. 59 Calculate quarterly payment: n=20; i/y = 9. 25%/4; PV = 70000; FV=0; PMT= -4, 411. 15 Total amount paid per year under each payment type: With monthly payments = 12* $1, 461. 59 = $17, 539. 08 With quarterly payments = 4*$4, 411. 15 = $17, 644. 60 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -27
ADDITIONAL PROBLEMS WITH ANSWERS Problem 3 (ANSWER) Calculate monthly payment: n=60; i/y = 9. 25%/12; PV = 70000; FV=0; PMT= -1, 461. 59 Calculate quarterly payment: n=20; i/y = 9. 25%/4; PV = 70000; FV=0; PMT= -4, 411. 15 Total amount paid per year under each payment type: With monthly payments = 12* $1, 461. 59 = $17, 539. 08 With quarterly payments = 4*$4, 411. 15 = $17, 644. 60 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -27
 ADDITIONAL PROBLEMS WITH ANSWERS Problem 3 (ANSWER continued) Total interest paid under monthly compounding: Total paid - Amount borrowed = 60*$1, 461. 59 - $70, 000 = $87, 695. 4 - $70, 000 = $17, 695. 4 Total interest paid under quarterly compounding: 20 *$4, 411. 15 -$70, 000 = $88, 223 - $70, 000 = $18, 223 Since less interest is paid over the 5 years with the monthly payment terms, Patrick should accept monthly rather than quarterly payment terms. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -28
ADDITIONAL PROBLEMS WITH ANSWERS Problem 3 (ANSWER continued) Total interest paid under monthly compounding: Total paid - Amount borrowed = 60*$1, 461. 59 - $70, 000 = $87, 695. 4 - $70, 000 = $17, 695. 4 Total interest paid under quarterly compounding: 20 *$4, 411. 15 -$70, 000 = $88, 223 - $70, 000 = $18, 223 Since less interest is paid over the 5 years with the monthly payment terms, Patrick should accept monthly rather than quarterly payment terms. Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -28
 ADDITIONAL PROBLEMS WITH ANSWERS Problem 4 Computing Payment for an Early Payoff: You have just taken on a 30 -year, 6%, $300, 000 mortgage and would like to pay it off in 20 years. By how much will your monthly payment have to change to accomplish this objective? Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -29
ADDITIONAL PROBLEMS WITH ANSWERS Problem 4 Computing Payment for an Early Payoff: You have just taken on a 30 -year, 6%, $300, 000 mortgage and would like to pay it off in 20 years. By how much will your monthly payment have to change to accomplish this objective? Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -29
 ADDITIONAL PROBLEMS WITH ANSWERS Problem 4 (ANSWER) Calculate the current monthly payment under the 30 -year, 6% terms: n=360; i/y = 6%/12; PV = 300, 000; FV=0; CPT PMT 1, 798. 65 Next, calculate the payment required to pay off the loan in 20 years or 240 payments: n=240; i/y = 6%/12; PV = 300, 000; FV=0; CPT PMT 2, 149. 29 The increase in monthly payment required to pay off the loan in 20 years = $2, 149. 29 - $1, 798. 65 = $350. 64 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -30
ADDITIONAL PROBLEMS WITH ANSWERS Problem 4 (ANSWER) Calculate the current monthly payment under the 30 -year, 6% terms: n=360; i/y = 6%/12; PV = 300, 000; FV=0; CPT PMT 1, 798. 65 Next, calculate the payment required to pay off the loan in 20 years or 240 payments: n=240; i/y = 6%/12; PV = 300, 000; FV=0; CPT PMT 2, 149. 29 The increase in monthly payment required to pay off the loan in 20 years = $2, 149. 29 - $1, 798. 65 = $350. 64 Copyright © 2010 Pearson Prentice Hall. All rights reserved. 5 -30


