Chapter 3 What Do Interest Rates Mean and






















3_present_value.ppt
- Количество слайдов: 39
 Chapter 3 What Do Interest Rates Mean and What is Their Role in Valuation? Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-1
Chapter 3 What Do Interest Rates Mean and What is Their Role in Valuation? Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-1
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-2 Preview We develop a better understanding of interest rates. We examine the terminology and calculation of various rates, and we show the importance of these rates in our lives and the general economy. Topics include: Measuring Interest Rates The Distinction Between Real and Nominal Interest Rates The Distinction Between Interest Rates and Returns
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-2 Preview We develop a better understanding of interest rates. We examine the terminology and calculation of various rates, and we show the importance of these rates in our lives and the general economy. Topics include: Measuring Interest Rates The Distinction Between Real and Nominal Interest Rates The Distinction Between Interest Rates and Returns
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-3 The Big Picture Since interest rates are among the most closely watched variables in the economy, it is imperative that what exactly is meant by the phrase interest rates is understood. In this chapter, we will see that a concept known as yield to maturity (YTM) is the most accurate measure of interest rates.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-3 The Big Picture Since interest rates are among the most closely watched variables in the economy, it is imperative that what exactly is meant by the phrase interest rates is understood. In this chapter, we will see that a concept known as yield to maturity (YTM) is the most accurate measure of interest rates.
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-4 The Big Picture Any description of interest rates will entail understanding certain vernacular and definitions, most of which will not only pertain directly to interest rates but will also be vital to understanding many other foundational concepts presented later in the text.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-4 The Big Picture Any description of interest rates will entail understanding certain vernacular and definitions, most of which will not only pertain directly to interest rates but will also be vital to understanding many other foundational concepts presented later in the text.
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-5 Present Value Introduction Different debt instruments have very different streams of cash payments to the holder (known as cash flows), with very different timing. All else being equal, debt instruments are evaluated against one another based on the amount of each cash flow and the timing of each cash flow. This evaluation, where the analysis of the amount and timing of a debt instrument’s cash flows lead to its yield to maturity or interest rate, is called present value analysis.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-5 Present Value Introduction Different debt instruments have very different streams of cash payments to the holder (known as cash flows), with very different timing. All else being equal, debt instruments are evaluated against one another based on the amount of each cash flow and the timing of each cash flow. This evaluation, where the analysis of the amount and timing of a debt instrument’s cash flows lead to its yield to maturity or interest rate, is called present value analysis.
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-6 1. Present Value The concept of present value (or present discounted value) is based on the commonsense notion that a dollar of cash flow paid to you one year from now is less valuable to you than a dollar paid to you today. This notion is true because you could invest the dollar in a savings account that earns interest and have more than a dollar in one year. The term present value (PV) refers to the current value of a future amount of money, or a series of payments, evaluated at a given interest rate.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-6 1. Present Value The concept of present value (or present discounted value) is based on the commonsense notion that a dollar of cash flow paid to you one year from now is less valuable to you than a dollar paid to you today. This notion is true because you could invest the dollar in a savings account that earns interest and have more than a dollar in one year. The term present value (PV) refers to the current value of a future amount of money, or a series of payments, evaluated at a given interest rate.
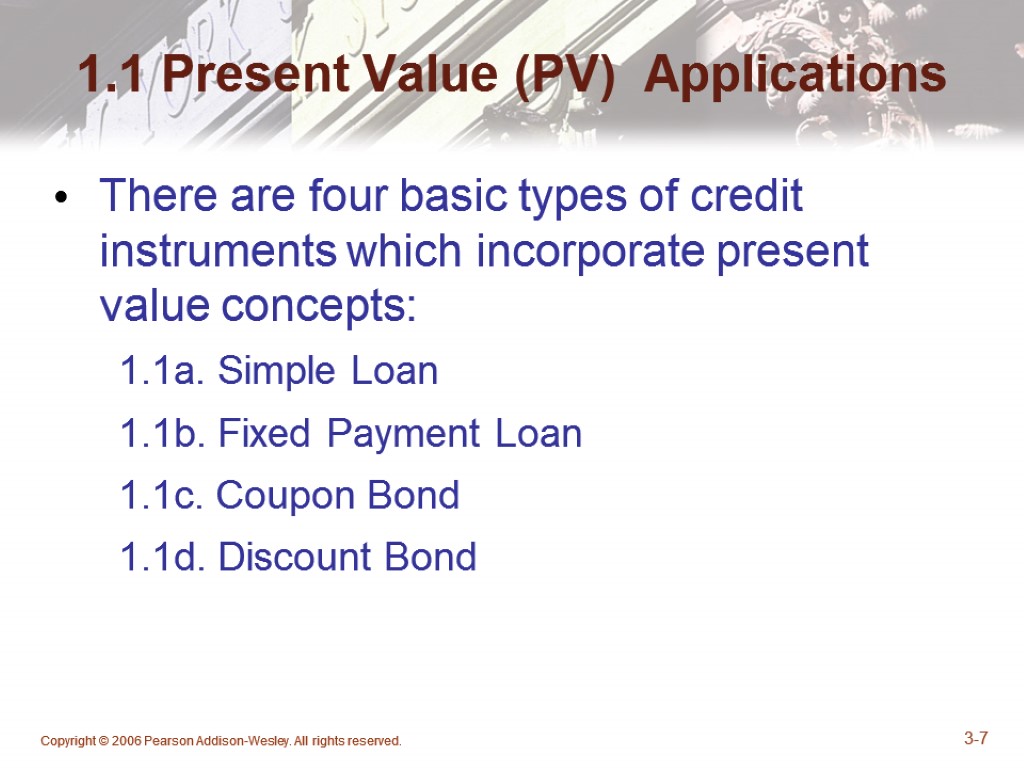
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-7 1.1 Present Value (PV) Applications There are four basic types of credit instruments which incorporate present value concepts: 1.1a. Simple Loan 1.1b. Fixed Payment Loan 1.1c. Coupon Bond 1.1d. Discount Bond
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-7 1.1 Present Value (PV) Applications There are four basic types of credit instruments which incorporate present value concepts: 1.1a. Simple Loan 1.1b. Fixed Payment Loan 1.1c. Coupon Bond 1.1d. Discount Bond
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-8 1.1a. Simple Loan Terms: Present Value Concept Loan Principal: the amount of funds the lender provides to the borrower. Maturity Date: the date the loan must be repaid; the Loan Term is from initiation to maturity date. Interest Payment: the cash amount that the borrower must pay the lender for the use of the loan principal. Simple Interest Rate: the interest payment divided by the loan principal; the percentage of principal that must be paid as interest to the lender. Convention is to express on an annual basis, irrespective of the loan term.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-8 1.1a. Simple Loan Terms: Present Value Concept Loan Principal: the amount of funds the lender provides to the borrower. Maturity Date: the date the loan must be repaid; the Loan Term is from initiation to maturity date. Interest Payment: the cash amount that the borrower must pay the lender for the use of the loan principal. Simple Interest Rate: the interest payment divided by the loan principal; the percentage of principal that must be paid as interest to the lender. Convention is to express on an annual basis, irrespective of the loan term.
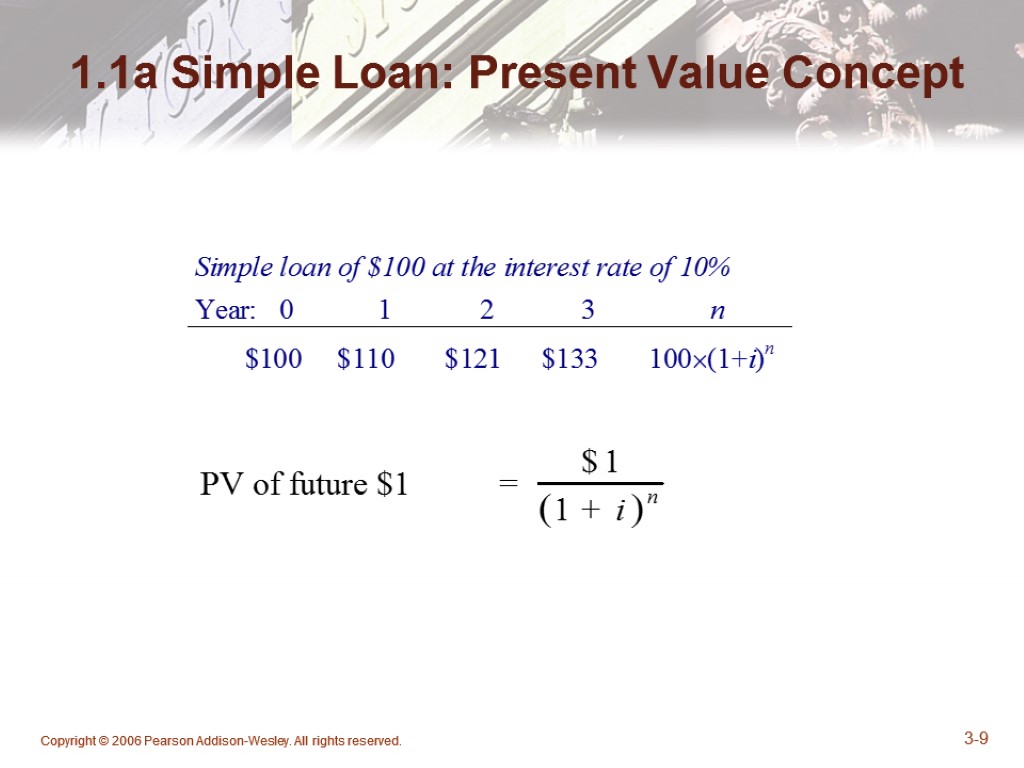
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-9 1.1a Simple Loan: Present Value Concept
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-9 1.1a Simple Loan: Present Value Concept
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-10 1.1a Simple Loan (cont.) The previous example reinforces the concept that $100 today is preferable to $100 a year from now since today’s $100 could be lent out (or deposited) at 10% interest to be worth $110 one year from now, or $121 in two years or $133 in three years. or The present value (PV) of $110 to be received one year from now is $100 if discounted at 10%. The PV of $121 ......
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-10 1.1a Simple Loan (cont.) The previous example reinforces the concept that $100 today is preferable to $100 a year from now since today’s $100 could be lent out (or deposited) at 10% interest to be worth $110 one year from now, or $121 in two years or $133 in three years. or The present value (PV) of $110 to be received one year from now is $100 if discounted at 10%. The PV of $121 ......
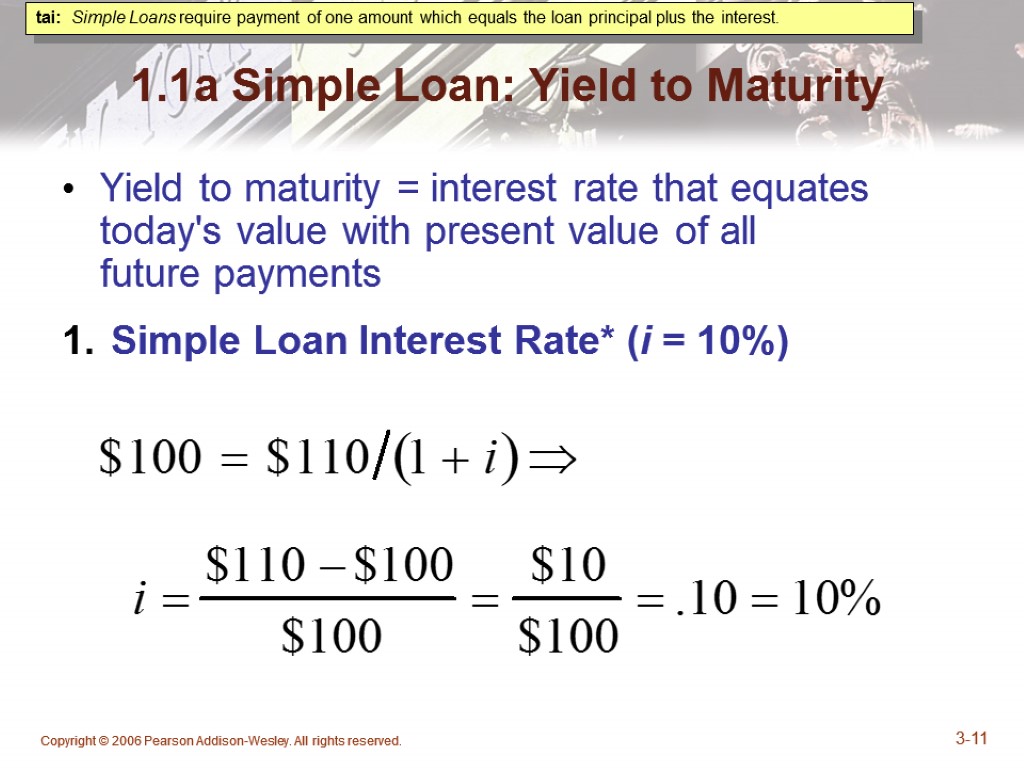
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-11 1.1a Simple Loan: Yield to Maturity Yield to maturity = interest rate that equates today's value with present value of all future payments Simple Loan Interest Rate* (i = 10%) tai: Simple Loans require payment of one amount which equals the loan principal plus the interest.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-11 1.1a Simple Loan: Yield to Maturity Yield to maturity = interest rate that equates today's value with present value of all future payments Simple Loan Interest Rate* (i = 10%) tai: Simple Loans require payment of one amount which equals the loan principal plus the interest.
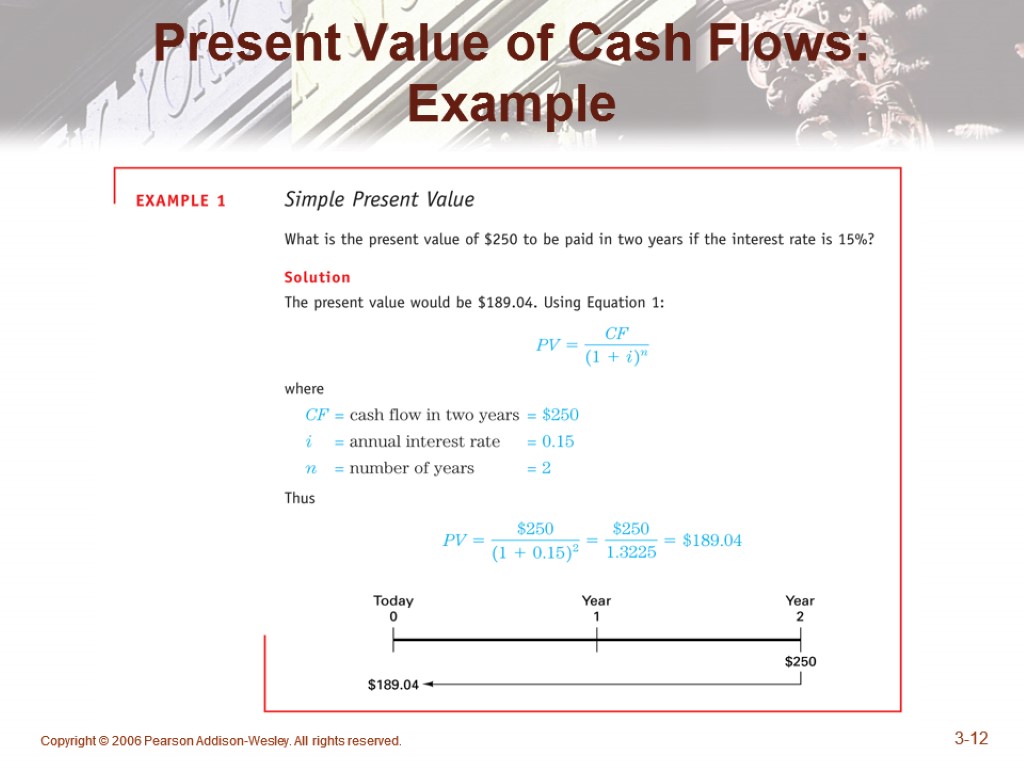
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-12 Present Value of Cash Flows: Example
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-12 Present Value of Cash Flows: Example
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-13 1.1b Fixed-Payment Loan Terms: Present Value Concept 1.1b Fixed-Payment Loans are loans where the loan principal and interest are repaid in several payments, often monthly, in equal dollar amounts over the loan term. The loan of equal payments is an example of an annuity. Installment Loans, such as auto loans and home mortgages are frequently of the fixed-payment type.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-13 1.1b Fixed-Payment Loan Terms: Present Value Concept 1.1b Fixed-Payment Loans are loans where the loan principal and interest are repaid in several payments, often monthly, in equal dollar amounts over the loan term. The loan of equal payments is an example of an annuity. Installment Loans, such as auto loans and home mortgages are frequently of the fixed-payment type.
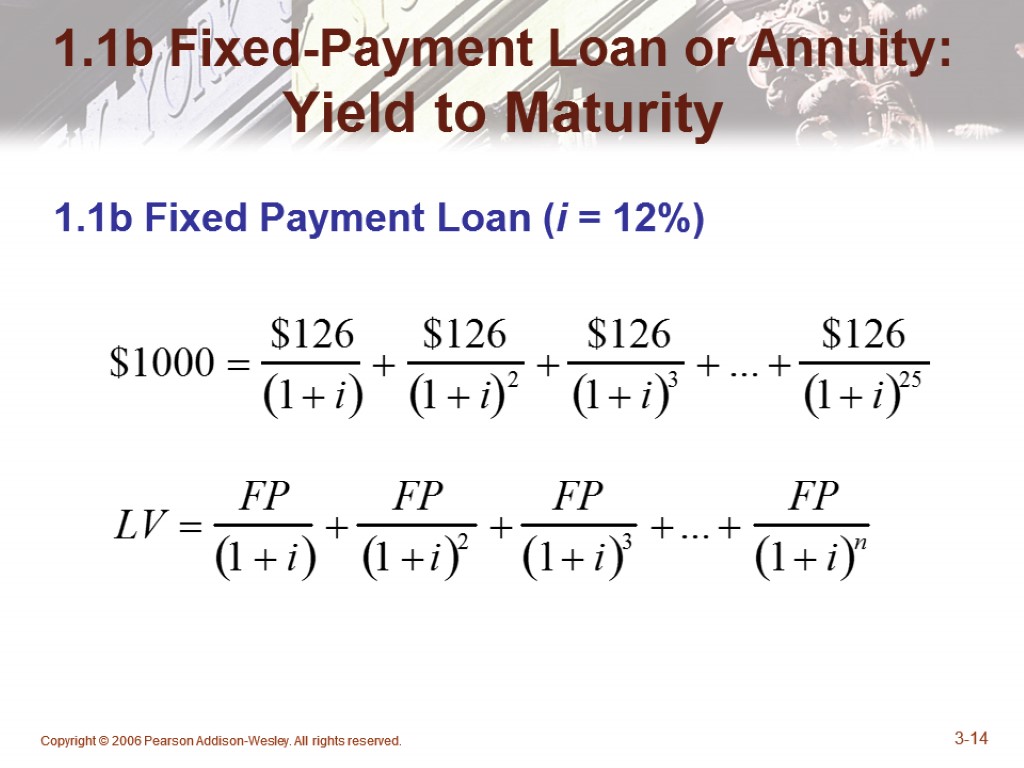
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-14 1.1b Fixed-Payment Loan or Annuity: Yield to Maturity 1.1b Fixed Payment Loan (i = 12%)
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-14 1.1b Fixed-Payment Loan or Annuity: Yield to Maturity 1.1b Fixed Payment Loan (i = 12%)
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-15 1.1C Coupon Bonds: Yield to Maturity 1.1C Coupon Bond (Coupon rate = 10% = C/F)
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-15 1.1C Coupon Bonds: Yield to Maturity 1.1C Coupon Bond (Coupon rate = 10% = C/F)
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-16 1.1C Coupon Bonds: Yield to Maturity For a 5 year maturity coupon bond that sold for exactly $1,121 (market price) and if its coupon payments are as follows, then its yield to maturity is: $1,121= $110/(1 + i) + $121/(1 + i)2 + $133/(1 + i)3 + $146.4/(1 + i)4 + $161/(1 + i)5 + $1,000/(1 + i)5 If we try i as 11%, we have: $1,121= $110 x 0.901+ $121x 0.812 + $133 x 0.731+ $146.4 x 0.659 + $161 x0.593 + $1,000x0.593 = $99.11 +$98.3 + $97.2 + $96.5 + $688.5 = $1,079.6 If we try10%, we have: $1,121= $110x0.909 + $121x0.826 +$133x 0.751 + $146.4 x 0.683 + $1,161 x 0.621 =$100 +$100 + $100 + $100 +$100 +$621 = $1,121
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-16 1.1C Coupon Bonds: Yield to Maturity For a 5 year maturity coupon bond that sold for exactly $1,121 (market price) and if its coupon payments are as follows, then its yield to maturity is: $1,121= $110/(1 + i) + $121/(1 + i)2 + $133/(1 + i)3 + $146.4/(1 + i)4 + $161/(1 + i)5 + $1,000/(1 + i)5 If we try i as 11%, we have: $1,121= $110 x 0.901+ $121x 0.812 + $133 x 0.731+ $146.4 x 0.659 + $161 x0.593 + $1,000x0.593 = $99.11 +$98.3 + $97.2 + $96.5 + $688.5 = $1,079.6 If we try10%, we have: $1,121= $110x0.909 + $121x0.826 +$133x 0.751 + $146.4 x 0.683 + $1,161 x 0.621 =$100 +$100 + $100 + $100 +$100 +$621 = $1,121
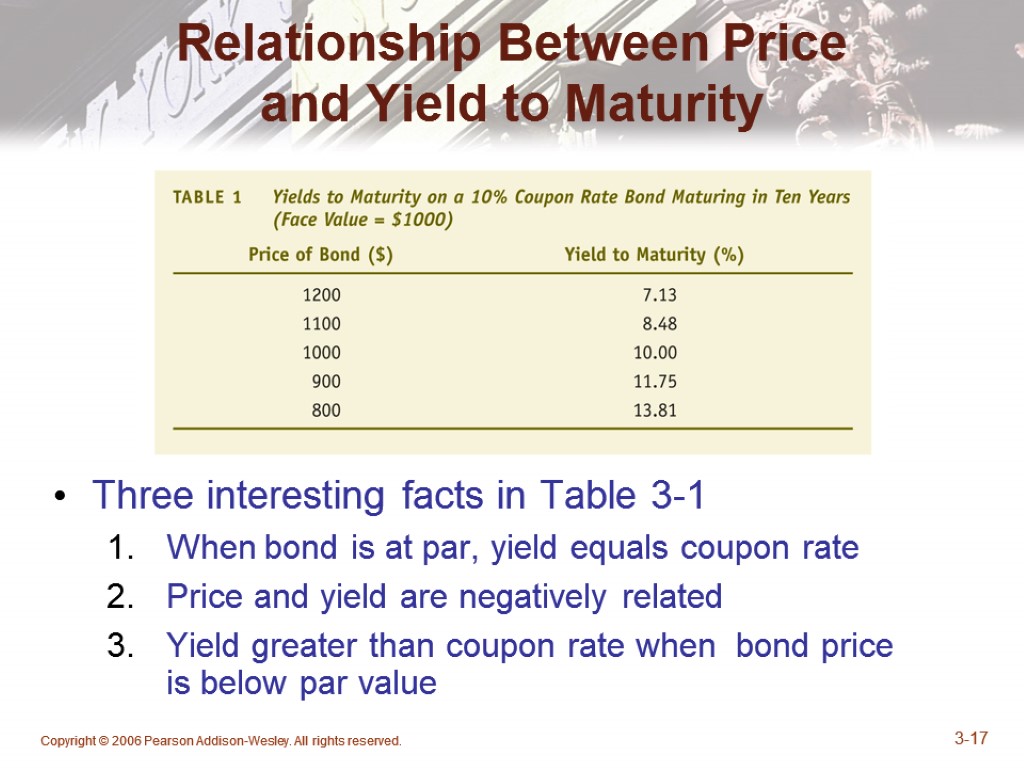
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-17 Relationship Between Price and Yield to Maturity Three interesting facts in Table 3-1 When bond is at par, yield equals coupon rate Price and yield are negatively related Yield greater than coupon rate when bond price is below par value
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-17 Relationship Between Price and Yield to Maturity Three interesting facts in Table 3-1 When bond is at par, yield equals coupon rate Price and yield are negatively related Yield greater than coupon rate when bond price is below par value
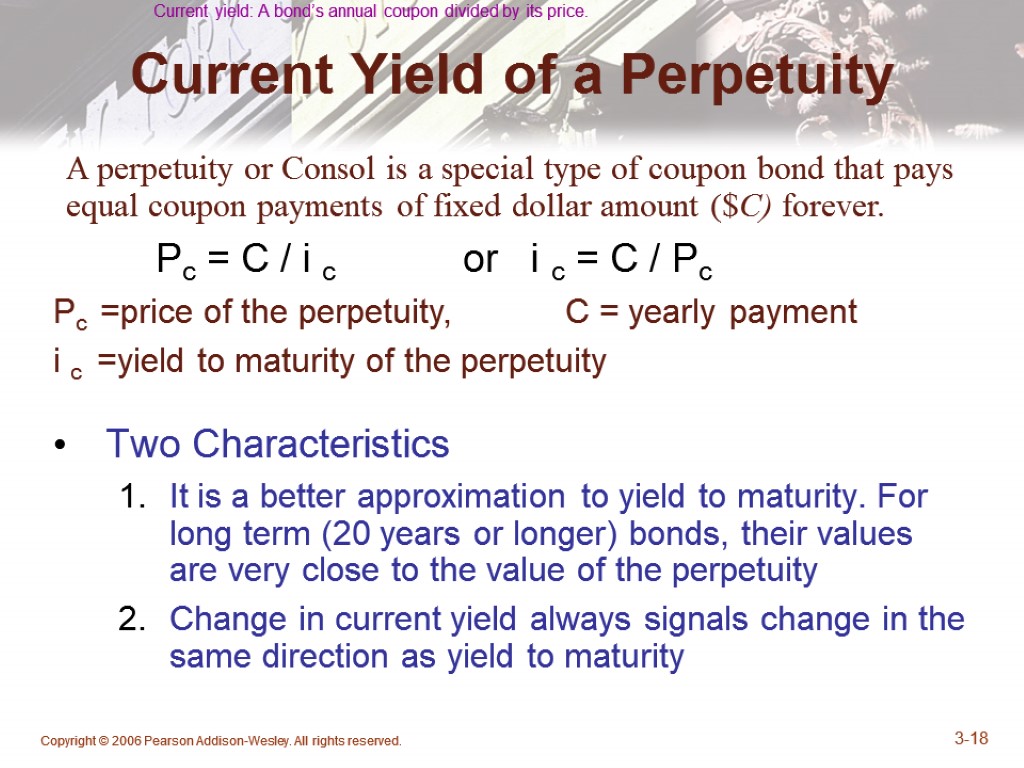
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-18 Current Yield of a Perpetuity Two Characteristics It is a better approximation to yield to maturity. For long term (20 years or longer) bonds, their values are very close to the value of the perpetuity Change in current yield always signals change in the same direction as yield to maturity A perpetuity or Consol is a special type of coupon bond that pays equal coupon payments of fixed dollar amount ($C) forever. Pc = C / i c or i c = C / Pc Pc =price of the perpetuity, C = yearly payment i c =yield to maturity of the perpetuity Current yield: A bond’s annual coupon divided by its price.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-18 Current Yield of a Perpetuity Two Characteristics It is a better approximation to yield to maturity. For long term (20 years or longer) bonds, their values are very close to the value of the perpetuity Change in current yield always signals change in the same direction as yield to maturity A perpetuity or Consol is a special type of coupon bond that pays equal coupon payments of fixed dollar amount ($C) forever. Pc = C / i c or i c = C / Pc Pc =price of the perpetuity, C = yearly payment i c =yield to maturity of the perpetuity Current yield: A bond’s annual coupon divided by its price.
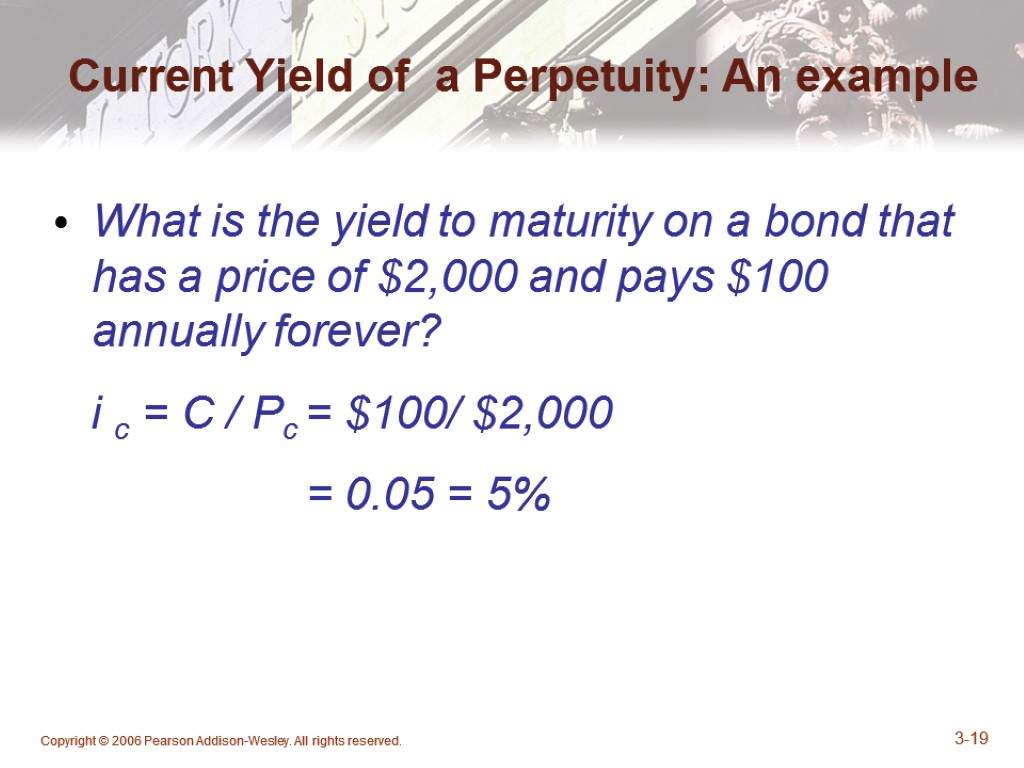
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-19 Current Yield of a Perpetuity: An example What is the yield to maturity on a bond that has a price of $2,000 and pays $100 annually forever? i c = C / Pc = $100/ $2,000 = 0.05 = 5%
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-19 Current Yield of a Perpetuity: An example What is the yield to maturity on a bond that has a price of $2,000 and pays $100 annually forever? i c = C / Pc = $100/ $2,000 = 0.05 = 5%
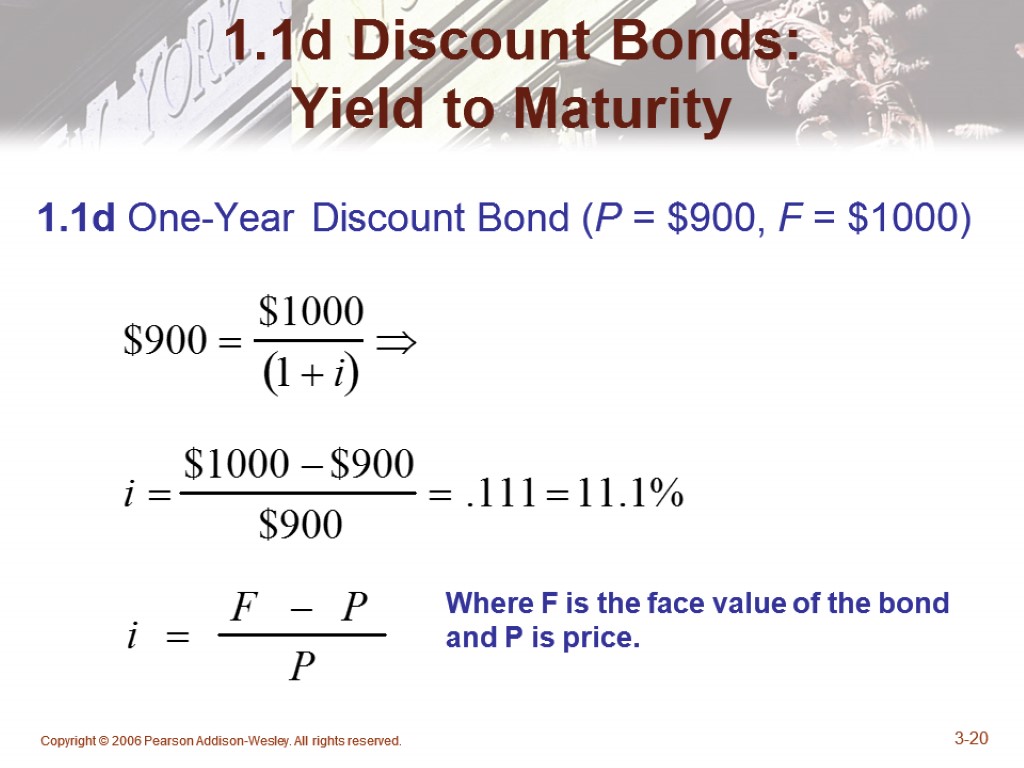
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-20 1.1d Discount Bonds: Yield to Maturity 1.1d One-Year Discount Bond (P = $900, F = $1000) Where F is the face value of the bond and P is price.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-20 1.1d Discount Bonds: Yield to Maturity 1.1d One-Year Discount Bond (P = $900, F = $1000) Where F is the face value of the bond and P is price.
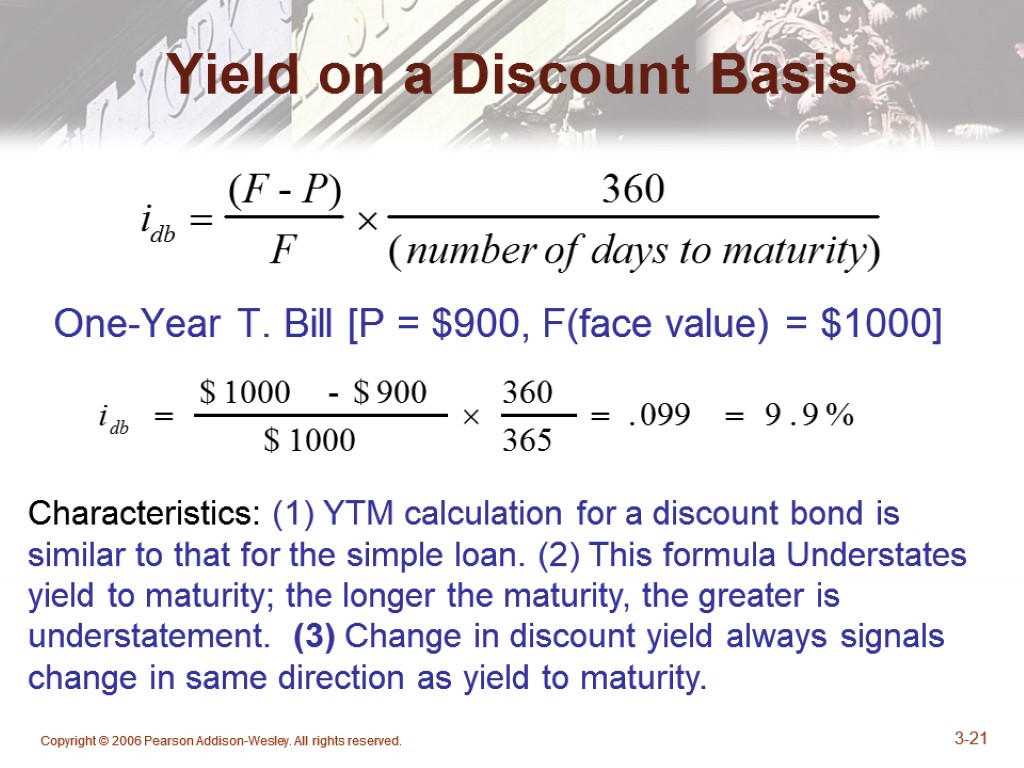
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-21 Characteristics: (1) YTM calculation for a discount bond is similar to that for the simple loan. (2) This formula Understates yield to maturity; the longer the maturity, the greater is understatement. (3) Change in discount yield always signals change in same direction as yield to maturity. Yield on a Discount Basis One-Year T. Bill [P = $900, F(face value) = $1000]
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-21 Characteristics: (1) YTM calculation for a discount bond is similar to that for the simple loan. (2) This formula Understates yield to maturity; the longer the maturity, the greater is understatement. (3) Change in discount yield always signals change in same direction as yield to maturity. Yield on a Discount Basis One-Year T. Bill [P = $900, F(face value) = $1000]
 Bond Page of the Newspaper
Bond Page of the Newspaper
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-23 2. Distinction Between Real and Nominal Interest Rates Real interest rate Interest rate that is adjusted for expected changes in the price level* Real interest rate more accurately reflects true cost of borrowing When real rate is low, greater incentives to borrow and less to lend Where i is the nominal interest rate, ir is the real interest rate and is the inflation rate.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-23 2. Distinction Between Real and Nominal Interest Rates Real interest rate Interest rate that is adjusted for expected changes in the price level* Real interest rate more accurately reflects true cost of borrowing When real rate is low, greater incentives to borrow and less to lend Where i is the nominal interest rate, ir is the real interest rate and is the inflation rate.
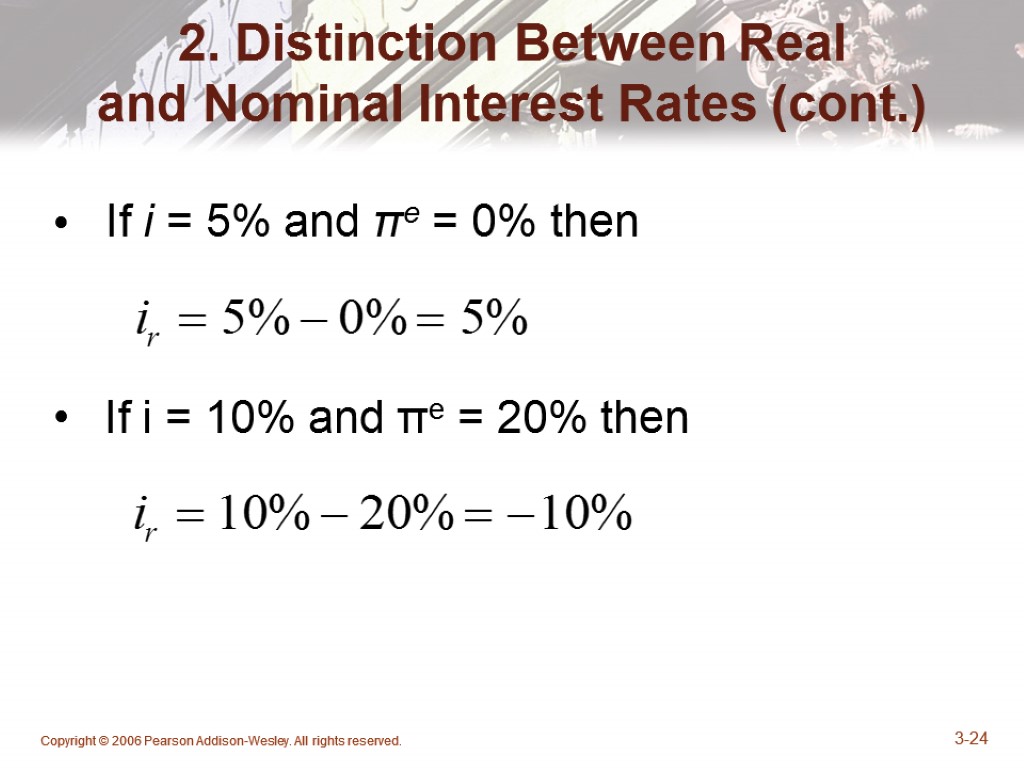
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-24 2. Distinction Between Real and Nominal Interest Rates (cont.) If i = 5% and πe = 0% then If i = 10% and πe = 20% then
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-24 2. Distinction Between Real and Nominal Interest Rates (cont.) If i = 5% and πe = 0% then If i = 10% and πe = 20% then
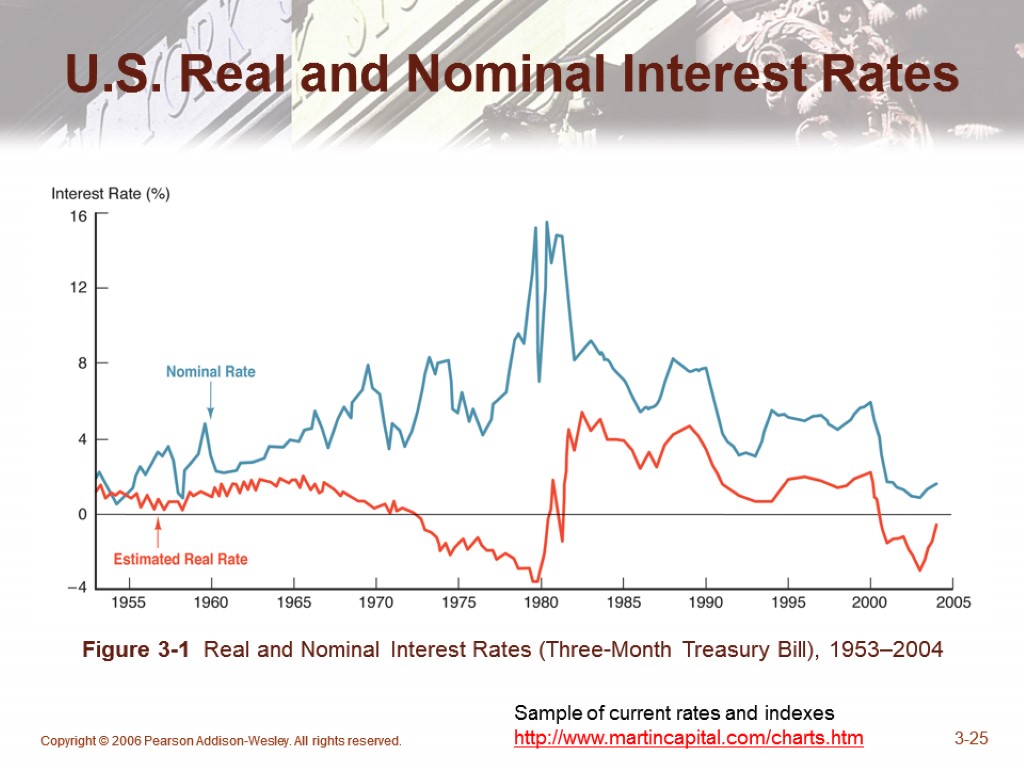
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-25 U.S. Real and Nominal Interest Rates Figure 3-1 Real and Nominal Interest Rates (Three-Month Treasury Bill), 1953–2004 Sample of current rates and indexes http://www.martincapital.com/charts.htm
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-25 U.S. Real and Nominal Interest Rates Figure 3-1 Real and Nominal Interest Rates (Three-Month Treasury Bill), 1953–2004 Sample of current rates and indexes http://www.martincapital.com/charts.htm
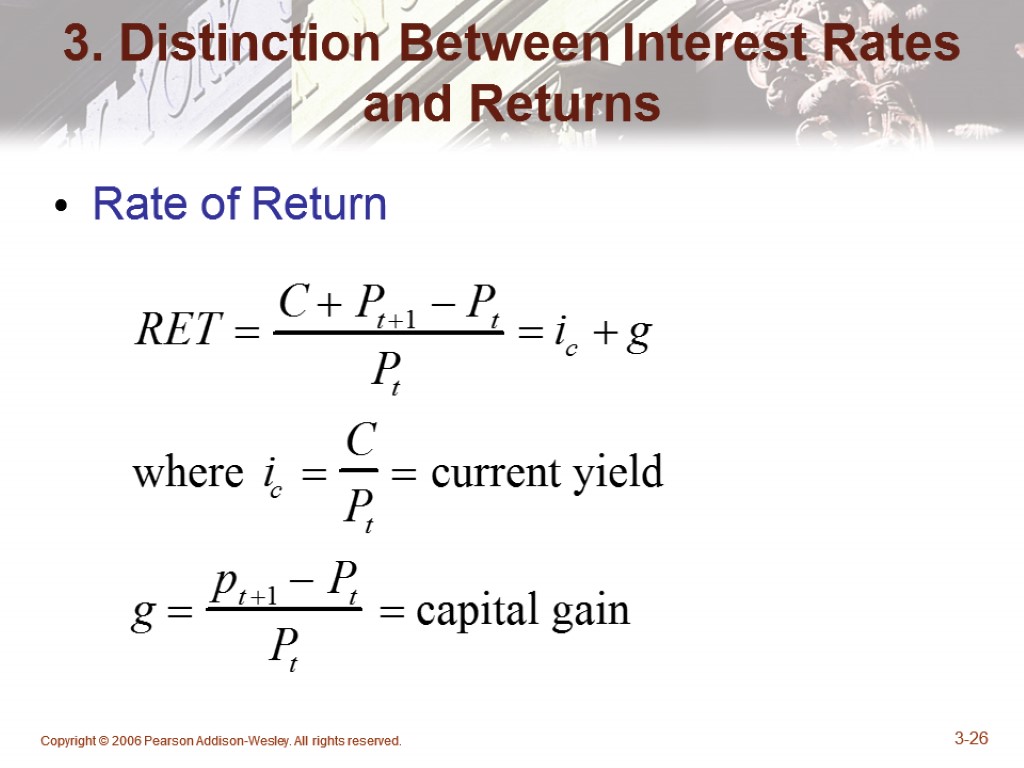
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-26 3. Distinction Between Interest Rates and Returns Rate of Return
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-26 3. Distinction Between Interest Rates and Returns Rate of Return
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-27 3.1 Key Facts about the Relationship Between Rates and Returns Sample of current coupon rates and yields on government bonds http://www.bloomberg.com/markets/iyc.html
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-27 3.1 Key Facts about the Relationship Between Rates and Returns Sample of current coupon rates and yields on government bonds http://www.bloomberg.com/markets/iyc.html
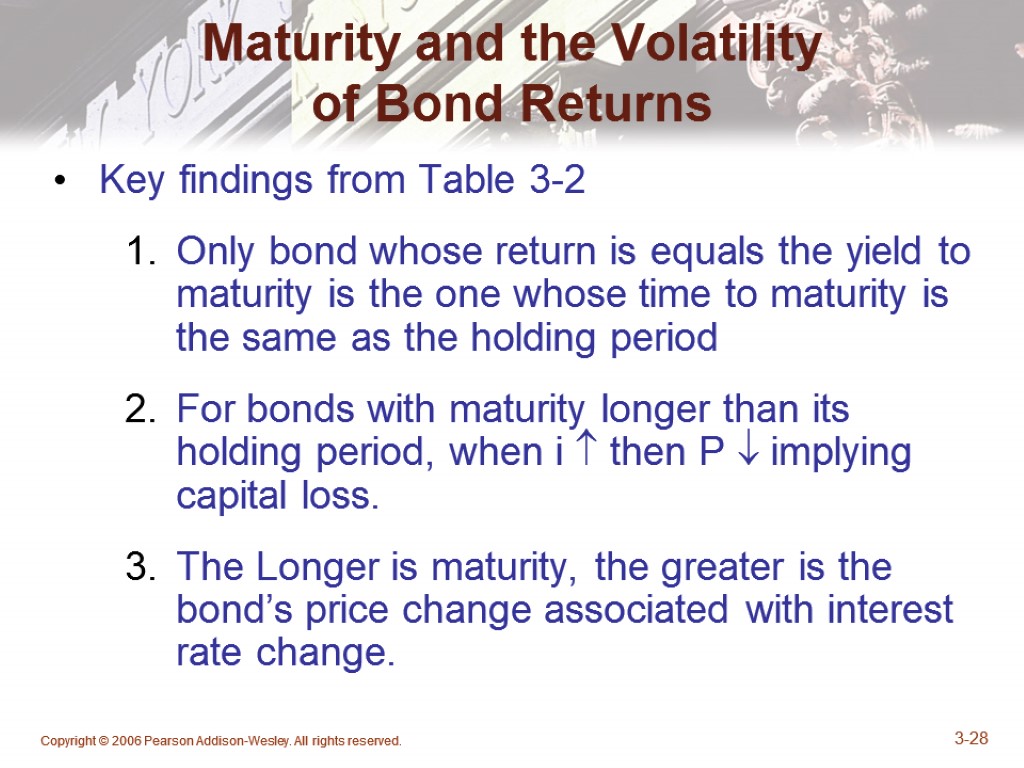
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-28 Maturity and the Volatility of Bond Returns Key findings from Table 3-2 Only bond whose return is equals the yield to maturity is the one whose time to maturity is the same as the holding period For bonds with maturity longer than its holding period, when i then P implying capital loss. The Longer is maturity, the greater is the bond’s price change associated with interest rate change.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-28 Maturity and the Volatility of Bond Returns Key findings from Table 3-2 Only bond whose return is equals the yield to maturity is the one whose time to maturity is the same as the holding period For bonds with maturity longer than its holding period, when i then P implying capital loss. The Longer is maturity, the greater is the bond’s price change associated with interest rate change.
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-29 Maturity and the Volatility of Bond Returns (cont.) Key findings from Table 3-2 (continued) The longer is a bond to its maturity, the lower the rate of return associated with the increase in the interest rate. Bond with high initial interest rate can still have negative return if i See p. 58 Textbook
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-29 Maturity and the Volatility of Bond Returns (cont.) Key findings from Table 3-2 (continued) The longer is a bond to its maturity, the lower the rate of return associated with the increase in the interest rate. Bond with high initial interest rate can still have negative return if i See p. 58 Textbook
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-30 Maturity and the Volatility of Bond Returns (cont.) Conclusion from Table 3-2 analysis Prices and returns more volatile for long-term bonds because have higher interest-rate risk No interest-rate risk for any bond whose maturity equals holding period
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-30 Maturity and the Volatility of Bond Returns (cont.) Conclusion from Table 3-2 analysis Prices and returns more volatile for long-term bonds because have higher interest-rate risk No interest-rate risk for any bond whose maturity equals holding period
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-31 Reinvestment Risk Occurs if an investor’s holding period is longer than the term to maturity of the bond, since the i at the time of reinvestment is uncertain. If the holding period is longer than the term to maturity of the bond, then (2.1) the investor gain from i , (2.2) lose when i
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-31 Reinvestment Risk Occurs if an investor’s holding period is longer than the term to maturity of the bond, since the i at the time of reinvestment is uncertain. If the holding period is longer than the term to maturity of the bond, then (2.1) the investor gain from i , (2.2) lose when i
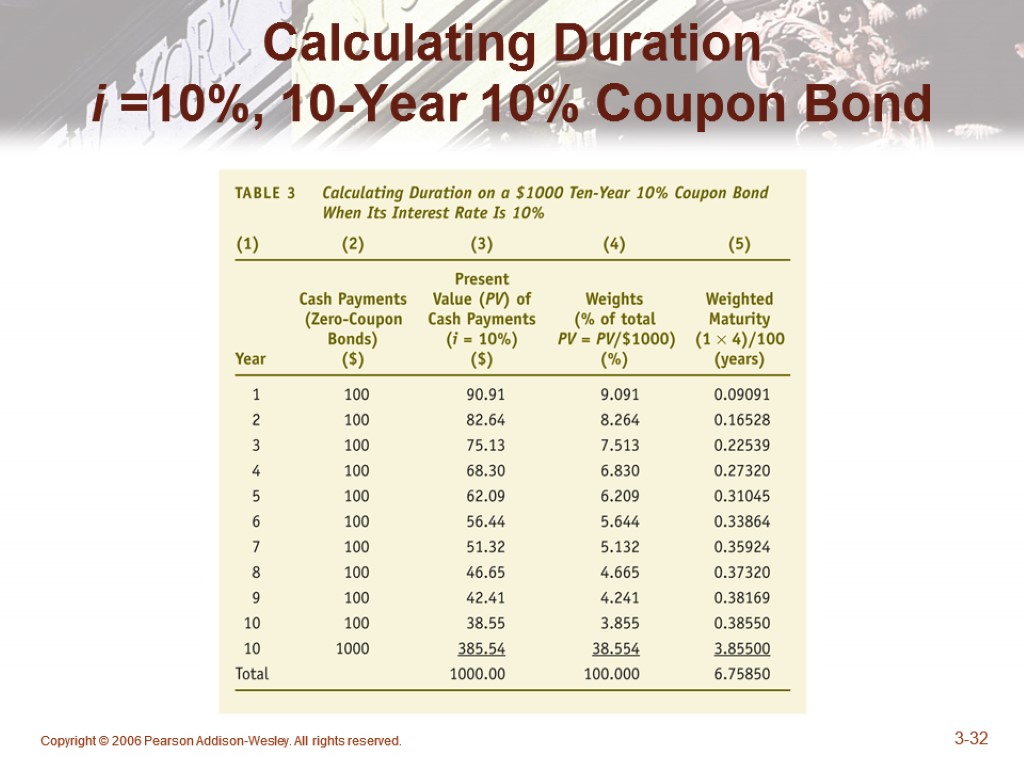
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-32 Calculating Duration i =10%, 10-Year 10% Coupon Bond
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-32 Calculating Duration i =10%, 10-Year 10% Coupon Bond
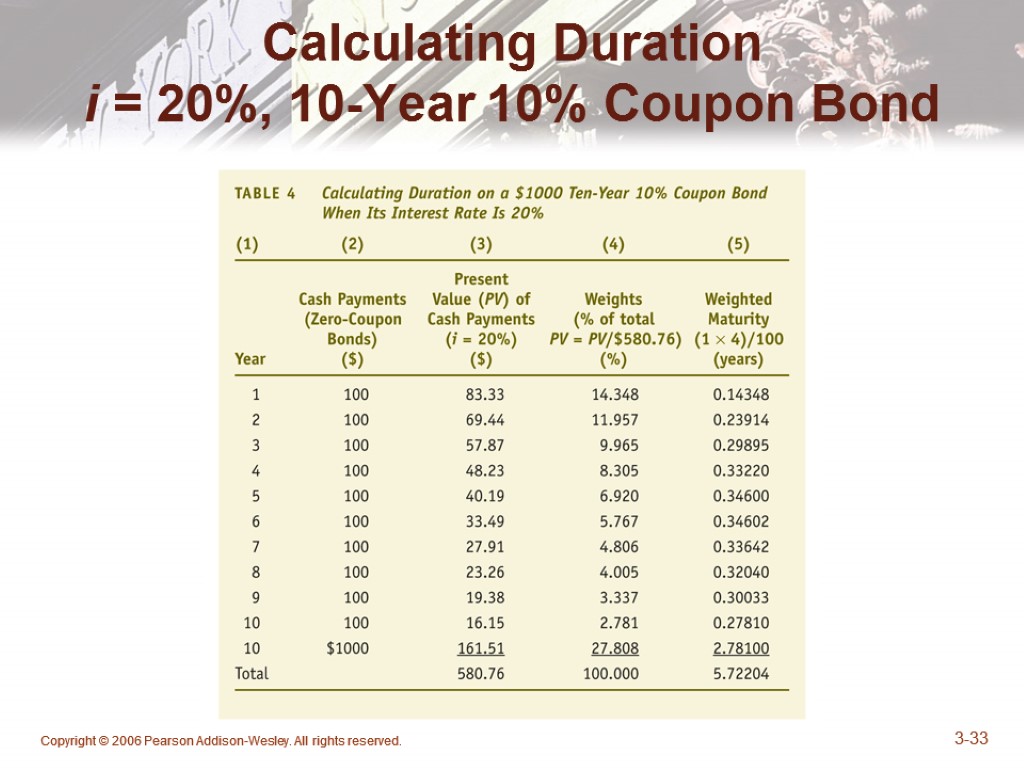
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-33 Calculating Duration i = 20%, 10-Year 10% Coupon Bond
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-33 Calculating Duration i = 20%, 10-Year 10% Coupon Bond
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-34 Formula for Duration Key facts about duration All else equal, when the maturity of a bond lengthens, the duration rises as well All else equal, when interest rates rise, the duration of a coupon bond fall
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-34 Formula for Duration Key facts about duration All else equal, when the maturity of a bond lengthens, the duration rises as well All else equal, when interest rates rise, the duration of a coupon bond fall
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-35 Formula for Duration The higher is the coupon rate on the bond, the shorter is the duration of the bond Duration is additive: the duration of a portfolio of securities is the weighted-average of the durations of the individual securities, with the weights equaling the proportion of the portfolio invested in each
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-35 Formula for Duration The higher is the coupon rate on the bond, the shorter is the duration of the bond Duration is additive: the duration of a portfolio of securities is the weighted-average of the durations of the individual securities, with the weights equaling the proportion of the portfolio invested in each
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-36 Duration and Interest-Rate Risk i 10% to 11%: Table 3-4, 10% coupon bond
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-36 Duration and Interest-Rate Risk i 10% to 11%: Table 3-4, 10% coupon bond
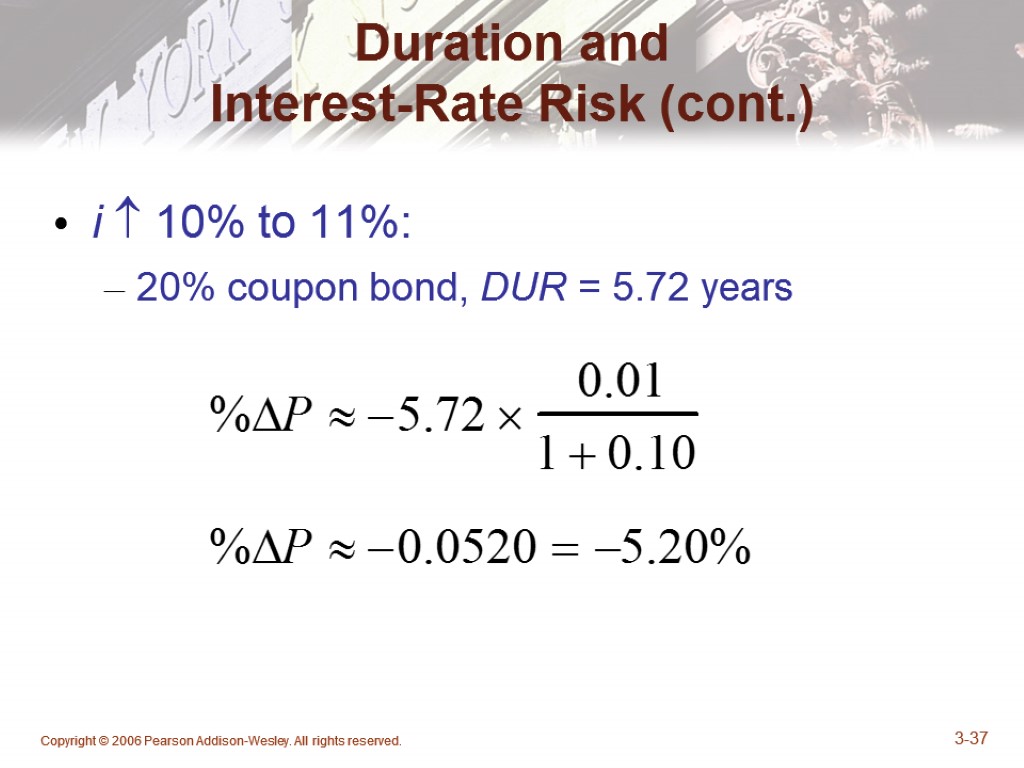
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-37 Duration and Interest-Rate Risk (cont.) i 10% to 11%: 20% coupon bond, DUR = 5.72 years
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-37 Duration and Interest-Rate Risk (cont.) i 10% to 11%: 20% coupon bond, DUR = 5.72 years
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-38 The greater is the duration of a security, the greater is the percentage change in the market value of the security for a given change in interest rates Therefore, the greater is the duration of a security, the greater is its interest-rate risk Duration and Interest-Rate Risk (cont.)
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-38 The greater is the duration of a security, the greater is the percentage change in the market value of the security for a given change in interest rates Therefore, the greater is the duration of a security, the greater is its interest-rate risk Duration and Interest-Rate Risk (cont.)
 Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-39 Summary Measuring Interest Rates: We examined several techniques for measuring the interest rate required on debt instruments. The Distinction Between Real and Nominal Interest Rates: We examined the meaning of interest in the context of price inflation. The Distinction Between Interest Rates and Returns: We examined what each means and how they should be viewed for asset valuation.
Copyright © 2006 Pearson Addison-Wesley. All rights reserved. 3-39 Summary Measuring Interest Rates: We examined several techniques for measuring the interest rate required on debt instruments. The Distinction Between Real and Nominal Interest Rates: We examined the meaning of interest in the context of price inflation. The Distinction Between Interest Rates and Returns: We examined what each means and how they should be viewed for asset valuation.

