Chapter 24 Alternating Current Circuits Unlike a battery

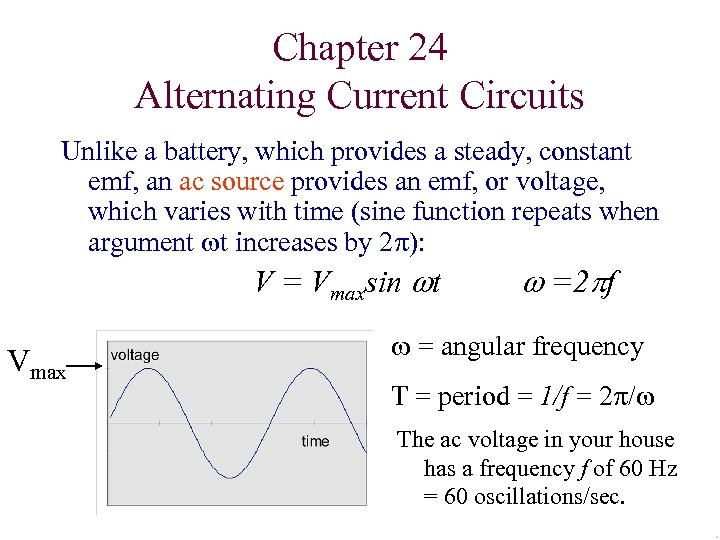
Chapter 24 Alternating Current Circuits Unlike a battery, which provides a steady, constant emf, an ac source provides an emf, or voltage, which varies with time (sine function repeats when argument wt increases by 2 p): V = Vmaxsin wt Vmax w =2 pf w = angular frequency T = period = 1/f = 2 p/w The ac voltage in your house has a frequency f of 60 Hz = 60 oscillations/sec.

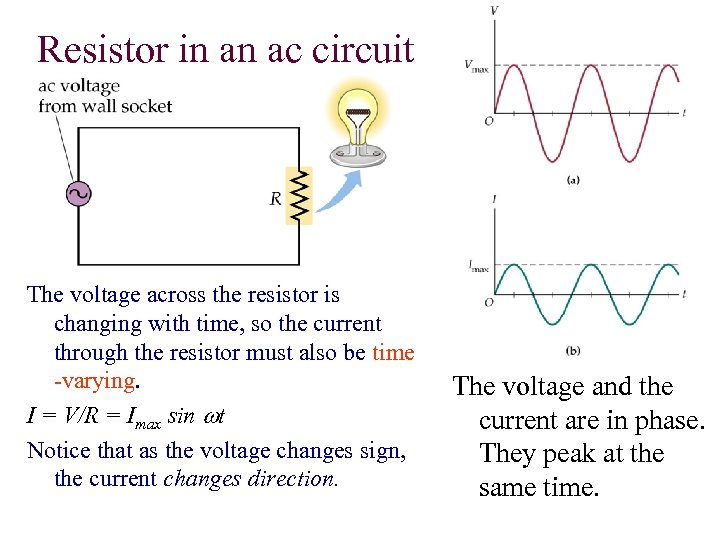
Resistor in an ac circuit The voltage across the resistor is changing with time, so the current through the resistor must also be time -varying. I = V/R = Imax sin wt Notice that as the voltage changes sign, the current changes direction. The voltage and the current are in phase. They peak at the same time.

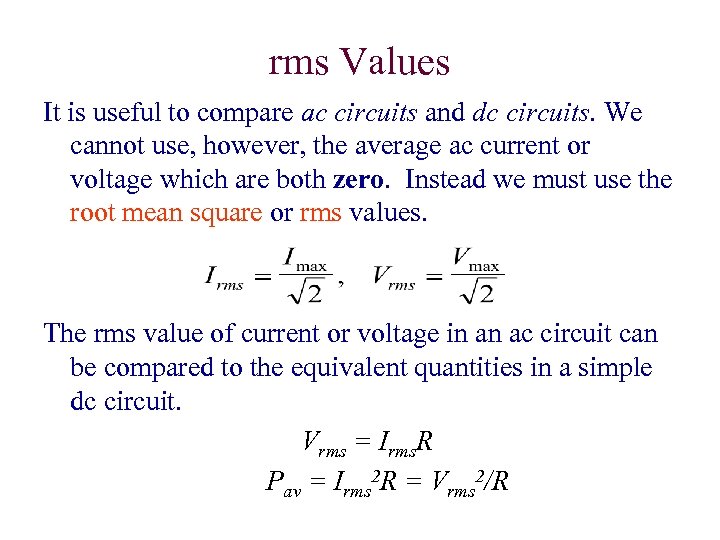
rms Values It is useful to compare ac circuits and dc circuits. We cannot use, however, the average ac current or voltage which are both zero. Instead we must use the root mean square or rms values. The rms value of current or voltage in an ac circuit can be compared to the equivalent quantities in a simple dc circuit. Vrms = Irms. R Pav = Irms 2 R = Vrms 2/R

Root Mean Square Voltage and Current Vrms = Square root of the mean (average) of V-squared. Average AC Power consumed by a resistor independent of form of V vs t curve

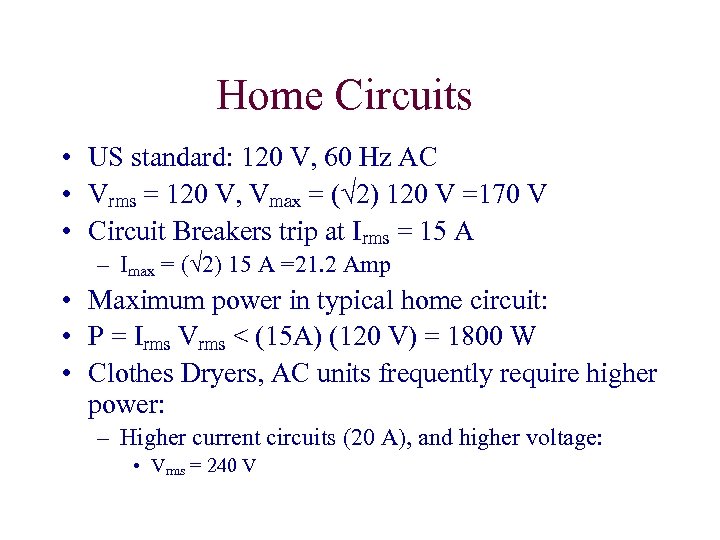
Home Circuits • US standard: 120 V, 60 Hz AC • Vrms = 120 V, Vmax = ( 2) 120 V =170 V • Circuit Breakers trip at Irms = 15 A – Imax = ( 2) 15 A =21. 2 Amp • Maximum power in typical home circuit: • P = Irms Vrms < (15 A) (120 V) = 1800 W • Clothes Dryers, AC units frequently require higher power: – Higher current circuits (20 A), and higher voltage: • Vrms = 240 V

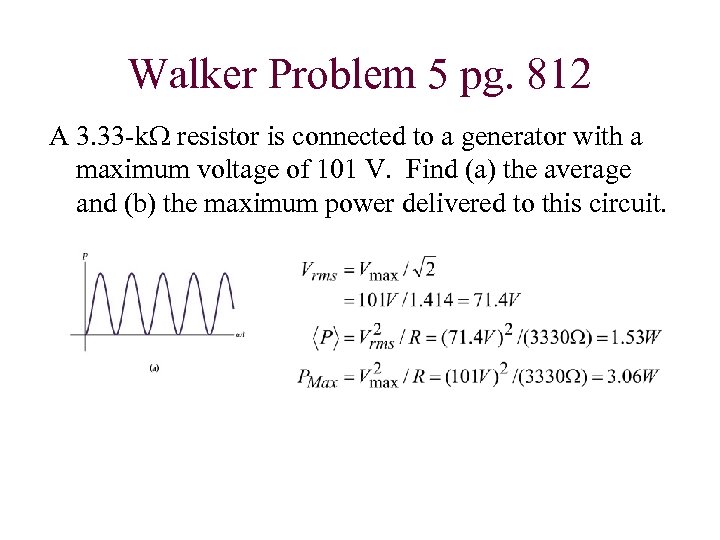
Walker Problem 5 pg. 812 A 3. 33 -k. W resistor is connected to a generator with a maximum voltage of 101 V. Find (a) the average and (b) the maximum power delivered to this circuit.

Capacitors in ac circuits The “resistance” of the capacitor to current in the circuit is known as capacitive reactance XC = 1/(w. C) SI unit of reactance: Ohm (W) = s/F Vrms = Irms. XC OR Vmax = Imax. XC The voltage lags behind the current by 90°. V=Q/C: When I>0, capacitor is charging, When I<0, capacitor is dis-charging. The average power consumed by a capacitor in an ac circuit is zero. Every ½ cycle capacitor stockpiles energy; During next ½ cycle capacitor disharges energy to circuit.

Walker Problem 14, pg. 813 An rms voltage of 10. 0 V with a frequency of 1. 00 k. Hz is applied to a 0. 395 -m. F capacitor. (a) What is the rms current in this circuit? (b) By what factor does the current change if the frequency of the voltage is doubled? (c) Calculate the current for a frequency of 2. 00 k. Hz. If frequency is doubled, XC drops by factor of 2, Current is doubled: Irms = 49. 6 m. A @2. 00 k. Hz

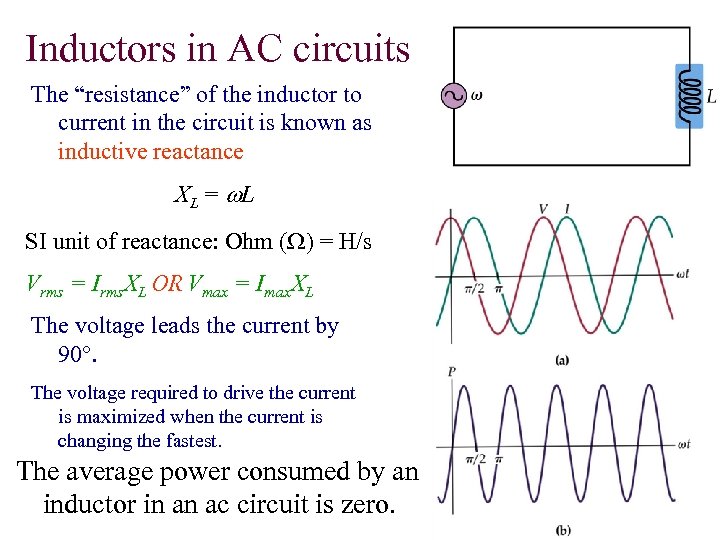
Inductors in AC circuits The “resistance” of the inductor to current in the circuit is known as inductive reactance X L = w. L SI unit of reactance: Ohm (W) = H/s Vrms = Irms. XL OR Vmax = Imax. XL The voltage leads the current by 90°. The voltage required to drive the current is maximized when the current is changing the fastest. The average power consumed by an inductor in an ac circuit is zero.

Walker Problem 29, pg. 813 What is the rms current in a 125 -m. H inductor when it is connected to a 60. 0 -Hz generator with an rms voltage of 115 Hz?

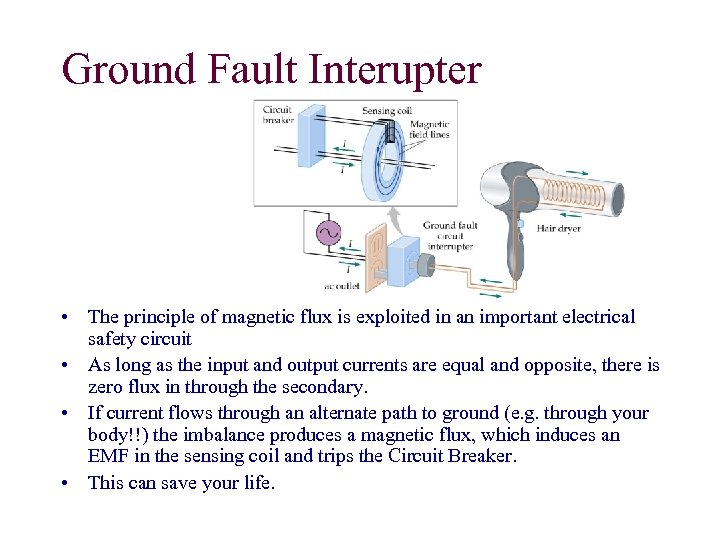
Ground Fault Interupter • The principle of magnetic flux is exploited in an important electrical safety circuit • As long as the input and output currents are equal and opposite, there is zero flux in through the secondary. • If current flows through an alternate path to ground (e. g. through your body!!) the imbalance produces a magnetic flux, which induces an EMF in the sensing coil and trips the Circuit Breaker. • This can save your life.

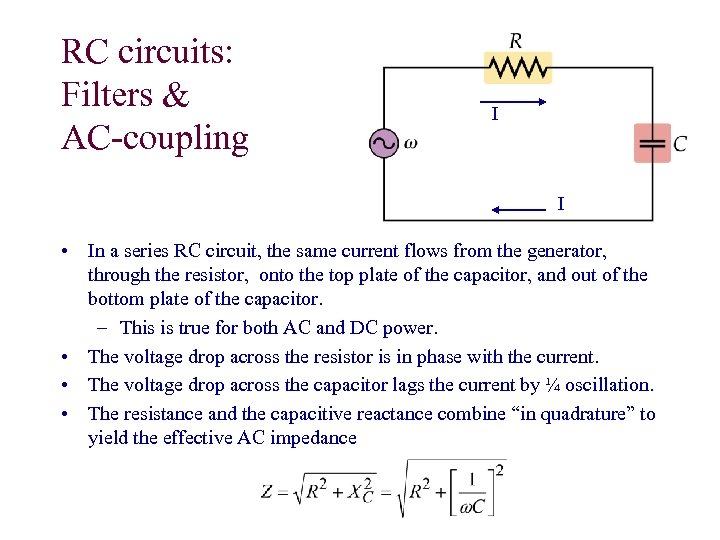
RC circuits: Filters & AC-coupling I I • In a series RC circuit, the same current flows from the generator, through the resistor, onto the top plate of the capacitor, and out of the bottom plate of the capacitor. – This is true for both AC and DC power. • The voltage drop across the resistor is in phase with the current. • The voltage drop across the capacitor lags the current by ¼ oscillation. • The resistance and the capacitive reactance combine “in quadrature” to yield the effective AC impedance

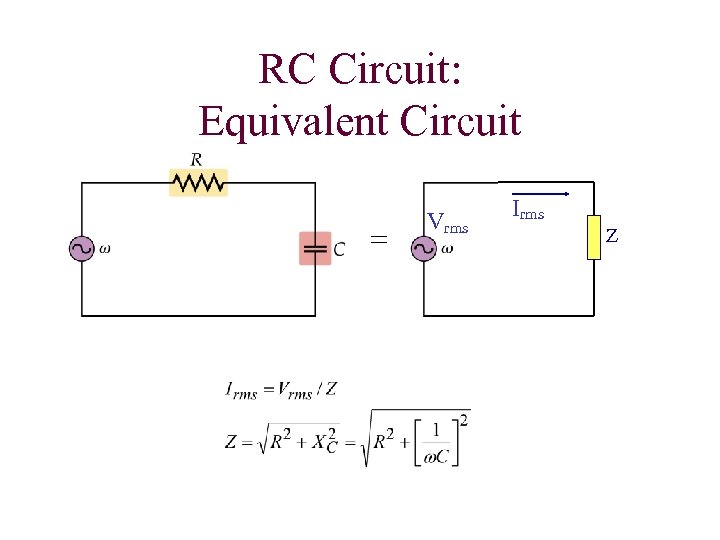
RC Circuit: Equivalent Circuit = Vrms Irms Z

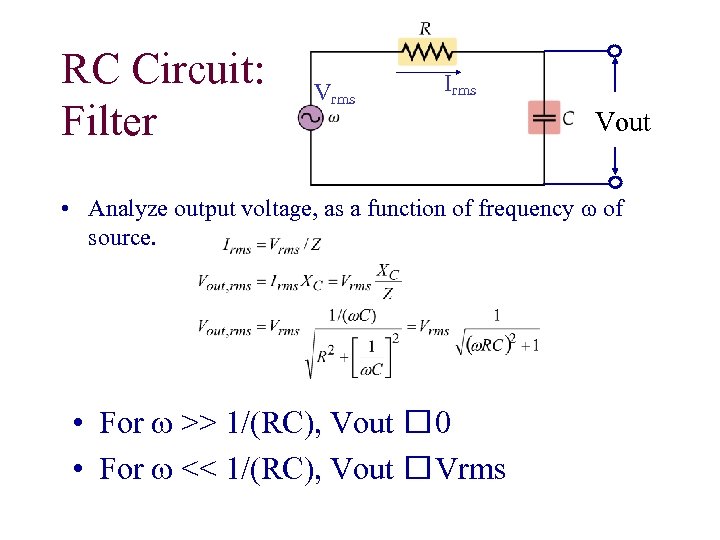
RC Circuit: Filter Vrms Irms Vout • Analyze output voltage, as a function of frequency w of source. • For w >> 1/(RC), Vout 0 • For w << 1/(RC), Vout Vrms

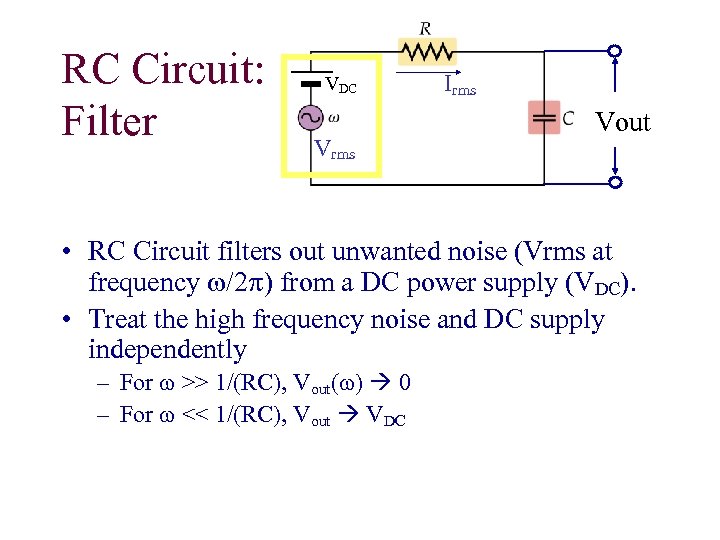
RC Circuit: Filter VDC Vrms Irms Vout • RC Circuit filters out unwanted noise (Vrms at frequency w/2 p) from a DC power supply (VDC). • Treat the high frequency noise and DC supply independently – For w >> 1/(RC), Vout(w) 0 – For w << 1/(RC), Vout VDC

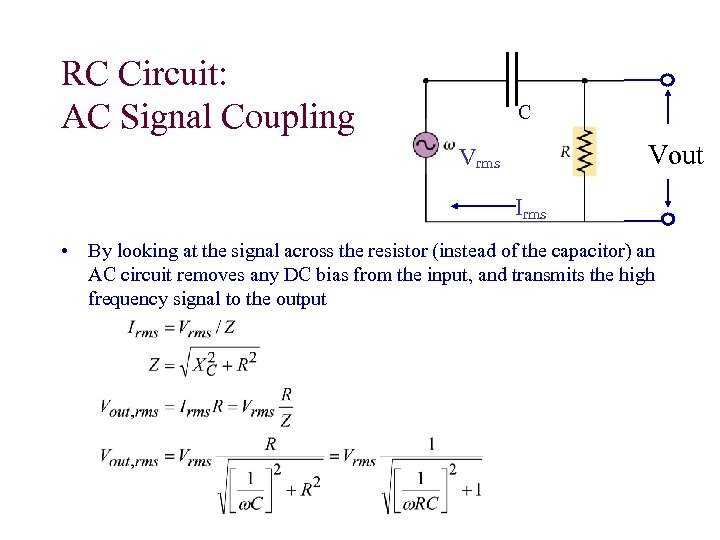
RC Circuit: AC Signal Coupling C Vout Vrms Irms • By looking at the signal across the resistor (instead of the capacitor) an AC circuit removes any DC bias from the input, and transmits the high frequency signal to the output

AC Coupling C Vout Vrms Irms • At high frequency, w>>1/(RC), the capacitor acts as a short circuit, Vout = Vrms • At low frequency w << 1/(RC), the capacitor acts as an open circuit, Vout 0

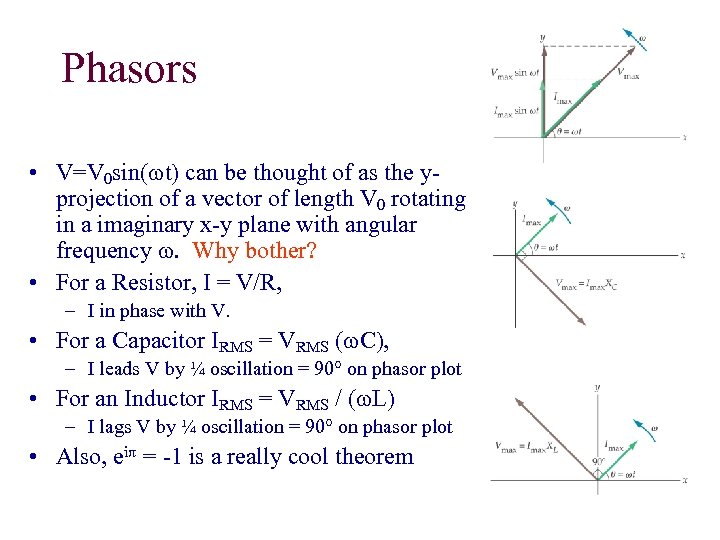
Phasors • V=V 0 sin(wt) can be thought of as the yprojection of a vector of length V 0 rotating in a imaginary x-y plane with angular frequency w. Why bother? • For a Resistor, I = V/R, – I in phase with V. • For a Capacitor IRMS = VRMS (w. C), – I leads V by ¼ oscillation = 90° on phasor plot • For an Inductor IRMS = VRMS / (w. L) – I lags V by ¼ oscillation = 90° on phasor plot • Also, eip = -1 is a really cool theorem

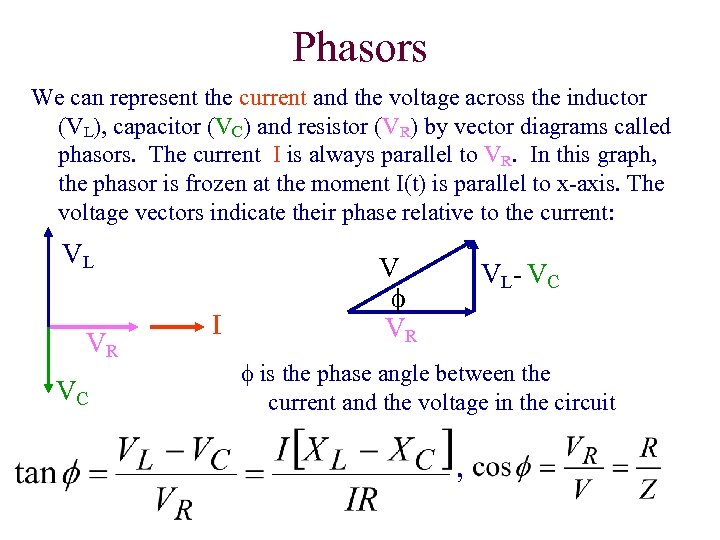
Phasors We can represent the current and the voltage across the inductor (VL), capacitor (VC) and resistor (VR) by vector diagrams called phasors. The current I is always parallel to VR. In this graph, the phasor is frozen at the moment I(t) is parallel to x-axis. The voltage vectors indicate their phase relative to the current: VL VR VC I V f VR VL - VC f is the phase angle between the current and the voltage in the circuit ,

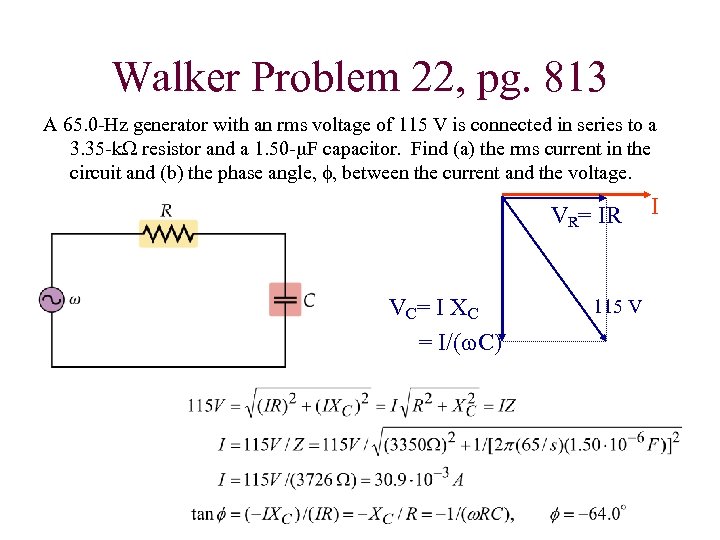
Walker Problem 22, pg. 813 A 65. 0 -Hz generator with an rms voltage of 115 V is connected in series to a 3. 35 -k. W resistor and a 1. 50 -m. F capacitor. Find (a) the rms current in the circuit and (b) the phase angle, f, between the current and the voltage. VR= IR VC = I X C = I/(w. C) 115 V I

RC Circuit, Alternating Current I(t) erms • • • VR = I(t) R VC = Q(t)/C VR, rms= Irms R VC, rms = Irms /(w. C) erms = Irms Z Z = [R 2+XC 2]1/2

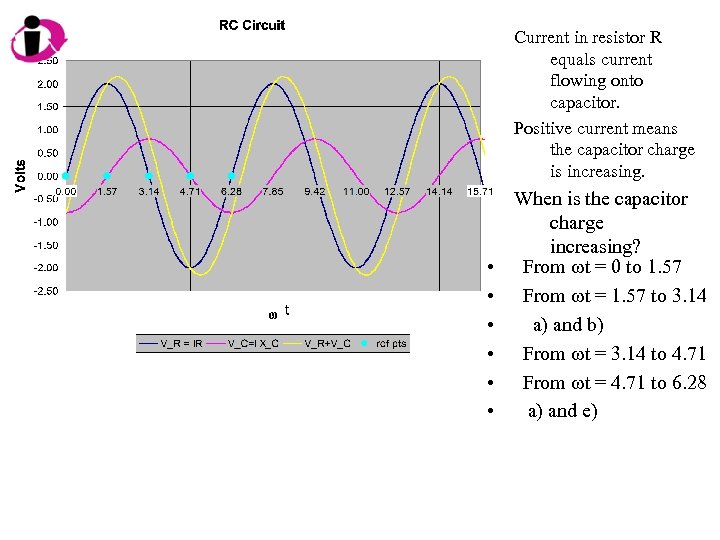
Current in resistor R equals current flowing onto capacitor. Positive current means the capacitor charge is increasing. • • • When is the capacitor charge increasing? From wt = 0 to 1. 57 From wt = 1. 57 to 3. 14 a) and b) From wt = 3. 14 to 4. 71 From wt = 4. 71 to 6. 28 a) and e)

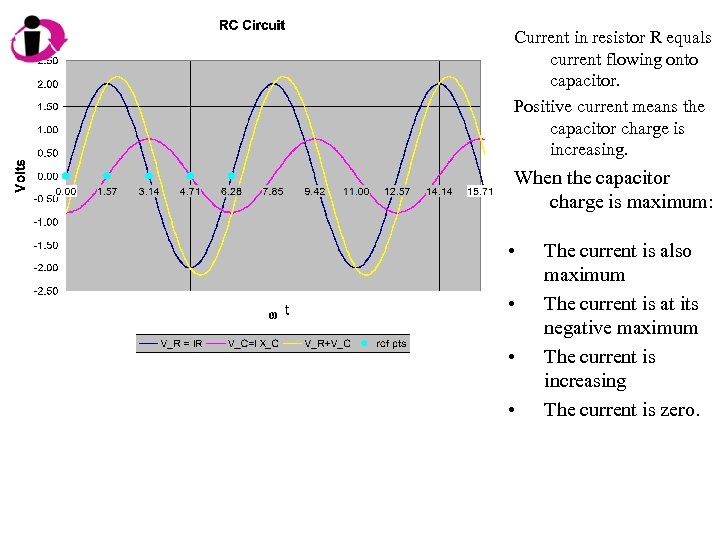
Current in resistor R equals current flowing onto capacitor. Positive current means the capacitor charge is increasing. When the capacitor charge is maximum: • • The current is also maximum The current is at its negative maximum The current is increasing The current is zero.

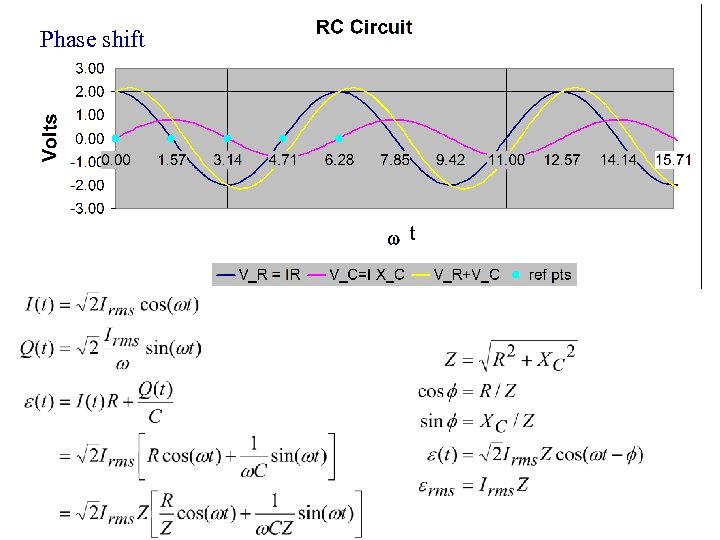
Phase shift

VR VC e VR VR VC e e VC

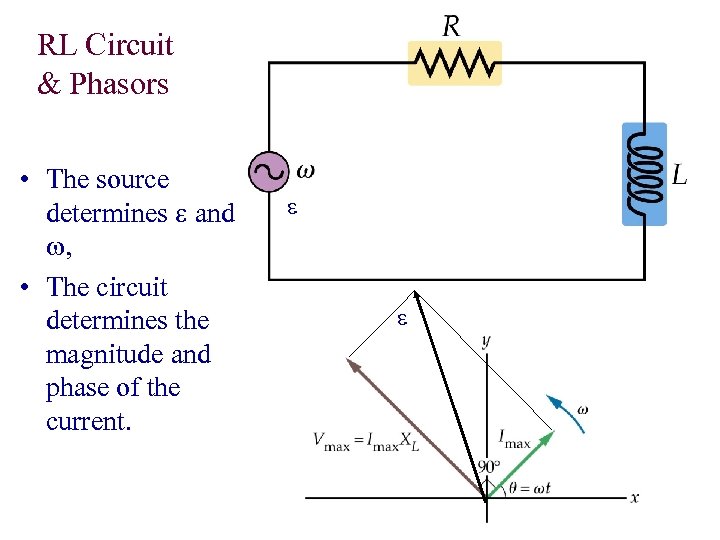
RL Circuit & Phasors • The source determines e and w, • The circuit determines the magnitude and phase of the current. e e

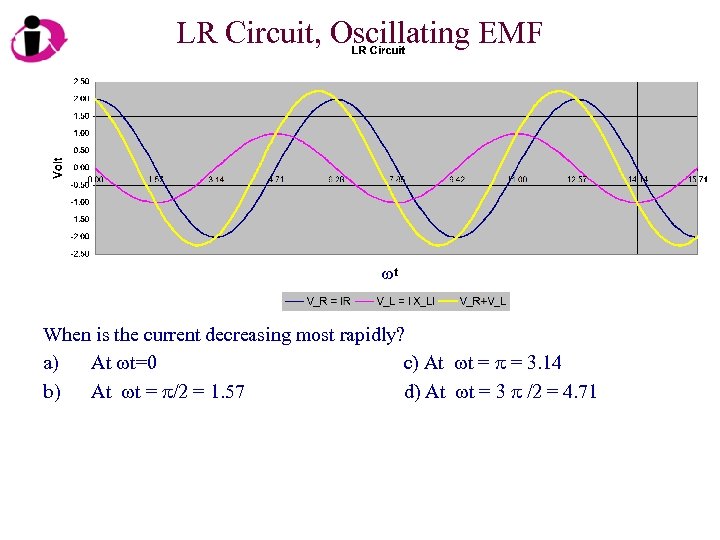
LR Circuit, Oscillating EMF w When is the current decreasing most rapidly? a) At wt=0 c) At wt = p = 3. 14 b) At wt = p/2 = 1. 57 d) At wt = 3 p /2 = 4. 71

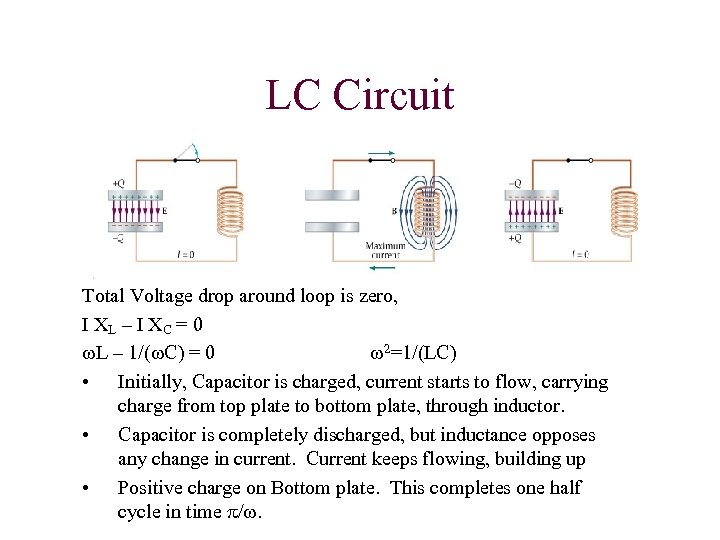
LC Circuit Total Voltage drop around loop is zero, I XL – I XC = 0 w. L – 1/(w. C) = 0 w 2=1/(LC) • Initially, Capacitor is charged, current starts to flow, carrying charge from top plate to bottom plate, through inductor. • Capacitor is completely discharged, but inductance opposes any change in current. Current keeps flowing, building up • Positive charge on Bottom plate. This completes one half cycle in time p/w.

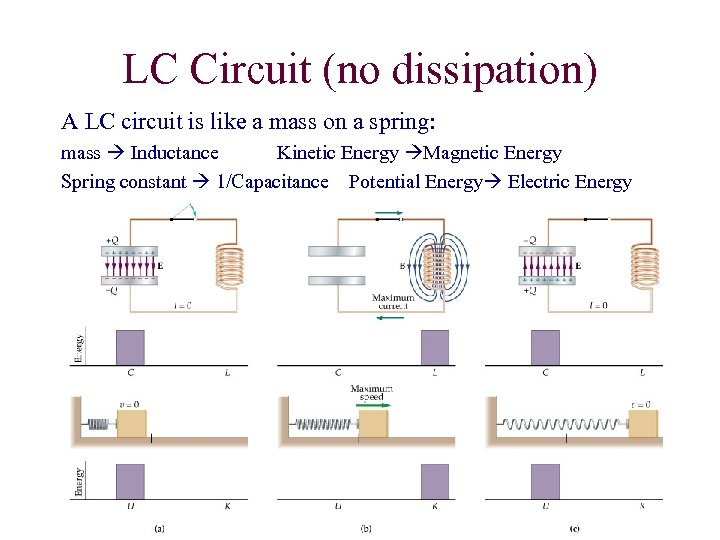
LC Circuit (no dissipation) A LC circuit is like a mass on a spring: mass Inductance Kinetic Energy Magnetic Energy Spring constant 1/Capacitance Potential Energy Electric Energy

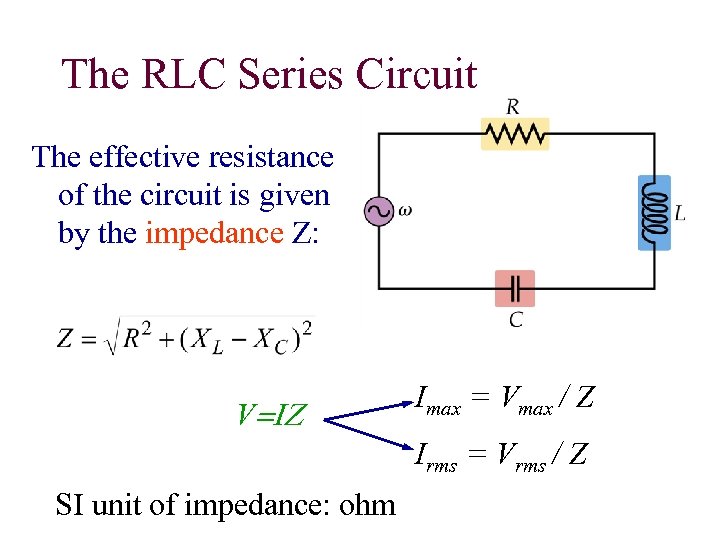
The RLC Series Circuit The effective resistance of the circuit is given by the impedance Z: V=IZ Imax = Vmax / Z Irms = Vrms / Z SI unit of impedance: ohm

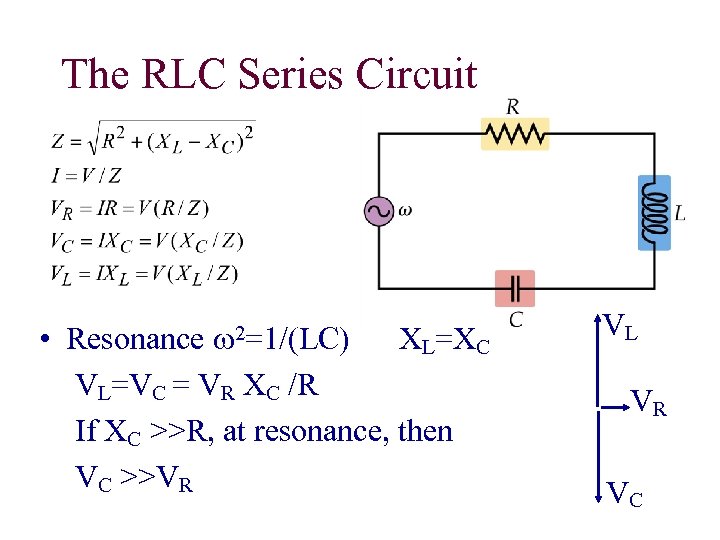
The RLC Series Circuit w 2=1/(LC) • Resonance XL=XC VL=VC = VR XC /R If XC >>R, at resonance, then VC >>VR VL VR VC

Walker Problem 40, pg. 814 Find the rms voltage across each element in an RLC circuit with R = 9. 9 k. W, C = 0. 15 m. F, and L = 25 m. H. The generator supplies an rms voltage of 115 V at a frequency of 60. 0 Hz. (b) What is the phase angle between the voltage and the current in the circuit?

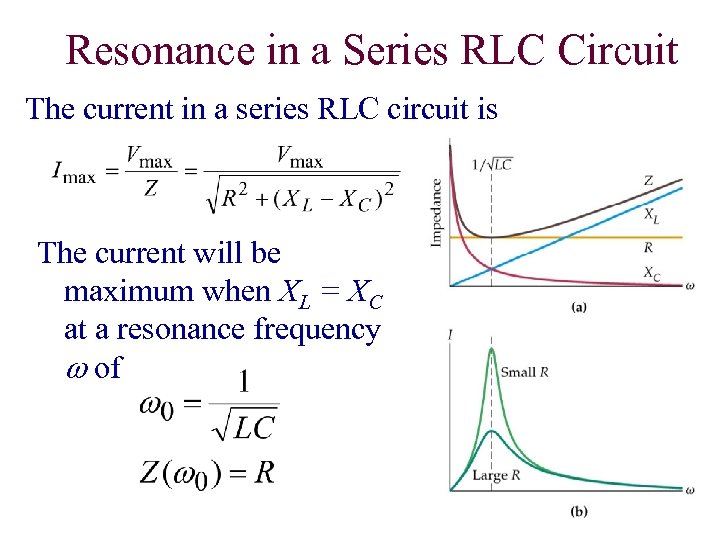
Resonance in a Series RLC Circuit The current in a series RLC circuit is The current will be maximum when XL = XC at a resonance frequency w of

Energy Storage at Resonance • Energy in Inductor § EL= (1/2) L(Irms)2 • Energy lost in one cycle § ER= PT = (Irms)2 R(2 p/w) = (1/2) L(Irms)2 • [Energy Stored]/[Energy dissipated in one cycle] § § § Oscillator ‘Q-value’ = EL / ER= Lw/(4 p. R) At Resonance w = 1/(LC)1/2 ‘Q-Value’ = (L/C)1/2 /(4 p. R) Jefferson Lab `Q-Value’=109 RLC oscillator in lab, L = 0. 010 H, R=15 W, C = 1. 00 e-4 F ‘Q-Value’ = (0. 01 H/0. 0001 F) 1/2 /(4 p 15 W) = (10 W)/(15 W 4 p) = 0. 4 § Resonance frequency = 1/[2 p(LC)1/2] = 160 Hz

Jefferson Lab Accelerator • RLC Circuit: f = 1. 5 GHz – Free oscillation decays by factor e=2. 718 in 109 oscillations – “Like a church bell that rings for a month”: (109)/(440/sec) =26 days